Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 108068 by I want to learn more last updated on 14/Aug/20

Answered by Sarah85 last updated on 14/Aug/20

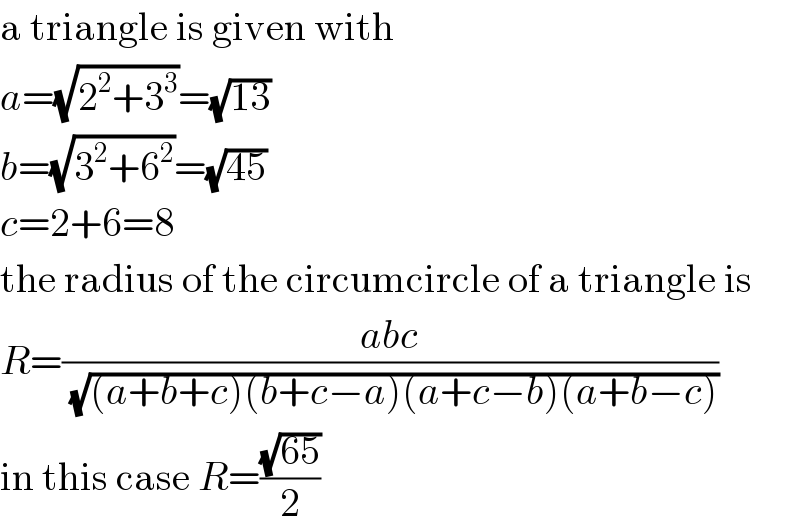
a triangle is given with a=(√(2^2 +3^3 ))=(√(13)) b=(√(3^2 +6^2 ))=(√(45)) c=2+6=8 the radius of the circumcircle of a triangle is R=((abc)/( (√((a+b+c)(b+c−a)(a+c−b)(a+b−c))))) in this case R=((√(65))/2)

$$\mathrm{a}\:\mathrm{triangle}\:\mathrm{is}\:\mathrm{given}\:\mathrm{with} \\ $$$${a}=\sqrt{\mathrm{2}^{\mathrm{2}} +\mathrm{3}^{\mathrm{3}} }=\sqrt{\mathrm{13}} \\ $$$${b}=\sqrt{\mathrm{3}^{\mathrm{2}} +\mathrm{6}^{\mathrm{2}} }=\sqrt{\mathrm{45}} \\ $$$${c}=\mathrm{2}+\mathrm{6}=\mathrm{8} \\ $$$$\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circumcircle}\:\mathrm{of}\:\mathrm{a}\:\mathrm{triangle}\:\mathrm{is} \\ $$$${R}=\frac{{abc}}{\:\sqrt{\left({a}+{b}+{c}\right)\left({b}+{c}−{a}\right)\left({a}+{c}−{b}\right)\left({a}+{b}−{c}\right)}} \\ $$$$\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:{R}=\frac{\sqrt{\mathrm{65}}}{\mathrm{2}} \\ $$

Commented by I want to learn more last updated on 14/Aug/20

I really appreciate sir

$$\mathrm{I}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{sir} \\ $$

Answered by 1549442205PVT last updated on 14/Aug/20

Commented by 1549442205PVT last updated on 14/Aug/20

EB=6,EA=2,EC=3.ΔAEC⋍ΔDEB(a.a) ⇒((AE)/(DE))=((CE)/(EB))⇒AE.EB=CE.DE ⇒DE=(AE.EB)/CE=2.6/3=4(cm) Denote by F,G the midpoint of AB and CD respectively.We have FA=FB=4,GC=GD=3.5 Draw the diameter IH then ΔAEH⋍ΔIEB(a.a)⇒((AE)/(IE))=((EH)/(EB)) ⇒EI.EH=AE.EB=12⇔(R−OE)(R+OE) =8⇒R^2 −OE^2 =12(1).On ther other hands,by Pythagor′s theorem we have OF^2 =OB^2 −BF^2 =R^2 −4^2 OG^2 =OD^2 −DG^2 =R^2 −3.5^2 =EF^2 ⇒OE^2 =EF^2 +OF^2 =2R^2 −(4^2 +3.5^2 )(2) From (1),(2) we get R^2 −12=2R^2 −(4^2 +3.5^2 ) ⇔R^2 =4^2 +3.5^2 −12=4+12.25=16.25 ⇒R=(√(16.25))=(√((65)/4))=((√(65))/2)

$$\mathrm{EB}=\mathrm{6},\mathrm{EA}=\mathrm{2},\mathrm{EC}=\mathrm{3}.\Delta\mathrm{AEC}\backsimeq\Delta\mathrm{DEB}\left(\mathrm{a}.\mathrm{a}\right) \\ $$$$\Rightarrow\frac{\mathrm{AE}}{\mathrm{DE}}=\frac{\mathrm{CE}}{\mathrm{EB}}\Rightarrow\mathrm{AE}.\mathrm{EB}=\mathrm{CE}.\mathrm{DE} \\ $$$$\Rightarrow\mathrm{DE}=\left(\mathrm{AE}.\mathrm{EB}\right)/\mathrm{CE}=\mathrm{2}.\mathrm{6}/\mathrm{3}=\mathrm{4}\left(\mathrm{cm}\right) \\ $$$$\mathrm{Denote}\:\mathrm{by}\:\mathrm{F},\mathrm{G}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of}\:\mathrm{AB} \\ $$$$\mathrm{and}\:\mathrm{CD}\:\mathrm{respectively}.\mathrm{We}\:\mathrm{have} \\ $$$$\mathrm{FA}=\mathrm{FB}=\mathrm{4},\mathrm{GC}=\mathrm{GD}=\mathrm{3}.\mathrm{5} \\ $$$$\mathrm{Draw}\:\mathrm{the}\:\mathrm{diameter}\:\mathrm{IH}\:\mathrm{then} \\ $$$$\Delta\mathrm{AEH}\backsimeq\Delta\mathrm{IEB}\left(\mathrm{a}.\mathrm{a}\right)\Rightarrow\frac{\mathrm{AE}}{\mathrm{IE}}=\frac{\mathrm{EH}}{\mathrm{EB}} \\ $$$$\Rightarrow\mathrm{EI}.\mathrm{EH}=\mathrm{AE}.\mathrm{EB}=\mathrm{12}\Leftrightarrow\left(\mathrm{R}−\mathrm{OE}\right)\left(\mathrm{R}+\mathrm{OE}\right) \\ $$$$=\mathrm{8}\Rightarrow\mathrm{R}^{\mathrm{2}} −\mathrm{OE}^{\mathrm{2}} =\mathrm{12}\left(\mathrm{1}\right).\mathrm{On}\:\mathrm{ther}\:\mathrm{other} \\ $$$$\mathrm{hands},\mathrm{by}\:\mathrm{Pythagor}'\mathrm{s}\:\mathrm{theorem}\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{OF}^{\mathrm{2}} =\mathrm{OB}^{\mathrm{2}} −\mathrm{BF}^{\mathrm{2}} =\mathrm{R}^{\mathrm{2}} −\mathrm{4}^{\mathrm{2}} \\ $$$$\mathrm{OG}^{\mathrm{2}} =\mathrm{OD}^{\mathrm{2}} −\mathrm{DG}^{\mathrm{2}} =\mathrm{R}^{\mathrm{2}} −\mathrm{3}.\mathrm{5}^{\mathrm{2}} =\mathrm{EF}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{OE}^{\mathrm{2}} =\mathrm{EF}^{\mathrm{2}} +\mathrm{OF}^{\mathrm{2}} =\mathrm{2R}^{\mathrm{2}} −\left(\mathrm{4}^{\mathrm{2}} +\mathrm{3}.\mathrm{5}^{\mathrm{2}} \right)\left(\mathrm{2}\right) \\ $$$$\mathrm{From}\:\left(\mathrm{1}\right),\left(\mathrm{2}\right)\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{R}^{\mathrm{2}} −\mathrm{12}=\mathrm{2R}^{\mathrm{2}} −\left(\mathrm{4}^{\mathrm{2}} +\mathrm{3}.\mathrm{5}^{\mathrm{2}} \right) \\ $$$$\Leftrightarrow\mathrm{R}^{\mathrm{2}} =\mathrm{4}^{\mathrm{2}} +\mathrm{3}.\mathrm{5}^{\mathrm{2}} −\mathrm{12}=\mathrm{4}+\mathrm{12}.\mathrm{25}=\mathrm{16}.\mathrm{25} \\ $$$$\Rightarrow\boldsymbol{\mathrm{R}}=\sqrt{\mathrm{16}.\mathrm{25}}=\sqrt{\frac{\mathrm{65}}{\mathrm{4}}}=\frac{\sqrt{\mathrm{65}}}{\mathrm{2}} \\ $$

Commented by I want to learn more last updated on 14/Aug/20

I really appreciate sir

$$\mathrm{I}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{sir} \\ $$

Answered by $@y@m last updated on 14/Aug/20

Let us consider the △ADB, a=BD=3(√5) d=AB=8 b=AD=(√(13)) sin ∠ABD=(3/(3(√5)))=(1/( (√5))) ar(△ADB)=(1/2).a.d.sin ∠ABD =(1/2)×3(√5)×8×(1/( (√5)))=12 Formula: Radius of circumcirle of △ADB R=((abd)/(4.ar(ADB))) =((3(√5)×8×(√(13)))/(4×12)) =((√(65))/2)

$${Let}\:{us}\:{consider}\:{the}\:\bigtriangleup{ADB}, \\ $$$${a}={BD}=\mathrm{3}\sqrt{\mathrm{5}} \\ $$$${d}={AB}=\mathrm{8} \\ $$$${b}={AD}=\sqrt{\mathrm{13}} \\ $$$$\mathrm{sin}\:\angle{ABD}=\frac{\mathrm{3}}{\mathrm{3}\sqrt{\mathrm{5}}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}} \\ $$$${ar}\left(\bigtriangleup{ADB}\right)=\frac{\mathrm{1}}{\mathrm{2}}.{a}.{d}.\mathrm{sin}\:\angle{ABD} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}×\mathrm{3}\sqrt{\mathrm{5}}×\mathrm{8}×\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}=\mathrm{12} \\ $$$${Formula}:\:{Radius}\:{of}\:{circumcirle} \\ $$$${of}\:\bigtriangleup{ADB} \\ $$$${R}=\frac{{abd}}{\mathrm{4}.{ar}\left({ADB}\right)} \\ $$$$=\frac{\mathrm{3}\sqrt{\mathrm{5}}×\mathrm{8}×\sqrt{\mathrm{13}}}{\mathrm{4}×\mathrm{12}} \\ $$$$=\frac{\sqrt{\mathrm{65}}}{\mathrm{2}} \\ $$

Commented by I want to learn more last updated on 14/Aug/20

I really appreciate sir

$$\mathrm{I}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{sir} \\ $$

Answered by mr W last updated on 14/Aug/20

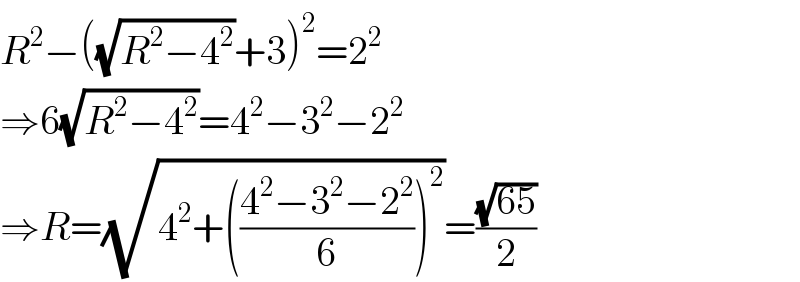
R^2 −((√(R^2 −4^2 ))+3)^2 =2^2 ⇒6(√(R^2 −4^2 ))=4^2 −3^2 −2^2 ⇒R=(√(4^2 +(((4^2 −3^2 −2^2 )/6))^2 ))=((√(65))/2)

$${R}^{\mathrm{2}} −\left(\sqrt{{R}^{\mathrm{2}} −\mathrm{4}^{\mathrm{2}} }+\mathrm{3}\right)^{\mathrm{2}} =\mathrm{2}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{6}\sqrt{{R}^{\mathrm{2}} −\mathrm{4}^{\mathrm{2}} }=\mathrm{4}^{\mathrm{2}} −\mathrm{3}^{\mathrm{2}} −\mathrm{2}^{\mathrm{2}} \\ $$$$\Rightarrow{R}=\sqrt{\mathrm{4}^{\mathrm{2}} +\left(\frac{\mathrm{4}^{\mathrm{2}} −\mathrm{3}^{\mathrm{2}} −\mathrm{2}^{\mathrm{2}} }{\mathrm{6}}\right)^{\mathrm{2}} }=\frac{\sqrt{\mathrm{65}}}{\mathrm{2}} \\ $$

Commented by I want to learn more last updated on 17/Aug/20

Thanks sir, i appreciate.

$$\mathrm{Thanks}\:\mathrm{sir},\:\:\mathrm{i}\:\mathrm{appreciate}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com