Evaluate : Ω=∫_0 ^( 1) ∫_0 ^( 1) (1/(2−x^2 − y^2 )) dxdy=??? ★★♣♣★★


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 108954 by mnjuly1970 last updated on 20/Aug/20

$E v a l u a t e :$ $Ω = \int_{0}^{1} \int_{0}^{1} \frac{1}{2 - x^{2} - y^{2}} d x d y = ? ? ?$ $★ ★ ♣ ♣ ★ ★$
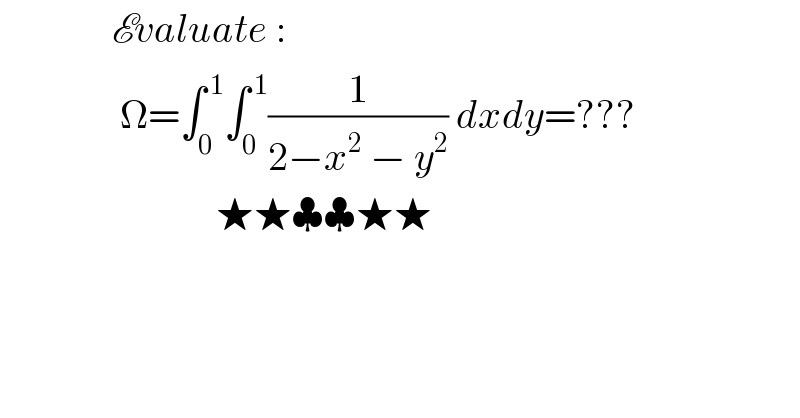
Commented by kaivan.ahmadi last updated on 20/Aug/20

$x = r c o s θ, y = r s i n θ \Rightarrow - x^{2} - y^{2} = - r^{2}$ $0 ⩽ y ⩽, 0 ⩽ x ⩽ 1 \Rightarrow 0 ⩽ θ ⩽ \frac{π}{2}, 0 ⩽ r ⩽ 1$ $Ω = \int_{0}^{\frac{π}{2}} \int_{0}^{1} \frac{1}{2 - r^{2}} r d r d θ$ $f i r s t f i n d \int_{0}^{1} \frac{r}{2 - r^{2}} d r$ $u = 2 - r^{2} \Rightarrow d u = - 2 r d r$ $\Rightarrow \frac{- 1}{2} \int \frac{d u}{u} = \frac{- 1}{2} l n u = \frac{- 1}{2} l n (2 - r^{2}) ∣_{0}^{1} = \frac{- 1}{2} (l n 1 - l n 2) =$ $\frac{l n 2}{2}$ $\Rightarrow Ω = \int_{0}^{\frac{π}{2}} \frac{l n 2}{2} d θ = \frac{l n 2}{2} \times \frac{π}{2} = \frac{π}{4} l n 2$
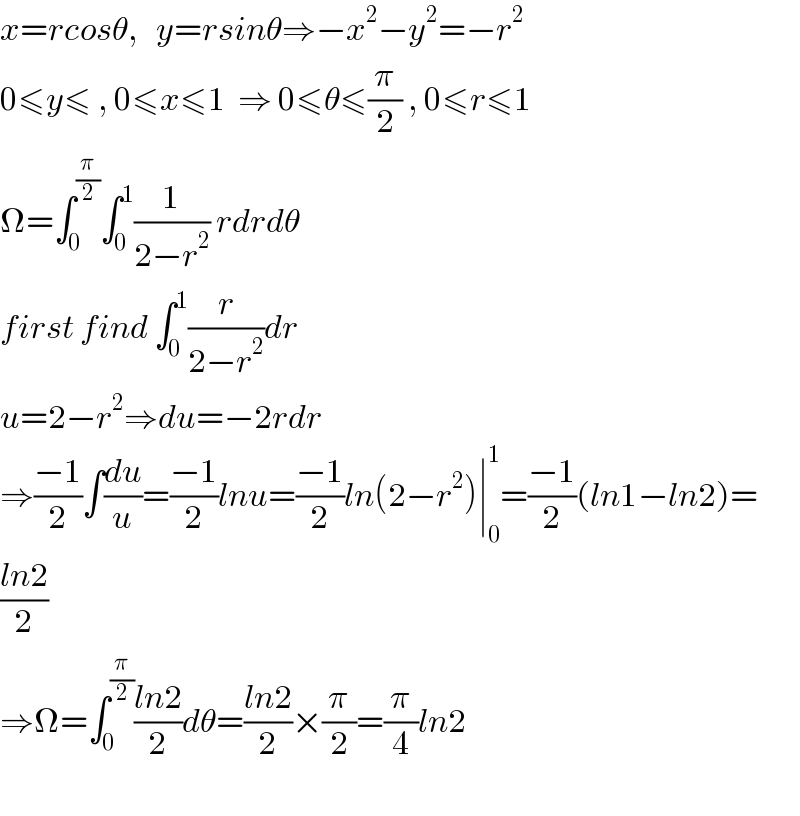
Commented by mnjuly1970 last updated on 20/Aug/20

$0 ⩽ r ⩽ s e c (θ) & 0 ⩽ θ ⩽ \frac{π}{4} b e c a u s e$ $i n t e g r a n d i s s y m m e t r i c$ $f (x, y) = f (y, x)$ $f i n a l a n s w e r i s G : c a t a l a n c o n s t a n t . . .$
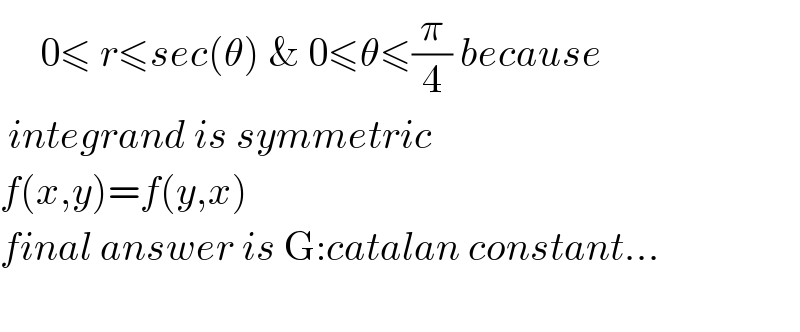
Commented by kaivan.ahmadi last updated on 20/Aug/20

$H i s i r, w h y d o w e u s e a d i f i c u l t w a y w h e n w e h a v e a$ $h a v e a s i m p l e w a y ?$
Commented by mathmax by abdo last updated on 20/Aug/20

$0 ⩽ x ⩽ 1 and 0 ⩽ y ⩽ 1 \Rightarrow 0 ⩽ x^{2} + y^{2} ⩽ 2 \Rightarrow 0 ⩽ r ⩽ {\sqrt{2}}^{'} . .!$
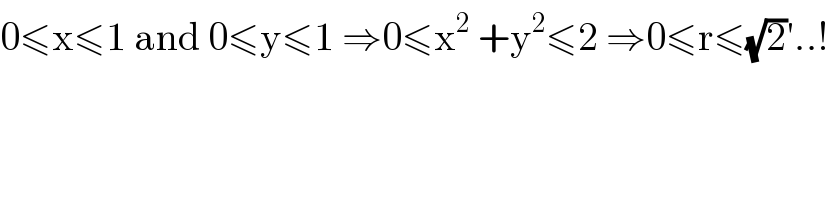
Commented by kaivan.ahmadi last updated on 21/Aug/20

$s t u d y p o l a r c o o r d i n a t e s y s t e m p l e a s e .$
	Answered by mathmax by abdo last updated on 20/Aug/20
	$Ω =∫_0 ^1 ∫_0 ^1 ((dxdy)/(2−x^2 −y^2 )) we considere the diffeomorphism { ((x =rcosθ _(⇒ ) Ω =∫∫ _(o≤r≤(√2)and 0≤θ≤(π/2)) (1/(2−r^2 ))r dr dθ)),((y =rsinθ)) :} =(π/2)∫_0 ^(√2) ((rdr)/((2−r^2 ))) =−(π/4) ∫_0 ^(√2) ((−2r)/(2−r^2 ))dr =−(π/4)[ln∣2−r^2 ∣]_0 ^(√2) =∞ this integral is divergent...$
	$Ω = \int_{0}^{1} \int_{0}^{1} \frac{dxdy}{2 - x^{2} - y^{2}} we considere the diffeomorphism$ ${\begin{cases} x = rcos θ_{\Rightarrow} Ω = \int \int_{o ⩽ r ⩽ \sqrt{2} and 0 ⩽ θ ⩽ \frac{π}{2}} \frac{1}{2 - r^{2}} r dr d θ \\ y = rsin θ \end{cases}$ $= \frac{π}{2} \int_{0}^{\sqrt{2}} \frac{rdr}{(2 - r^{2})} = - \frac{π}{4} \int_{0}^{\sqrt{2}} \frac{- 2 r}{2 - r^{2}} dr = - \frac{π}{4} {[\ln ∣ 2 - r^{2} ∣]}_{0}^{\sqrt{2}} = \infty$ $this integral is divergent . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum