
Question and Answers Forum
Question Number 109090 by ajfour last updated on 21/Aug/20
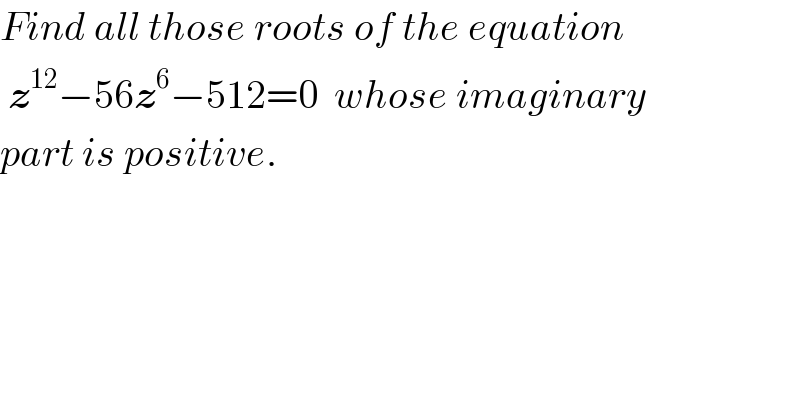
Answered by floor(10²Eta[1]) last updated on 21/Aug/20

Commented by ajfour last updated on 21/Aug/20
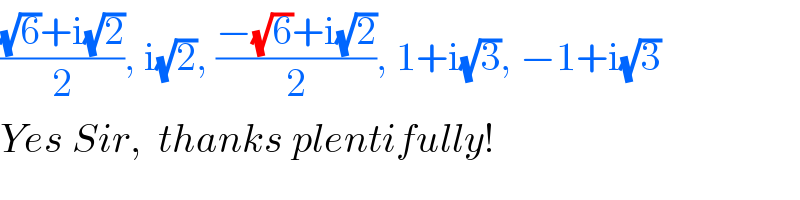
| ||
Question and Answers Forum | ||
Question Number 109090 by ajfour last updated on 21/Aug/20 | ||
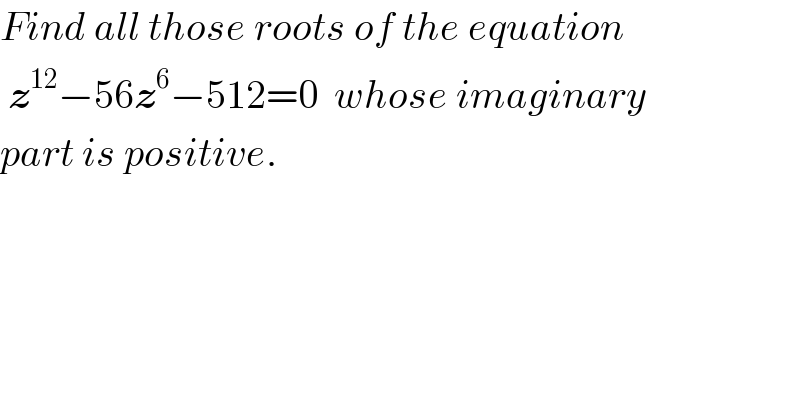 | ||
Answered by floor(10²Eta[1]) last updated on 21/Aug/20 | ||
 | ||
| ||
Commented by ajfour last updated on 21/Aug/20 | ||
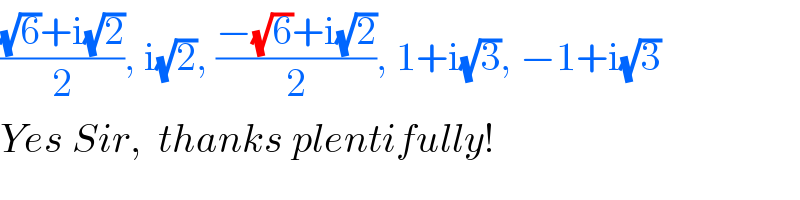 | ||