
Question Number 110056 by mathdave last updated on 27/Aug/20

$${prove}\:{that}\: \\ $$$$\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}^{\mathrm{4}} {x}−\mathrm{6sin}^{\mathrm{2}} {x}\mathrm{cos}^{\mathrm{2}} {x}+\mathrm{sin}^{\mathrm{4}} {x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\frac{\pi}{{e}^{\mathrm{4}} } \\ $$
Answered by mathmax by abdo last updated on 26/Aug/20

$$\mathrm{A}\:=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}−\mathrm{sin}^{\mathrm{4}} \mathrm{x}\:−\mathrm{6sin}^{\mathrm{2}} \mathrm{x}\:\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{A}\:=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}\:−\mathrm{6sin}^{\mathrm{2}} \mathrm{x}\:\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)−\mathrm{6}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\left(\mathrm{2x}\right)\right)^{\mathrm{2}} }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)−\frac{\mathrm{3}}{\mathrm{2}}\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2x}\right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$=\int_{−\infty} ^{+\infty\:} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}−\frac{\mathrm{3}}{\mathrm{4}}\int_{−\infty} ^{+\infty} \:\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$$$=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{ex}−\frac{\mathrm{3}}{\mathrm{4}}\left(\pi\right)+\frac{\mathrm{3}}{\mathrm{4}}\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:\:\mathrm{we}\:\mathrm{have}\: \\ $$$$\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:=\mathrm{Re}\left(\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{i2x}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\right)\:\mathrm{and}\: \\ $$$$\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{i2z}} }{\mathrm{z}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:=\mathrm{2i}\pi\:×\mathrm{Res}\left(\mathrm{f},\mathrm{i}\right)\:=\mathrm{2i}\pi×\frac{\mathrm{e}^{−\mathrm{2}} }{\mathrm{2i}}\:=\:\pi\mathrm{e}^{−\mathrm{2}} \:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:=\frac{\pi}{\mathrm{e}^{\mathrm{2}} }\:\:\mathrm{also}\:\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:=\mathrm{Re}\left(\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{i4x}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\right) \\ $$$$\mathrm{and}\:\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{i4z}} }{\mathrm{z}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dz}\:=\mathrm{2i}\pi\:\mathrm{Re}\left(\mathrm{f},\mathrm{i}\right)\:=\mathrm{2i}\pi×\frac{\mathrm{e}^{−\mathrm{4}} }{\mathrm{2i}}\:=\frac{\pi}{\mathrm{e}^{\mathrm{4}} }\:\Rightarrow\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$$$=\frac{\pi}{\mathrm{e}^{\mathrm{4}} }\:\Rightarrow\:\mathrm{A}\:=\frac{\pi}{\mathrm{e}^{\mathrm{2}} }−\frac{\mathrm{3}\pi}{\mathrm{4}}\:+\frac{\mathrm{3}}{\mathrm{4}}.\frac{\pi}{\mathrm{e}^{\mathrm{4}} }\:=\frac{\pi}{\mathrm{e}^{\mathrm{2}} }\:+\frac{\mathrm{3}\pi}{\mathrm{4e}^{\mathrm{4}} }−\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$$\mathrm{something}\:\mathrm{went}\:\mathrm{wrong}\:\mathrm{in}\:\mathrm{the}\:\mathrm{question}...! \\ $$$$ \\ $$
Commented by mathdave last updated on 27/Aug/20

$${i}\:{will}\:{work}\:{it}\:{now} \\ $$
Commented by mathdave last updated on 26/Aug/20

$${the}\:{question}\:{is}\:{very}\:{correct} \\ $$
Commented by mathmax by abdo last updated on 26/Aug/20
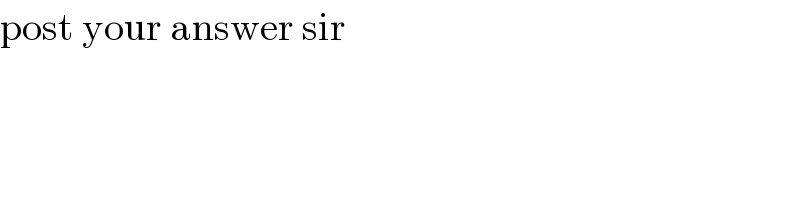
$$\mathrm{post}\:\mathrm{your}\:\mathrm{answer}\:\mathrm{sir}\: \\ $$
Commented by mathmax by abdo last updated on 27/Aug/20
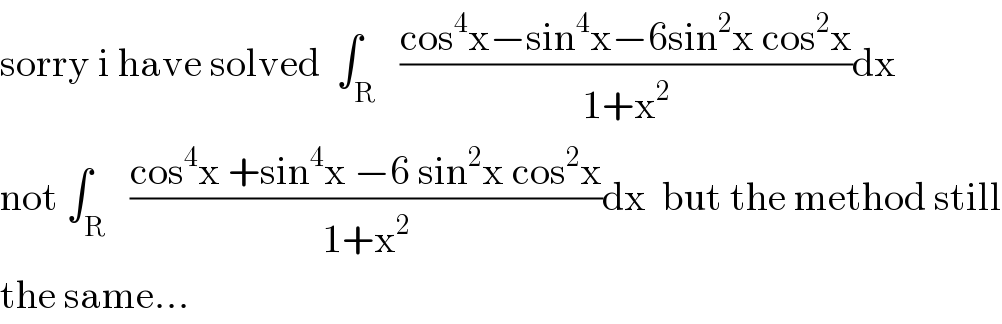
$$\mathrm{sorry}\:\mathrm{i}\:\mathrm{have}\:\mathrm{solved}\:\:\int_{\mathrm{R}} \:\:\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}−\mathrm{sin}^{\mathrm{4}} \mathrm{x}−\mathrm{6sin}^{\mathrm{2}} \mathrm{x}\:\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$\mathrm{not}\:\int_{\mathrm{R}} \:\:\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}\:+\mathrm{sin}^{\mathrm{4}} \mathrm{x}\:−\mathrm{6}\:\mathrm{sin}^{\mathrm{2}} \mathrm{x}\:\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\:\mathrm{but}\:\mathrm{the}\:\mathrm{method}\:\mathrm{still} \\ $$$$\mathrm{the}\:\mathrm{same}... \\ $$
Answered by mathdave last updated on 27/Aug/20
![solution let I=∫_R ((cos^4 x+2cos^2 xsin^2 x+sin^4 x−8cos^2 xsin^2 x)/(1+x^2 ))dx I=∫_R (((cos^2 x+sin^2 x)^2 −2.2^2 sin^2 xcos^2 x)/(1+x^2 ))dx I=∫_R ((1−2sin^2 (2x))/(1+x^2 ))dx=∫_(−∞) ^(+∞) ((cos(4x))/(1+x^2 ))dx.....(x) let t=4 then I(t)=∫_(−∞) ^(+∞) ((cos(tx))/(1+x^2 ))dx.....(r) I(0)=∫_(−∞) ^(+∞) ((cos(0))/(1+x^2 ))dx=∫_R (1/(1+x^2 ))dx=[tan^(−1) (x)]_(−∞) ^(+∞) I(0)=(π/2)−(−(π/2))=π............(1) differentiate equation (x) under ( t) I′(t)=−∫_R ((xsin(tx))/(1+x^2 ))dx=∫_R (((x^2 +1−1)sin(tx))/(x(1+x^2 )))dx I′(t)=∫_(−∞) ^(+∞) ((sin(tx))/x)+∫_R ((sin(tx))/(x(1+x^2 )))dx..........(2) let I_1 (t)=∫_(−∞) ^(+∞) ((sin(tx))/x)dx=2∫_0 ^∞ ((sin(tx))/x)dx but Maz identity say that I(a)=∫_0 ^∞ ((sin(ax))/x^n )dx=(π/(2Γ(n)sin(((nπ)/2))))a^(n−1) has n=1 and a=t ∵I_1 (t)=2∫_0 ^∞ ((sin(tx))/x)dx=2•((πt^((1−1)) )/(2Γ(1)sin((π/2))))=2•(π/2)=π.....(xx) putting equation (xx) into equation (2) I′(t)=−π+∫_R ((sin(tx))/(x(1+x^2 )))dx......(3) ( at t=0) I′(0)=−π.......(4) again differrentiate equation (3) under (t) I′′(t)=∫_(−∞) ^(+∞) ((cos(tx))/(1+x^2 ))dx=I(t) (this is the same as equatn (r) then I′′(t)−I(t)=0 (taking the laplace transform) L[I′′(t)]−L[(I(t)]=0 s^2 I(s)−sI(0)−I′(0)−I(s)=0 but values of I(0)=π and I′(0)=−π s^2 I(s)−πs+π−I(s)=0 I(s)[s^2 +1]=π[s−1] I(s)[(s+1)(s−1)]=π[s−1] I(s)=(π/(s+1)) (now taking inverse laplace transform) L^(−1) [I(s)]=πL^(−1) [(1/(s+1))] I(t)=πe^(−t) ( but t=4) I(4)=πe^(−4) =(π/e^4 ) ∵∫_(−∞) ^(+∞) ((cos^4 x−6cos^2 xsin^2 x+sin^4 x)/(1+x^2 ))dx=(π/e^4 ) Q.E.D by mathdave(27/08/2020)](Q110085.png)
$${solution}\: \\ $$$${let} \\ $$$${I}=\int_{{R}} \frac{\mathrm{cos}^{\mathrm{4}} {x}+\mathrm{2cos}^{\mathrm{2}} {x}\mathrm{sin}^{\mathrm{2}} {x}+\mathrm{sin}^{\mathrm{4}} {x}−\mathrm{8cos}^{\mathrm{2}} {x}\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$${I}=\int_{{R}} \frac{\left(\mathrm{cos}^{\mathrm{2}} {x}+\mathrm{sin}^{\mathrm{2}} {x}\right)^{\mathrm{2}} −\mathrm{2}.\mathrm{2}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} {x}\mathrm{cos}^{\mathrm{2}} {x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$${I}=\int_{{R}} \frac{\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}\left(\mathrm{4}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}.....\left({x}\right) \\ $$$${let}\:\:\:{t}=\mathrm{4}\:\:\:\:{then}\:\:{I}\left({t}\right)=\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}\left({tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}.....\left({r}\right) \\ $$$${I}\left(\mathrm{0}\right)=\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}\left(\mathrm{0}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\int_{{R}} \frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\left[\mathrm{tan}^{−\mathrm{1}} \left({x}\right)\right]_{−\infty} ^{+\infty} \\ $$$${I}\left(\mathrm{0}\right)=\frac{\pi}{\mathrm{2}}−\left(−\frac{\pi}{\mathrm{2}}\right)=\pi............\left(\mathrm{1}\right) \\ $$$${differentiate}\:{equation}\:\left({x}\right)\:\:{under}\:\left(\:{t}\right) \\ $$$${I}'\left({t}\right)=−\int_{{R}} \frac{{x}\mathrm{sin}\left({tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\int_{{R}} \frac{\left({x}^{\mathrm{2}} +\mathrm{1}−\mathrm{1}\right)\mathrm{sin}\left({tx}\right)}{{x}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx} \\ $$$${I}'\left({t}\right)=\int_{−\infty} ^{+\infty} \frac{\mathrm{sin}\left({tx}\right)}{{x}}+\int_{{R}} \frac{\mathrm{sin}\left({tx}\right)}{{x}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx}..........\left(\mathrm{2}\right) \\ $$$${let}\:\:{I}_{\mathrm{1}} \left({t}\right)=\int_{−\infty} ^{+\infty} \frac{\mathrm{sin}\left({tx}\right)}{{x}}{dx}=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\left({tx}\right)}{{x}}{dx} \\ $$$$ \\ $$$${but}\:{Maz}\:\:{identity}\:{say}\:{that} \\ $$$${I}\left({a}\right)=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\left({ax}\right)}{{x}^{{n}} }{dx}=\frac{\pi}{\mathrm{2}\Gamma\left({n}\right)\mathrm{sin}\left(\frac{{n}\pi}{\mathrm{2}}\right)}{a}^{{n}−\mathrm{1}} \\ $$$$\:\:\:\:{has}\:\:\:\:{n}=\mathrm{1}\:\:\:{and}\:\:\:{a}={t} \\ $$$$\because{I}_{\mathrm{1}} \left({t}\right)=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\left({tx}\right)}{{x}}{dx}=\mathrm{2}\bullet\frac{\pi{t}^{\left(\mathrm{1}−\mathrm{1}\right)} }{\mathrm{2}\Gamma\left(\mathrm{1}\right)\mathrm{sin}\left(\frac{\pi}{\mathrm{2}}\right)}=\mathrm{2}\bullet\frac{\pi}{\mathrm{2}}=\pi.....\left({xx}\right) \\ $$$${putting}\:{equation}\:\left({xx}\right)\:\:{into}\:{equation}\:\left(\mathrm{2}\right) \\ $$$${I}'\left({t}\right)=−\pi+\int_{{R}} \frac{\mathrm{sin}\left({tx}\right)}{{x}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx}......\left(\mathrm{3}\right)\:\:\:\left(\:{at}\:\:\:{t}=\mathrm{0}\right) \\ $$$${I}'\left(\mathrm{0}\right)=−\pi.......\left(\mathrm{4}\right) \\ $$$${again}\:{differrentiate}\:\:{equation}\:\:\left(\mathrm{3}\right)\:{under}\:\:\left({t}\right) \\ $$$${I}''\left({t}\right)=\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}\left({tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}={I}\left({t}\right)\:\:\:\:\left({this}\:{is}\:{the}\:{same}\:{as}\:{equatn}\:\:\left({r}\right)\right. \\ $$$${then}\:\:\:\:\:{I}''\left({t}\right)−{I}\left({t}\right)=\mathrm{0}\:\:\:\:\:\left({taking}\:{the}\:{laplace}\:{transform}\right) \\ $$$${L}\left[{I}''\left({t}\right)\right]−{L}\left[\left({I}\left({t}\right)\right]=\mathrm{0}\right. \\ $$$${s}^{\mathrm{2}} {I}\left({s}\right)−{sI}\left(\mathrm{0}\right)−{I}'\left(\mathrm{0}\right)−{I}\left({s}\right)=\mathrm{0} \\ $$$${but}\:{values}\:{of}\:\:\:\:{I}\left(\mathrm{0}\right)=\pi\:\:\:\:{and}\:\:\:\:{I}'\left(\mathrm{0}\right)=−\pi \\ $$$${s}^{\mathrm{2}} {I}\left({s}\right)−\pi{s}+\pi−{I}\left({s}\right)=\mathrm{0} \\ $$$${I}\left({s}\right)\left[{s}^{\mathrm{2}} +\mathrm{1}\right]=\pi\left[{s}−\mathrm{1}\right] \\ $$$${I}\left({s}\right)\left[\left({s}+\mathrm{1}\right)\left({s}−\mathrm{1}\right)\right]=\pi\left[{s}−\mathrm{1}\right] \\ $$$${I}\left({s}\right)=\frac{\pi}{{s}+\mathrm{1}}\:\:\:\:\:\:\left({now}\:{taking}\:{inverse}\:{laplace}\:{transform}\right) \\ $$$${L}^{−\mathrm{1}} \left[{I}\left({s}\right)\right]=\pi{L}^{−\mathrm{1}} \left[\frac{\mathrm{1}}{{s}+\mathrm{1}}\right] \\ $$$${I}\left({t}\right)=\pi{e}^{−{t}} \:\:\:\:\:\:\:\left(\:{but}\:\:\:{t}=\mathrm{4}\right) \\ $$$${I}\left(\mathrm{4}\right)=\pi{e}^{−\mathrm{4}} =\frac{\pi}{{e}^{\mathrm{4}} } \\ $$$$\because\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}^{\mathrm{4}} {x}−\mathrm{6cos}^{\mathrm{2}} {x}\mathrm{sin}^{\mathrm{2}} {x}+\mathrm{sin}^{\mathrm{4}} {x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\frac{\pi}{{e}^{\mathrm{4}} }\:\:\:\:\:\:\:{Q}.{E}.{D} \\ $$$${by}\:{mathdave}\left(\mathrm{27}/\mathrm{08}/\mathrm{2020}\right) \\ $$
