Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 110269 by Khanacademy last updated on 28/Aug/20

Commented by bemath last updated on 28/Aug/20

−(1/2)

$$−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Commented by Khanacademy last updated on 28/Aug/20

?

$$? \\ $$

Commented by john santu last updated on 28/Aug/20

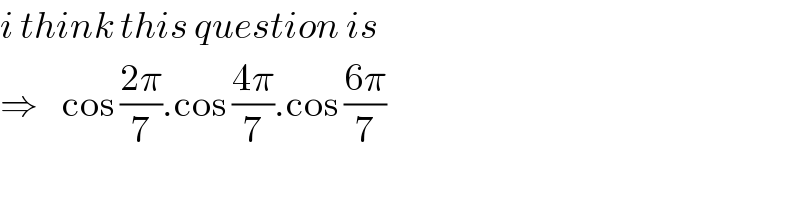
i think this question is ⇒ cos ((2π)/7).cos ((4π)/7).cos ((6π)/7)

$${i}\:{think}\:{this}\:{question}\:{is}\: \\ $$$$\Rightarrow\:\:\:\:\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}} \\ $$

Commented by bemath last updated on 28/Aug/20

if cos ((2π)/7).cos ((4π)/7).cos ((6π)/7) = p 2psin ((2π)/7)= sin ((4π)/7).cos ((4π)/7).cos ((6π)/7) 4psin ((2π)/7)=sin ((8π)/7).cos ((6π)/7) 8psin ((2π)/7)=sin ((14π)/7)+sin ((2π)/7) [ sin ((14π)/7) = 0 ] ⇒p=(1/8)

$${if}\:\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}}\:=\:{p} \\ $$$$\mathrm{2}{p}\mathrm{sin}\:\frac{\mathrm{2}\pi}{\mathrm{7}}=\:\mathrm{sin}\:\frac{\mathrm{4}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}} \\ $$$$\mathrm{4}{p}\mathrm{sin}\:\frac{\mathrm{2}\pi}{\mathrm{7}}=\mathrm{sin}\:\frac{\mathrm{8}\pi}{\mathrm{7}}.\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}} \\ $$$$\mathrm{8}{p}\mathrm{sin}\:\frac{\mathrm{2}\pi}{\mathrm{7}}=\mathrm{sin}\:\frac{\mathrm{14}\pi}{\mathrm{7}}+\mathrm{sin}\:\frac{\mathrm{2}\pi}{\mathrm{7}} \\ $$$$\left[\:\mathrm{sin}\:\frac{\mathrm{14}\pi}{\mathrm{7}}\:=\:\mathrm{0}\:\right]\:\Rightarrow{p}=\frac{\mathrm{1}}{\mathrm{8}} \\ $$

Answered by Dwaipayan Shikari last updated on 28/Aug/20

cos2θ+cos4θ+cos6θ θ=(π/7) 7θ=π (1/(2sinθ))(sin3θ−sinθ+sin5θ−sin3θ+sin7θ−sin5θ) (1/(2sinθ))(sin7θ−sinθ)=−((sinθ)/(2sinθ))=−(1/2) (sin7θ=0)

$${cos}\mathrm{2}\theta+{cos}\mathrm{4}\theta+{cos}\mathrm{6}\theta\:\:\:\:\:\:\:\:\theta=\frac{\pi}{\mathrm{7}}\:\:\mathrm{7}\theta=\pi \\ $$$$\frac{\mathrm{1}}{\mathrm{2}{sin}\theta}\left({sin}\mathrm{3}\theta−{sin}\theta+{sin}\mathrm{5}\theta−{sin}\mathrm{3}\theta+{sin}\mathrm{7}\theta−{sin}\mathrm{5}\theta\right) \\ $$$$\frac{\mathrm{1}}{\mathrm{2}{sin}\theta}\left({sin}\mathrm{7}\theta−{sin}\theta\right)=−\frac{{sin}\theta}{\mathrm{2}{sin}\theta}=−\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\:\:\left({sin}\mathrm{7}\theta=\mathrm{0}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com