
Question Number 110608 by Engr_Jidda last updated on 29/Aug/20
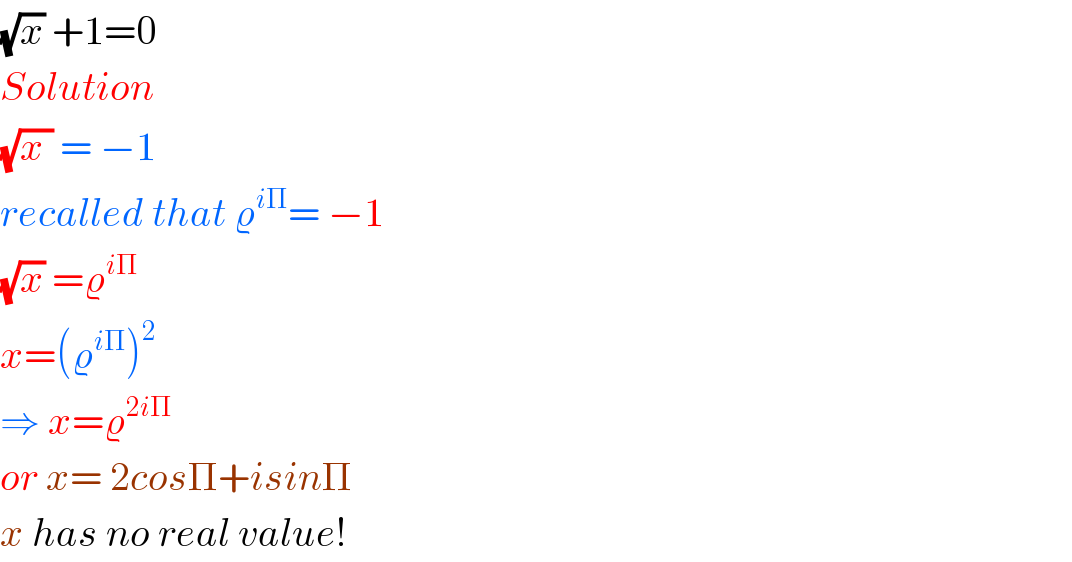
$$\sqrt{{x}}\:+\mathrm{1}=\mathrm{0} \\ $$$${Solution} \\ $$$$\sqrt{{x}\:}\:=\:−\mathrm{1} \\ $$$${recalled}\:{that}\:\varrho^{{i}\Pi} =\:−\mathrm{1} \\ $$$$\sqrt{{x}}\:=\varrho^{{i}\Pi} \\ $$$${x}=\left(\varrho^{{i}\Pi} \right)^{\mathrm{2}} \\ $$$$\Rightarrow\:{x}=\varrho^{\mathrm{2}{i}\Pi} \\ $$$${or}\:{x}=\:\mathrm{2}{cos}\Pi+{isin}\Pi \\ $$$${x}\:{has}\:{no}\:{real}\:{value}! \\ $$
Commented by Her_Majesty last updated on 29/Aug/20

$${you}'{re}\:{wrong} \\ $$$${x}={e}^{\mathrm{2}{i}\pi} \:\Leftrightarrow\:{x}={cos}\mathrm{2}\pi\:+{isin}\mathrm{2}\pi=\mathrm{1} \\ $$$${anyway}\:{you}'{re}\:{wrong}\:{because}\:{we}\:{are}\:{not} \\ $$$${allowed}\:{to}\:{square}\:{both}\:{sides}\:{of}\:{any}\:{equation}; \\ $$$${this}\:{leads}\:{to}\:{false}\:{solutions}: \\ $$$${x}=\mathrm{3} \\ $$$${x}^{\mathrm{2}} =\mathrm{9}\:\Rightarrow\:{x}=\pm\mathrm{3} \\ $$$$ \\ $$$${in}\:{our}\:{case}\:{we}\:{have}\:{to}\:{set}\:{ranges}\:{first} \\ $$$${if}\:{x}\in\mathbb{C}\:\Rightarrow\:{x}={re}^{{i}\theta} \:{with}\:{r}\in\mathbb{R}\wedge{r}\geqslant\mathrm{0}\:{and} \\ $$$$\theta\in\mathbb{R}\wedge\begin{cases}{−\pi\leqslant\theta<\pi}\\{\mathrm{0}\leqslant\theta<\mathrm{2}\pi}\end{cases}\:\left({both}\:{are}\:{in}\:{use}\right) \\ $$$$\sqrt{{x}}=−\mathrm{1} \\ $$$$\sqrt{{re}^{{i}\theta} }=−\mathrm{1} \\ $$$$\sqrt{{r}}{e}^{{i}\theta/\mathrm{2}} =−\mathrm{1} \\ $$$${but}\:−\mathrm{1}={e}^{\pm{i}\pi} \\ $$$$\sqrt{{r}}{e}^{{i}\theta/\mathrm{2}} ={e}^{\pm{i}\pi} \:\Leftrightarrow\:{r}=\mathrm{1}\wedge\theta/\mathrm{2}=\pm\pi\:\Leftrightarrow\:\theta=\pm\mathrm{2}\pi \\ $$$${but}\:\theta\:{is}\:{not}\:{in}\:{our}\:{range}\:\Rightarrow\:{we}\:{have}\:{to} \\ $$$${subtract}/{add}\:\mathrm{2}\pi\:\Rightarrow\:\theta=\mathrm{0} \\ $$$$\Rightarrow\:{x}=\sqrt{{x}}={e}^{\mathrm{0}} =\mathrm{1}\neq−\mathrm{1}\:\Rightarrow\:{no}\:{solution}\:{at}\:{all} \\ $$
