Question and Answers Forum

All Questions      Topic List

Permutation and Combination Questions

Previous in All Question      Next in All Question      

Previous in Permutation and Combination      Next in Permutation and Combination      

Question Number 110729 by mr W last updated on 30/Aug/20

How many ways can the letters in the word MATHEMATICS be rearranged such that the word formed neither starts nor ends with a vowel, and any four consecutive letters must contain at least a vowel?

$$\mathrm{How}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{the}\:\mathrm{letters}\:\mathrm{in} \\ $$$$\mathrm{the}\:\mathrm{word}\:\boldsymbol{\mathrm{MATHEMATICS}} \\ $$$$\mathrm{be}\:\mathrm{rearranged}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{word} \\ $$$$\mathrm{formed}\:\mathrm{neither}\:\mathrm{starts}\:\mathrm{nor}\:\mathrm{ends}\:\mathrm{with}\:\mathrm{a}\: \\ $$$$\mathrm{vowel},\:\mathrm{and}\:\mathrm{any}\:\mathrm{four}\:\mathrm{consecutive}\: \\ $$$$\mathrm{letters}\:\mathrm{must}\:\mathrm{contain}\:\mathrm{at}\:\mathrm{least}\:\mathrm{a}\:\mathrm{vowel}? \\ $$

Answered by mr W last updated on 30/Aug/20

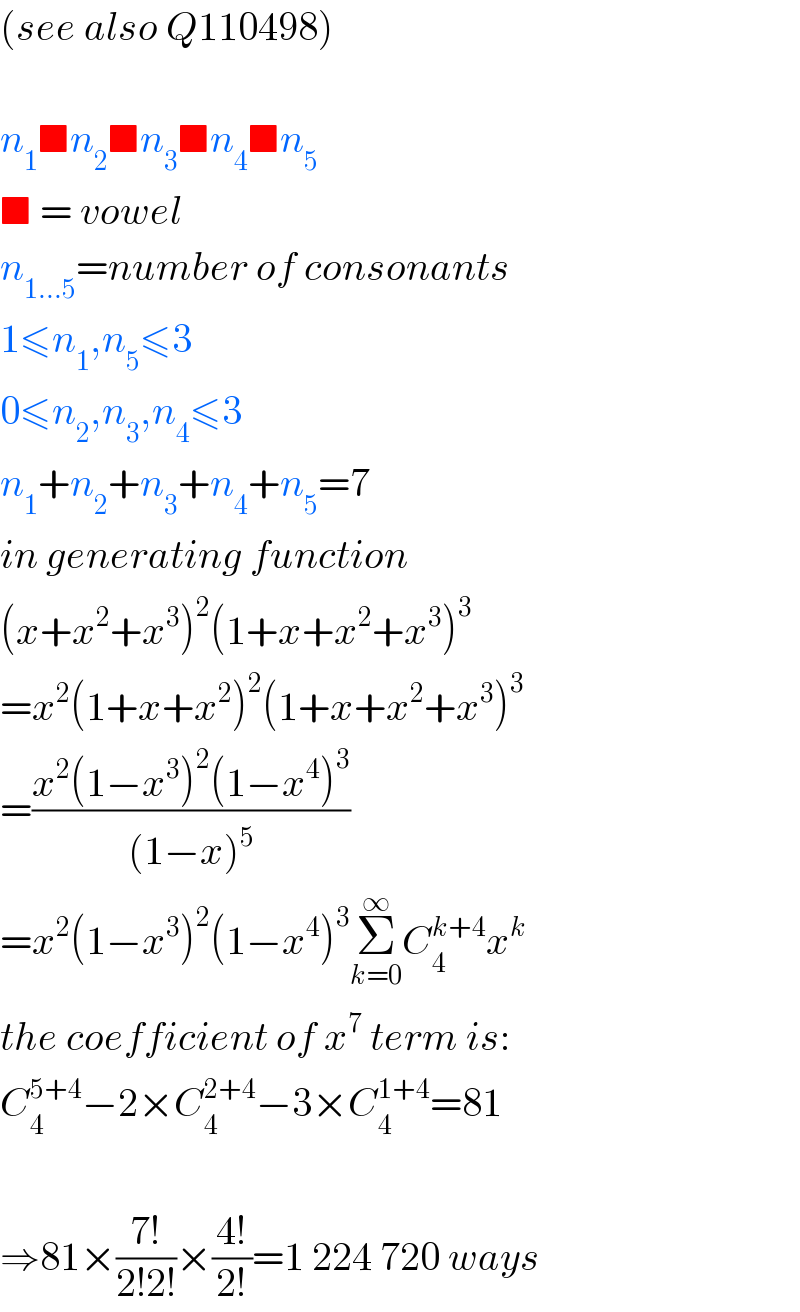
(see also Q110498) n_1 ■n_2 ■n_3 ■n_4 ■n_5 ■ = vowel n_(1...5) =number of consonants 1≤n_1 ,n_5 ≤3 0≤n_2 ,n_3 ,n_4 ≤3 n_1 +n_2 +n_3 +n_4 +n_5 =7 in generating function (x+x^2 +x^3 )^2 (1+x+x^2 +x^3 )^3 =x^2 (1+x+x^2 )^2 (1+x+x^2 +x^3 )^3 =((x^2 (1−x^3 )^2 (1−x^4 )^3 )/((1−x)^5 )) =x^2 (1−x^3 )^2 (1−x^4 )^3 Σ_(k=0) ^∞ C_4 ^(k+4) x^k the coefficient of x^7 term is: C_4 ^(5+4) −2×C_4 ^(2+4) −3×C_4 ^(1+4) =81 ⇒81×((7!)/(2!2!))×((4!)/(2!))=1 224 720 ways

$$\left({see}\:{also}\:{Q}\mathrm{110498}\right) \\ $$$$ \\ $$$${n}_{\mathrm{1}} \blacksquare{n}_{\mathrm{2}} \blacksquare{n}_{\mathrm{3}} \blacksquare{n}_{\mathrm{4}} \blacksquare{n}_{\mathrm{5}} \\ $$$$\blacksquare\:=\:{vowel} \\ $$$${n}_{\mathrm{1}...\mathrm{5}} ={number}\:{of}\:{consonants} \\ $$$$\mathrm{1}\leqslant{n}_{\mathrm{1}} ,{n}_{\mathrm{5}} \leqslant\mathrm{3} \\ $$$$\mathrm{0}\leqslant{n}_{\mathrm{2}} ,{n}_{\mathrm{3}} ,{n}_{\mathrm{4}} \leqslant\mathrm{3} \\ $$$${n}_{\mathrm{1}} +{n}_{\mathrm{2}} +{n}_{\mathrm{3}} +{n}_{\mathrm{4}} +{n}_{\mathrm{5}} =\mathrm{7} \\ $$$${in}\:{generating}\:{function} \\ $$$$\left({x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} \right)^{\mathrm{2}} \left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} \right)^{\mathrm{3}} \\ $$$$={x}^{\mathrm{2}} \left(\mathrm{1}+{x}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} \right)^{\mathrm{3}} \\ $$$$=\frac{{x}^{\mathrm{2}} \left(\mathrm{1}−{x}^{\mathrm{3}} \right)^{\mathrm{2}} \left(\mathrm{1}−{x}^{\mathrm{4}} \right)^{\mathrm{3}} }{\left(\mathrm{1}−{x}\right)^{\mathrm{5}} } \\ $$$$={x}^{\mathrm{2}} \left(\mathrm{1}−{x}^{\mathrm{3}} \right)^{\mathrm{2}} \left(\mathrm{1}−{x}^{\mathrm{4}} \right)^{\mathrm{3}} \underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{C}_{\mathrm{4}} ^{{k}+\mathrm{4}} {x}^{{k}} \\ $$$${the}\:{coefficient}\:{of}\:{x}^{\mathrm{7}} \:{term}\:{is}: \\ $$$${C}_{\mathrm{4}} ^{\mathrm{5}+\mathrm{4}} −\mathrm{2}×{C}_{\mathrm{4}} ^{\mathrm{2}+\mathrm{4}} −\mathrm{3}×{C}_{\mathrm{4}} ^{\mathrm{1}+\mathrm{4}} =\mathrm{81} \\ $$$$ \\ $$$$\Rightarrow\mathrm{81}×\frac{\mathrm{7}!}{\mathrm{2}!\mathrm{2}!}×\frac{\mathrm{4}!}{\mathrm{2}!}=\mathrm{1}\:\mathrm{224}\:\mathrm{720}\:{ways} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com