
Question and Answers Forum
Question Number 111528 by bemath last updated on 04/Sep/20
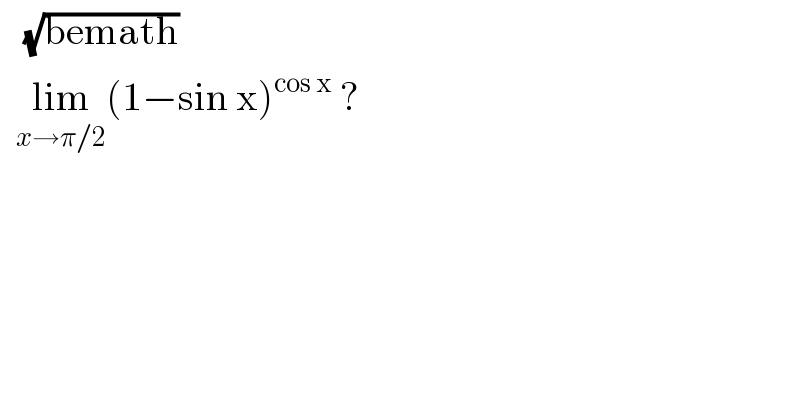
Answered by bobhans last updated on 04/Sep/20
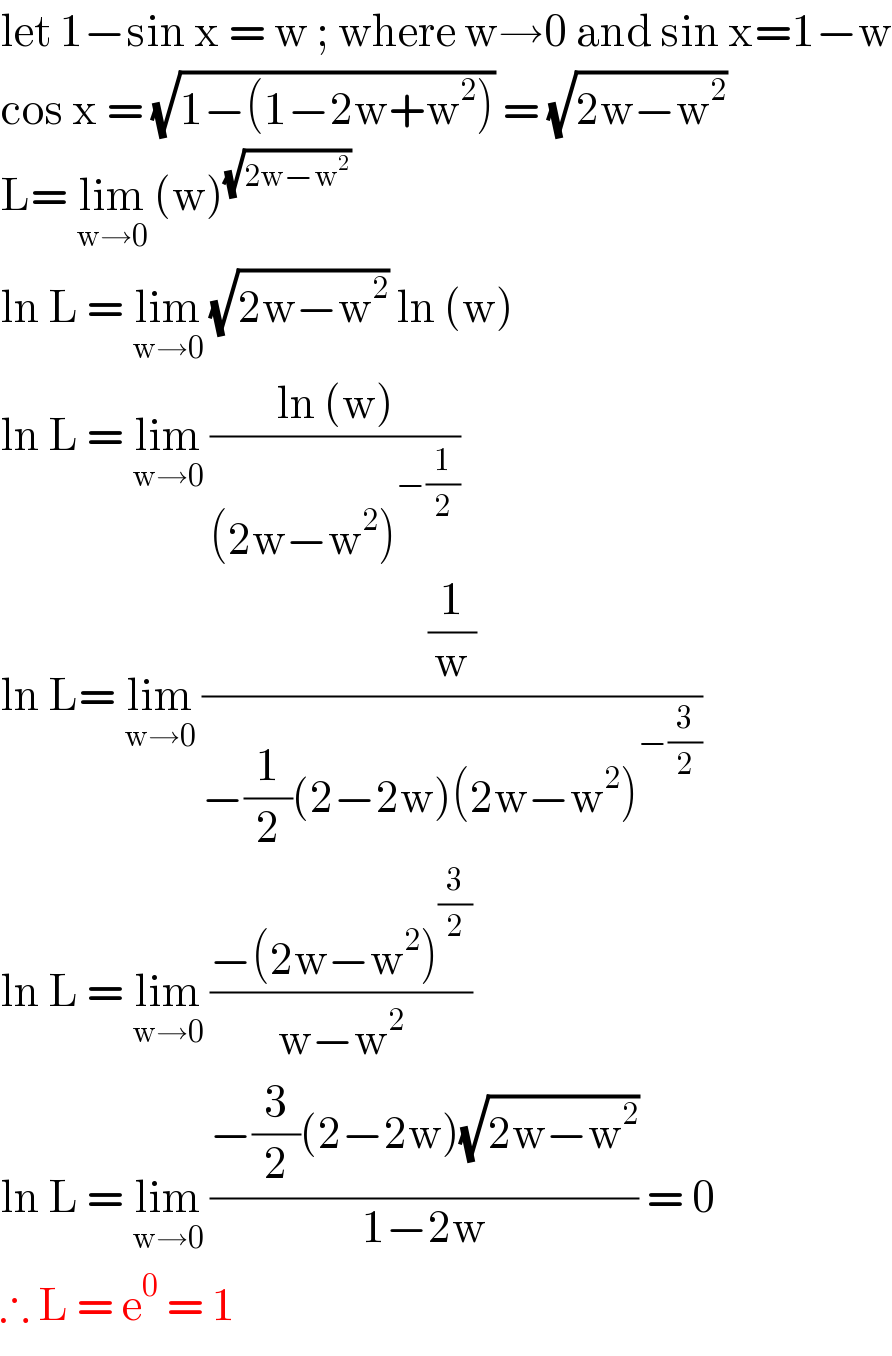
Answered by Dwaipayan Shikari last updated on 04/Sep/20
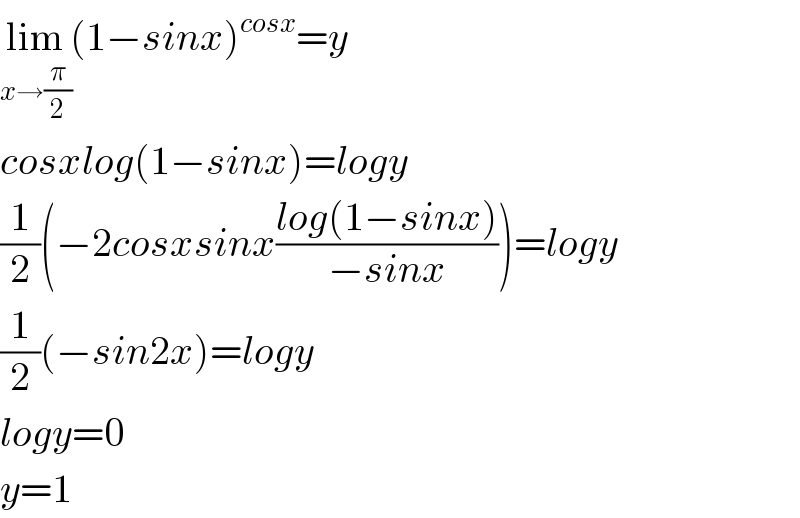
Answered by 1549442205PVT last updated on 04/Sep/20
![I= lim_(x→(π/2)) (1−sin x)^(cos x) lnI=lim_(x→(π/2)) [cosxln(1−sinx)] =lim_(x→(π/2)) ((ln(1−sinx))/(1/(cosx)))=lim_(x→(π/2)) (((1/(1−sinx))×(−cosx))/((sinx)/(cos^2 x))) (Since lim_(x→(π/2)) ((ln(1−sinx))/(1/(cosx)))=(∞/∞))⇒using L′Hopital =lim_(x→(π/2)) ((−cos^3 x)/((1−sinx)sinx))=lim_(x→π/2) ((3cos^2 xsinx)/((1−sinx)cosx−cosxsinx)) =lim_(x→(π/2)) ((3cosxsinx)/(1−2sinx))=(0/(−1))=0⇒I=e^0 =1](Q111582.png)
| ||
Question and Answers Forum | ||
Question Number 111528 by bemath last updated on 04/Sep/20 | ||
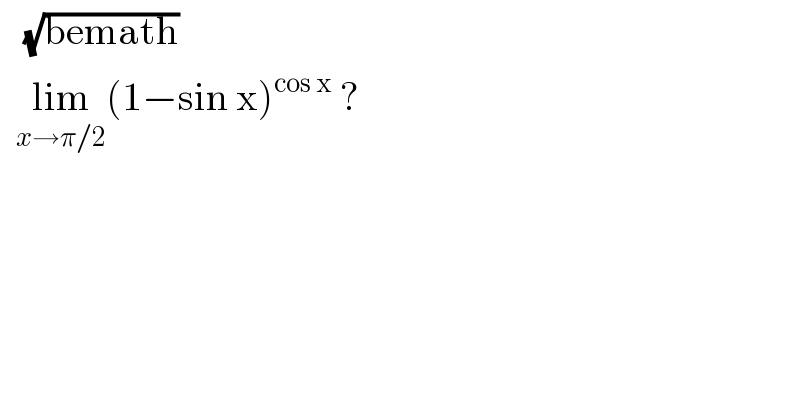 | ||
Answered by bobhans last updated on 04/Sep/20 | ||
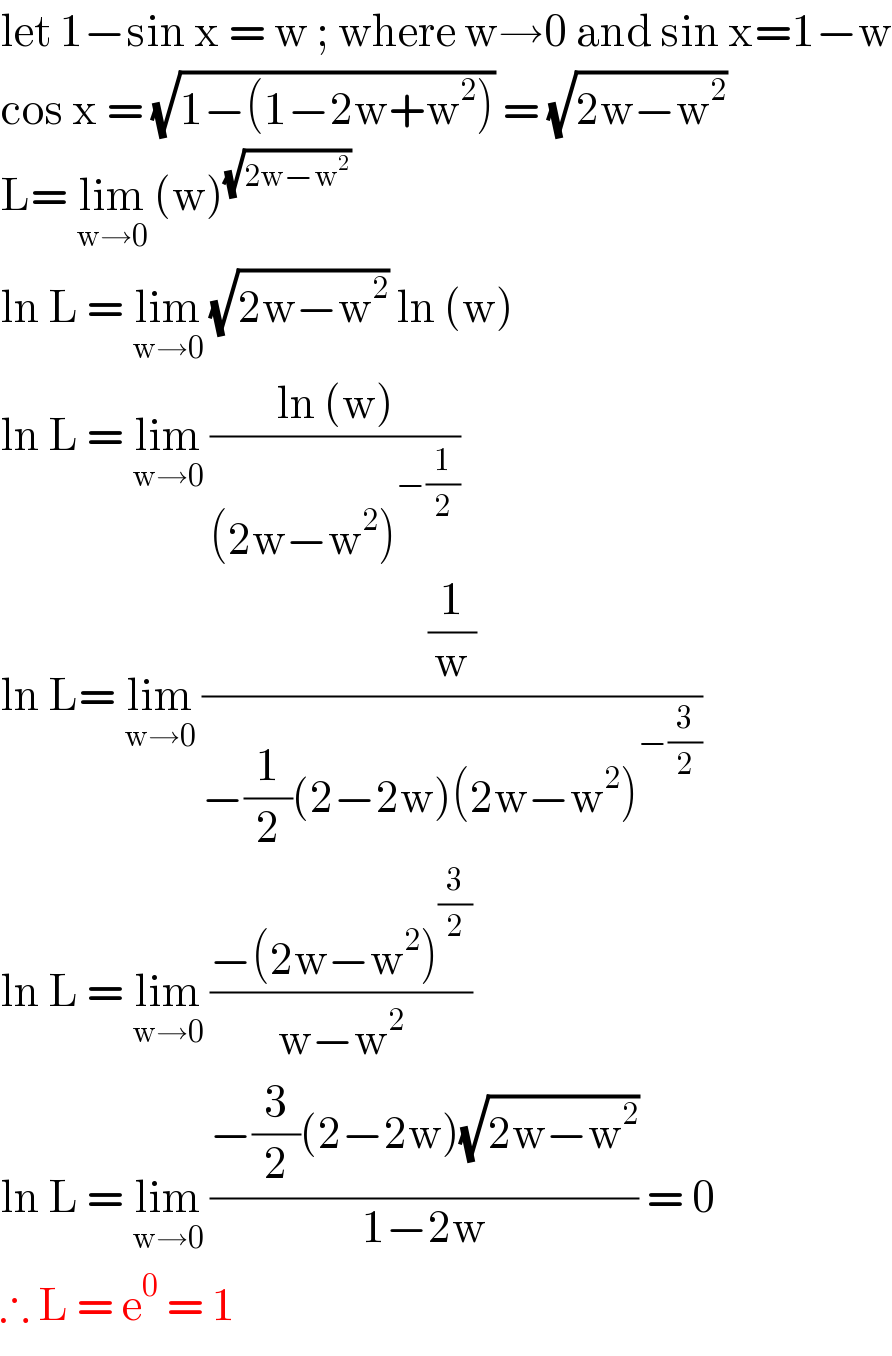 | ||
| ||
Answered by Dwaipayan Shikari last updated on 04/Sep/20 | ||
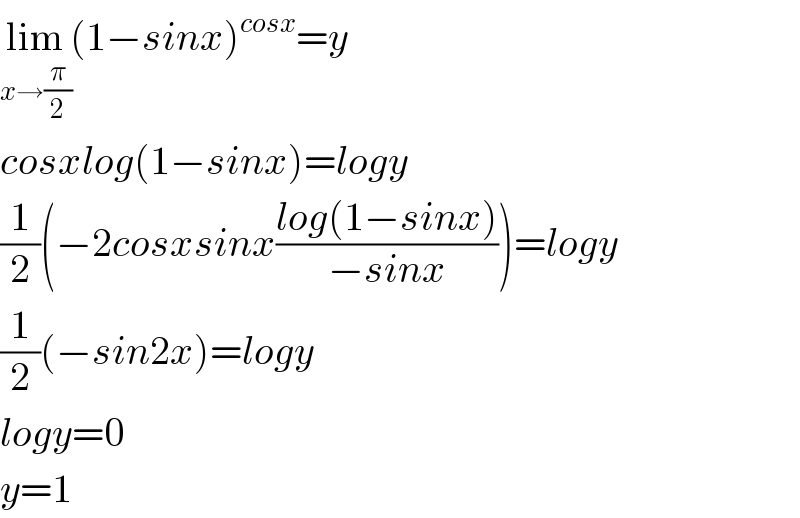 | ||
| ||
Answered by 1549442205PVT last updated on 04/Sep/20 | ||
![I= lim_(x→(π/2)) (1−sin x)^(cos x) lnI=lim_(x→(π/2)) [cosxln(1−sinx)] =lim_(x→(π/2)) ((ln(1−sinx))/(1/(cosx)))=lim_(x→(π/2)) (((1/(1−sinx))×(−cosx))/((sinx)/(cos^2 x))) (Since lim_(x→(π/2)) ((ln(1−sinx))/(1/(cosx)))=(∞/∞))⇒using L′Hopital =lim_(x→(π/2)) ((−cos^3 x)/((1−sinx)sinx))=lim_(x→π/2) ((3cos^2 xsinx)/((1−sinx)cosx−cosxsinx)) =lim_(x→(π/2)) ((3cosxsinx)/(1−2sinx))=(0/(−1))=0⇒I=e^0 =1](Q111582.png) | ||
| ||