Question Number 111617 by mathdave last updated on 04/Sep/20
$${show}\:{that} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{ln}\left(\mathrm{2cos}^{\mathrm{2}} {x}\right)}{\mathrm{cos2}{x}}{dx}=\frac{\pi^{\mathrm{2}} }{\mathrm{16}} \\ $$
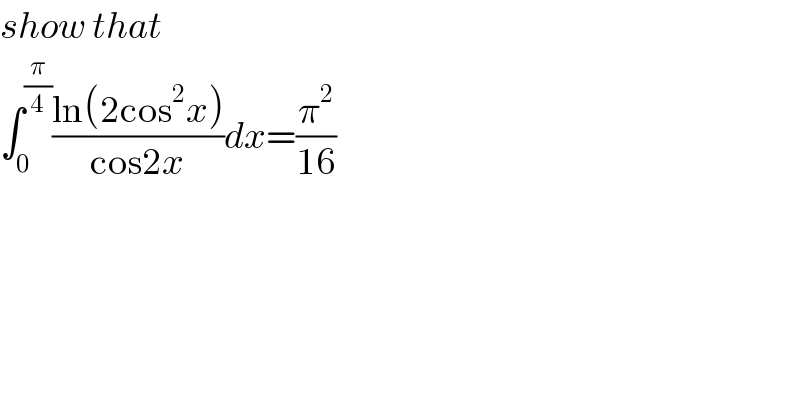
Answered by mathmax by abdo last updated on 04/Sep/20
![A =∫_0 ^(π/4) ((ln(2cos^2 x))/(cos(2x)))dx ⇒ A =∫_0 ^(π/4) ((ln(1+cos(2x)))/(cos(2x)))dx =_(2x=t) ∫_0 ^(π/2) ((ln(1+cost))/(cost)) dt let f(a) =∫_0 ^(π/2) ((ln(1+acost))/(cost)) dt (o<a<1) we have f^′ (a) =∫_0 ^(π/2) ((cost)/((1+acost)cost))dt =∫_0 ^(π/2) (dt/(1+acost)) =_(tan((t/2))=u) ∫_0 ^1 ((2du)/((1+u^2 )(1+a((1−u^2 )/(1+u^2 ))))) =2∫_0 ^1 (du/(1+u^2 +a−au^2 )) =2∫_0 ^1 (du/((1−a)u^2 +1+a)) =(2/(1−a)) ∫_0 ^1 (du/(u^2 +((1+a)/(1−a)))) =_(u=(√((1+a)/(1−a)))z) (2/(1−a))×((1−a)/(1+a)) ∫_0 ^(√((1−a)/(1+a))) (1/(1+z^2 ))×((√(1+a))/(√(1−a))) dz =(2/(√(1−a^2 ))) arctan(√((1−a)/(1+a))) ⇒ f(a) =2∫_0 ^a ((arctan(√((1−t)/(1+t))))/(√(1−t^2 ))) dt +c (c=f(0) =0) ⇒ f(a) =2∫_0 ^a ((arctan(√((1−t)/(1+t))))/(√(1−t^2 ))) dt and ∫_0 ^(π/2) ((ln(1+cosx))/(cosx)) dx =lim_(a→1) f(a) =2∫_0 ^1 ((arctan(√((1−t)/(1+t))))/(√(1−t^2 ))) dt =_(t =cosθ) 2∫_(π/2) ^0 ((arctan(√((2sin^2 ((θ/2)))/(2cos^2 ((θ/2))))))/(sinθ))(−sinθ)dθ =2∫_0 ^(π/2) arctan(tan((θ/2)))dθ =2 ∫_0 ^(π/2) (θ/2) dθ =[(θ^2 /2)]_0 ^(π/2) =(π^2 /8) ⇒ ★∫_0 ^(π/4) ((ln(2cos^2 x))/(cos(2x)))dx =(π^2 /8) ★](Q111681.png)
$$\mathrm{A}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{ln}\left(\mathrm{2cos}^{\mathrm{2}} \mathrm{x}\right)}{\mathrm{cos}\left(\mathrm{2x}\right)}\mathrm{dx}\:\Rightarrow\:\mathrm{A}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{cos}\left(\mathrm{2x}\right)\right)}{\mathrm{cos}\left(\mathrm{2x}\right)}\mathrm{dx} \\ $$$$=_{\mathrm{2x}=\mathrm{t}} \:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{cost}\right)}{\mathrm{cost}}\:\mathrm{dt}\:\:\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{acost}\right)}{\mathrm{cost}}\:\mathrm{dt}\:\:\left(\mathrm{o}<\mathrm{a}<\mathrm{1}\right) \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{f}^{'} \left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cost}}{\left(\mathrm{1}+\mathrm{acost}\right)\mathrm{cost}}\mathrm{dt}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dt}}{\mathrm{1}+\mathrm{acost}} \\ $$$$=_{\mathrm{tan}\left(\frac{\mathrm{t}}{\mathrm{2}}\right)=\mathrm{u}} \:\:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2du}}{\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{a}\frac{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\right)}\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{du}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} \:+\mathrm{a}−\mathrm{au}^{\mathrm{2}} } \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{du}}{\left(\mathrm{1}−\mathrm{a}\right)\mathrm{u}^{\mathrm{2}} \:+\mathrm{1}+\mathrm{a}}\:=\frac{\mathrm{2}}{\mathrm{1}−\mathrm{a}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} \:+\frac{\mathrm{1}+\mathrm{a}}{\mathrm{1}−\mathrm{a}}} \\ $$$$=_{\mathrm{u}=\sqrt{\frac{\mathrm{1}+\mathrm{a}}{\mathrm{1}−\mathrm{a}}}\mathrm{z}} \:\:\:\:\frac{\mathrm{2}}{\mathrm{1}−\mathrm{a}}×\frac{\mathrm{1}−\mathrm{a}}{\mathrm{1}+\mathrm{a}}\:\int_{\mathrm{0}} ^{\sqrt{\frac{\mathrm{1}−\mathrm{a}}{\mathrm{1}+\mathrm{a}}}} \:\:\:\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }×\frac{\sqrt{\mathrm{1}+\mathrm{a}}}{\sqrt{\mathrm{1}−\mathrm{a}}}\:\mathrm{dz} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\:\mathrm{arctan}\sqrt{\frac{\mathrm{1}−\mathrm{a}}{\mathrm{1}+\mathrm{a}}}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{a}} \:\frac{\mathrm{arctan}\sqrt{\frac{\mathrm{1}−\mathrm{t}}{\mathrm{1}+\mathrm{t}}}}{\sqrt{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }}\:\mathrm{dt}\:+\mathrm{c}\:\:\:\left(\mathrm{c}=\mathrm{f}\left(\mathrm{0}\right)\:=\mathrm{0}\right)\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{a}} \:\:\frac{\mathrm{arctan}\sqrt{\frac{\mathrm{1}−\mathrm{t}}{\mathrm{1}+\mathrm{t}}}}{\sqrt{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }}\:\mathrm{dt}\:\mathrm{and}\: \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{cosx}\right)}{\mathrm{cosx}}\:\mathrm{dx}\:=\mathrm{lim}_{\mathrm{a}\rightarrow\mathrm{1}} \mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arctan}\sqrt{\frac{\mathrm{1}−\mathrm{t}}{\mathrm{1}+\mathrm{t}}}}{\sqrt{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }}\:\mathrm{dt} \\ $$$$=_{\mathrm{t}\:=\mathrm{cos}\theta} \:\:\:\mathrm{2}\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{0}} \:\frac{\mathrm{arctan}\sqrt{\frac{\mathrm{2sin}^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}{\mathrm{2cos}^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}}}{\mathrm{sin}\theta}\left(−\mathrm{sin}\theta\right)\mathrm{d}\theta \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{arctan}\left(\mathrm{tan}\left(\frac{\theta}{\mathrm{2}}\right)\right)\mathrm{d}\theta\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\theta}{\mathrm{2}}\:\mathrm{d}\theta\:\:=\left[\frac{\theta^{\mathrm{2}} }{\mathrm{2}}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:\Rightarrow \\ $$$$\bigstar\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{ln}\left(\mathrm{2cos}^{\mathrm{2}} \mathrm{x}\right)}{\mathrm{cos}\left(\mathrm{2x}\right)}\mathrm{dx}\:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:\bigstar \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 04/Sep/20
$$\mathrm{sorry}\:\mathrm{error}\:\mathrm{at}\:\mathrm{line}\:\mathrm{2}\:\:\mathrm{we}\:\mathrm{have}\:\:\:\mathrm{A}\:=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{cosx}\right)}{\mathrm{cosx}}\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{A}\:=\frac{\pi^{\mathrm{2}} }{\mathrm{16}} \\ $$
Commented by Tawa11 last updated on 06/Sep/21
$$\mathrm{great}\:\mathrm{sir} \\ $$
Answered by mathdave last updated on 04/Sep/20
![solution from 2cos^2 x=1+cos2x I=∫_0 ^(π/4) ((ln(2cos^2 x))/(cos2x))dx=∫_0 ^(π/4) ((ln(1+cos2x))/(cos2x))dx let y=2x I=(1/2)∫_0 ^(π/2) ((ln(cosy))/(cosy))dy using Duis let I(α)=(1/2)∫_0 ^(π/2) ((ln(1+αcosy))/(cosy))dy I^′ (α)=(1/2)∫_0 ^(π/2) ∫_0 ^1 (1/(1+αcosy))dαdy but cosy=((1−tan^2 (y/2))/(1+tan^2 (y/2)))=((1−t^2 )/(1+t^2 )) let t=tan(y/2) and dy=((2dt)/(1+t^2 )) I=(1/2)∫_0 ^(π/2) ∫_0 ^1 (1/(1+α(((1−t^2 )/(1+t^2 )))))×((2dt)/(1+t^2 ))dtdα I=∫_0 ^(π/2) ∫_0 ^1 (1/((1+t^2 +α−αt^2 )))dtdα=∫_0 ^(π/2) ∫_0 ^1 (1/((1+α)+(1−α)t^2 ))dtdα I(α)=∫_0 ^(π/2) (1/(1−α))∫_0 ^1 (1/(((√((1+α)/(1−α))))^2 +t^2 ))dαdt but t=tan(y/2)=tan(π/4)=1 I(α)=∫_0 ^1 (1/(1−α))•(1/(√((1+α)/(1−α))))tan^(−1) [(t/(√((1+α)/(1−α))))]_0 ^(π/2) dα I(α)=∫_0 ^1 (1/(1−α))•((√(1−α^2 ))/(1+α))tan^(−1) [(1/(√((1+α)/(1−α))))]dα I(α)=∫_0 ^1 (1/(√(1−α^2 )))tan^(−1) [(√((1−α)/(1+α)))]dα let α=cosy and dα=−sinydy and tan(y/2)=(√((1−cosy)/(1+cosy)))=(√((1−α)/(1+α))) =∫_(π/2) ^0 (1/(siny))tan^(−1) [(√((1−cosy)/(1+cosy)))](−siny)dy =(1/2)∫_0 ^(π/2) tan^(−1) [tan(y/2)]dy=∫_0 ^(π/2) (y/2)dy=(1/2)[(y^2 /2)]_0 ^(π/2) =(1/4)[((π/2))^2 ]=(π^2 /(16)) ∫_0 ^(π/4) ((ln(2cos^2 x))/(cos2x))dx=(π^2 /(16)) Q.E.D by mathdave(04/09/2020)](Q111718.png)
$${solution} \\ $$$${from}\:\mathrm{2cos}^{\mathrm{2}} {x}=\mathrm{1}+\mathrm{cos2}{x} \\ $$$${I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{ln}\left(\mathrm{2cos}^{\mathrm{2}} {x}\right)}{\mathrm{cos2}{x}}{dx}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{cos2}{x}\right)}{\mathrm{cos2}{x}}{dx} \\ $$$${let}\:{y}=\mathrm{2}{x} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{ln}\left(\mathrm{cos}{y}\right)}{\mathrm{cos}{y}}{dy}\:\:\:\:\:{using}\:{Duis} \\ $$$${let}\:{I}\left(\alpha\right)=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{ln}\left(\mathrm{1}+\alpha\mathrm{cos}{y}\right)}{\mathrm{cos}{y}}{dy} \\ $$$${I}^{'} \left(\alpha\right)=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{1}+\alpha\mathrm{cos}{y}}{d}\alpha{dy} \\ $$$${but}\:\mathrm{cos}{y}=\frac{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \frac{{y}}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \frac{{y}}{\mathrm{2}}}=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }\:\:\:\:\:\:{let}\:\:{t}=\mathrm{tan}\frac{{y}}{\mathrm{2}}\:\:{and}\:\:{dy}=\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{1}+\alpha\left(\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }\right)}×\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }{dtd}\alpha \\ $$$${I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\left(\mathrm{1}+{t}^{\mathrm{2}} +\alpha−\alpha{t}^{\mathrm{2}} \right)}{dtd}\alpha=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\left(\mathrm{1}+\alpha\right)+\left(\mathrm{1}−\alpha\right){t}^{\mathrm{2}} }{dtd}\alpha \\ $$$${I}\left(\alpha\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{1}}{\mathrm{1}−\alpha}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\left(\sqrt{\frac{\mathrm{1}+\alpha}{\mathrm{1}−\alpha}}\right)^{\mathrm{2}} +{t}^{\mathrm{2}} }{d}\alpha{dt} \\ $$$${but}\:{t}=\mathrm{tan}\frac{{y}}{\mathrm{2}}=\mathrm{tan}\frac{\pi}{\mathrm{4}}=\mathrm{1} \\ $$$${I}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{1}−\alpha}\bullet\frac{\mathrm{1}}{\sqrt{\frac{\mathrm{1}+\alpha}{\mathrm{1}−\alpha}}}\mathrm{tan}^{−\mathrm{1}} \left[\frac{{t}}{\sqrt{\frac{\mathrm{1}+\alpha}{\mathrm{1}−\alpha}}}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {d}\alpha \\ $$$${I}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{1}−\alpha}\bullet\frac{\sqrt{\mathrm{1}−\alpha^{\mathrm{2}} }}{\mathrm{1}+\alpha}\mathrm{tan}^{−\mathrm{1}} \left[\frac{\mathrm{1}}{\sqrt{\frac{\mathrm{1}+\alpha}{\mathrm{1}−\alpha}}}\right]{d}\alpha \\ $$$${I}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\sqrt{\mathrm{1}−\alpha^{\mathrm{2}} }}\mathrm{tan}^{−\mathrm{1}} \left[\sqrt{\frac{\mathrm{1}−\alpha}{\mathrm{1}+\alpha}}\right]{d}\alpha \\ $$$${let}\:\:\alpha=\mathrm{cos}{y}\:\:\:{and}\:{d}\alpha=−\mathrm{sin}{ydy} \\ $$$${and}\:\mathrm{tan}\frac{{y}}{\mathrm{2}}=\sqrt{\frac{\mathrm{1}−\mathrm{cos}{y}}{\mathrm{1}+\mathrm{cos}{y}}}=\sqrt{\frac{\mathrm{1}−\alpha}{\mathrm{1}+\alpha}} \\ $$$$=\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{0}} \frac{\mathrm{1}}{\mathrm{sin}{y}}\mathrm{tan}^{−\mathrm{1}} \left[\sqrt{\frac{\mathrm{1}−\mathrm{cos}{y}}{\mathrm{1}+\mathrm{cos}{y}}}\right]\left(−\mathrm{sin}{y}\right){dy} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{tan}^{−\mathrm{1}} \left[\mathrm{tan}\frac{{y}}{\mathrm{2}}\right]{dy}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{y}}{\mathrm{2}}{dy}=\frac{\mathrm{1}}{\mathrm{2}}\left[\frac{{y}^{\mathrm{2}} }{\mathrm{2}}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\left[\left(\frac{\pi}{\mathrm{2}}\right)^{\mathrm{2}} \right]=\frac{\pi^{\mathrm{2}} }{\mathrm{16}} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{ln}\left(\mathrm{2cos}^{\mathrm{2}} {x}\right)}{\mathrm{cos2}{x}}{dx}=\frac{\pi^{\mathrm{2}} }{\mathrm{16}}\:\:\:\:\:\:\:\:{Q}.{E}.{D} \\ $$$${by}\:{mathdave}\left(\mathrm{04}/\mathrm{09}/\mathrm{2020}\right) \\ $$