Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 112060 by Aina Samuel Temidayo last updated on 06/Sep/20

In a trapezium, ABCD, with AB parallel to CD. If M is the midpoint of line segment AD and P is a point on line BC such that MP is perpendicular to BC. Show that, we need only the lengths of line segments MP and BC to calculate the area ABCD.

$$\mathrm{In}\:\mathrm{a}\:\mathrm{trapezium},\:\mathrm{ABCD},\:\mathrm{with}\:\mathrm{AB} \\ $$$$\mathrm{parallel}\:\mathrm{to}\:\mathrm{CD}.\:\mathrm{If}\:\mathrm{M}\:\mathrm{is}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of} \\ $$$$\mathrm{line}\:\mathrm{segment}\:\mathrm{AD}\:\mathrm{and}\:\mathrm{P}\:\mathrm{is}\:\mathrm{a}\:\mathrm{point}\:\mathrm{on} \\ $$$$\mathrm{line}\:\mathrm{BC}\:\mathrm{such}\:\mathrm{that}\:\mathrm{MP}\:\mathrm{is}\:\mathrm{perpendicular} \\ $$$$\mathrm{to}\:\mathrm{BC}.\:\mathrm{Show}\:\mathrm{that},\:\mathrm{we}\:\mathrm{need}\:\mathrm{only}\:\mathrm{the} \\ $$$$\mathrm{lengths}\:\mathrm{of}\:\mathrm{line}\:\mathrm{segments}\:\mathrm{MP}\:\mathrm{and}\:\mathrm{BC} \\ $$$$\mathrm{to}\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{area}\:\mathrm{ABCD}. \\ $$

Answered by 1549442205PVT last updated on 06/Sep/20

Commented by Aina Samuel Temidayo last updated on 06/Sep/20

Thanks. Please what about the solution?

$$\mathrm{Thanks}.\:\mathrm{Please}\:\mathrm{what}\:\mathrm{about}\:\mathrm{the} \\ $$$$\mathrm{solution}? \\ $$

Commented by 1549442205PVT last updated on 06/Sep/20

Denote by G midpoint of BC Suppose known MP=m,BC=a,then S_(BMC) =((BC.MP)/2)=((am)/2).We prove that S_(ABCD) =2S_(BMC) .Indeed,Denote byI,H orthogonal projections of points B and C on MG respectively.Then S_(CMG) = ((MG.CH)/2),S_(BMG) =((MG.BI)/2) S_(BMC) =S_(CMG) +S_(BMG) =((MG(BI+CH))/2)(1) Since M,G are midpoints of BC and AD ,MG is midline of the trapezium ABCD.Since AB//CD,BI+CH equal to altitude h of the trapezium . Therefore,MG=((AB+CD)/2),BI+CH=h and S_(ABCD) =(((AB+CD).h)/2)=MG.h(2) From (1) and (2) we infer S_(ABCD) =2S_(BMC) =a.m .That thing show that only need know the length of the line segments BC and MP we can calculate the area of the trapezium

$$\mathrm{Denote}\:\mathrm{by}\:\mathrm{G}\:\mathrm{midpoint}\:\mathrm{of}\:\mathrm{BC}\: \\ $$$$\mathrm{Suppose}\:\mathrm{known}\:\mathrm{MP}=\mathrm{m},\mathrm{BC}=\mathrm{a},\mathrm{then} \\ $$$$\mathrm{S}_{\mathrm{BMC}} =\frac{\mathrm{BC}.\mathrm{MP}}{\mathrm{2}}=\frac{\mathrm{am}}{\mathrm{2}}.\mathrm{We}\:\mathrm{prove}\:\mathrm{that} \\ $$$$\mathrm{S}_{\mathrm{ABCD}} =\mathrm{2S}_{\mathrm{BMC}} .\mathrm{Indeed},\mathrm{Denote}\:\mathrm{byI},\mathrm{H} \\ $$$$\mathrm{orthogonal}\:\mathrm{projections}\:\mathrm{of}\:\mathrm{points}\:\mathrm{B}\:\mathrm{and} \\ $$$$\mathrm{C}\:\mathrm{on}\:\mathrm{MG}\:\mathrm{respectively}.\mathrm{Then}\:\mathrm{S}_{\mathrm{CMG}} = \\ $$$$\frac{\mathrm{MG}.\mathrm{CH}}{\mathrm{2}},\mathrm{S}_{\mathrm{BMG}} =\frac{\mathrm{MG}.\mathrm{BI}}{\mathrm{2}} \\ $$$$\mathrm{S}_{\mathrm{BMC}} =\mathrm{S}_{\mathrm{CMG}} +\mathrm{S}_{\mathrm{BMG}} =\frac{\mathrm{MG}\left(\mathrm{BI}+\mathrm{CH}\right)}{\mathrm{2}}\left(\mathrm{1}\right) \\ $$$$\mathrm{Since}\:\mathrm{M},\mathrm{G}\:\mathrm{are}\:\mathrm{midpoints}\:\mathrm{of}\:\mathrm{BC}\:\mathrm{and} \\ $$$$\mathrm{AD}\:,\mathrm{MG}\:\mathrm{is}\:\mathrm{midline}\:\mathrm{of}\:\mathrm{the}\:\mathrm{trapezium} \\ $$$$\mathrm{ABCD}.\mathrm{Since}\:\mathrm{AB}//\mathrm{CD},\mathrm{BI}+\mathrm{CH}\:\mathrm{equal} \\ $$$$\mathrm{to}\:\mathrm{altitude}\:\mathrm{h}\:\mathrm{of}\:\mathrm{the}\:\mathrm{trapezium}\:. \\ $$$$\mathrm{Therefore},\mathrm{MG}=\frac{\mathrm{AB}+\mathrm{CD}}{\mathrm{2}},\mathrm{BI}+\mathrm{CH}=\mathrm{h} \\ $$$$\mathrm{and}\:\mathrm{S}_{\mathrm{ABCD}} =\frac{\left(\mathrm{AB}+\mathrm{CD}\right).\mathrm{h}}{\mathrm{2}}=\mathrm{MG}.\mathrm{h}\left(\mathrm{2}\right) \\ $$$$\mathrm{From}\:\left(\mathrm{1}\right)\:\mathrm{and}\:\left(\mathrm{2}\right)\:\mathrm{we}\:\mathrm{infer} \\ $$$$\mathrm{S}_{\mathrm{ABCD}} =\mathrm{2S}_{\mathrm{BMC}} =\mathrm{a}.\mathrm{m}\:.\mathrm{That}\:\mathrm{thing}\:\mathrm{show} \\ $$$$\mathrm{that}\:\mathrm{only}\:\mathrm{need}\:\mathrm{know}\:\mathrm{the}\:\mathrm{length}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{line}\:\mathrm{segments}\:\mathrm{BC}\:\mathrm{and}\:\mathrm{MP}\:\mathrm{we}\:\mathrm{can} \\ $$$$\mathrm{calculate}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{trapezium} \\ $$

Commented by Aina Samuel Temidayo last updated on 06/Sep/20

Please check my other questions out. I need help please.

$$\mathrm{Please}\:\mathrm{check}\:\mathrm{my}\:\mathrm{other}\:\mathrm{questions}\:\mathrm{out}.\:\mathrm{I} \\ $$$$\mathrm{need}\:\mathrm{help}\:\mathrm{please}. \\ $$

Commented by Aina Samuel Temidayo last updated on 06/Sep/20

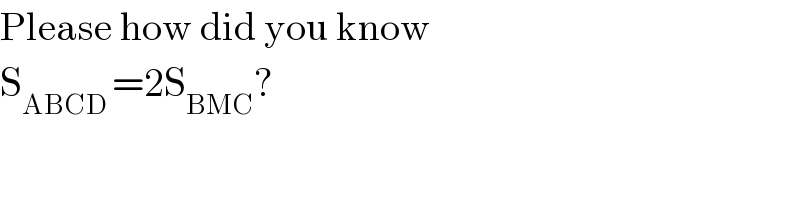
Please how did you know S_(ABCD ) =2S_(BMC) ?

$$\mathrm{Please}\:\mathrm{how}\:\mathrm{did}\:\mathrm{you}\:\mathrm{know} \\ $$$$\mathrm{S}_{\mathrm{ABCD}\:} =\mathrm{2S}_{\mathrm{BMC}} ? \\ $$

Commented by 1549442205PVT last updated on 06/Sep/20

I proved above!It follows from(1)&(2)

$$\mathrm{I}\:\mathrm{proved}\:\mathrm{above}!\mathrm{It}\:\mathrm{follows}\:\mathrm{from}\left(\mathrm{1}\right)\&\left(\mathrm{2}\right) \\ $$

Commented by Aina Samuel Temidayo last updated on 06/Sep/20

Ok. Thanks. Please check the question I just posted.

$$\mathrm{Ok}.\:\mathrm{Thanks}.\:\mathrm{Please}\:\mathrm{check}\:\mathrm{the} \\ $$$$\mathrm{question}\:\mathrm{I}\:\mathrm{just}\:\mathrm{posted}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com