find ∫_0 ^1 ((ln(1+x^2 ))/(1+x))dx


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 112446 by mathmax by abdo last updated on 07/Sep/20

$find \int_{0}^{1} \frac{\ln (1 + x^{2})}{1 + x} dx$
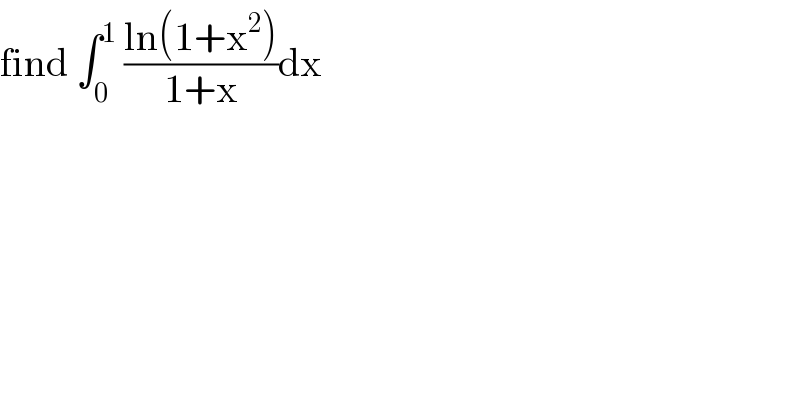
	Answered by mathdave last updated on 08/Sep/20

	$s o l u t i o n t o$ $l e t I = \int_{0}^{1} \frac{\ln (1 + x^{2})}{1 + x} d x u s i n g I B P$ $I =∣ \ln (1 + x) \ln (1 + x^{2}) ∣_{0}^{1} - 2 \int_{0}^{1} \frac{x \ln (1 + x)}{1 + x^{2}} d x$ $I = \ln^{2} (2) - 2 I_{1} . . . . . . . . (1)$ $L e t$ $I_{1} = \int_{0}^{1} \frac{x \ln (1 + x)}{1 + x^{2}} d x = \int_{0}^{1} [\frac{(1 + x^{2} - 1)}{1 + x^{2}}] \frac{\ln (1 + x)}{x} d x$ $I_{1} = \int_{0}^{1} \frac{\ln (1 + x)}{x} d x - \int_{0}^{1} \frac{\ln (1 + x)}{x (1 + x^{2})} d x$ $a d o p t f a y n m a n n t r i c k$ $I_{1} = \int_{0}^{1} \frac{\ln (1 + x)}{x} d x - \int_{0}^{1} \frac{1}{1 + x^{2}} (\int_{0}^{1} \frac{1}{1 + x y} d y) d x$ $I_{1} = η (2) - \int_{0}^{1} \frac{1}{1 + y^{2}} \int_{0}^{1} [\frac{y^{2}}{1 + x y} + \frac{1 - x y}{1 + x^{2}}] d x d y$ $I_{1} = \frac{π^{2}}{12} - \int_{0}^{1} \frac{1}{1 + y^{2}} [y \ln (1 + y) + \frac{π}{4} - \frac{y}{2} \ln 2] d y$ $I_{1} = \frac{π^{2}}{12} - \int_{0}^{1} \frac{y \ln (1 + y)}{1 + y^{2}} - \frac{π}{4} \int_{0}^{1} \frac{1}{1 + y^{2}} d y + \frac{1}{2} \ln 2 \int_{0}^{1} \frac{y}{1 + y^{2}} d y$ $I_{1} = \frac{π^{2}}{12} - I_{1} - \frac{π^{2}}{16} + \frac{\ln^{2} (2)}{4}$ $2 I_{1} = \frac{π^{2}}{48} + \frac{\ln^{2} (2)}{4}$ $∵ I_{1} = \frac{π^{2}}{96} + \frac{\ln^{2} (2)}{8}$ $r e c a l l t h a t I = \ln^{2} (2) - 2 I_{1} . . . . . . . . . (1)$ $∵ I = \ln^{2} (2) - 2 [\frac{π^{2}}{96} + \frac{\ln^{2} (2)}{8}]$ $I = \ln^{2} (2) - \frac{. π^{2}}{48} - \frac{\ln^{2} (2)}{4}$ $I = \frac{3}{4} \ln^{2} (2) - \frac{π^{2}}{48}$ $∵ \int_{0}^{1} \frac{\ln (1 + x^{2})}{1 + x} d x = \frac{3}{4} \ln^{2} (2) - \frac{π^{2}}{48}$ $b y m a t h d a v e (08 / 09 / 2020)$
	Commented by mathmax by abdo last updated on 08/Sep/20

	$thanks$
	Commented by Tawa11 last updated on 06/Sep/21

	$great sir$
	Answered by mnjuly1970 last updated on 08/Sep/20

	Commented by mathmax by abdo last updated on 08/Sep/20

	$thanks$
	Commented by mnjuly1970 last updated on 08/Sep/20

	$g r a t e f u l s i r . t o m e$ $y o u a r e^{'} {o s t a d}^{'} (i n o u r$ $l a n g u a g e m e a n s^{'} m a s t e r$ $a n d {s c h o l a r}^{'} i n h i s f i e l d)$ $i n m a t h e m a t i c s . t h a n k$ $y o u s o m u c h s i r f o r y o u r$ $e f f o r t . . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum