Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 114299 by bemath last updated on 18/Sep/20

solve lim_(p→∞) (((p^2 +2)/(p+2)))^((p+2)/(p^2 +2)) ?

$$\:{solve}\:\underset{{p}\rightarrow\infty} {\mathrm{lim}}\:\left(\frac{{p}^{\mathrm{2}} +\mathrm{2}}{{p}+\mathrm{2}}\right)^{\frac{{p}+\mathrm{2}}{{p}^{\mathrm{2}} +\mathrm{2}}} \:? \\ $$

Commented by mohammad17 last updated on 18/Sep/20

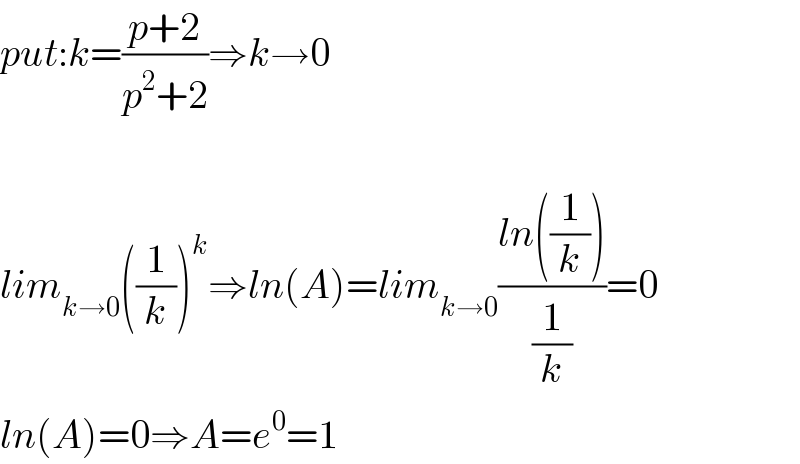
put:k=((p+2)/(p^2 +2))⇒k→0 lim_(k→0) ((1/k))^k ⇒ln(A)=lim_(k→0) ((ln((1/k)))/(1/k))=0 ln(A)=0⇒A=e^0 =1

$${put}:{k}=\frac{{p}+\mathrm{2}}{{p}^{\mathrm{2}} +\mathrm{2}}\Rightarrow{k}\rightarrow\mathrm{0} \\ $$$$ \\ $$$${lim}_{{k}\rightarrow\mathrm{0}} \left(\frac{\mathrm{1}}{{k}}\right)^{{k}} \Rightarrow{ln}\left({A}\right)={lim}_{{k}\rightarrow\mathrm{0}} \frac{{ln}\left(\frac{\mathrm{1}}{{k}}\right)}{\frac{\mathrm{1}}{{k}}}=\mathrm{0} \\ $$$${ln}\left({A}\right)=\mathrm{0}\Rightarrow{A}={e}^{\mathrm{0}} =\mathrm{1} \\ $$

Answered by 1549442205PVT last updated on 18/Sep/20

I=lim (_(p→∞t) ((p^2 +2)/(p+2)))^((p+2)/(p^2 +2)) lnI=lim_(p→∞) [((p+2)/(p^2 +2))ln(((p^2 +2)/(p+2)))] =lim_(p→∞) ((ln(((p^2 +2)/(p+2))))/((p^2 +2)/(p+2))).This is the form(∞/∞) Applying L′Hopital rule we have lnI=lim_(p→∞) ((((p+2)/(p^2 +2))×((2p(p+2)−(p^2 +2))/((p+2)^2 )))/((2p(p+2)−(p^2 +2))/((p+2)^2 ))) =lim_(p→∞) ((p+2)/(p^2 +2))=0⇒I=e^0 =1

$$\mathrm{I}=\underset{\mathrm{p}\rightarrow\infty\mathrm{t}} {\mathrm{lim}\:\left(}\frac{{p}^{\mathrm{2}} +\mathrm{2}}{{p}+\mathrm{2}}\right)^{\frac{{p}+\mathrm{2}}{{p}^{\mathrm{2}} +\mathrm{2}}} \: \\ $$$$\mathrm{lnI}=\underset{\mathrm{p}\rightarrow\infty} {\mathrm{lim}}\left[\frac{\mathrm{p}+\mathrm{2}}{\mathrm{p}^{\mathrm{2}} +\mathrm{2}}\mathrm{ln}\left(\frac{\mathrm{p}^{\mathrm{2}} +\mathrm{2}}{\mathrm{p}+\mathrm{2}}\right)\right] \\ $$$$=\underset{\mathrm{p}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{ln}\left(\frac{\mathrm{p}^{\mathrm{2}} +\mathrm{2}}{\mathrm{p}+\mathrm{2}}\right)}{\frac{\mathrm{p}^{\mathrm{2}} +\mathrm{2}}{\mathrm{p}+\mathrm{2}}}.\mathrm{This}\:\mathrm{is}\:\mathrm{the}\:\mathrm{form}\frac{\infty}{\infty} \\ $$$$\mathrm{Applying}\:\mathrm{L}'\mathrm{Hopital}\:\mathrm{rule}\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{lnI}=\underset{\mathrm{p}\rightarrow\infty} {\mathrm{lim}}\frac{\frac{\mathrm{p}+\mathrm{2}}{\mathrm{p}^{\mathrm{2}} +\mathrm{2}}×\frac{\mathrm{2p}\left(\mathrm{p}+\mathrm{2}\right)−\left(\mathrm{p}^{\mathrm{2}} +\mathrm{2}\right)}{\left(\mathrm{p}+\mathrm{2}\right)^{\mathrm{2}} }}{\frac{\mathrm{2p}\left(\mathrm{p}+\mathrm{2}\right)−\left(\mathrm{p}^{\mathrm{2}} +\mathrm{2}\right)}{\left(\mathrm{p}+\mathrm{2}\right)^{\mathrm{2}} }} \\ $$$$=\underset{\mathrm{p}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{p}+\mathrm{2}}{\mathrm{p}^{\mathrm{2}} +\mathrm{2}}=\mathrm{0}\Rightarrow\mathrm{I}=\mathrm{e}^{\mathrm{0}} =\mathrm{1} \\ $$$$ \\ $$

Answered by mathmax by abdo last updated on 19/Sep/20

let f(x) =(((x^2 +2)/(x+2)))^((x+2)/(x^2 +2)) ⇒f(x) =e^(((x+2)/(x^2 +2))ln(((x^2 +2)/(x+2))) ) we have at +∞ ((x+2)/(x^2 +2))ln(((x^2 +2)/(x+2)))∼(1/x)ln(x)→0 ⇒lim_(x→+∞) f(x)=e^0 =1 =

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)\:=\left(\frac{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}}{\mathrm{x}+\mathrm{2}}\right)^{\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}}} \:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\:=\mathrm{e}^{\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}}\mathrm{ln}\left(\frac{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}}{\mathrm{x}+\mathrm{2}}\right)\:} \:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{at}\:+\infty\:\:\:\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}}\mathrm{ln}\left(\frac{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}}{\mathrm{x}+\mathrm{2}}\right)\sim\frac{\mathrm{1}}{\mathrm{x}}\mathrm{ln}\left(\mathrm{x}\right)\rightarrow\mathrm{0}\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow+\infty} \mathrm{f}\left(\mathrm{x}\right)=\mathrm{e}^{\mathrm{0}} \:=\mathrm{1} \\ $$$$= \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com