Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 114326 by 1549442205PVT last updated on 18/Sep/20

a)Find a four−digit number that satisfies the following condition: the sum of the squares of the extreme digits is equal to 13;the sum of the squares of the middle digits is equal to 85;if we substract 1089 from the desired number we obtain a number containing the same digits as the desired number but in reverse order. b)Prove that if the sum k+m+n of three natural numbers is divisible by 6 then k^3 +m^3 +n^3 is also divisible by6

$$\left.\mathrm{a}\right)\mathrm{Find}\:\mathrm{a}\:\mathrm{four}−\mathrm{digit}\:\mathrm{number}\:\mathrm{that} \\ $$$$\mathrm{satisfies}\:\mathrm{the}\:\mathrm{following}\:\mathrm{condition}: \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{squares}\:\mathrm{of}\:\mathrm{the}\:\mathrm{extreme}\:\mathrm{digits} \\ $$$$\mathrm{is}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{13};\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{squares}\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{middle}\:\mathrm{digits}\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{85};\mathrm{if}\:\mathrm{we} \\ $$$$\mathrm{substract}\:\mathrm{1089}\:\mathrm{from}\:\mathrm{the}\:\mathrm{desired} \\ $$$$\mathrm{number}\:\mathrm{we}\:\mathrm{obtain}\:\mathrm{a}\:\mathrm{number}\:\mathrm{containing} \\ $$$$\mathrm{the}\:\mathrm{same}\:\mathrm{digits}\:\mathrm{as}\:\mathrm{the}\:\mathrm{desired}\:\mathrm{number} \\ $$$$\mathrm{but}\:\mathrm{in}\:\mathrm{reverse}\:\mathrm{order}. \\ $$$$\left.\mathrm{b}\right)\mathrm{Prove}\:\mathrm{that}\:\mathrm{if}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{k}+\mathrm{m}+\mathrm{n}\:\mathrm{of}\: \\ $$$$\mathrm{three}\:\mathrm{natural}\:\mathrm{numbers}\:\mathrm{is}\:\mathrm{divisible}\:\mathrm{by}\: \\ $$$$\mathrm{6}\:\mathrm{then}\:\mathrm{k}^{\mathrm{3}} +\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} \:\mathrm{is}\:\mathrm{also}\:\:\mathrm{divisible}\:\mathrm{by6} \\ $$

Commented by Rasheed.Sindhi last updated on 18/Sep/20

Counter example 6 ∣ (1+2+3) but 6 ∤ (1^2 +2^2 +3^2 )

$${Counter}\:{example} \\ $$$$\mathrm{6}\:\mid\:\left(\mathrm{1}+\mathrm{2}+\mathrm{3}\right)\:{but}\:\mathrm{6}\:\nmid\:\left(\mathrm{1}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} \right) \\ $$

Commented by 1549442205PVT last updated on 18/Sep/20

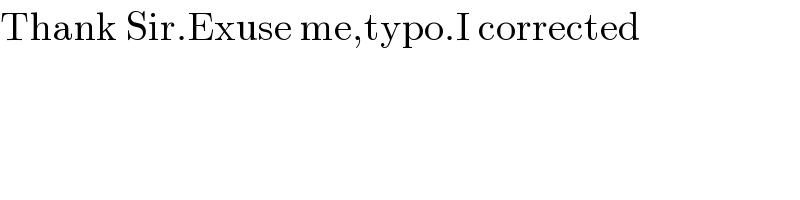
Thank Sir.Exuse me,typo.I corrected

$$\mathrm{Thank}\:\mathrm{Sir}.\mathrm{Exuse}\:\mathrm{me},\mathrm{typo}.\mathrm{I}\:\mathrm{corrected} \\ $$

Answered by mr W last updated on 18/Sep/20

[abcd] a^2 +d^2 =13 ⇒(a,d)=(2,3) or (3,2) b^2 +c^2 =85 ⇒(b,c)=(9,2) or (2,9) or (6,7) or (7,6) [abcd]−1089=[dcba] ⇒a>d ⇒a=3, d=2 [3bc2]−1089=[2cb3] 3000+100b+10c+2−1089=2000+100c+10b+3 ⇒b=c+1 ⇒b=7, c=6 ⇒the number is 3762.

$$\left[{abcd}\right] \\ $$$${a}^{\mathrm{2}} +{d}^{\mathrm{2}} =\mathrm{13}\:\Rightarrow\left({a},{d}\right)=\left(\mathrm{2},\mathrm{3}\right)\:{or}\:\left(\mathrm{3},\mathrm{2}\right) \\ $$$${b}^{\mathrm{2}} +{c}^{\mathrm{2}} =\mathrm{85}\:\Rightarrow\left({b},{c}\right)=\left(\mathrm{9},\mathrm{2}\right)\:{or}\:\left(\mathrm{2},\mathrm{9}\right)\:{or}\:\left(\mathrm{6},\mathrm{7}\right)\:{or}\:\left(\mathrm{7},\mathrm{6}\right) \\ $$$$\left[{abcd}\right]−\mathrm{1089}=\left[{dcba}\right] \\ $$$$\Rightarrow{a}>{d}\:\Rightarrow{a}=\mathrm{3},\:{d}=\mathrm{2} \\ $$$$\left[\mathrm{3}{bc}\mathrm{2}\right]−\mathrm{1089}=\left[\mathrm{2}{cb}\mathrm{3}\right] \\ $$$$\mathrm{3000}+\mathrm{100}{b}+\mathrm{10}{c}+\mathrm{2}−\mathrm{1089}=\mathrm{2000}+\mathrm{100}{c}+\mathrm{10}{b}+\mathrm{3} \\ $$$$\Rightarrow{b}={c}+\mathrm{1}\:\Rightarrow{b}=\mathrm{7},\:{c}=\mathrm{6} \\ $$$$\Rightarrow{the}\:{number}\:{is}\:\mathrm{3762}. \\ $$

Commented by Rasheed.Sindhi last updated on 18/Sep/20

You′re faster sir!

$${You}'{re}\:{faster}\:{sir}! \\ $$

Commented by mr W last updated on 18/Sep/20

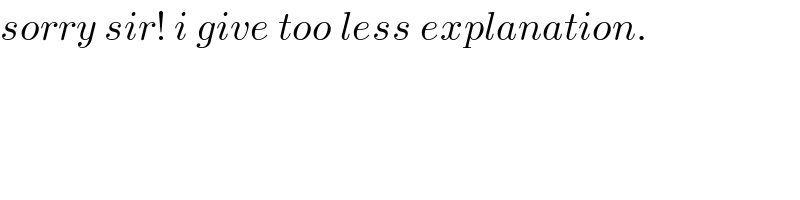
sorry sir! i give too less explanation.

$${sorry}\:{sir}!\:{i}\:{give}\:{too}\:{less}\:{explanation}. \\ $$

Commented by mr W last updated on 18/Sep/20

can you comfirm Q113710 sir?

$${can}\:{you}\:{comfirm}\:{Q}\mathrm{113710}\:{sir}? \\ $$

Commented by Rasheed.Sindhi last updated on 18/Sep/20

Sorry sir I can′t.

$${Sorry}\:{sir}\:{I}\:{can}'{t}. \\ $$

Commented by Rasheed.Sindhi last updated on 18/Sep/20

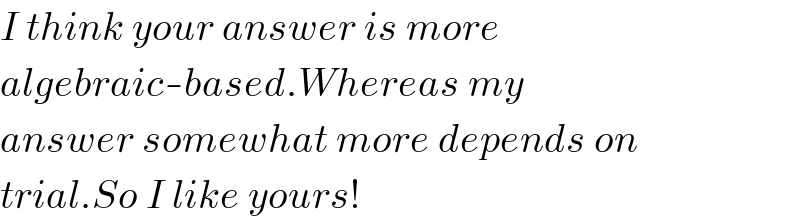
I think your answer is more algebraic-based.Whereas my answer somewhat more depends on trial.So I like yours!

$${I}\:{think}\:{your}\:{answer}\:{is}\:{more} \\ $$$${algebraic}-{based}.{Whereas}\:{my} \\ $$$${answer}\:{somewhat}\:{more}\:{depends}\:{on} \\ $$$${trial}.{So}\:{I}\:{like}\:{yours}! \\ $$

Commented by mr W last updated on 18/Sep/20

thanks sir!

$${thanks}\:{sir}! \\ $$

Answered by Rasheed.Sindhi last updated on 18/Sep/20

Reauired number: abcd a^2 +d^2 =13.......(i) b^2 +c^2 =85.........(ii) abcd−1089=dcba.....(iii) dcba<abcd⇒d<a a^2 +d^2 =13 ∧ d<a⇒a^2 =9∧d^2 =4 ⇒a=3 ∧ d=2 The number is now 3bc2 b^2 +c^2 =85 85 is the sum of 81 and 4 (square or 36 and 49 ((square numbers) So {b,c}={2,9} or {6,7} The number may be 3922 or 3292 or 3672 or 3762 Applying condition(iii) The number is 3762

$${Reauired}\:{number}:\:{abcd} \\ $$$${a}^{\mathrm{2}} +{d}^{\mathrm{2}} =\mathrm{13}.......\left({i}\right) \\ $$$${b}^{\mathrm{2}} +{c}^{\mathrm{2}} =\mathrm{85}.........\left({ii}\right) \\ $$$${abcd}−\mathrm{1089}={dcba}.....\left({iii}\right) \\ $$$${dcba}<{abcd}\Rightarrow{d}<{a} \\ $$$${a}^{\mathrm{2}} +{d}^{\mathrm{2}} =\mathrm{13}\:\wedge\:{d}<{a}\Rightarrow{a}^{\mathrm{2}} =\mathrm{9}\wedge{d}^{\mathrm{2}} =\mathrm{4} \\ $$$$\Rightarrow{a}=\mathrm{3}\:\wedge\:{d}=\mathrm{2} \\ $$$${The}\:{number}\:{is}\:{now}\:\mathrm{3}{bc}\mathrm{2} \\ $$$${b}^{\mathrm{2}} +{c}^{\mathrm{2}} =\mathrm{85} \\ $$$$\mathrm{85}\:{is}\:{the}\:{sum}\:{of}\:\mathrm{81}\:{and}\:\mathrm{4}\:\left({square}\right. \\ $$$${or}\:\mathrm{36}\:{and}\:\mathrm{49}\:\left(\left({square}\:{numbers}\right)\right. \\ $$$${So}\:\left\{{b},{c}\right\}=\left\{\mathrm{2},\mathrm{9}\right\}\:{or}\:\left\{\mathrm{6},\mathrm{7}\right\} \\ $$$${The}\:{number}\:{may}\:{be} \\ $$$$\mathrm{3922}\:{or}\:\mathrm{3292}\:{or}\:\mathrm{3672}\:{or}\:\mathrm{3762} \\ $$$${Applying}\:{condition}\left({iii}\right) \\ $$$${The}\:{number}\:{is}\:\mathrm{3762} \\ $$$$ \\ $$$$ \\ $$

Answered by MJS_new last updated on 18/Sep/20

6∣(k+m+n) k+m+n=6x k=6x−m−n k^3 +m^3 +n^3 = =18x(12x^2 −6(m+n)x+(m+n)^2 )−3mn(m+n) 6∣(18x(12x^2 −6(m+n)x+(m+n)^2 )) we need to show 6∣(3mn(m+n)) ⇔ 2∣mn(m+n) easy to see this is true if 2∣m ∨ 2∣n and if both m, n are uneven 2∣(m+n) ⇒ proved

$$\mathrm{6}\mid\left({k}+{m}+{n}\right) \\ $$$${k}+{m}+{n}=\mathrm{6}{x} \\ $$$${k}=\mathrm{6}{x}−{m}−{n} \\ $$$${k}^{\mathrm{3}} +{m}^{\mathrm{3}} +{n}^{\mathrm{3}} = \\ $$$$=\mathrm{18}{x}\left(\mathrm{12}{x}^{\mathrm{2}} −\mathrm{6}\left({m}+{n}\right){x}+\left({m}+{n}\right)^{\mathrm{2}} \right)−\mathrm{3}{mn}\left({m}+{n}\right) \\ $$$$\mathrm{6}\mid\left(\mathrm{18}{x}\left(\mathrm{12}{x}^{\mathrm{2}} −\mathrm{6}\left({m}+{n}\right){x}+\left({m}+{n}\right)^{\mathrm{2}} \right)\right) \\ $$$$\mathrm{we}\:\mathrm{need}\:\mathrm{to}\:\mathrm{show}\:\mathrm{6}\mid\left(\mathrm{3}{mn}\left({m}+{n}\right)\right) \\ $$$$\Leftrightarrow\:\mathrm{2}\mid{mn}\left({m}+{n}\right) \\ $$$$\mathrm{easy}\:\mathrm{to}\:\mathrm{see}\:\mathrm{this}\:\mathrm{is}\:\mathrm{true}\:\mathrm{if}\:\mathrm{2}\mid{m}\:\vee\:\mathrm{2}\mid{n} \\ $$$$\mathrm{and}\:\mathrm{if}\:\mathrm{both}\:{m},\:{n}\:\mathrm{are}\:\mathrm{uneven}\:\mathrm{2}\mid\left({m}+{n}\right) \\ $$$$\Rightarrow\:\mathrm{proved} \\ $$

Answered by 1549442205PVT last updated on 20/Sep/20

Thank all Sirs a)Denote the number we need find by mnpq^(−) .From the hypothesis we have m^2 +q^2 =13=3^2 +2^2 ⇒mq^(−) ∈{32,23} n^2 +q^2 =85=9^2 +2^2 =7^2 +6^2 ⇒np^(−) ∈{92,29,76,67} From the condition mnpq^(−) −1089=qpnm^(−) ⇔mnpq^(−) −qpnm^(−) =1089⇒m=3,q=2 we hav mnpq^(−) ∈{3922,3292,3762,3672} Hence,3np2^(−) −2pn3^(−) =1089.This gives us np^(−) =76⇒mnpq^(−) =3762 b)Applying the identity a^3 +b^3 +c^3 −3abc=(a+b+c)(a^2 +b^2 +c^2 −ab−bc−ca) we have: k^3 +m^3 +n^3 =3mnk+(k+m+n)(k^2 +m^2 +n^2 −km−kn−mn) From the hypothesis m+n+k⋮6 ⇒m+n+k=6p(p∈Z)⇒among three numbers m,n,k at least one number is even (since if all three numbers are odd then m+n+k is odd then m+n+k isn′t divisible by 2,so isn′t divisible by 6) ⇒3mnk⋮6,but m+n+k⋮6 ⇒m^3 +n^3 +k^3 ⋮6(Q.E.D)

$$\mathrm{Thank}\:\mathrm{all}\:\mathrm{Sirs} \\ $$$$\left.\mathrm{a}\right)\mathrm{Denote}\:\mathrm{the}\:\mathrm{number}\:\mathrm{we}\:\mathrm{need}\:\mathrm{find}\:\mathrm{by} \\ $$$$\overline {\mathrm{mnpq}}.\mathrm{From}\:\mathrm{the}\:\mathrm{hypothesis}\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{m}^{\mathrm{2}} +\mathrm{q}^{\mathrm{2}} =\mathrm{13}=\mathrm{3}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} \Rightarrow\overline {\mathrm{mq}}\in\left\{\mathrm{32},\mathrm{23}\right\} \\ $$$$\mathrm{n}^{\mathrm{2}} +\mathrm{q}^{\mathrm{2}} =\mathrm{85}=\mathrm{9}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} =\mathrm{7}^{\mathrm{2}} +\mathrm{6}^{\mathrm{2}} \Rightarrow\overline {\mathrm{np}}\in\left\{\mathrm{92},\mathrm{29},\mathrm{76},\mathrm{67}\right\} \\ $$$$\mathrm{From}\:\mathrm{the}\:\mathrm{condition}\:\overline {\mathrm{mnpq}}−\mathrm{1089}=\overline {\mathrm{qpnm}} \\ $$$$\Leftrightarrow\overline {\mathrm{mnpq}}−\overline {\mathrm{qpnm}}=\mathrm{1089}\Rightarrow\mathrm{m}=\mathrm{3},\mathrm{q}=\mathrm{2} \\ $$$$\mathrm{we}\:\mathrm{hav}\:\overline {\mathrm{mnpq}}\in\left\{\mathrm{3922},\mathrm{3292},\mathrm{3762},\mathrm{3672}\right\} \\ $$$$\mathrm{Hence},\overline {\mathrm{3np2}}−\overline {\mathrm{2pn3}}=\mathrm{1089}.\mathrm{This}\:\mathrm{gives} \\ $$$$\mathrm{us}\:\overline {\mathrm{np}}=\mathrm{76}\Rightarrow\overline {\mathrm{mnpq}}=\mathrm{3762} \\ $$$$\left.\mathrm{b}\right)\mathrm{Applying}\:\mathrm{the}\:\mathrm{identity} \\ $$$$\mathrm{a}^{\mathrm{3}} +\mathrm{b}^{\mathrm{3}} +\mathrm{c}^{\mathrm{3}} −\mathrm{3abc}=\left(\mathrm{a}+\mathrm{b}+\mathrm{c}\right)\left(\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} −\mathrm{ab}−\mathrm{bc}−\mathrm{ca}\right) \\ $$$$\mathrm{we}\:\mathrm{have}: \\ $$$$\mathrm{k}^{\mathrm{3}} +\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} =\mathrm{3mnk}+\left(\mathrm{k}+\mathrm{m}+\mathrm{n}\right)\left(\mathrm{k}^{\mathrm{2}} +\mathrm{m}^{\mathrm{2}} \right. \\ $$$$\left.+\mathrm{n}^{\mathrm{2}} −\mathrm{km}−\mathrm{kn}−\mathrm{mn}\right) \\ $$$$\mathrm{From}\:\mathrm{the}\:\mathrm{hypothesis}\:\mathrm{m}+\mathrm{n}+\mathrm{k}\vdots\mathrm{6} \\ $$$$\Rightarrow\mathrm{m}+\mathrm{n}+\mathrm{k}=\mathrm{6p}\left(\mathrm{p}\in\mathrm{Z}\right)\Rightarrow\mathrm{among}\:\mathrm{three} \\ $$$$\mathrm{numbers}\:\mathrm{m},\mathrm{n},\mathrm{k}\:\mathrm{at}\:\mathrm{least}\:\mathrm{one}\:\mathrm{number} \\ $$$$\mathrm{is}\:\mathrm{even}\:\left(\mathrm{since}\:\:\mathrm{if}\:\mathrm{all}\:\mathrm{three}\:\mathrm{numbers}\right. \\ $$$$\mathrm{are}\:\mathrm{odd}\:\mathrm{then}\:\mathrm{m}+\mathrm{n}+\mathrm{k}\:\mathrm{is}\:\mathrm{odd}\:\mathrm{then} \\ $$$$\mathrm{m}+\mathrm{n}+\mathrm{k}\:\mathrm{isn}'\mathrm{t}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{2},\mathrm{so}\:\mathrm{isn}'\mathrm{t} \\ $$$$\left.\mathrm{divisible}\:\mathrm{by}\:\mathrm{6}\right) \\ $$$$\Rightarrow\mathrm{3mnk}\vdots\mathrm{6},\mathrm{but}\:\mathrm{m}+\mathrm{n}+\mathrm{k}\vdots\mathrm{6} \\ $$$$\Rightarrow\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} +\mathrm{k}^{\mathrm{3}} \vdots\mathrm{6}\left(\boldsymbol{\mathrm{Q}}.\boldsymbol{\mathrm{E}}.\boldsymbol{\mathrm{D}}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com