Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 11433 by FilupS last updated on 26/Mar/17

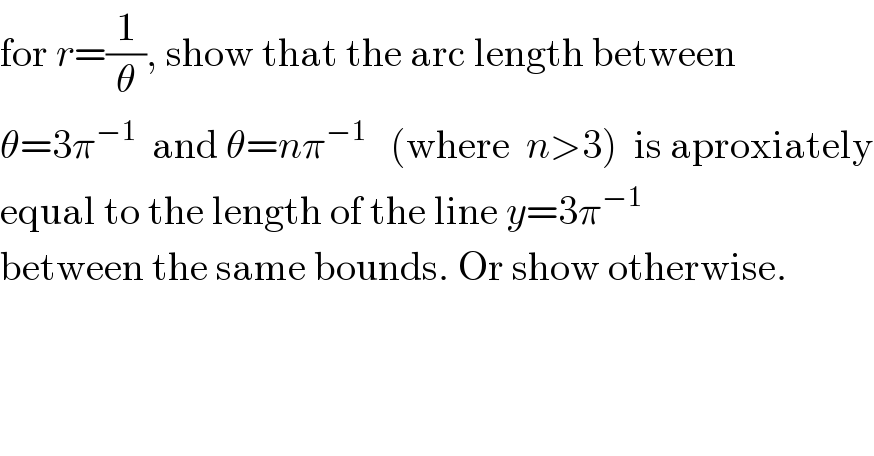
for r=(1/θ), show that the arc length between θ=3π^(−1) and θ=nπ^(−1) (where n>3) is aproxiately equal to the length of the line y=3π^(−1) between the same bounds. Or show otherwise.

$$\mathrm{for}\:{r}=\frac{\mathrm{1}}{\theta},\:\mathrm{show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{arc}\:\mathrm{length}\:\mathrm{between} \\ $$ $$\theta=\mathrm{3}\pi^{−\mathrm{1}} \:\:\mathrm{and}\:\theta={n}\pi^{−\mathrm{1}} \:\:\:\left(\mathrm{where}\:\:{n}>\mathrm{3}\right)\:\:\mathrm{is}\:\mathrm{aproxiately} \\ $$ $$\mathrm{equal}\:\mathrm{to}\:\mathrm{the}\:\mathrm{length}\:\mathrm{of}\:\mathrm{the}\:\mathrm{line}\:{y}=\mathrm{3}\pi^{−\mathrm{1}} \\ $$ $$\mathrm{between}\:\mathrm{the}\:\mathrm{same}\:\mathrm{bounds}.\:\mathrm{Or}\:\mathrm{show}\:\mathrm{otherwise}. \\ $$ $$ \\ $$

Commented byFilupS last updated on 26/Mar/17

Commented by@ANTARES_VY last updated on 26/Mar/17

what is the name of the program..

$$\boldsymbol{\mathrm{what}}\:\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{the}}\:\:\boldsymbol{\mathrm{name}}\:\:\boldsymbol{\mathrm{of}}\:\:\boldsymbol{\mathrm{the}}\:\:\boldsymbol{\mathrm{program}}.. \\ $$

Commented byFilupS last updated on 26/Mar/17

desmos

$$\mathrm{desmos} \\ $$

Commented bymrW1 last updated on 26/Mar/17

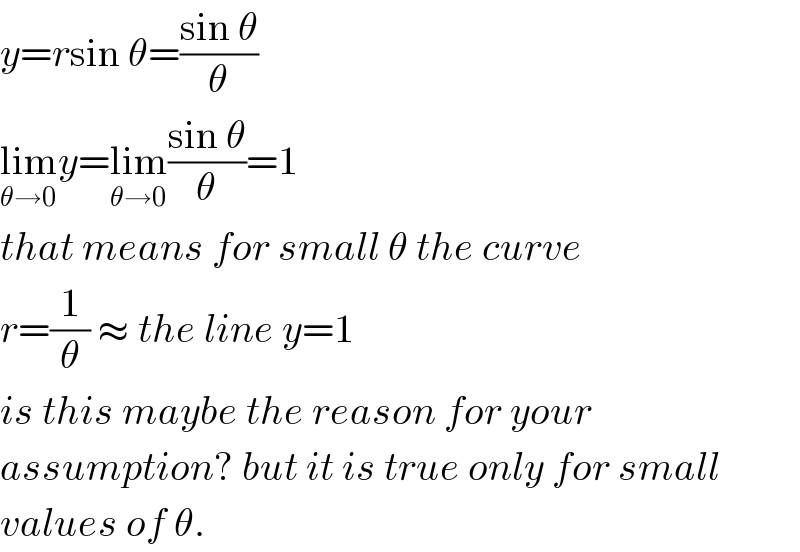
y=rsin θ=((sin θ)/θ) lim_(θ→0) y=lim_(θ→0) ((sin θ)/θ)=1 that means for small θ the curve r=(1/θ) ≈ the line y=1 is this maybe the reason for your assumption? but it is true only for small values of θ.

$${y}={r}\mathrm{sin}\:\theta=\frac{\mathrm{sin}\:\theta}{\theta} \\ $$ $$\underset{\theta\rightarrow\mathrm{0}} {\mathrm{lim}}{y}=\underset{\theta\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}\:\theta}{\theta}=\mathrm{1} \\ $$ $${that}\:{means}\:{for}\:{small}\:\theta\:{the}\:{curve} \\ $$ $${r}=\frac{\mathrm{1}}{\theta}\:\approx\:{the}\:{line}\:{y}=\mathrm{1} \\ $$ $${is}\:{this}\:{maybe}\:{the}\:{reason}\:{for}\:{your} \\ $$ $${assumption}?\:{but}\:{it}\:{is}\:{true}\:{only}\:{for}\:{small} \\ $$ $${values}\:{of}\:\theta. \\ $$

Commented byFilupS last updated on 26/Mar/17

This makes sense! Thanks

$$\mathrm{This}\:\mathrm{makes}\:\mathrm{sense}!\:\mathrm{Thanks} \\ $$

Answered by mrW1 last updated on 26/Mar/17

curve r=(1/θ): (dr/dθ)=−(1/θ^2 ) (√(r^2 +((dr/dθ))^2 ))=(√((1/θ^2 )+(1/θ^4 )))=((√(1+θ^2 ))/θ^2 ) L=∫_θ_1 ^θ_2 (√(r^2 +((dr/dθ))^2 ))dθ=∫_θ_1 ^θ_2 ((√(1+θ^2 ))/θ^2 )dθ [−((√(1+θ^2 ))/θ)+ln (θ+(√(1+θ^2 )))]_θ_1 ^θ_2 =[((√(1+θ_1 ^2 ))/θ_1 )−((√(1+θ_2 ^2 ))/θ_2 )+ln (((ϑ_2 +(√(1+θ_2 ^2 )))/(θ_1 +(√(1+θ_1 ^2 )))))] with θ_1 =3π^(−1) and θ_2 =nπ^(−1) line y=3π^(−1) : ⇒x=y×cot θ=3π^(−1) cot θ x_1 =3π^(−1) cot θ_1 x_2 =3π^(−1) cot θ_2 L_1 =∣∫_x_1 ^x_2 (√(1+(y′)^2 ))dx∣=∣∫_x_1 ^x_2 dx∣=x_1 −x_2 =3π^(−1) (cot θ_1 −cot θ_2 ) =3π^(−1) [cot (3π^(−1) )−cot (nπ^(−1) )] L≠L_1

$${curve}\:{r}=\frac{\mathrm{1}}{\theta}: \\ $$ $$\frac{{dr}}{{d}\theta}=−\frac{\mathrm{1}}{\theta^{\mathrm{2}} } \\ $$ $$\sqrt{{r}^{\mathrm{2}} +\left(\frac{{dr}}{{d}\theta}\right)^{\mathrm{2}} }=\sqrt{\frac{\mathrm{1}}{\theta^{\mathrm{2}} }+\frac{\mathrm{1}}{\theta^{\mathrm{4}} }}=\frac{\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }}{\theta^{\mathrm{2}} } \\ $$ $${L}=\int_{\theta_{\mathrm{1}} } ^{\theta_{\mathrm{2}} } \sqrt{{r}^{\mathrm{2}} +\left(\frac{{dr}}{{d}\theta}\right)^{\mathrm{2}} }{d}\theta=\int_{\theta_{\mathrm{1}} } ^{\theta_{\mathrm{2}} } \frac{\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }}{\theta^{\mathrm{2}} }{d}\theta \\ $$ $$\left[−\frac{\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }}{\theta}+\mathrm{ln}\:\left(\theta+\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }\right)\right]_{\theta_{\mathrm{1}} } ^{\theta_{\mathrm{2}} } \\ $$ $$=\left[\frac{\sqrt{\mathrm{1}+\theta_{\mathrm{1}} ^{\mathrm{2}} }}{\theta_{\mathrm{1}} }−\frac{\sqrt{\mathrm{1}+\theta_{\mathrm{2}} ^{\mathrm{2}} }}{\theta_{\mathrm{2}} }+\mathrm{ln}\:\left(\frac{\vartheta_{\mathrm{2}} +\sqrt{\mathrm{1}+\theta_{\mathrm{2}} ^{\mathrm{2}} }}{\theta_{\mathrm{1}} +\sqrt{\mathrm{1}+\theta_{\mathrm{1}} ^{\mathrm{2}} }}\right)\right] \\ $$ $${with}\:\theta_{\mathrm{1}} =\mathrm{3}\pi^{−\mathrm{1}} \:{and}\:\theta_{\mathrm{2}} ={n}\pi^{−\mathrm{1}} \\ $$ $$ \\ $$ $$ \\ $$ $${line}\:{y}=\mathrm{3}\pi^{−\mathrm{1}} : \\ $$ $$\Rightarrow{x}={y}×\mathrm{cot}\:\theta=\mathrm{3}\pi^{−\mathrm{1}} \mathrm{cot}\:\theta \\ $$ $${x}_{\mathrm{1}} =\mathrm{3}\pi^{−\mathrm{1}} \mathrm{cot}\:\theta_{\mathrm{1}} \\ $$ $${x}_{\mathrm{2}} =\mathrm{3}\pi^{−\mathrm{1}} \mathrm{cot}\:\theta_{\mathrm{2}} \\ $$ $${L}_{\mathrm{1}} =\mid\int_{{x}_{\mathrm{1}} } ^{{x}_{\mathrm{2}} } \sqrt{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }{dx}\mid=\mid\int_{{x}_{\mathrm{1}} } ^{{x}_{\mathrm{2}} } {dx}\mid={x}_{\mathrm{1}} −{x}_{\mathrm{2}} \\ $$ $$=\mathrm{3}\pi^{−\mathrm{1}} \left(\mathrm{cot}\:\theta_{\mathrm{1}} −\mathrm{cot}\:\theta_{\mathrm{2}} \right) \\ $$ $$=\mathrm{3}\pi^{−\mathrm{1}} \left[\mathrm{cot}\:\left(\mathrm{3}\pi^{−\mathrm{1}} \right)−\mathrm{cot}\:\left({n}\pi^{−\mathrm{1}} \right)\right] \\ $$ $$ \\ $$ $${L}\neq{L}_{\mathrm{1}} \\ $$

Commented bymrW1 last updated on 26/Mar/17

Commented byajfour last updated on 26/Mar/17

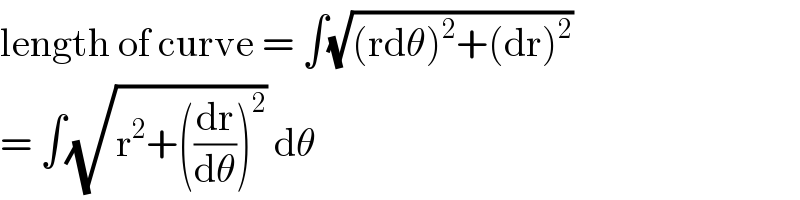
length of curve = ∫(√((rdθ)^2 +(dr)^2 )) = ∫(√(r^2 +((dr/dθ))^2 )) dθ

$$\mathrm{length}\:\mathrm{of}\:\mathrm{curve}\:=\:\int\sqrt{\left(\mathrm{rd}\theta\right)^{\mathrm{2}} +\left(\mathrm{dr}\right)^{\mathrm{2}} } \\ $$ $$=\:\int\sqrt{\mathrm{r}^{\mathrm{2}} +\left(\frac{\mathrm{dr}}{\mathrm{d}\theta}\right)^{\mathrm{2}} }\:\mathrm{d}\theta \\ $$

Commented bymrW1 last updated on 26/Mar/17

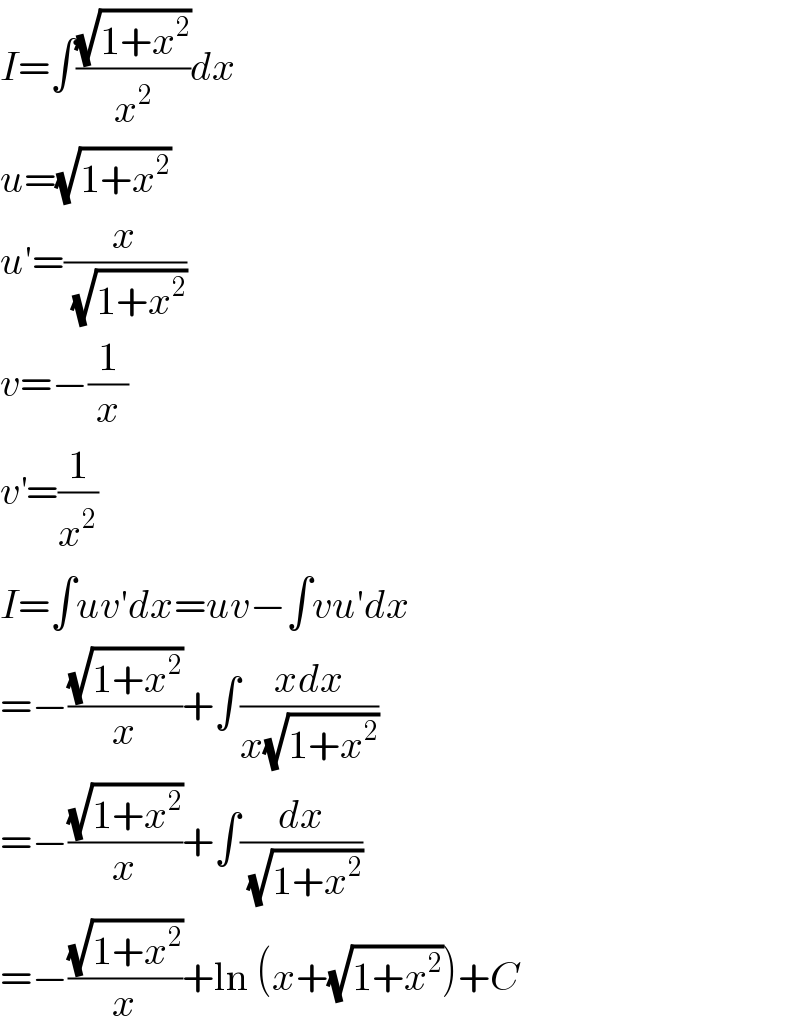
I=∫((√(1+x^2 ))/x^2 )dx u=(√(1+x^2 )) u′=(x/(√(1+x^2 ))) v=−(1/x) v^′ =(1/x^2 ) I=∫uv′dx=uv−∫vu′dx =−((√(1+x^2 ))/x)+∫((xdx)/(x(√(1+x^2 )))) =−((√(1+x^2 ))/x)+∫(dx/(√(1+x^2 ))) =−((√(1+x^2 ))/x)+ln (x+(√(1+x^2 )))+C

$${I}=\int\frac{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}{{x}^{\mathrm{2}} }{dx} \\ $$ $${u}=\sqrt{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$ $${u}'=\frac{{x}}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$ $${v}=−\frac{\mathrm{1}}{{x}} \\ $$ $${v}^{'} =\frac{\mathrm{1}}{{x}^{\mathrm{2}} } \\ $$ $${I}=\int{uv}'{dx}={uv}−\int{vu}'{dx} \\ $$ $$=−\frac{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}{{x}}+\int\frac{{xdx}}{{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$ $$=−\frac{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}{{x}}+\int\frac{{dx}}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$ $$=−\frac{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}{{x}}+\mathrm{ln}\:\left({x}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right)+{C} \\ $$

Commented bymrW1 last updated on 26/Mar/17

you are right!

$${you}\:{are}\:{right}! \\ $$

Commented byajfour last updated on 26/Mar/17

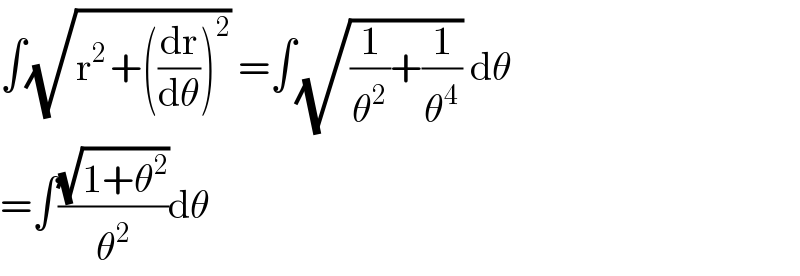
∫(√(r^(2 ) +((dr/dθ))^2 )) =∫(√((1/θ^2 )+(1/θ^4 ))) dθ =∫((√(1+θ^2 ))/θ^2 )dθ

$$\int\sqrt{\mathrm{r}^{\mathrm{2}\:} +\left(\frac{\mathrm{dr}}{\mathrm{d}\theta}\right)^{\mathrm{2}} }\:=\int\sqrt{\frac{\mathrm{1}}{\theta^{\mathrm{2}} }+\frac{\mathrm{1}}{\theta^{\mathrm{4}} }}\:\mathrm{d}\theta \\ $$ $$=\int\frac{\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }}{\theta^{\mathrm{2}} }\mathrm{d}\theta \\ $$

Commented byajfour last updated on 26/Mar/17

and if φ^2 =1+θ^2 φdφ =θdθ ∫((√(1+θ^2 ))/θ^2 ) dθ = ∫(φ^2 /((φ^2 −1)^(3/2) )) d∅ otherwise if θ=cot φ ∫((√(1+θ^2 ))/θ^2 ) dθ = −∫ ((cosec ^3 φ)/(cot^2 φ)) dφ =∫ ((sec^2 φ)/(sin φ)) dφ unable to integrate it..

$$\mathrm{and}\:\mathrm{if}\:\phi^{\mathrm{2}} =\mathrm{1}+\theta^{\mathrm{2}} \\ $$ $$\phi\mathrm{d}\phi\:=\theta\mathrm{d}\theta \\ $$ $$\int\frac{\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }}{\theta^{\mathrm{2}} }\:\mathrm{d}\theta\:=\:\int\frac{\phi^{\mathrm{2}} }{\left(\phi^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{3}/\mathrm{2}} }\:\mathrm{d}\emptyset \\ $$ $$\mathrm{otherwise}\:\mathrm{if}\:\theta=\mathrm{cot}\:\phi \\ $$ $$\int\frac{\sqrt{\mathrm{1}+\theta^{\mathrm{2}} }}{\theta^{\mathrm{2}} }\:\mathrm{d}\theta\:=\:−\int\:\frac{\mathrm{cosec}\:\:^{\mathrm{3}} \phi}{\mathrm{cot}\:^{\mathrm{2}} \phi}\:\mathrm{d}\phi \\ $$ $$=\int\:\frac{\mathrm{sec}\:^{\mathrm{2}} \phi}{\mathrm{sin}\:\phi}\:\mathrm{d}\phi\:\:\:\:\mathrm{unable}\:\mathrm{to}\:\mathrm{integrate}\:\mathrm{it}.. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com