Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 114511 by 1549442205PVT last updated on 19/Sep/20

$$\mathrm{Find}\mathrm{numbers}\mathrm{which}\mathrm{are}\mathrm{common}$$$$\mathrm{terms}\mathrm{of}\mathrm{the}\mathrm{two}\mathrm{following}\mathrm{arithmetic}$$$$\mathrm{progression}:$$$$3,7,11,...,407\mathrm{and}2,9,16,...,709$$

Commented by bobhans last updated on 19/Sep/20

$$let{A}_n=\{3,7,11,...,23,...,51,...,407\}$$$$B_n=\{2,9,16,23,...,51,...,709\}$$$$wewantfind{C}_n=A_n\cap B_n$$$$consider{C}_n=\{23,51,...\}$$$$C_n=23+(n-1).28$$$$C_n=28n-5<407$$$$28n<412;n=14$$$$therefore{C}_n=\{23,51,79,...,387\}$$

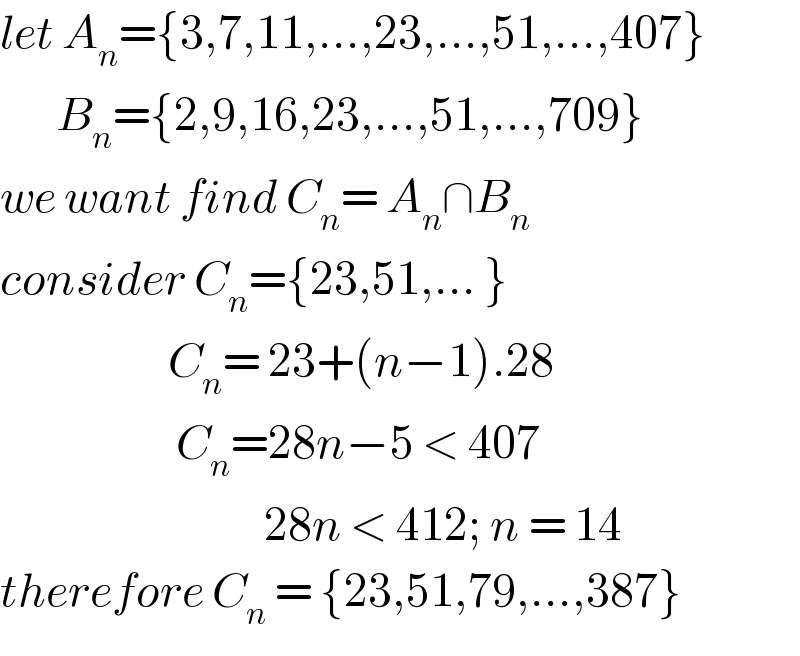
Commented by bemath last updated on 19/Sep/20

$$superb...gavekudos$$

Commented by 1549442205PVT last updated on 19/Sep/20

$$\mathrm{Thank}\mathrm{Sir}.$$

Answered by PRITHWISH SEN 2 last updated on 19/Sep/20

$$3+({\mathrm{n}}_1-1)4=2+({\mathrm{n}}_2-1)7$$$${4\mathrm{n}}_1-1={7\mathrm{n}}_2-5$$$${7\mathrm{n}}_2-{4\mathrm{n}}_1=4$$$${7\mathrm{n}}_2=4({\mathrm{n}}_1+1)$$$$\because 4\mid {\mathrm{n}}_2\mathbf{and}7\mid ({\mathbf{n}}_1+1)$$$$\Rightarrow {\mathrm{n}}_1=6\mathrm{and}{\mathrm{n}}_2=4$$$$\therefore \mathrm{the}{1}^{\mathrm{st}}\mathrm{term}\mathrm{is}=4.6-1=7.4-5=23$$$$\mathrm{Now}\mathrm{we}\mathrm{have}\mathrm{to}\mathrm{find}\mathrm{an}\mathrm{A}.\mathrm{P}\mathrm{whose}{1}^{\mathrm{st}}\mathrm{term}\mathrm{is}23$$$$\mathrm{and}\mathrm{c}.\mathrm{d}\mathrm{is}=4.7=28\mathrm{and}\mathrm{last}\mathrm{term}\mathrm{is}⩽407$$$$\therefore {\mathbf{t}}_{\mathbf{n}}=23+(\mathbf{n}-1)28⩽407$$$$\Rightarrow (\mathbf{n}-1)28⩽384\Rightarrow \mathrm{n}⩽14$$$$\therefore \mathrm{the}\mathrm{no}.\mathrm{of}\mathrm{terms}=14$$$$$$$$$$

Commented by Rasheed.Sindhi last updated on 19/Sep/20

$$\mathcal{N}iceci\mathcal{N}$$$$\mathcal{S}iri\mathcal{S}$$$$!$$

Commented by PRITHWISH SEN 2 last updated on 19/Sep/20

$$\mathrm{thank}\mathrm{you}\mathrm{sir}.$$

Commented by 1549442205PVT last updated on 19/Sep/20

$$\mathrm{Thank}\mathrm{sir},\mathrm{solution}\mathrm{is}\mathrm{pretty}\mathrm{clear}$$

Answered by 1549442205PVT last updated on 19/Sep/20

$$\mathrm{It}\mathrm{is}\mathrm{clear}\mathrm{that}\mathrm{the}\mathrm{general}\mathrm{term}\mathrm{of}\mathrm{the}$$$$\mathrm{first}\mathrm{A}.\mathrm{P}\mathrm{is}\mathrm{of}\mathrm{the}\mathrm{form}{\mathrm{a}}_{\mathrm{n}}=3+4(\mathrm{n}-1);$$$$\mathrm{the}\mathrm{indicated}\mathrm{terms}\mathrm{of}\mathrm{the}\mathrm{progression}$$$$\mathrm{are}\mathrm{associated}\mathrm{with}\mathrm{the}\mathrm{values}\mathrm{n}=1,2,...$$$$,102.\mathrm{Similarly},\mathrm{the}\mathrm{terms}\mathrm{of}\mathrm{the}\mathrm{second}$$$$\mathrm{A}.\mathrm{P}\mathrm{are}\mathrm{obtained}\mathrm{from}\mathrm{the}\mathrm{formula}$$$${\mathrm{b}}_{\mathrm{k}}=2+7(\mathrm{k}-1),\mathrm{k}=1,2,...,102.\mathrm{The}\mathrm{problem}$$$$\mathrm{thus}\mathrm{consists}\mathrm{in}\mathrm{finding}\mathrm{all}\mathrm{numbers}\mathrm{n}$$$$\mathrm{and}\mathrm{k},1⩽\mathrm{n}⩽102,1⩽\mathrm{k}⩽102,\mathrm{for}\mathrm{which}$$$${\mathrm{a}}_{\mathrm{n}}={\mathrm{b}}_{\mathrm{k}},\mathrm{that}\mathrm{is},4\mathrm{n}+4=7\mathrm{k}$$$$\mathrm{From}\mathrm{the}\mathrm{equation}4(\mathrm{n}+1)=7\mathrm{k}\mathrm{it}\mathrm{is}$$$$\mathrm{evident}\mathrm{that}\mathrm{k}\mathrm{is}\mathrm{a}\mathrm{multiple}\mathrm{of}4,\mathrm{that}$$$$\mathrm{is},\mathrm{k}=4\mathrm{s}\mathrm{with}\mathrm{s}=\overline{1...25}(\mathrm{since}1⩽\mathrm{k}⩽102)$$$$.\mathrm{But}\mathrm{if}\mathrm{k}=4\mathrm{s}\mathrm{then}4(\mathrm{n}+1)=7.4\mathrm{s},\mathrm{or}$$$$\mathrm{n}=7\mathrm{s}-1.\mathrm{Since}1⩽\mathrm{n}⩽102,\mathrm{only}\mathrm{the}$$$$\mathrm{numbers}1,2,...,14\mathrm{are}\mathrm{permissible}$$$$\mathrm{values}\mathrm{of}\mathrm{s}.\mathrm{Thus},\mathrm{we}\mathrm{have}14\mathrm{numbers}$$$$\mathrm{that}\mathrm{are}\mathrm{common}\mathrm{to}\mathrm{both}\mathrm{A}.\mathrm{Ps}$$$$\mathrm{The}\mathrm{numbers}\mathrm{themselves}\mathrm{are}\mathrm{readily}$$$$\mathrm{found}\mathrm{either}\mathrm{from}\mathrm{the}\mathrm{formula}\mathrm{for}{\mathrm{a}}_{\mathrm{n}}$$$$\mathrm{when}\mathrm{n}=7\mathrm{s}-1,\mathrm{s}=\overline{1...14},\mathrm{or}\mathrm{from}\mathrm{the}$$$$\mathrm{formula}\mathrm{for}{\mathrm{b}}_{\mathrm{k}},\mathrm{for}\mathrm{k}=4\mathrm{s},\mathrm{s}=\overline{1...14}$$

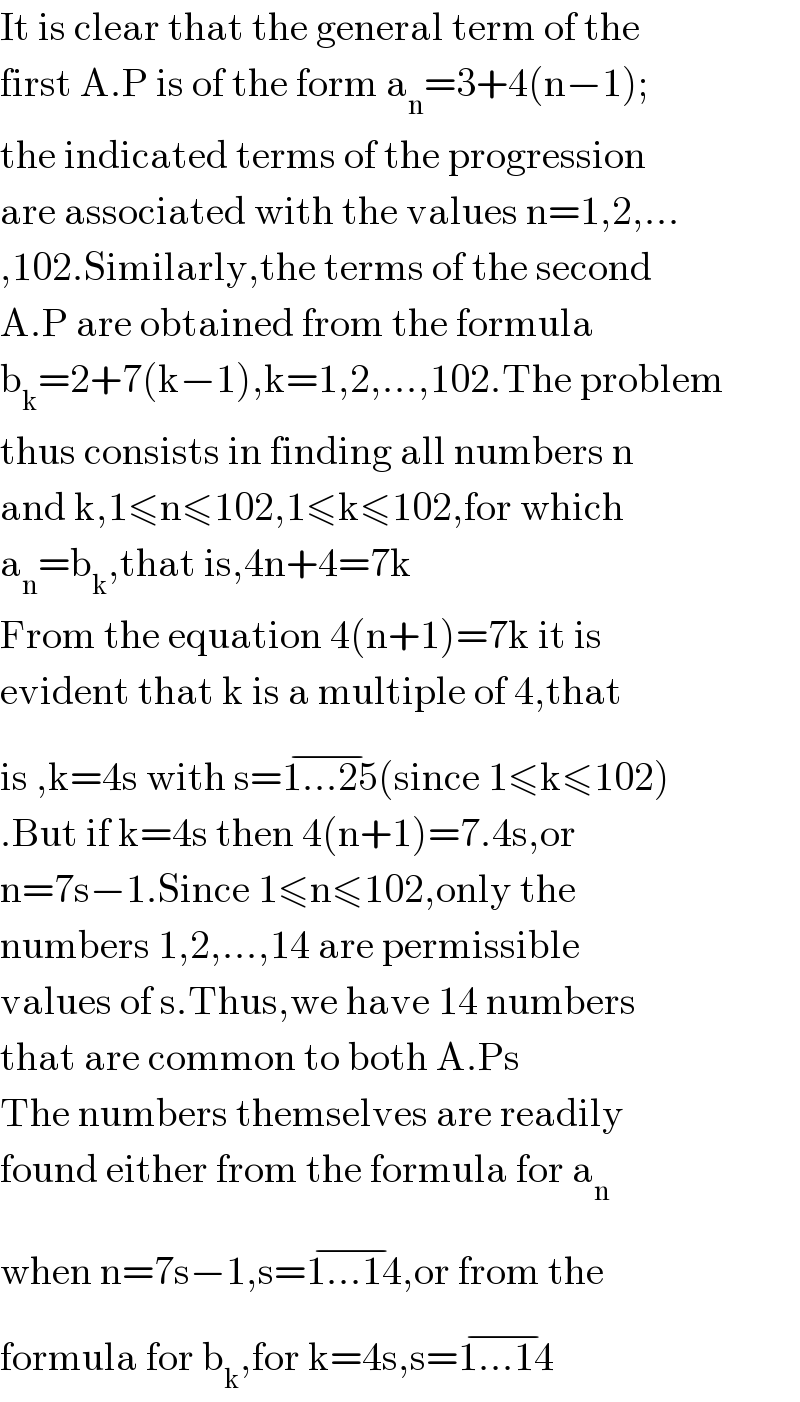
Commented by PRITHWISH SEN 2 last updated on 19/Sep/20

$$\mathrm{great}\mathrm{sir}$$

Commented by 1549442205PVT last updated on 21/Sep/20

$$\mathrm{Thank}\mathrm{Sir}.\mathrm{You}\mathrm{are}\mathrm{welcome}.$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com