Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 114754 by bemath last updated on 21/Sep/20

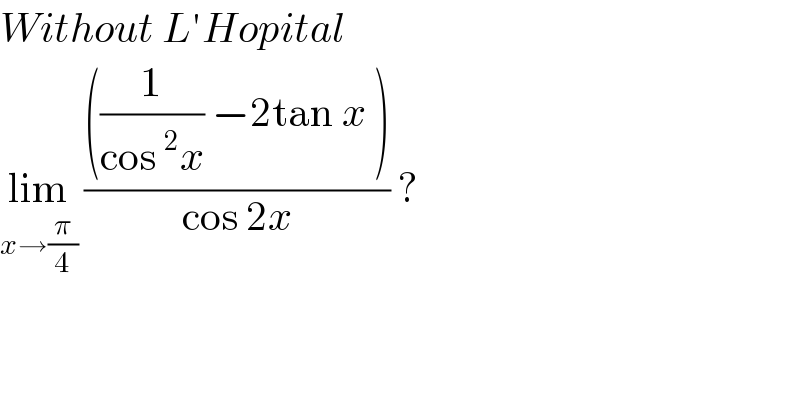
Without L′Hopital lim_(x→(π/4)) ((((1/(cos^2 x)) −2tan x ))/(cos 2x)) ?

$${Without}\:{L}'{Hopital}\: \\ $$$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\:\frac{\left(\frac{\mathrm{1}}{\mathrm{cos}\:^{\mathrm{2}} {x}}\:−\mathrm{2tan}\:{x}\:\right)}{\mathrm{cos}\:\mathrm{2}{x}}\:? \\ $$

Commented by bemath last updated on 21/Sep/20

gave kudos all sir

$${gave}\:{kudos}\:{all}\:{sir} \\ $$

Answered by john santu last updated on 21/Sep/20

let x = (π/4)+h , h→0 lim_(h→0) ((sec^2 ((π/4)+h)−2tan((π/4)+h) )/(cos ((π/2)+2h))) = lim_(h→0) ((tan^2 ((π/4)+h)−2tan ((π/4)+h)+1)/(−sin 2h)) = lim_(h→0) (((1−tan ((π/4)+h))^2 )/(−sin 2h)) = lim_(h→0) (([1−(((1+tan h)/(1−tan h)))]^2 )/(−sin 2h)) = lim_(h→0) (((−2tan h)^2 )/(−sin 2h)) =lim_(h→0) ((4 tan^2 h)/(−sin 2h)) = 0

$${let}\:{x}\:=\:\frac{\pi}{\mathrm{4}}+{h}\:,\:{h}\rightarrow\mathrm{0} \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sec}\:^{\mathrm{2}} \left(\frac{\pi}{\mathrm{4}}+{h}\right)−\mathrm{2tan}\left(\frac{\pi}{\mathrm{4}}+{h}\right)\:}{\mathrm{cos}\:\left(\frac{\pi}{\mathrm{2}}+\mathrm{2}{h}\right)}\:= \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\pi}{\mathrm{4}}+{h}\right)−\mathrm{2tan}\:\left(\frac{\pi}{\mathrm{4}}+{h}\right)+\mathrm{1}}{−\mathrm{sin}\:\mathrm{2}{h}}\:= \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\left(\mathrm{1}−\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}+{h}\right)\right)^{\mathrm{2}} }{−\mathrm{sin}\:\mathrm{2}{h}}\:= \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\left[\mathrm{1}−\left(\frac{\mathrm{1}+\mathrm{tan}\:{h}}{\mathrm{1}−\mathrm{tan}\:{h}}\right)\right]^{\mathrm{2}} }{−\mathrm{sin}\:\mathrm{2}{h}}\:= \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\left(−\mathrm{2tan}\:{h}\right)^{\mathrm{2}} }{−\mathrm{sin}\:\mathrm{2}{h}}\:=\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{4}\:\mathrm{tan}\:^{\mathrm{2}} {h}}{−\mathrm{sin}\:\mathrm{2}{h}}\:=\:\mathrm{0} \\ $$

Answered by Olaf last updated on 21/Sep/20

lim_(x→(π/4)) ((1+tan^2 x−2tanx)/(cos2x)) lim_(x→(π/4)) (((1−tan^2 x)^2 )/(cos2x)) u = (π/4)−x lim_(u→0) (([1−tan((π/4)−u)^2 ]^2 )/(cos((π/2)−2u))) lim_(u→0) (([1−(((tan(π/4)−tanu)/(1+tan(π/4)tanu)))^2 ]^2 )/(cos((π/2)−2u))) lim_(u→0) (([1−(((1−tanu)/(1+tanu)))^2 ]^2 )/(sin2u)) lim_(u→0) (([1−(((1−tanu)/(1+tanu)))^2 ]^2 )/(sin2u)) lim_(u→0) (([((4tan^2 u)/((1+tanu)^2 ))]^2 )/(sin2u)) lim_(u→0) (([((4u^2 )/((1+u)^2 ))]^2 )/(2u)) = 0

$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\frac{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} {x}−\mathrm{2tan}{x}}{\mathrm{cos2}{x}} \\ $$$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\frac{\left(\mathrm{1}−\mathrm{tan}^{\mathrm{2}} {x}\right)^{\mathrm{2}} }{\mathrm{cos2}{x}} \\ $$$${u}\:=\:\frac{\pi}{\mathrm{4}}−{x} \\ $$$$\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\mathrm{1}−\mathrm{tan}\left(\frac{\pi}{\mathrm{4}}−{u}\right)^{\mathrm{2}} \right]^{\mathrm{2}} }{\mathrm{cos}\left(\frac{\pi}{\mathrm{2}}−\mathrm{2}{u}\right)} \\ $$$$\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\mathrm{1}−\left(\frac{\mathrm{tan}\frac{\pi}{\mathrm{4}}−\mathrm{tan}{u}}{\mathrm{1}+\mathrm{tan}\frac{\pi}{\mathrm{4}}\mathrm{tan}{u}}\right)^{\mathrm{2}} \right]^{\mathrm{2}} }{\mathrm{cos}\left(\frac{\pi}{\mathrm{2}}−\mathrm{2}{u}\right)} \\ $$$$\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\mathrm{1}−\left(\frac{\mathrm{1}−\mathrm{tan}{u}}{\mathrm{1}+\mathrm{tan}{u}}\right)^{\mathrm{2}} \right]^{\mathrm{2}} }{\mathrm{sin2}{u}} \\ $$$$\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\mathrm{1}−\left(\frac{\mathrm{1}−\mathrm{tan}{u}}{\mathrm{1}+\mathrm{tan}{u}}\right)^{\mathrm{2}} \right]^{\mathrm{2}} }{\mathrm{sin2}{u}} \\ $$$$\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\frac{\mathrm{4tan}^{\mathrm{2}} {u}}{\left(\mathrm{1}+\mathrm{tan}{u}\right)^{\mathrm{2}} }\right]^{\mathrm{2}} }{\mathrm{sin2}{u}} \\ $$$$\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\frac{\mathrm{4}{u}^{\mathrm{2}} }{\left(\mathrm{1}+{u}\right)^{\mathrm{2}} }\right]^{\mathrm{2}} }{\mathrm{2}{u}}\:=\:\mathrm{0} \\ $$

Answered by bobhans last updated on 21/Sep/20

in other way lim_(x→(π/4)) (((tan x−1)^2 )/(cos^2 x−sin^2 x)) = lim_(x→(π/4)) (((cos x−sin x)^2 )/(cos^2 x(cos x−sin x)(cos x+sin x))) = lim_(x→(π/4)) ((cos x−sin x)/(cos^2 x(cos x+sin x))) = 0

$${in}\:{other}\:{way}\: \\ $$$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\:\frac{\left(\mathrm{tan}\:{x}−\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{cos}\:^{\mathrm{2}} {x}−\mathrm{sin}\:^{\mathrm{2}} {x}}\:=\:\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\:\frac{\left(\mathrm{cos}\:{x}−\mathrm{sin}\:{x}\right)^{\mathrm{2}} }{\mathrm{cos}\:^{\mathrm{2}} {x}\left(\mathrm{cos}\:{x}−\mathrm{sin}\:{x}\right)\left(\mathrm{cos}\:{x}+\mathrm{sin}\:{x}\right)} \\ $$$$=\:\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}{\mathrm{cos}\:^{\mathrm{2}} {x}\left(\mathrm{cos}\:{x}+\mathrm{sin}\:{x}\right)}\:=\:\mathrm{0}\: \\ $$

Answered by MJS_new last updated on 21/Sep/20

(1/(cos^2 x))=1+tan^2 x cos 2x =−1+2cos^2 x =((1−t^2 )/(1+t^2 )) ⇒ lim_(x→(π/4)) (((1−tan x)(1+tan^2 x))/(1+tan x)) =0

$$\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \:{x}}=\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:{x} \\ $$$$\mathrm{cos}\:\mathrm{2}{x}\:=−\mathrm{1}+\mathrm{2cos}^{\mathrm{2}} \:{x}\:=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$\Rightarrow \\ $$$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{4}}} {\mathrm{lim}}\:\frac{\left(\mathrm{1}−\mathrm{tan}\:{x}\right)\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:{x}\right)}{\mathrm{1}+\mathrm{tan}\:{x}}\:=\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com