
Question and Answers Forum
Question Number 115033 by bobhans last updated on 23/Sep/20
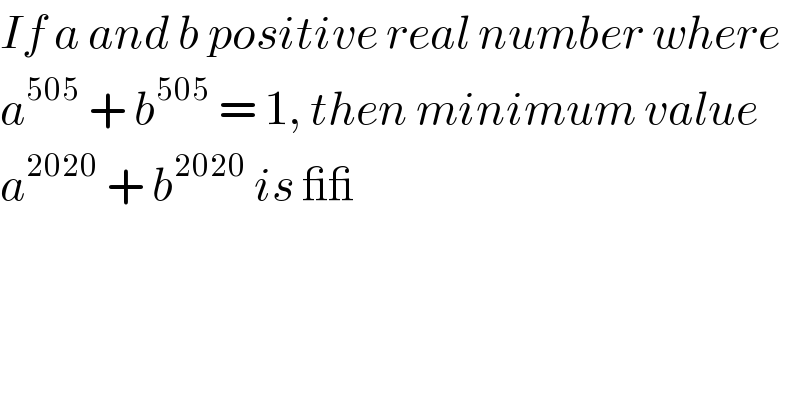
Answered by 1549442205PVT last updated on 23/Sep/20
![Put a^(505) =x,b^(505) =y⇒x+y=1.We need find minimum value of P=x^4 +y^4 Since x,y>0,1=x+y=((√x)−(√y))^2 +2(√(xy))≥2(√(xy)) ⇒(√(xy))≤1/2⇒xy≤1/4(1).Hence, x^4 +y^4 =(x+y)^4 −4xy(x^2 +y^4 )−6x^2 y^2 =1−4xy[(x+y)^2 −2xy]−6x^2 y^2 =1−4xy(1−2xy)−6(xy)^2 =1−4xy+2(xy)^2 =2(xy−(1/4))^2 −3xy+(7/8)≥0−3.(1/4)+(7/8) =(1/8).The equality ocurrs if and only if x=y=1/2⇔a=b=^(505) (√(1/2)) Thus (a^(2020) +b^(2020) )_(min) =(1/8) when a=b=(1/( ^(505) (√2)))](Q115044.png)
Answered by bemath last updated on 23/Sep/20

| ||
Question and Answers Forum | ||
Question Number 115033 by bobhans last updated on 23/Sep/20 | ||
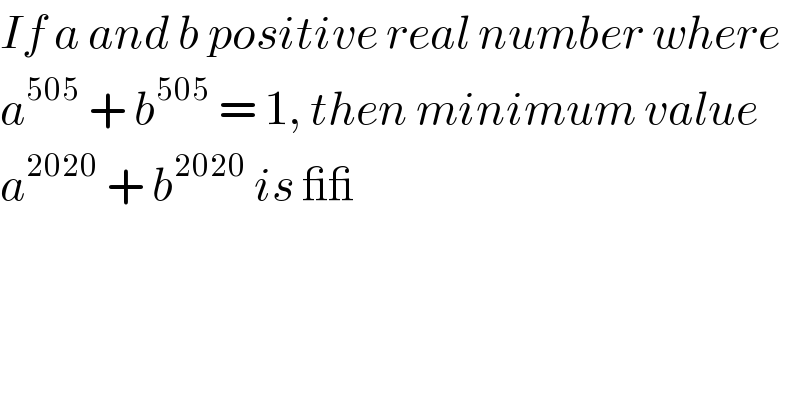 | ||
Answered by 1549442205PVT last updated on 23/Sep/20 | ||
![Put a^(505) =x,b^(505) =y⇒x+y=1.We need find minimum value of P=x^4 +y^4 Since x,y>0,1=x+y=((√x)−(√y))^2 +2(√(xy))≥2(√(xy)) ⇒(√(xy))≤1/2⇒xy≤1/4(1).Hence, x^4 +y^4 =(x+y)^4 −4xy(x^2 +y^4 )−6x^2 y^2 =1−4xy[(x+y)^2 −2xy]−6x^2 y^2 =1−4xy(1−2xy)−6(xy)^2 =1−4xy+2(xy)^2 =2(xy−(1/4))^2 −3xy+(7/8)≥0−3.(1/4)+(7/8) =(1/8).The equality ocurrs if and only if x=y=1/2⇔a=b=^(505) (√(1/2)) Thus (a^(2020) +b^(2020) )_(min) =(1/8) when a=b=(1/( ^(505) (√2)))](Q115044.png) | ||
| ||
Answered by bemath last updated on 23/Sep/20 | ||
 | ||