
Question and Answers Forum
Question Number 115229 by mathdave last updated on 24/Sep/20
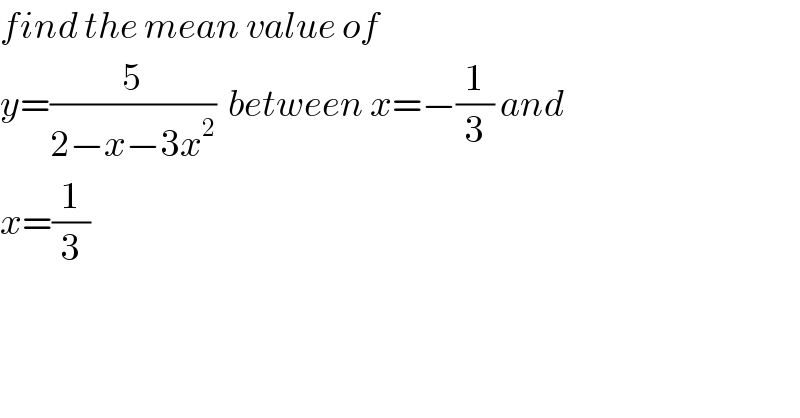
Answered by Olaf last updated on 24/Sep/20
![y^_ = (1/((1/3)−(−(1/3))))∫_(−(1/3)) ^(+(1/3)) (5/(2−x−3x^2 ))dx y^_ = −(5/2)∫_(−(1/3)) ^(+(1/3)) (1/(x^2 +(1/3)x−(2/3)))dx y^_ = −(5/2)∫_(−(1/3)) ^(+(1/3)) (1/((x+(1/6))^2 −((25)/(36))))dx y^_ = −(5/2)∫_(−(1/3)) ^(+(1/3)) (1/(((25)/(36))[((6/5)(x+(1/6)))^2 −1]))dx u = (1/5)(6x+1) y^_ = −3∫_(−(1/5)) ^(+(3/5)) (1/(u^2 −1))du y^_ = −3[(1/2)ln∣((u−1)/(u+1))∣]_(−(1/5)) ^(3/5) y^_ = −(3/2)[ln∣((−(2/5))/(8/5))∣−ln∣((−(6/5))/(4/5))∣] y^_ = −(3/2)[ln((1/4))−ln((3/2))] y^_ = −(3/2)ln((1/6)) y^_ = (3/2)ln6](Q115251.png)
Commented by mathdave last updated on 24/Sep/20

Answered by Bird last updated on 24/Sep/20
![the mean value is (1/(b−a))∫_a ^b f(x)dx =(1/((1/3)−(−(1/3))))∫_(−(1/3)) ^(1/(3 )) (5/(−3x^2 −x+2))dx =−((15)/2) ∫_(−(1/3)) ^(1/3) (dx/(3x^2 +x−2)) Δ =1−4(−6) =25 ⇒x_1 =((−1+5)/6) =(2/3) and x_2 =((−1−5)/6) =−1 ⇒ M_f =−((15)/2) ∫_(−(1/3)) ^(1/3) (dx/(3(x−(2/3))(x+1))) =−(5/2)×(3/5) ∫_(−(1/3)) ^(1/3) ((1/(x−(2/3)))−(1/(x+1)))dx =−(3/2)[ln∣((x−(2/3))/(x+1))∣]_(−(1/3)) ^(1/3) =−(3/2){ln∣((−(1/3))/(4/3))∣−ln∣((−1)/(2/3))∣} =−(3/2){ln((3/4))−ln((3/2))} =−(3/2)ln((3/4)×(2/3)) =−(3/2)ln((1/2)) =((3ln(2))/2)](Q115295.png)
| ||
Question and Answers Forum | ||
Question Number 115229 by mathdave last updated on 24/Sep/20 | ||
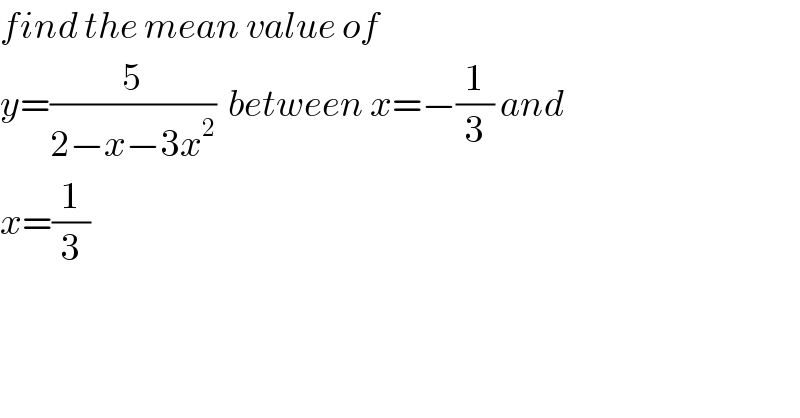 | ||
Answered by Olaf last updated on 24/Sep/20 | ||
![y^_ = (1/((1/3)−(−(1/3))))∫_(−(1/3)) ^(+(1/3)) (5/(2−x−3x^2 ))dx y^_ = −(5/2)∫_(−(1/3)) ^(+(1/3)) (1/(x^2 +(1/3)x−(2/3)))dx y^_ = −(5/2)∫_(−(1/3)) ^(+(1/3)) (1/((x+(1/6))^2 −((25)/(36))))dx y^_ = −(5/2)∫_(−(1/3)) ^(+(1/3)) (1/(((25)/(36))[((6/5)(x+(1/6)))^2 −1]))dx u = (1/5)(6x+1) y^_ = −3∫_(−(1/5)) ^(+(3/5)) (1/(u^2 −1))du y^_ = −3[(1/2)ln∣((u−1)/(u+1))∣]_(−(1/5)) ^(3/5) y^_ = −(3/2)[ln∣((−(2/5))/(8/5))∣−ln∣((−(6/5))/(4/5))∣] y^_ = −(3/2)[ln((1/4))−ln((3/2))] y^_ = −(3/2)ln((1/6)) y^_ = (3/2)ln6](Q115251.png) | ||
| ||
Commented by mathdave last updated on 24/Sep/20 | ||
 | ||
Answered by Bird last updated on 24/Sep/20 | ||
![the mean value is (1/(b−a))∫_a ^b f(x)dx =(1/((1/3)−(−(1/3))))∫_(−(1/3)) ^(1/(3 )) (5/(−3x^2 −x+2))dx =−((15)/2) ∫_(−(1/3)) ^(1/3) (dx/(3x^2 +x−2)) Δ =1−4(−6) =25 ⇒x_1 =((−1+5)/6) =(2/3) and x_2 =((−1−5)/6) =−1 ⇒ M_f =−((15)/2) ∫_(−(1/3)) ^(1/3) (dx/(3(x−(2/3))(x+1))) =−(5/2)×(3/5) ∫_(−(1/3)) ^(1/3) ((1/(x−(2/3)))−(1/(x+1)))dx =−(3/2)[ln∣((x−(2/3))/(x+1))∣]_(−(1/3)) ^(1/3) =−(3/2){ln∣((−(1/3))/(4/3))∣−ln∣((−1)/(2/3))∣} =−(3/2){ln((3/4))−ln((3/2))} =−(3/2)ln((3/4)×(2/3)) =−(3/2)ln((1/2)) =((3ln(2))/2)](Q115295.png) | ||
| ||