solve the Cauchy-Euler Differential Equation by substituting x=e^t x^3 (d^3 y/dx^3 ) + 2x^2 (d^2 y/dx^2 ) + 2y = 10x + ((10)/x)


Question and Answers Forum

All Questions Topic List
Differential Equation Questions
Previous in All Question Next in All Question
Previous in Differential Equation Next in Differential Equation
Question Number 116105 by ShakaLaka last updated on 30/Sep/20

$s o l v e t h e C a u c h y - E u l e r$ $D i f f e r e n t i a l E q u a t i o n b y$ $s u b s t i t u t i n g x = e^{t}$ $x^{3} \frac{d^{3} y}{{d x}^{3}} + 2 x^{2} \frac{d^{2} y}{{d x}^{2}} + 2 y = 10 x + \frac{10}{x}$
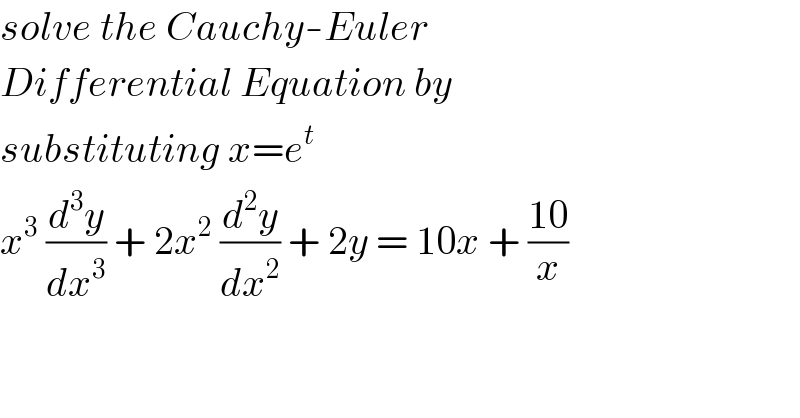
	Answered by TANMAY PANACEA last updated on 01/Oct/20
	$x=e^t →(dx/dt)=e^t (dy/dx)=(dy/dt)×(dt/dx)=(dy/dt)×(1/e^t )→e^t (dy/dx)=(dy/dt)→x(dy/dx)=(dy/dt)← (d/dx)(x(dy/dx))=(d/dt)((dy/dt))×(dt/dx) (x(d^2 y/dx^2 )+(dy/dx))×(dx/dt)=(d^2 y/dt^2 ) x(x(d^2 y/dx^2 )+(dy/dx))=(d^2 y/dt^2 ) x^2 (d^2 y/dx^2 )=(d^2 y/dt^2 )−(dy/dt)← (d/dx)(x^2 (d^2 y/dx^2 ))=(d/dt)((d^2 y/dt^2 )−(dy/dt))×(dt/dx) (x^2 (d^3 y/dx^3 )+2x.(d^2 y/dx^2 ))×(dx/dt)=(d^3 y/dt^3 )−(d^2 y/dt^2 ) x^3 (d^3 y/dx^3 )+2x^2 (d^2 y/dx^2 )=(d^3 y/dt^3 )−(d^2 y/dt^2 ) ★ so (d^3 y/dt^3 )−(d^2 y/dt^2 )+2y=10e^t +10e^(−t) now to be solved C.F m^3 −m^2 +2=0 =m^3 +m^2 −2m^2 −2m+2m+2 =m^2 (m+1) −2m (m+1) +2 (m+1) =(m+1)(m^2 −2m+2) m+1=0 m=−1 m^2 −2m+2=0 m=((2±(√(4−8)))/2)=((2±2i)/2)=1±i so m=−1,1±i C.F y=C_1 e^(−t) +C_2 e^((1+i)t) +C_3 e^((1−i)t) P.I correction pls(in black ) y=((10e^t )/(D^3 −D^2 +2))+((10e^(−t) )/(D^3 −D^2 +2)) [D=(d/dt)] (y/(10))=(e^t /(1−1+2))+(e^(−t) /((D+1)(D^2 −2D+2))) =(e^t /2)+(e^(−t) /((D−1+1){(−1)^2 −2(−1)+2})) =(e^t /2)+(e^(−t) /5)×(1/D) =(e^t /2)+(e^(−t) /5)×t y=C.F+P.I y=C_1 e^(−t) +C_2 e^((1+i)t) +C_3 e^((1−i)t) +[(e^t /2)+(e^(−t) /5)×t]×10 =C_1 x^(−1) +C_2 x^(1+i) +C_3 x^(1−i) +((5x)/1)+((2x^(−1) )/)×lnx$
	$x = e^{t} \to \frac{d x}{d t} = e^{t}$ $\frac{d y}{d x} = \frac{d y}{d t} \times \frac{d t}{d x} = \frac{d y}{d t} \times \frac{1}{e^{t}} \to e^{t} \frac{d y}{d x} = \frac{d y}{d t} \to x \frac{d y}{d x} = \frac{d y}{d t} \leftarrow$ $\frac{d}{d x} (x \frac{d y}{d x}) = \frac{d}{d t} (\frac{d y}{d t}) \times \frac{d t}{d x}$ $(x \frac{d^{2} y}{{d x}^{2}} + \frac{d y}{d x}) \times \frac{d x}{d t} = \frac{d^{2} y}{{d t}^{2}}$ $x (x \frac{d^{2} y}{{d x}^{2}} + \frac{d y}{d x}) = \frac{d^{2} y}{{d t}^{2}}$ $x^{2} \frac{d^{2} y}{{d x}^{2}} = \frac{d^{2} y}{{d t}^{2}} - \frac{d y}{d t} \leftarrow$ $\frac{d}{d x} (x^{2} \frac{d^{2} y}{{d x}^{2}}) = \frac{d}{d t} (\frac{d^{2} y}{{d t}^{2}} - \frac{d y}{d t}) \times \frac{d t}{d x}$ $(x^{2} \frac{d^{3} y}{{d x}^{3}} + 2 x . \frac{d^{2} y}{{d x}^{2}}) \times \frac{d x}{d t} = \frac{d^{3} y}{{d t}^{3}} - \frac{d^{2} y}{{d t}^{2}}$ $x^{3} \frac{d^{3} y}{{d x}^{3}} + 2 x^{2} \frac{d^{2} y}{{d x}^{2}} = \frac{d^{3} y}{{d t}^{3}} - \frac{d^{2} y}{{d t}^{2}} ★$ $s o$ $\frac{d^{3} y}{{d t}^{3}} - \frac{d^{2} y}{{d t}^{2}} + 2 y = 10 e^{t} + 10 e^{- t}$ $n o w t o b e s o l v e d$ $C . F$ $m^{3} - m^{2} + 2 = 0$ $= m^{3} + m^{2} - 2 m^{2} - 2 m + 2 m + 2$ $= m^{2} (m + 1) - 2 m (m + 1) + 2 (m + 1)$ $= (m + 1) (m^{2} - 2 m + 2)$ $m + 1 = 0 m = - 1$ $m^{2} - 2 m + 2 = 0$ $m = \frac{2 \pm \sqrt{4 - 8}}{2} = \frac{2 \pm 2 i}{2} = 1 \pm i$ $s o m = - 1, 1 \pm i$ $C . F$ $y = C_{1} e^{- t} + C_{2} e^{(1 + i) t} + C_{3} e^{(1 - i) t}$ $P . I$ $c o r r e c t i o n p l s (i n b l a c k)$ $y = \frac{10 e^{t}}{D^{3} - D^{2} + 2} + \frac{10 e^{- t}}{D^{3} - D^{2} + 2} [D = \frac{d}{d t}]$ $\frac{y}{10} = \frac{e^{t}}{1 - 1 + 2} + \frac{e^{- t}}{(D + 1) (D^{2} - 2 D + 2)}$ $= \frac{e^{t}}{2} + \frac{e^{- t}}{(D - 1 + 1) {{(- 1)}^{2} - 2 (- 1) + 2}}$ $= \frac{e^{t}}{2} + \frac{e^{- t}}{5} \times \frac{1}{D}$ $= \frac{e^{t}}{2} + \frac{e^{- t}}{5} \times t$ $y = C . F + P . I$ $y = C_{1} e^{- t} + C_{2} e^{(1 + i) t} + C_{3} e^{(1 - i) t} + [\frac{e^{t}}{2} + \frac{e^{- t}}{5} \times t] \times 10$ $= C_{1} x^{- 1} + C_{2} x^{1 + i} + C_{3} x^{1 - i} + \frac{5 x}{1} + \frac{2 x^{- 1}}{} \times l n x$
	Commented by ShakaLaka last updated on 01/Oct/20

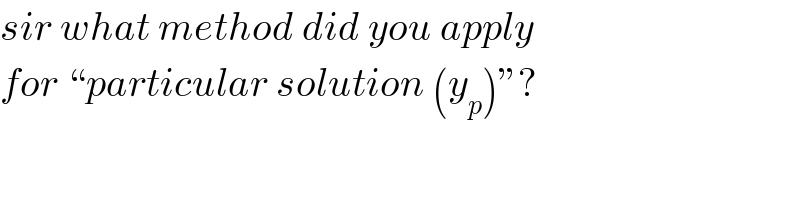
	$s i r w h a t m e t h o d d i d y o u a p p l y$ $f o r ‘ ‘ p a r t i c u l a r s o l u t i o n {(y_{p})}^{″} ?$
	Commented by ShakaLaka last updated on 01/Oct/20

	$s i r c a n w e a p p l y m o t h o d o f$ $u n d e t e r m i n e d c o e f f i t i e n t ? i f$ $y e s t h e n w h a t w i l l w e t a k e f o r$ $y_{p} f o r t h e R . H . S$ $Prime causes double exponent: use braces to clarify$
	Commented by TANMAY PANACEA last updated on 01/Oct/20

	$i f o r g o t t o p u t 10 . . .$ $l a t e r c o r r e c t e d$
	Commented by ShakaLaka last updated on 04/Oct/20

	$o h o k I g o t i t . T h a n k y o u :)$
	Answered by mindispower last updated on 01/Oct/20
	$y(x)=y(e^t )=z(t) x=e^t (dy/dx)=(dz/dt).(dt/dx)=z′(t).e^(−t) (d^2 y/dx^2 )=(z′′(t)e^(−t) −z′(t)e^(−t) ).e^(−t) (d^3 y/dx^3 )=(z′′′e^(−2t) −3z′′e^(−2t) +2z′e^(−2t) )e^(−t) equation equivalente e^(3t) (z′′′e^(−3t) −3z′′e^(−2t) +2z′e^(−3t) )+2e^(2t) (z′′−z′)e^(2t) +2z(t)=10(e^t +e^(−t) )=20ch(t) ⇔z′′′−z′′+2z=20ch(t) homegenius Z′′′−Z′′+2Z=0 X^3 −X^2 +2=0⇒(X+1)(X^2 −2X+2)=0 X∈{−1,1−i,1+i} Z(t)=se^(−t) +e^t (acos(t)+bsin(t)) particular solution z=ae^t +bte^(−t) z′=ae^t +be^(−t) −bte^(−t) z′′=ae^t −2be^(−t) +bte^(−t) z′′′=ae^t +3be^(−t) −bte^(−t) ⇒(ae^t +3be^(−t) −bte^(−t) )−(ae^t −2be^(−t) +bte^(−t) ) +2ae^t +2bte^(−t) =20ch(t) ⇒2ae^t +5be^(−t) =20ch(t) ⇒2a=10,5b=10 a=5,b=2 z(t)=se^(−t) +e^t (acos(t)+bsin(t))+5e^t +2te^(−t) y(x)=z(ln(x)) =(s/x)+x(acos(lnx)+bsin(ln(x))+5x+((2ln(x))/x)$
	$y (x) = y (e^{t}) = z (t)$ $x = e^{t}$ $\frac{d y}{d x} = \frac{d z}{d t} . \frac{d t}{d x} = z^{'} (t) . e^{- t}$ $\frac{d^{2} y}{{d x}^{2}} = (z^{″} (t) e^{- t} - z^{'} (t) e^{- t}) . e^{- t}$ $\frac{d^{3} y}{{d x}^{3}} = (z^{‴} e^{- 2 t} - 3 z^{″} e^{- 2 t} + 2 z^{'} e^{- 2 t}) e^{- t}$ $e q u a t i o n$ $e q u i v a l e n t e$ $e^{3 t} (z^{‴} e^{- 3 t} - 3 z^{″} e^{- 2 t} + 2 z^{'} e^{- 3 t}) + 2 e^{2 t} (z^{″} - z^{'}) e^{2 t}$ $+ 2 z (t) = 10 (e^{t} + e^{- t}) = 20 c h (t)$ $\Leftrightarrow z^{‴} - z^{″} + 2 z = 20 c h (t)$ $h o m e g e n i u s$ $Z^{‴} - Z^{″} + 2 Z = 0$ $X^{3} - X^{2} + 2 = 0 \Rightarrow (X + 1) (X^{2} - 2 X + 2) = 0$ $X \in {- 1, 1 - i, 1 + i}$ $Z (t) = {s e}^{- t} + e^{t} (a c o s (t) + b s i n (t))$ $p a r t i c u l a r s o l u t i o n$ $z = {a e}^{t} + {b t e}^{- t}$ $z^{'} = {a e}^{t} + {b e}^{- t} - {b t e}^{- t}$ $z^{″} = {a e}^{t} - 2 {b e}^{- t} + {b t e}^{- t}$ $z^{‴} = {a e}^{t} + 3 {b e}^{- t} - {b t e}^{- t}$ $\Rightarrow ({a e}^{t} + 3 {b e}^{- t} - {b t e}^{- t}) - ({a e}^{t} - 2 {b e}^{- t} + {b t e}^{- t})$ $+ 2 {a e}^{t} + 2 {b t e}^{- t} = 20 c h (t)$ $\Rightarrow 2 {a e}^{t} + 5 {b e}^{- t} = 20 c h (t)$ $\Rightarrow 2 a = 10, 5 b = 10$ $a = 5, b = 2$ $z (t) = {s e}^{- t} + e^{t} (a c o s (t) + b s i n (t)) + 5 e^{t} + 2 {t e}^{- t}$ $y (x) = z (l n (x))$ $= \frac{s}{x} + x (a c o s (l n x) + b s i n (l n (x)) + 5 x + \frac{2 l n (x)}{x}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum