∫_0 ^( ∞) ((tan^(−1) ((x/4))−tan^(−1) ((x/6)))/x) dx =?


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 117213 by bobhans last updated on 10/Oct/20

$\underset{0}{\int^{\infty}} \frac{\tan^{- 1} (\frac{x}{4}) - \tan^{- 1} (\frac{x}{6})}{x} dx = ?$
	Answered by TANMAY PANACEA last updated on 10/Oct/20

	$t a k i n g h e l p f r o m b o o k s$ $F r u l l a n i i n t r e g a l$ $\int_{0}^{\infty} \frac{f (a x) - f (b x)}{x} d x = (A - B) l n (\frac{b}{a})$ $A = \lim_{x \to 0} f (x) B = \lim_{x \to \infty} f (x)$ $h e r e f (x) = {t a n}^{- 1} (x)$ $\lim_{x \to 0} {t a n}^{- 1} (x) = 0 = A$ $\lim_{x \to \infty} {t a n}^{- 1} (x) = {t a n}^{- 1} (\infty) = \frac{π}{2} = B$ $a = \frac{1}{4} b = \frac{1}{6}$ $a n s w e r = (0 - \frac{π}{2}) l n (\frac{1 \times 4}{6 \times 1}) = \frac{π}{2} l n (\frac{3}{2})$
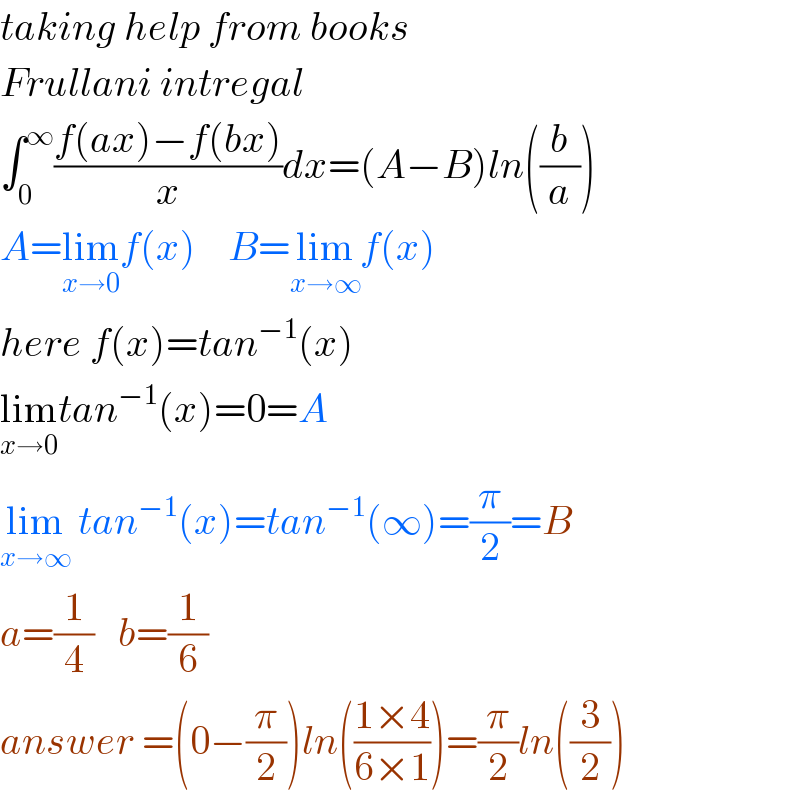
	Commented by bobhans last updated on 10/Oct/20

	$waw . . . . do you have that book ?$
	Commented by TANMAY PANACEA last updated on 10/Oct/20

	$y e s s i r . . . h a r d c o p y . . . i d o n o t k n o w h o w t o s h a r e i m a g e$ $o r d o c u m e n t s i n p d f f o r m$
	Commented by bemath last updated on 10/Oct/20

	$m a y b e s i r c a n s e n t b y g m a i l$
	Answered by AbduraufKodiriy last updated on 10/Oct/20

	$I = \int_{0}^{\infty} \frac{a r c t a n (\frac{x}{4}) - a r c t a n (\frac{x}{6})}{x} d x = \int_{0}^{\infty} \frac{a r c t a n (\frac{2 x}{x^{2} + 24})}{x} d x$ $I (t) = \int_{0}^{\infty} \frac{a r c t a n (\frac{2 t x}{x^{2} + 24})}{x} d x \Rightarrow I (0) = 0$ $I^{'} (t) = \int_{0}^{\infty} \partial_{t} \frac{a r c t a n (\frac{2 t x}{x^{2} + 24})}{x} d x = \int_{0}^{\infty} \frac{2 (x^{2} + 24)}{x^{4} + 48 x^{2} + 576 + 4 t^{2} x^{2}} d x =$ $= 2 \int_{0}^{\infty} \frac{1 + \frac{24}{x^{2}}}{x^{2} + \frac{576}{x^{2}} + 48 + 4 t^{2}} d x = 2 \int_{0}^{\infty} \frac{d (x - \frac{24}{x})}{{(x - \frac{24}{x})}^{2} + 96 + 4 t^{2}} =$ $= \frac{2}{2 \sqrt{24 + t^{2}}} a r c t a n (\frac{x - \frac{24}{x}}{2 \sqrt{24 + t^{2}}}) ∣_{0}^{\infty} + C = \frac{1}{\sqrt{24 + t^{2}}} a r c t a n (\frac{x^{2} - 24}{2 x \sqrt{24 + t^{2}}}) ∣_{0}^{\infty} =$ $= \frac{1}{\sqrt{24 + t^{2}}} (\frac{π}{2} - (- \frac{π}{2})) = \frac{π}{\sqrt{24 + t^{2}}} \Rightarrow$ $\Rightarrow I (t) = π l n (t + \sqrt{t^{2} + 24}) + C$ $I (0) = \frac{π}{2} l n 24 + C = 0 \Rightarrow C = - \frac{π}{2} l n 24$ $S o, I (t) = π l n (t + \sqrt{t^{2} + 24}) - \frac{π}{2} l n 24$ $I = I (1) = π l n (1 + 5) - \frac{π}{2} l n 24 = \frac{π}{2} l n \frac{36}{24} = \frac{π}{2} l n \frac{3}{2}$
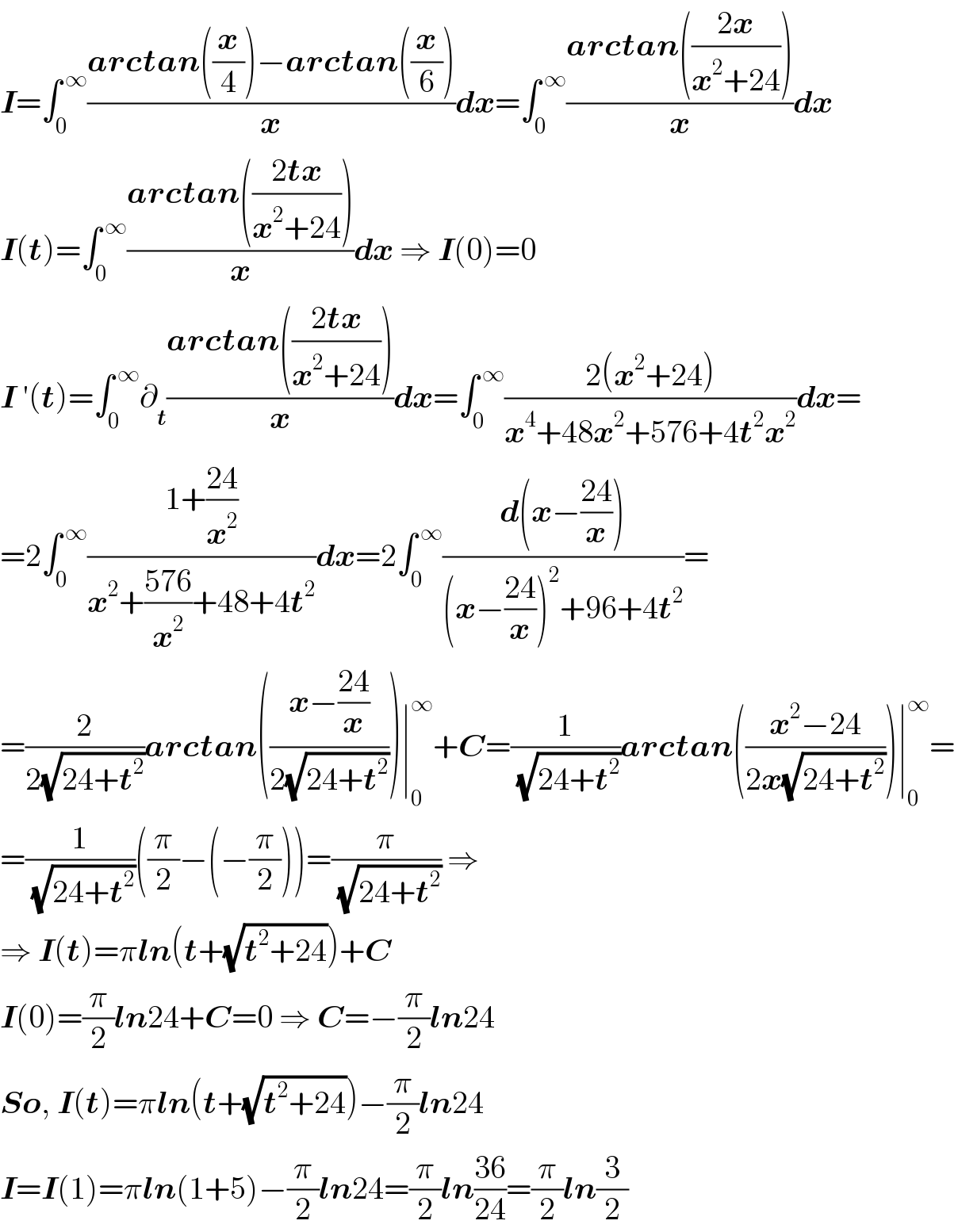
	Answered by mathmax by abdo last updated on 10/Oct/20

	$I = \int_{0}^{\infty} \frac{\arctan (ax) - \arctan (bx)}{x} dx = limI (ξ) with I (ξ) = \int_{0}^{ξ} \frac{\arctan (ax) - \arctan (bx)}{x} dx = I$ $\int_{0}^{\infty} (. . .) dx = \lim_{ξ \to + \infty} I (ξ)$ $I (ξ) = \int_{0}^{ξ} \frac{\arctan (ax)}{x} dx (\to ax = u) - \int_{0}^{ξ} \frac{\arctan (bx)}{x} dx (bx = v)$ $= \int_{0}^{a ξ} \frac{arctanu}{u} du - \int_{0}^{b ξ} \frac{arctanv}{v} dv = \int_{b ξ}^{a ξ} \frac{\arctan (u)}{u} du$ $\exists c \in] b ξ, a ξ [/ \int_{b ξ}^{a ξ} \frac{\arctan (u)}{u} du = arctanc \int_{b ξ}^{a ξ} \frac{du}{u} = arctancln (\frac{a}{b})$ $\Rightarrow \lim_{ξ \to + \infty} I (ξ) = \frac{π}{2} \ln (\frac{a}{b}) with a = \frac{1}{4} and b = \frac{1}{6} \Rightarrow \frac{a}{b} = \frac{6}{4} = \frac{3}{2}$ $\Rightarrow \int_{0}^{\infty} \frac{\arctan (\frac{x}{4}) - \arctan (\frac{x}{6})}{x} dx = \frac{π}{2} \ln (\frac{3}{2})$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum