Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

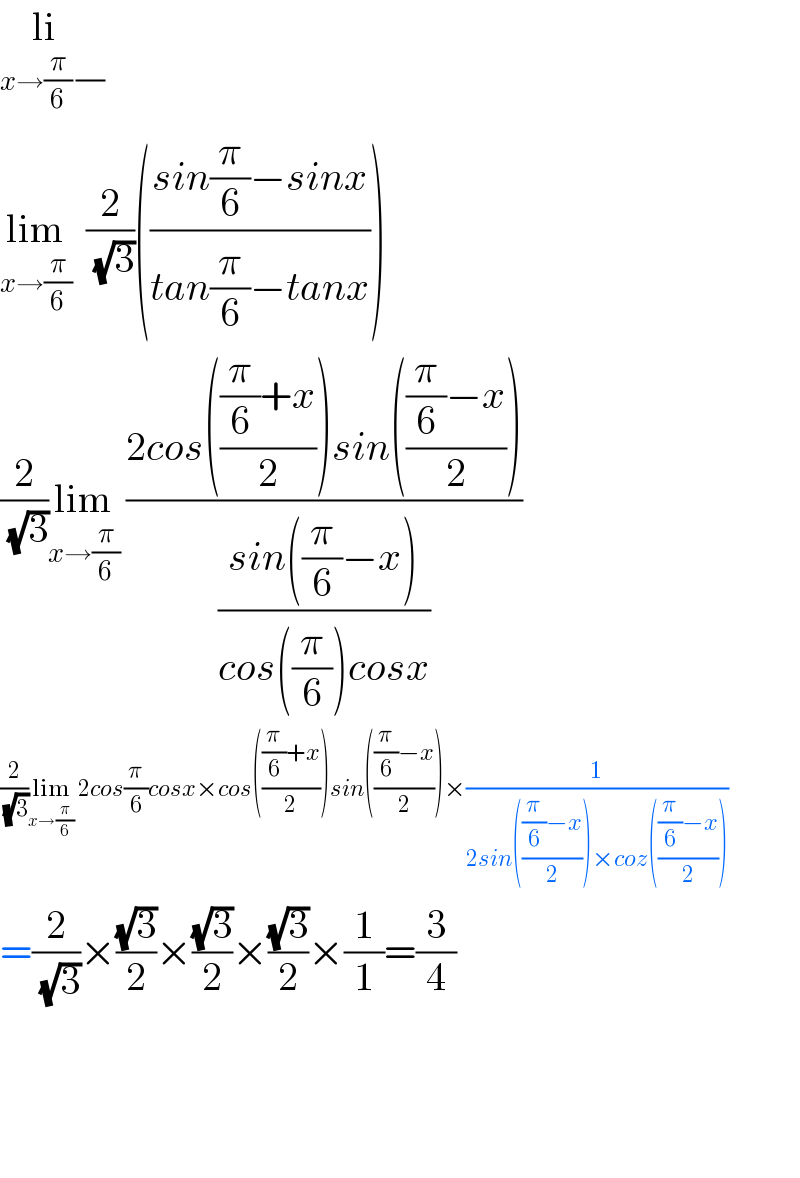
Question Number 117412 by bobhans last updated on 11/Oct/20

$$\underset{x\to \frac{\pi }{6}}{\lim }\frac{1-2\sin \mathrm{x}}{1-\sqrt{3}\tan \mathrm{x}}=?$$

Commented by Lordose last updated on 11/Oct/20

$$\mathrm{you}\mathrm{edited}\mathrm{the}\mathrm{question}.?$$

Commented by bobhans last updated on 11/Oct/20

$$\mathrm{yes}.\mathrm{typo}$$

Answered by Lordose last updated on 11/Oct/20

$$\frac{1-2\left(\frac{1}{2}\right)}{\sqrt{3}-\frac{\sqrt{3}}{3}}=\frac{0}{\frac{2\sqrt{3}}{3}}=0$$

Answered by Olaf last updated on 11/Oct/20

$$\underset{x\to \frac{\pi }{6}}{\lim }\frac{-2\cos x}{-\sqrt{3}(1+{\tan }^{2}x)}=\frac{-2\frac{\sqrt{3}}{2}}{-\sqrt{3}(1+{\left(\frac{1}{\sqrt{3}}\right)}^2)}$$$$=\frac{3}{4}$$$$\left({\mathrm{Hospital}}^{\prime }\mathrm{s}\mathrm{rule}\right)$$

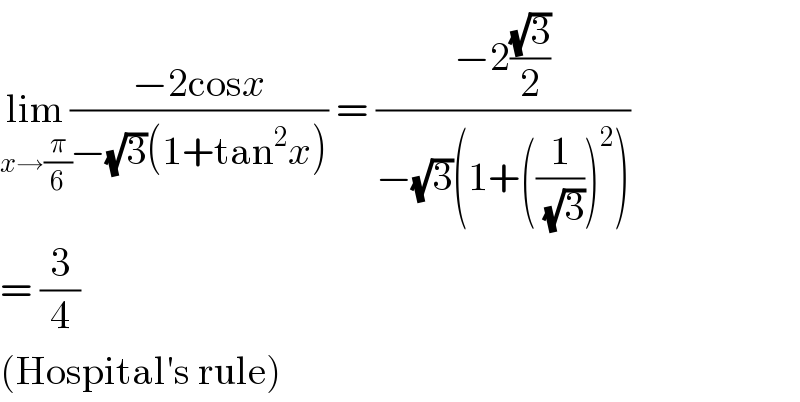
Answered by bemath last updated on 11/Oct/20

$$\underset{x\to \frac{\pi }{6}}{\lim }\frac{1-2\sin \mathrm{x}}{1-\sqrt{3}\tan \mathrm{x}}=?$$$$\mathrm{Solution}:$$$$\mathrm{Without}{\mathrm{L}}^{\prime }\mathrm{Hopital}$$$$\mathrm{letting}\mathrm{w}=\mathrm{x}-\frac{\pi }{6}$$$$\underset{\mathrm{w}\to 0}{\lim }\frac{1-2\sin (\mathrm{w}+\frac{\pi }{6})}{1-\sqrt{3}\tan (\mathrm{w}+\frac{\pi }{6})}=$$$$\underset{\mathrm{w}\to 0}{\lim }\frac{1-2(\frac{\sqrt{3}}{2}\sin \mathrm{w}+\frac{1}{2}\cos \mathrm{w})}{1-\sqrt{3}\left(\frac{\frac{1}{\sqrt{3}}+\tan \mathrm{w}}{1-\frac{1}{\sqrt{3}}\tan \mathrm{w}}\right)}=$$$$\underset{\mathrm{w}\to 0}{\lim }\frac{1-\sqrt{3}\sin \mathrm{w}-\cos \mathrm{w}}{1-\sqrt{3}\left(\frac{1+\sqrt{3}\tan \mathrm{w}}{\sqrt{3}-\tan \mathrm{w}}\right)}=$$$$\underset{\mathrm{w}\to 0}{\lim }\frac{(\sqrt{3}-\tan \mathrm{w})(1-\cos \mathrm{w}-\sqrt{3}\sin \mathrm{w})}{\sqrt{3}-\tan \mathrm{w}-\sqrt{3}-3\tan \mathrm{w}}=$$$$\sqrt{3}\underset{\mathrm{w}\to 0}{\lim }\frac{2\sin {}^2\left(\frac{1}{2}\mathrm{w}\right)-\sqrt{3}\sin \mathrm{w}}{-4\tan \mathrm{w}}=$$$$\sqrt{3}\underset{\mathrm{w}\to 0}{\lim }\frac{2\sin \left(\frac{1}{2}\mathrm{w}\right)(\sin \left(\frac{1}{2}\mathrm{w}\right)-\sqrt{3}\cos \left(\frac{1}{2}\mathrm{w}\right))}{-4\tan \mathrm{w}}=$$$$\frac{\sqrt{3}}{-4}\times (-\sqrt{3})=\frac{3}{4}$$

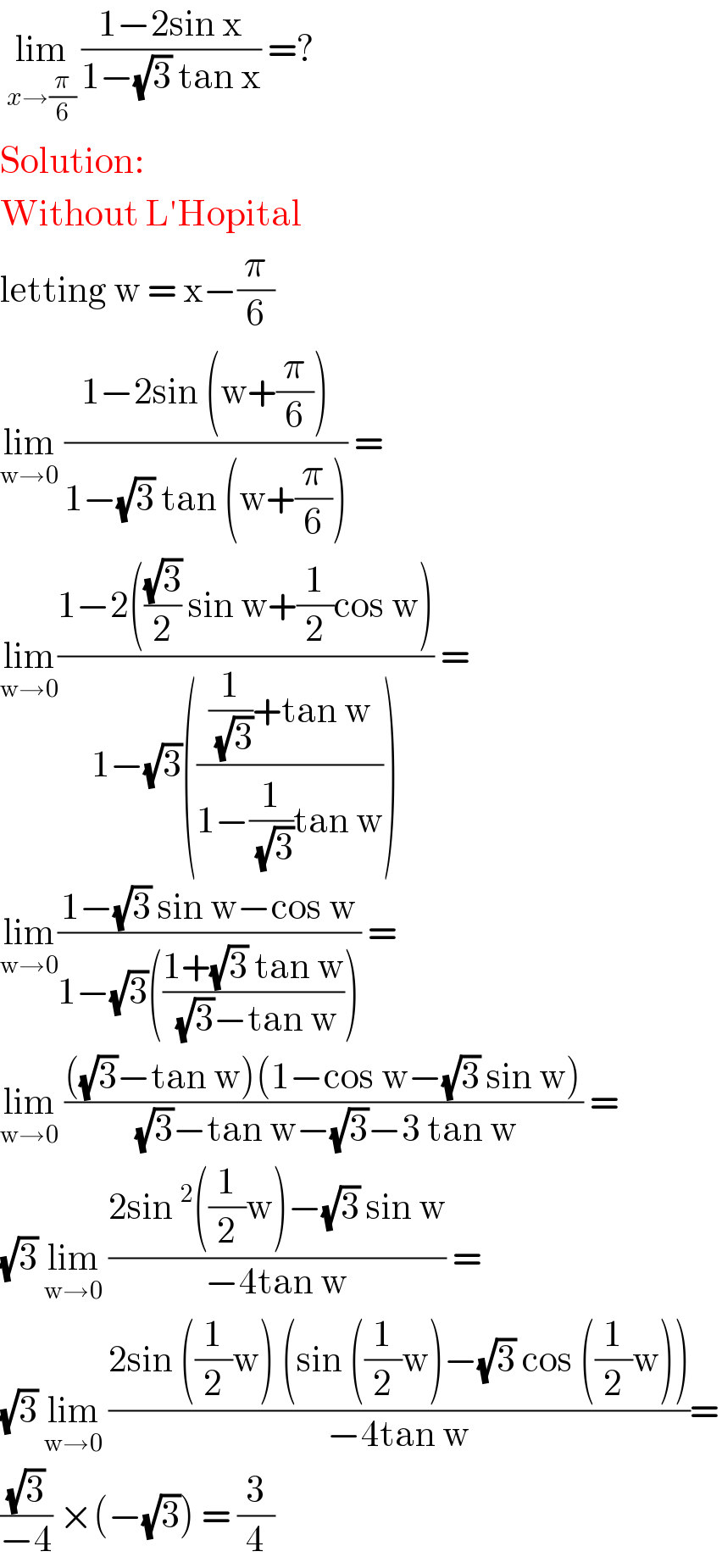
Commented by TANMAY PANACEA last updated on 11/Oct/20

$$\underset{x\to \frac{\pi }{6}\frac{}{}}{\mathrm{li}}$$$$\underset{x\to \frac{\pi }{6}}{\lim }\frac{2}{\sqrt{3}}\left(\frac{sin\frac{\pi }{6}-sinx}{tan\frac{\pi }{6}-tanx}\right)$$$$\frac{2}{\sqrt{3}}\underset{x\to \frac{\pi }{6}}{\lim }\frac{2cos\left(\frac{\frac{\pi }{6}+x}{2}\right)sin\left(\frac{\frac{\pi }{6}-x}{2}\right)}{\frac{sin(\frac{\pi }{6}-x)}{cos\left(\frac{\pi }{6}\right)cosx}}$$$$\frac{2}{\sqrt{3}}\underset{x\to \frac{\pi }{6}}{\lim }2cos\frac{\pi }{6}cosx\times cos\left(\frac{\frac{\pi }{6}+x}{2}\right)sin\left(\frac{\frac{\pi }{6}-x}{2}\right)\times \frac{1}{2sin\left(\frac{\frac{\pi }{6}-x}{2}\right)\times coz\left(\frac{\frac{\pi }{6}-x}{2}\right)}$$$$=\frac{2}{\sqrt{3}}\times \frac{\sqrt{3}}{2}\times \frac{\sqrt{3}}{2}\times \frac{\sqrt{3}}{2}\times \frac{1}{1}=\frac{3}{4}$$$$$$$$$$$$$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com