
Question Number 117470 by TANMAY PANACEA last updated on 11/Oct/20
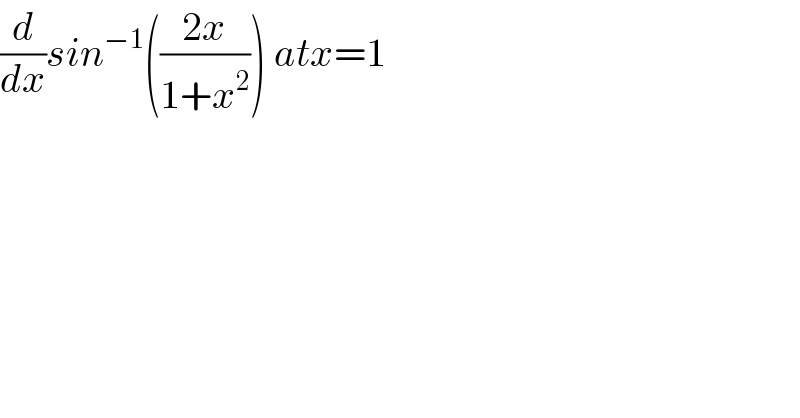
$$\frac{{d}}{{dx}}{sin}^{−\mathrm{1}} \left(\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right)\:{atx}=\mathrm{1} \\ $$
Commented by TANMAY PANACEA last updated on 12/Oct/20

$${thank}\:{you}\:{sir} \\ $$
Commented by Dwaipayan Shikari last updated on 11/Oct/20
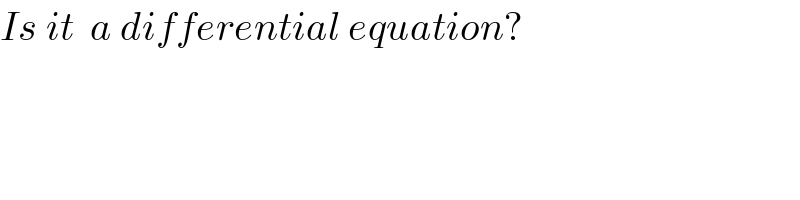
$${Is}\:{it}\:\:{a}\:{differential}\:{equation}? \\ $$
Commented by TANMAY PANACEA last updated on 11/Oct/20
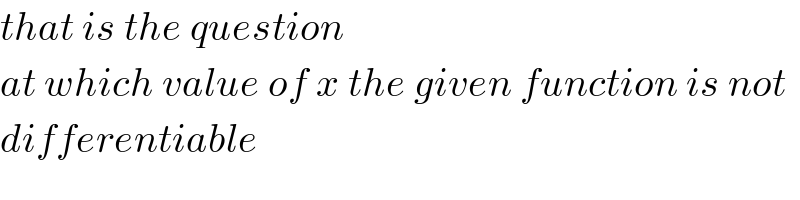
$${that}\:{is}\:{the}\:{question} \\ $$$${at}\:{which}\:{value}\:{of}\:{x}\:{the}\:{given}\:{function}\:{is}\:{not} \\ $$$${differentiable} \\ $$
Commented by MJS_new last updated on 12/Oct/20
![(d/dx)[arcsin ((2x)/(1+x^2 ))]=(1/( (√(1−(((2x)/(1+x^2 )))^2 ))))×((2(1−x^2 ))/((1+x^2 )^2 ))= =−(2/(1+x^2 ))sign (x^2 −1) but sign 0 is not defined ⇒ sin^(−1) ((2x)/(1+x^2 )) is not differentiable at x=±1](Q117479.png)
$$\frac{{d}}{{dx}}\left[\mathrm{arcsin}\:\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right]=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−\left(\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right)^{\mathrm{2}} }}×\frac{\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }= \\ $$$$=−\frac{\mathrm{2}}{\mathrm{1}+{x}^{\mathrm{2}} }\mathrm{sign}\:\left({x}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\mathrm{but}\:\mathrm{sign}\:\mathrm{0}\:\mathrm{is}\:\mathrm{not}\:\mathrm{defined} \\ $$$$\Rightarrow\:\mathrm{sin}^{−\mathrm{1}} \:\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\mathrm{is}\:\mathrm{not}\:\mathrm{differentiable}\:\mathrm{at}\:{x}=\pm\mathrm{1} \\ $$
Commented by TANMAY PANACEA last updated on 12/Oct/20

$$\boldsymbol{{from}}\:\boldsymbol{{graph}}\:\boldsymbol{{it}}\:\boldsymbol{{is}}\:\boldsymbol{{clear}}\:\boldsymbol{{that}}\:\boldsymbol{{we}}\:\boldsymbol{{can}}\:\boldsymbol{{not}}\:\boldsymbol{{draw}} \\ $$$$\boldsymbol{{tangent}}\:\boldsymbol{{at}}\:\boldsymbol{{x}}=\mathrm{1}\:\boldsymbol{{so}}\:\boldsymbol{{it}}\:\boldsymbol{{is}}\:\boldsymbol{{not}}\:\boldsymbol{{differentiable}} \\ $$$$\boldsymbol{{at}}\:\boldsymbol{{x}}=\mathrm{1} \\ $$
Answered by Lordose last updated on 11/Oct/20
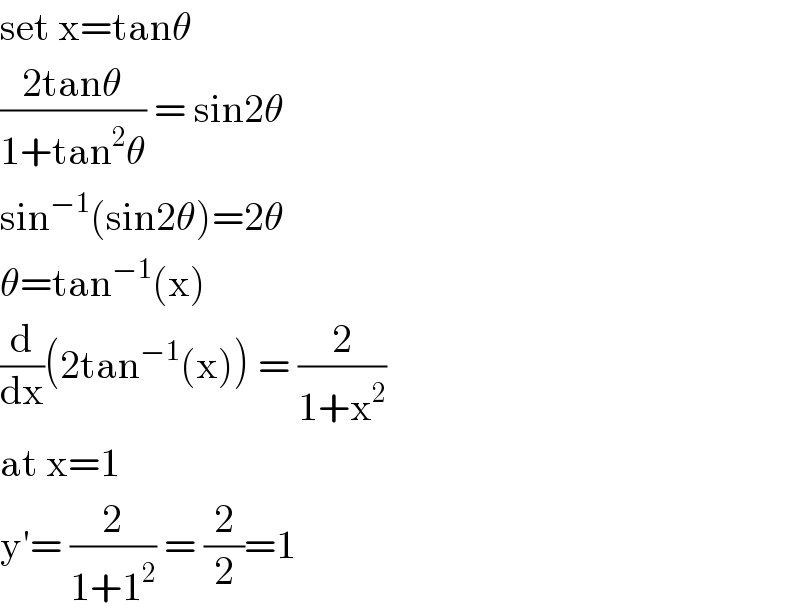
$$\mathrm{set}\:\mathrm{x}=\mathrm{tan}\theta \\ $$$$\frac{\mathrm{2tan}\theta}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta}\:=\:\mathrm{sin2}\theta \\ $$$$\mathrm{sin}^{−\mathrm{1}} \left(\mathrm{sin2}\theta\right)=\mathrm{2}\theta \\ $$$$\theta=\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{x}\right) \\ $$$$\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{2tan}^{−\mathrm{1}} \left(\mathrm{x}\right)\right)\:=\:\frac{\mathrm{2}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} } \\ $$$$\mathrm{at}\:\mathrm{x}=\mathrm{1} \\ $$$$\mathrm{y}'=\:\frac{\mathrm{2}}{\mathrm{1}+\mathrm{1}^{\mathrm{2}} }\:=\:\frac{\mathrm{2}}{\mathrm{2}}=\mathrm{1} \\ $$
Commented by TANMAY PANACEA last updated on 12/Oct/20
$${sir}\:{from}\:{graph}\:{it}\:{is}\:{clear}\:{that}\:{given}\:{function} \\ $$$${is}\:{not}\:{differntiable}\:{at}\:{x}=\mathrm{1} \\ $$
Answered by Olaf last updated on 11/Oct/20

$$\frac{{d}\mathrm{arcsin}{u}}{{du}}\:=\:\frac{{du}}{{dx}}.\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{u}^{\mathrm{2}} }} \\ $$$${u}\:=\:\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\frac{{du}}{{dx}}\:=\:\mathrm{2}\frac{\mathrm{1}+{x}^{\mathrm{2}} −{x}\left(\mathrm{2}{x}\right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\:=\:\frac{\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)} \\ $$$$\frac{{d}\mathrm{arcsin}{u}}{{du}}\:=\:\frac{\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} \sqrt{\mathrm{1}−\left(\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right)^{\mathrm{2}} }} \\ $$$$\frac{{d}\mathrm{arcsin}{u}}{{du}}\:=\:\frac{\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\sqrt{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{4}{x}^{\mathrm{2}} }} \\ $$$$\frac{{d}\mathrm{arcsin}{u}}{{du}}\:=\:\frac{\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\sqrt{\left({x}^{\mathrm{4}} −\mathrm{2}{x}^{\mathrm{2}} +\mathrm{1}\right.}} \\ $$$$\frac{{d}\mathrm{arcsin}{u}}{{du}}\:=\:\frac{\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\sqrt{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}}\:=\:\frac{\mathrm{2}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\Rightarrow\:\frac{{d}\mathrm{arcsin}{u}}{{du}}\:=\:\mathrm{1}\:\mathrm{at}\:{x}\:=\:\mathrm{1} \\ $$$$ \\ $$$$ \\ $$
Answered by mathmax by abdo last updated on 11/Oct/20
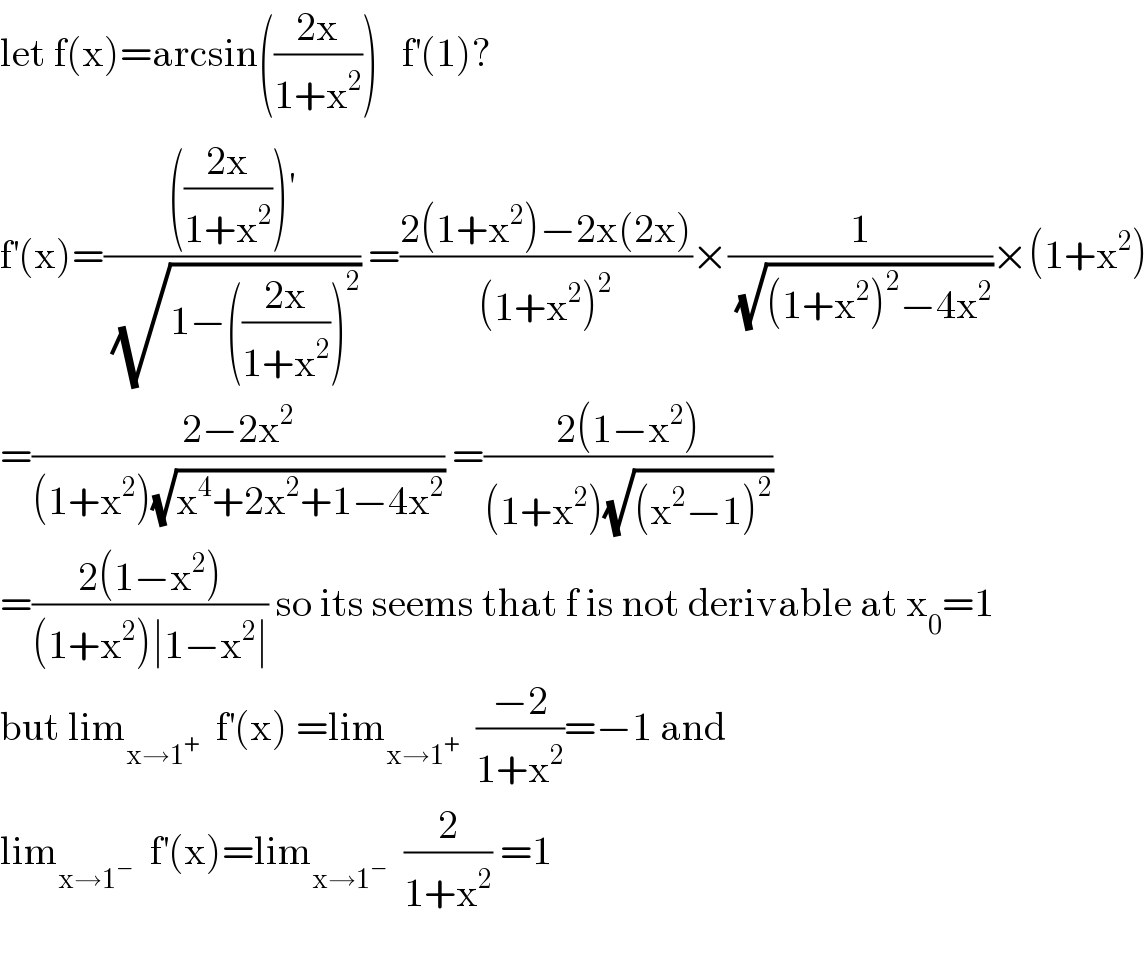
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{arcsin}\left(\frac{\mathrm{2x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\right)\:\:\:\mathrm{f}^{'} \left(\mathrm{1}\right)? \\ $$$$\mathrm{f}^{'} \left(\mathrm{x}\right)=\frac{\left(\frac{\mathrm{2x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\right)^{'} }{\sqrt{\mathrm{1}−\left(\frac{\mathrm{2x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\right)^{\mathrm{2}} }}\:=\frac{\mathrm{2}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)−\mathrm{2x}\left(\mathrm{2x}\right)}{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{2}} }×\frac{\mathrm{1}}{\sqrt{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{4x}^{\mathrm{2}} }}×\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right) \\ $$$$=\frac{\mathrm{2}−\mathrm{2x}^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\sqrt{\mathrm{x}^{\mathrm{4}} +\mathrm{2x}^{\mathrm{2}} +\mathrm{1}−\mathrm{4x}^{\mathrm{2}} }}\:=\frac{\mathrm{2}\left(\mathrm{1}−\mathrm{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\sqrt{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} }} \\ $$$$=\frac{\mathrm{2}\left(\mathrm{1}−\mathrm{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\mid\mathrm{1}−\mathrm{x}^{\mathrm{2}} \mid}\:\mathrm{so}\:\mathrm{its}\:\mathrm{seems}\:\mathrm{that}\:\mathrm{f}\:\mathrm{is}\:\mathrm{not}\:\mathrm{derivable}\:\mathrm{at}\:\mathrm{x}_{\mathrm{0}} =\mathrm{1} \\ $$$$\mathrm{but}\:\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{1}^{+} } \:\:\mathrm{f}^{'} \left(\mathrm{x}\right)\:=\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{1}^{+} } \:\:\frac{−\mathrm{2}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }=−\mathrm{1}\:\mathrm{and} \\ $$$$\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{1}^{−} } \:\:\mathrm{f}^{'} \left(\mathrm{x}\right)=\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{1}^{−} } \:\:\frac{\mathrm{2}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:=\mathrm{1} \\ $$$$ \\ $$
