x^4 −⌊5x^2 ⌋+4=0


Question and Answers Forum

All Questions Topic List
Others Questions
Previous in All Question Next in All Question
Previous in Others Next in Others
Question Number 117620 by Dwaipayan Shikari last updated on 12/Oct/20

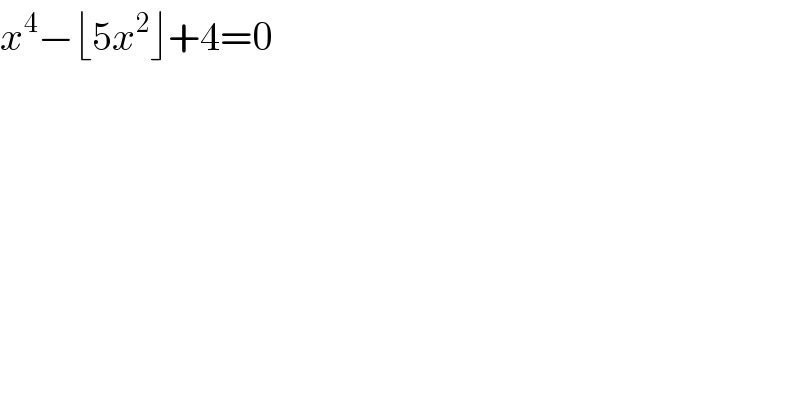
$x^{4} - ⌊ 5 x^{2} ⌋ + 4 = 0$
	Answered by mr W last updated on 12/Oct/20

	$x^{4} = ⌊ 5 x^{2} ⌋ - 4 \in Z$ $\Rightarrow x \in Z$ $\Rightarrow 5 x^{2} \in Z$ $\Rightarrow x^{4} - 5 x^{2} + 4 = 0$ $\Rightarrow (x^{2} - 1) (x^{2} - 4) = 0$ $\Rightarrow x = \pm 1, \pm 2$
	Commented by floor(10²Eta[1]) last updated on 12/Oct/20

	$missing the solutions \pm^{4} \sqrt{14} and \pm^{4} \sqrt{15}$
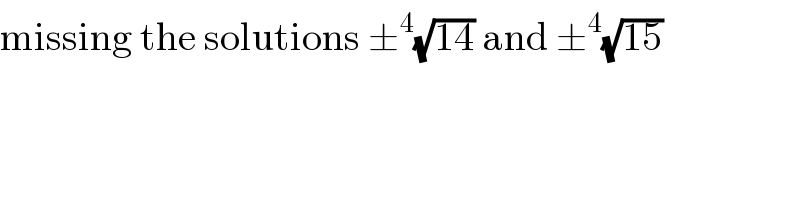
	Commented by mr W last updated on 13/Oct/20

	$y e s, y o u a r e r i g h t!$
	Answered by AbduraufKodiriy last updated on 12/Oct/20
	$x^2 =t t^2 −⌊5t⌋+4=0 ⌊5t⌋=t^2 +4 ∈ Z {5t}=−(t^2 −5t+4) 0≤−(t^2 −5t+4)<1 ⇒ t∈[1; ((5−(√5))/2))∪(((5+(√5))/2); 4] Thus, (t^2 +4)∈[5; ((23−5(√5))/2))∪(((23+5(√5))/2); 20] approximately: (t^2 +4)∈[5; 5.9)∪(17.09; 20] So, 1) t^2 +4=5 ⇒ t=1 2) t^2 +4=18 ⇒ t=(√(14)); 3) t^2 +4=19 ⇒ t=(√(15)); 4) t^2 +4=20 ⇒ t=4 Answer: x_(1,2) =±1, x_(3,4) =±((14))^(1/4) , x_(5,6) =±((15))^(1/4) , x_(7,8) =±2$
	$x^{2} = t$ $t^{2} - ⌊ 5 t ⌋ + 4 = 0$ $⌊ 5 t ⌋ = t^{2} + 4 \in Z$ ${5 t} = - (t^{2} - 5 t + 4)$ $0 ⩽ - (t^{2} - 5 t + 4) < 1 \Rightarrow t \in [1; \frac{5 - \sqrt{5}}{2}) \cup (\frac{5 + \sqrt{5}}{2}; 4]$ $T h u s, (t^{2} + 4) \in [5; \frac{23 - 5 \sqrt{5}}{2}) \cup (\frac{23 + 5 \sqrt{5}}{2}; 20]$ $a p p r o x i m a t e l y : (t^{2} + 4) \in [5; 5.9) \cup (17.09; 20]$ $S o, 1) t^{2} + 4 = 5 \Rightarrow t = 1 2) t^{2} + 4 = 18 \Rightarrow t = \sqrt{14};$ $3) t^{2} + 4 = 19 \Rightarrow t = \sqrt{15}; 4) t^{2} + 4 = 20 \Rightarrow t = 4$ $A n s w e r : x_{1, 2} = \pm 1, x_{3, 4} = \pm \sqrt[4]{14}, x_{5, 6} = \pm \sqrt[4]{15}, x_{7, 8} = \pm 2$
	Answered by floor(10²Eta[1]) last updated on 12/Oct/20
	$x^4 −⌊5x^2 ⌋=−4∈Z but ⌊5x^2 ⌋∈Z⇒x^4 ∈N ⇒x∈Z or x=±^4 (√a), a∈N ⌊5x^2 ⌋=n⇒5x^2 =n+θ, n∈Z, 0≤θ<1 x^2 =((n+θ)/5) x^4 =((n^2 +2nθ+θ^2 )/(25)) ((n^2 +2nθ+θ^2 )/(25))−n+4=0 n^2 +(2θ−25)n+100+θ^2 =0 n=((25−2θ±(√(225−100θ)))/2)∈Z since 0≤θ<1 so: I case: 15≥(√(225−100θ))>11 ⇒40≥40−2θ≥25−2θ+(√(225−100θ))>36−2θ>34 ⇒20≥n>17∴n∈{18,19,20} II case: 8<10−2θ≤25−2θ−(√(225−100θ))<14−2θ≤14 4<n<7⇒n∈{5,6} ⇒n=5 x^2 =((5+θ)/5)=1+(θ/5)⇒1≤x^2 <(6/5)=1.2 x=±1 ⇒n=6(no sol.) ⇒n=18 x^2 =((18+θ)/5)⇒3.6≤x^2 <3.8⇒x∉N x^2 =(√a), a∈N⇒3.6^2 ≤a<3.8^2 ⇒a∈{13,14} ⇒x=±^4 (√(14)) ⇒n=19 x^2 =((19+θ)/5)⇒3.8≤x^2 <4⇒x∉N x^2 =(√a) ⇒3.8^2 ≤a<16⇒a=15 ⇒x=±^4 (√(15)) n=20 x^2 =((20+θ)/5)⇒4≤x^2 <5⇒x=±2 all the solutions are: x={±1, ±^4 (√(14)), ±^4 (√(15)), ±2}$
	$x^{4} - ⌊ {5 x}^{2} ⌋ = - 4 \in Z$ $but ⌊ {5 x}^{2} ⌋ \in Z \Rightarrow x^{4} \in N$ $\Rightarrow x \in Z or x = \pm^{4} \sqrt{a}, a \in N$ $⌊ {5 x}^{2} ⌋ = n \Rightarrow {5 x}^{2} = n + θ, n \in Z, 0 ⩽ θ < 1$ $x^{2} = \frac{n + θ}{5}$ $x^{4} = \frac{n^{2} + 2 n θ + θ^{2}}{25}$ $\frac{n^{2} + 2 n θ + θ^{2}}{25} - n + 4 = 0$ $n^{2} + (2 θ - 25) n + 100 + θ^{2} = 0$ $n = \frac{25 - 2 θ \pm \sqrt{225 - 100 θ}}{2} \in Z$ $since 0 ⩽ θ < 1 so :$ $I case :$ $15 ⩾ \sqrt{225 - 100 θ} > 11$ $\Rightarrow 40 ⩾ 40 - 2 θ ⩾ 25 - 2 θ + \sqrt{225 - 100 θ} > 36 - 2 θ > 34$ $\Rightarrow 20 ⩾ n > 17 ∴ n \in {18, 19, 20}$ $II case :$ $8 < 10 - 2 θ ⩽ 25 - 2 θ - \sqrt{225 - 100 θ} < 14 - 2 θ ⩽ 14$ $4 < n < 7 \Rightarrow n \in {5, 6}$ $\Rightarrow n = 5$ $x^{2} = \frac{5 + θ}{5} = 1 + \frac{θ}{5} \Rightarrow 1 ⩽ x^{2} < \frac{6}{5} = 1.2$ $x = \pm 1$ $\Rightarrow n = 6 (no sol .)$ $\Rightarrow n = 18$ $x^{2} = \frac{18 + θ}{5} \Rightarrow 3.6 ⩽ x^{2} < 3.8 \Rightarrow x \notin N$ $x^{2} = \sqrt{a}, a \in N \Rightarrow 3 . 6^{2} ⩽ a < 3 . 8^{2} \Rightarrow a \in {13, 14}$ $\Rightarrow x = \pm^{4} \sqrt{14}$ $\Rightarrow n = 19$ $x^{2} = \frac{19 + θ}{5} \Rightarrow 3.8 ⩽ x^{2} < 4 \Rightarrow x \notin N$ $x^{2} = \sqrt{a} \Rightarrow 3 . 8^{2} ⩽ a < 16 \Rightarrow a = 15$ $\Rightarrow x = \pm^{4} \sqrt{15}$ $n = 20$ $x^{2} = \frac{20 + θ}{5} \Rightarrow 4 ⩽ x^{2} < 5 \Rightarrow x = \pm 2$ $all the solutions are :$ $x = {\pm 1, \pm^{4} \sqrt{14}, \pm^{4} \sqrt{15}, \pm 2}$
	Commented by Dwaipayan Shikari last updated on 12/Oct/20

	$T h a n k i n g a l l o f y o u$
	Answered by MJS_new last updated on 12/Oct/20

	$let x = t^{1 / 4}$ $t - ⌊ 5 \sqrt{t} ⌋ + 4 = 0$ $n ⩽ 5 \sqrt{t} < n + 1 \Rightarrow \frac{n^{2}}{25} ⩽ t < \frac{{(n + 1)}^{2}}{25}$ $let t = \frac{{(n + α)}^{2}}{25} with 0 ⩽ α < 1 \Rightarrow ⌊ 5 \sqrt{t} ⌋ = n$ $\frac{{(n + α)}^{2}}{25} + - n + 4 = 0$ $n^{2} + (2 α - 25) n + α^{2} + 100 = 0$ $n = \frac{25}{2} - α \pm \frac{5}{2} \sqrt{9 - 4 α}$ $0 ⩽ α < 1 \Rightarrow 5 ⩽ n_{-} < \frac{23 - 5 \sqrt{5}}{2} \approx 5.9 \land \frac{23 + 5 \sqrt{5}}{2} \approx 17.09 < n_{+} ⩽ 20$ $n \in N \Rightarrow$ $α = 0 \land n_{-} = 5$ $α = 0 \land n_{+} = 20$ $α = - 19 + 5 \sqrt{15} \land n_{+} = 19$ $α = - 18 + 5 \sqrt{14} \land n_{+} = 18$ $\Rightarrow$ $t = 1 \Rightarrow x = \pm 1$ $t = 16 \Rightarrow x = \pm 2$ $t = 15 \Rightarrow x = \pm 15^{1 / 4}$ $t = 14 \Rightarrow x = \pm 14^{1 / 4}$
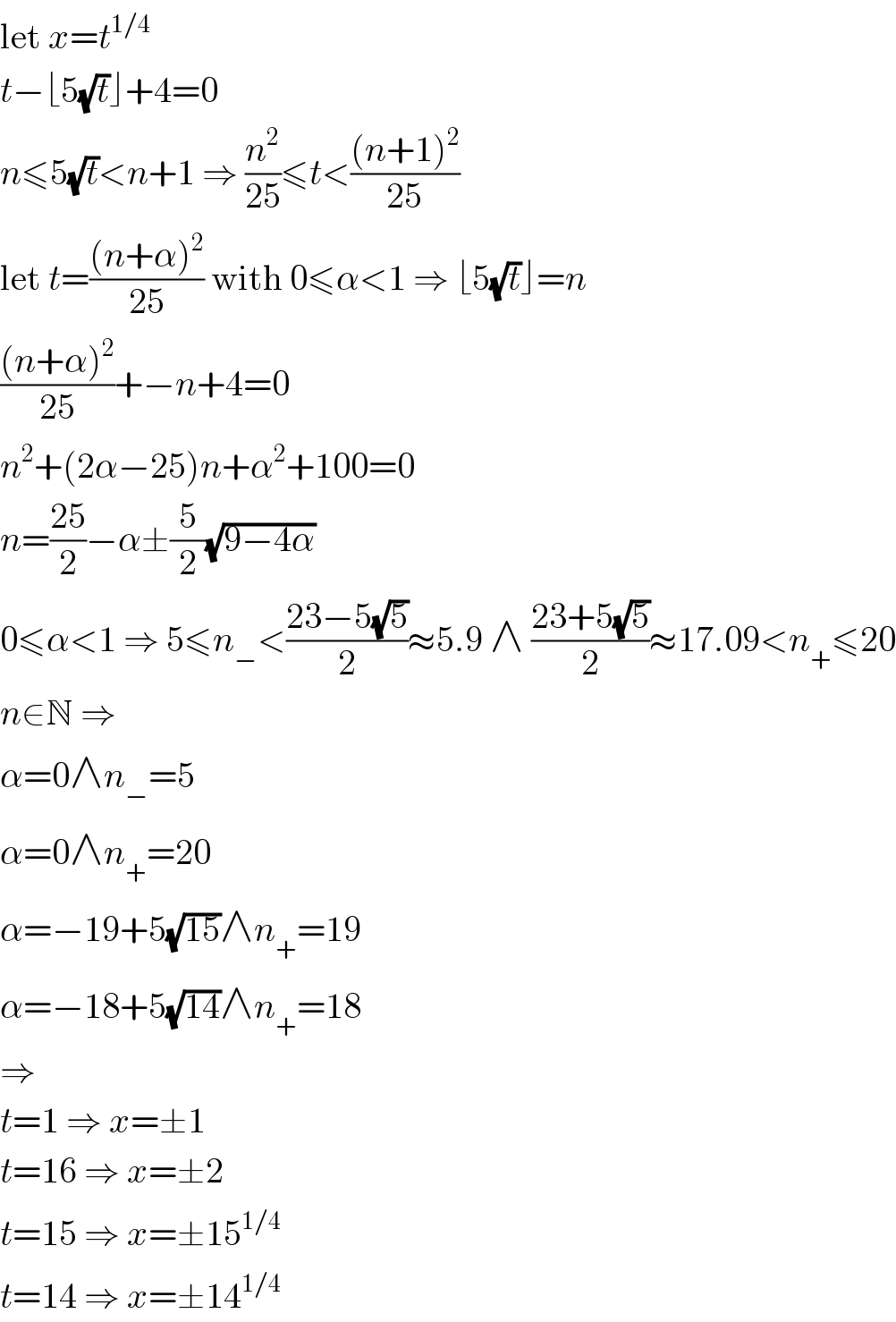
	Commented by MJS_new last updated on 12/Oct/20

	$tried to find a shorter path . . .$
	Answered by 1549442205PVT last updated on 13/Oct/20
	$x^4 −⌊5x^2 ⌋+4=0(1).Put x^4 +4=t(t≥4) ⇒x^2 =(√(t−4)).Then(1)⇔[5(√(t−4))]=t(∗) By the definition of function [x]we have 0≤5(√(t−4))−t<1 ⇔ { ((5(√(t−4))≥t(1))),((5(√(t−4))<t+1(2))) :}(∗∗) (1)⇔25(t−4)≥t^2 ⇔t^2 −25t+100≤0 ⇔(t−5)(t−20)≤0⇔5≤t≤20.Since t≥4,so by(∗)⇒t∈N⇒t∈{5,6,...,20}(3) (2)⇔25(t−4)<t^2 +2t+1 ⇔t^2 −23t+101>0⇔ t∈(−∞,((23−5(√5))/2))∪(((23+5(√5))/2),+∞) 5.9≈((23−5(√5))/2) and ((23+5(√5))/2)≈17.09 ⇒t∈{4,5}∪{18,19,20,21,...}(4) Combining (3)(4)we get t∈{5,18,19,20}are roots of the system of the inequalities (∗∗) i)t=5⇔x^4 +4=5⇔x^4 =1⇔x=±1 ii)t=18⇒x^4 +4=18⇔x=±^4 (√(14)) iii)t=19⇒x^4 +4=19⇔x=±^4 (√(15)) iv)t=20⇒x^4 +4=20⇔x=±2 Thus,roots of the system (∗∗) are x∈{±1;±2,±^4 (√(14)), ±^4 (√(15))}that is answer of our problem.$
	$x^{4} - ⌊ 5 x^{2} ⌋ + 4 = 0 (1) . Put x^{4} + 4 = t (t ⩾ 4)$ $\Rightarrow x^{2} = \sqrt{t - 4} . Then (1) \Leftrightarrow [5 \sqrt{t - 4}] = t ()$ $By the definition of function [x] we$ $have 0 ⩽ 5 \sqrt{t - 4} - t < 1$ $\Leftrightarrow {\begin{cases} 5 \sqrt{t - 4} ⩾ t (1) \\ 5 \sqrt{t - 4} < t + 1 (2) \end{cases} ( )$ $(1) \Leftrightarrow 25 (t - 4) ⩾ t^{2} \Leftrightarrow t^{2} - 25 t + 100 ⩽ 0$ $\Leftrightarrow (t - 5) (t - 20) ⩽ 0 \Leftrightarrow 5 ⩽ t ⩽ 20 . Since$ $t ⩾ 4, so by () \Rightarrow t \in N \Rightarrow t \in {5, 6, . . ., 20} (3)$ $(2) \Leftrightarrow 25 (t - 4) < t^{2} + 2 t + 1$ $\Leftrightarrow t^{2} - 23 t + 101 > 0 \Leftrightarrow$ $t \in (- \infty, \frac{23 - 5 \sqrt{5}}{2}) \cup (\frac{23 + 5 \sqrt{5}}{2}, + \infty)$ $5.9 \approx \frac{23 - 5 \sqrt{5}}{2} and \frac{23 + 5 \sqrt{5}}{2} \approx 17.09$ $\Rightarrow t \in {4, 5} \cup {18, 19, 20, 21, . . .} (4)$ $Combining (3) (4) we get$ $t \in {5, 18, 19, 20} are roots of the$ $system of the inequalities (* )$ $i) t = 5 \Leftrightarrow x^{4} + 4 = 5 \Leftrightarrow x^{4} = 1 \Leftrightarrow x = \pm 1$ $ii) t = 18 \Rightarrow x^{4} + 4 = 18 \Leftrightarrow x = \pm^{4} \sqrt{14}$ $iii) t = 19 \Rightarrow x^{4} + 4 = 19 \Leftrightarrow x = \pm^{4} \sqrt{15}$ $iv) t = 20 \Rightarrow x^{4} + 4 = 20 \Leftrightarrow x = \pm 2$ $Thus, roots of the system ( *) are$ $x \in {\pm 1; \pm 2, \pm^{4} \sqrt{14}, \pm^{4} \sqrt{15}} that is$ $answer of our problem .$
	Commented by floor(10²Eta[1]) last updated on 13/Oct/20

	$\pm^{4} \sqrt{14} and \pm^{4} \sqrt{15} are solutions too$
	Commented by 1549442205PVT last updated on 13/Oct/20

	$I mistaked and corrected . Thank sir$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum