Solution (d^2 y/dx^2 ) + 3(dy/dx) − 4y = x^2


Question and Answers Forum

All Questions Topic List
Differential Equation Questions
Previous in All Question Next in All Question
Previous in Differential Equation Next in Differential Equation
Question Number 117728 by syamil last updated on 13/Oct/20

$S o l u t i o n \frac{d^{2} y}{{d x}^{2}} + 3 \frac{d y}{d x} - 4 y = x^{2}$
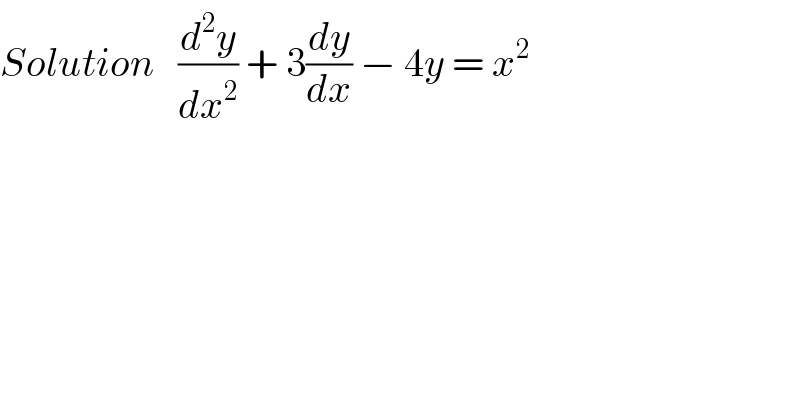
	Answered by TANMAY PANACEA last updated on 13/Oct/20

	$y = e^{m x}$ $(m^{2} + 3 m - 4) e^{m x} = 0 e^{m x} \neq 0$ $m^{2} + 4 m - m - 4 = 0$ $(m + 4) (m - 1) = 0 \to m = 1, - 4$ $C . F = C_{1} e^{x} + C_{2} e^{- 4 x}$ $P . I = \frac{x^{2}}{D^{2} + 3 D - 4} = \frac{x^{2}}{(D + 4) (D - 1)} = \frac{1}{5} (\frac{1}{D - 1} - \frac{1}{D + 4}) x^{2}$ $y = \frac{1}{5} [\frac{- 1}{1 - D} - \frac{1}{4 (1 + \frac{D}{4})}] x^{2}$ $y = \frac{- 1}{5} [{(1 - D)}^{- 1} + \frac{1}{4} {(1 + \frac{D}{4})}^{- 1}] x^{2}$ $y = \frac{- 1}{5} [(1 + D + D^{2} + D^{3} + . . .) + \frac{1}{4} (1 - \frac{D}{4} + \frac{D^{2}}{16} - \frac{D^{3}}{64})] x^{2}$ $y = \frac{- 1}{5} [(x^{2} + 2 x + 2) + \frac{1}{4} (x^{2} - \frac{2 x}{4} + \frac{2}{16})]$ $= \frac{- 1}{5} [\frac{5 x^{2}}{4} + 2 x (1 - \frac{1}{16}) + 2 + \frac{2}{64}]$ $= \frac{- 1}{5} [\frac{5 x^{2}}{4} + \frac{15 x}{8} + \frac{130}{64}]$ $y = C_{1} e^{x} + C_{2} e^{- 4 x} - \frac{1}{5} (\frac{5 x^{2}}{4} + \frac{15 x}{8} + \frac{65}{32})$
	Answered by john santu last updated on 14/Oct/20
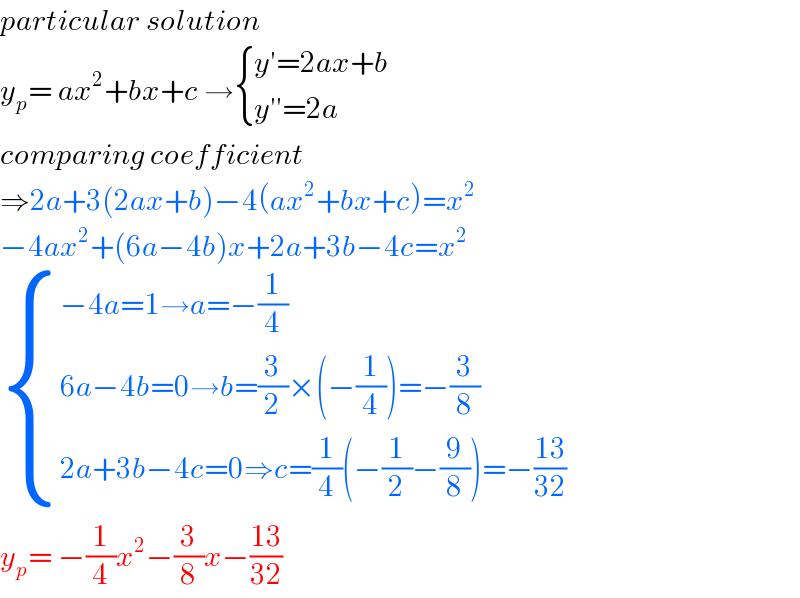
	$particular solution y_p = ax^2 +bx+c → { ((y′=2ax+b)),((y′′=2a)) :} comparing coefficient ⇒2a+3(2ax+b)−4(ax^2 +bx+c)=x^2 −4ax^2 +(6a−4b)x+2a+3b−4c=x^2 { ((−4a=1→a=−(1/4))),((6a−4b=0→b=(3/2)×(−(1/4))=−(3/8))),((2a+3b−4c=0⇒c=(1/4)(−(1/2)−(9/8))=−((13)/(32)))) :} y_p = −(1/4)x^2 −(3/8)x−((13)/(32))$
	$p a r t i c u l a r s o l u t i o n$ $y_{p} = {a x}^{2} + b x + c \to {\begin{cases} y^{'} = 2 a x + b \\ y^{″} = 2 a \end{cases}$ $c o m p a r i n g c o e f f i c i e n t$ $\Rightarrow 2 a + 3 (2 a x + b) - 4 ({a x}^{2} + b x + c) = x^{2}$ $- 4 {a x}^{2} + (6 a - 4 b) x + 2 a + 3 b - 4 c = x^{2}$ ${\begin{cases} - 4 a = 1 \to a = - \frac{1}{4} \\ 6 a - 4 b = 0 \to b = \frac{3}{2} \times (- \frac{1}{4}) = - \frac{3}{8} \\ 2 a + 3 b - 4 c = 0 \Rightarrow c = \frac{1}{4} (- \frac{1}{2} - \frac{9}{8}) = - \frac{13}{32} \end{cases}$ $y_{p} = - \frac{1}{4} x^{2} - \frac{3}{8} x - \frac{13}{32}$
	Answered by Bird last updated on 14/Oct/20
	$y^(′′) +3y^′ −4y =x^2 h→r^2 +3r−4=0 →Δ=9+16=25 ⇒r_1 =((−3+5)/2)=1 snd r_2 =((−3−5)/2)=−4 ⇒y_h =ae^x +b e^(−4x) =au_1 +bu_2 W(u_1 ,u_2 )= determinant (((e^x e^(−4x) )),((e^x −4e^(−4x) ))) =−4e^(−3x) −e^(−3x) =−5e^(−3x) ≠0 W_1 = determinant (((0 e^(−4x) )),((x^2 −4e^(−4x) )))=−x^2 e^(−4x) W_2 = determinant (((e^x 0)),((e^x x^2 )))=x^2 e^x v_1 =∫ (w_1 /w)dx =−∫ ((x^2 e^(−4x) )/(−5e^(−3x) ))dx =(1/5)∫x^2 e^(−x) dx =(1/5){−x^2 e^(−x) +∫ 2x e^(−x) } =(1/5){−x^2 e^(−x) +2(−xe^(−x) +∫e^(−x) dx)} =(1/5){−x^2 e^(−x) −2x e^(−x) −2e^(−x) } =(1/5)(−x^2 −2x−2)e^(−x) v_2 =∫ (w_2 /w)dx =∫ ((x^2 e^x )/(−5e^(−3x) ))dx =−(1/5)∫ x^2 e^(5x) dx =−(1/5){ (x^2 /5)e^(5x) −∫ ((2x)/5)e^(5x) dx} =−(1/5){ (x^2 /5)e^(5x) −(2/5)((x/5)e^(5x) −∫(1/5)e^(5x) dx)} =−(1/5){ ((x^2 e^(5x) )/5)−((2x)/(25))e^(5x) +(2/(125))e^(5x) } =−(1/5)((x^2 /5)−((2x)/(25))+(2/(125)))e^(5x) ⇒y_p =u_1 v_1 +u_2 v_2 =−(1/5)(x^2 +2x+2)−(1/5)((x^2 /5)−((2x)/(25))+(2/(25)))e^x general dolution is y =y_h +y_p$
	$y^{^{″}} + 3 y^{^{'}} - 4 y = x^{2}$ $h \to r^{2} + 3 r - 4 = 0 \to Δ = 9 + 16 = 25$ $\Rightarrow r_{1} = \frac{- 3 + 5}{2} = 1 s n d r_{2} = \frac{- 3 - 5}{2} = - 4$ $\Rightarrow y_{h} = {a e}^{x} + b e^{- 4 x} = {a u}_{1} + {b u}_{2}$ $W (u_{1}, u_{2}) = \| \begin{matrix} e^{x} e^{- 4 x} \\ e^{x} - 4 e^{- 4 x} \end{matrix} \|$ $= - 4 e^{- 3 x} - e^{- 3 x} = - 5 e^{- 3 x} \neq 0$ $W_{1} = \| \begin{matrix} 0 e^{- 4 x} \\ x^{2} - 4 e^{- 4 x} \end{matrix} \| = - x^{2} e^{- 4 x}$ $W_{2} = \| \begin{matrix} e^{x} 0 \\ e^{x} x^{2} \end{matrix} \| = x^{2} e^{x}$ $v_{1} = \int \frac{w_{1}}{w} d x = - \int \frac{x^{2} e^{- 4 x}}{- 5 e^{- 3 x}} d x$ $= \frac{1}{5} \int x^{2} e^{- x} d x$ $= \frac{1}{5} {- x^{2} e^{- x} + \int 2 x e^{- x}}$ $= \frac{1}{5} {- x^{2} e^{- x} + 2 (- {x e}^{- x} + \int e^{- x} d x)}$ $= \frac{1}{5} {- x^{2} e^{- x} - 2 x e^{- x} - 2 e^{- x}}$ $= \frac{1}{5} (- x^{2} - 2 x - 2) e^{- x}$ $v_{2} = \int \frac{w_{2}}{w} d x = \int \frac{x^{2} e^{x}}{- 5 e^{- 3 x}} d x$ $= - \frac{1}{5} \int x^{2} e^{5 x} d x$ $= - \frac{1}{5} {\frac{x^{2}}{5} e^{5 x} - \int \frac{2 x}{5} e^{5 x} d x}$ $= - \frac{1}{5} {\frac{x^{2}}{5} e^{5 x} - \frac{2}{5} (\frac{x}{5} e^{5 x} - \int \frac{1}{5} e^{5 x} d x)}$ $= - \frac{1}{5} {\frac{x^{2} e^{5 x}}{5} - \frac{2 x}{25} e^{5 x} + \frac{2}{125} e^{5 x}}$ $= - \frac{1}{5} (\frac{x^{2}}{5} - \frac{2 x}{25} + \frac{2}{125}) e^{5 x}$ $\Rightarrow y_{p} = u_{1} v_{1} + u_{2} v_{2}$ $= - \frac{1}{5} (x^{2} + 2 x + 2) - \frac{1}{5} (\frac{x^{2}}{5} - \frac{2 x}{25} + \frac{2}{25}) e^{x}$ $g e n e r a l d o l u t i o n i s$ $y = y_{h} + y_{p}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum