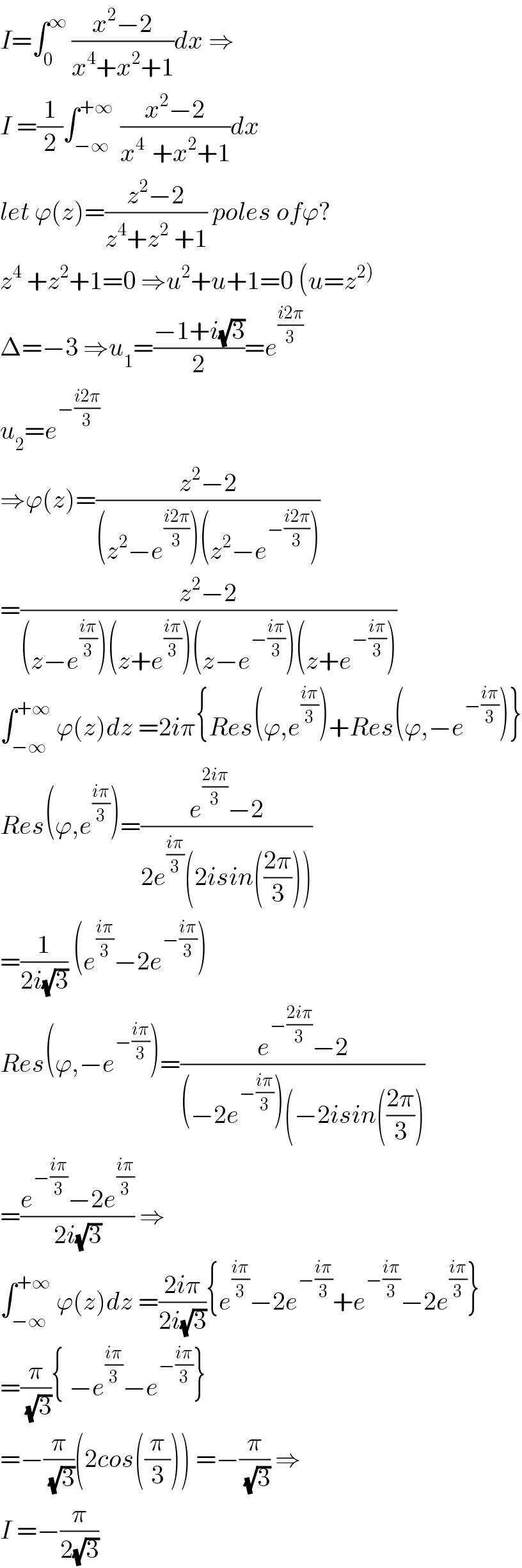Question and Answers Forum
Question Number 118030 by mathmax by abdo last updated on 14/Oct/20
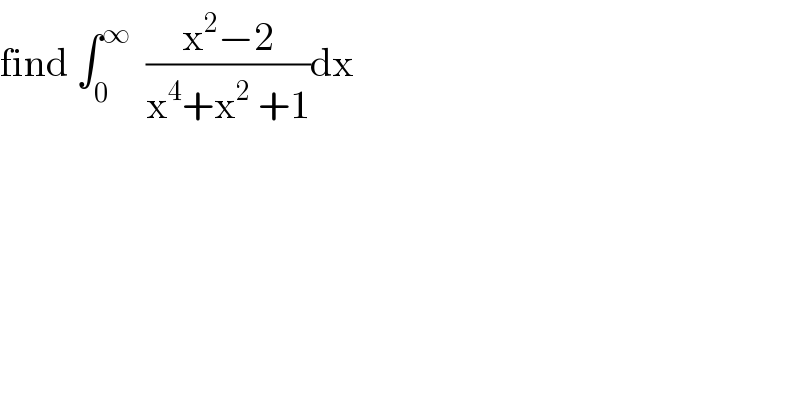
Commented by Ar Brandon last updated on 14/Oct/20
Answered by Ar Brandon last updated on 14/Oct/20
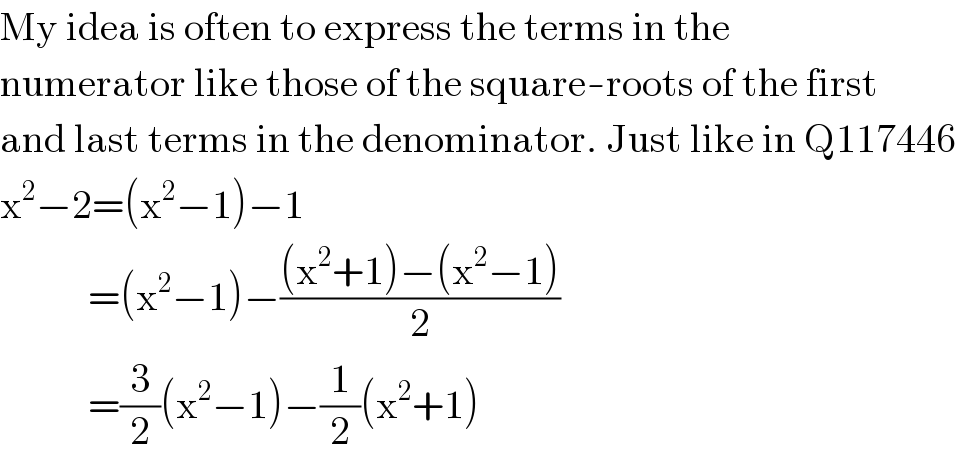
![I=∫_0 ^∞ ((x^2 −2)/(x^4 +x^2 +1))dx=(3/2)∫_0 ^∞ (((x^2 −1))/(x^4 +x^2 +1))dx−(1/2)∫_0 ^∞ (((x^2 +1))/(x^4 +x^2 +1))dx =(3/2)∫_0 ^∞ ((1−(1/x^2 ))/(x^2 +1+(1/x^2 )))dx−(1/2)∫_0 ^∞ ((1+(1/x^2 ))/(x^2 +1+(1/x^2 )))dx =(3/2)∫_0 ^∞ ((1−(1/x^2 ))/((x+(1/x))^2 −1))dx−(1/2)∫_0 ^∞ ((1+(1/x^2 ))/((x−(1/x))^2 +3))dx =(3/2)∫_(+∞) ^(+∞) (du/(u^2 −1))−(1/2)∫_(−∞) ^(+∞) (dv/(v^2 +3))=−(1/2)∫_(−∞) ^(+∞) (dv/(v^2 +3)) =−[(1/(2(√3)))Arctan((v/( (√3))))]_(−∞) ^(+∞) =−(1/(2(√3)))((π/2)+(π/2))=−(π/(2(√3)))](Q118039.png)
Commented by Lordose last updated on 14/Oct/20

Commented by Ar Brandon last updated on 14/Oct/20
Merci. J'suis ravi !
Commented by Ar Brandon last updated on 14/Oct/20
S'il vous plaît, pouvez-vous jeter un oeil sur la Question 117972 ? Il semble avoir un problème avec votre réponse.
Commented by Lordose last updated on 15/Oct/20
Je pense que la meilleure façon de résoudre la question 117927 est de dessiner les cartes.��
Commented by Ar Brandon last updated on 15/Oct/20
Je n'arrive pas à le faire malheureusement
Commented by Lordose last updated on 15/Oct/20
D'accord. Je suis un peu occupé maintenant. quand je serai libre, je résoudrai.
Commented by Ar Brandon last updated on 15/Oct/20
D'accord, merci
Answered by Bird last updated on 15/Oct/20