
Question and Answers Forum
Question Number 118566 by benjo_mathlover last updated on 18/Oct/20

Commented by benjo_mathlover last updated on 18/Oct/20

Commented by Dwaipayan Shikari last updated on 18/Oct/20
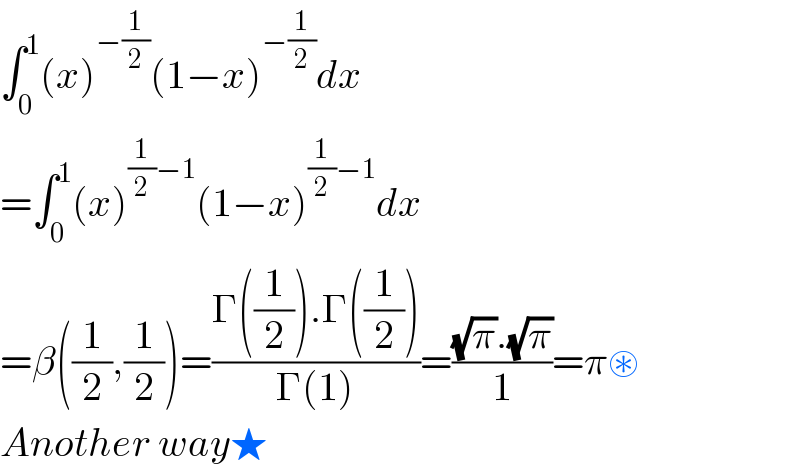
Answered by TANMAY PANACEA last updated on 18/Oct/20
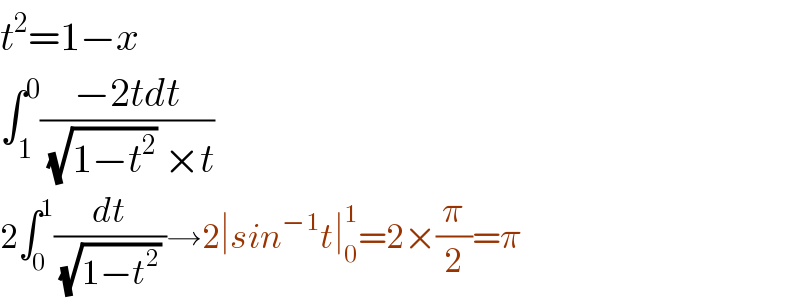
Answered by Dwaipayan Shikari last updated on 18/Oct/20
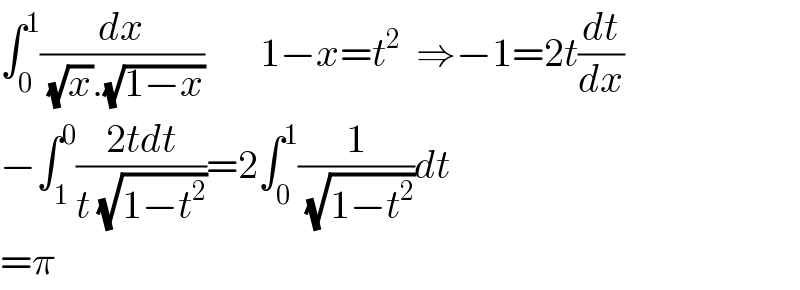
Answered by MJS_new last updated on 18/Oct/20
![∫_0 ^1 (dx/( (√x)(√(1−x))))= [t=((√(1−x))/( (√x))) → dx=−2(√x^3 )(√(1−x))dt] =−2∫_(+∞) ^0 (dt/(t^2 +1))=2∫_0 ^(+∞) (dt/(t^2 +1))= =2[arctan t]_0 ^(+∞) =π](Q118603.png)
Answered by 1549442205PVT last updated on 18/Oct/20
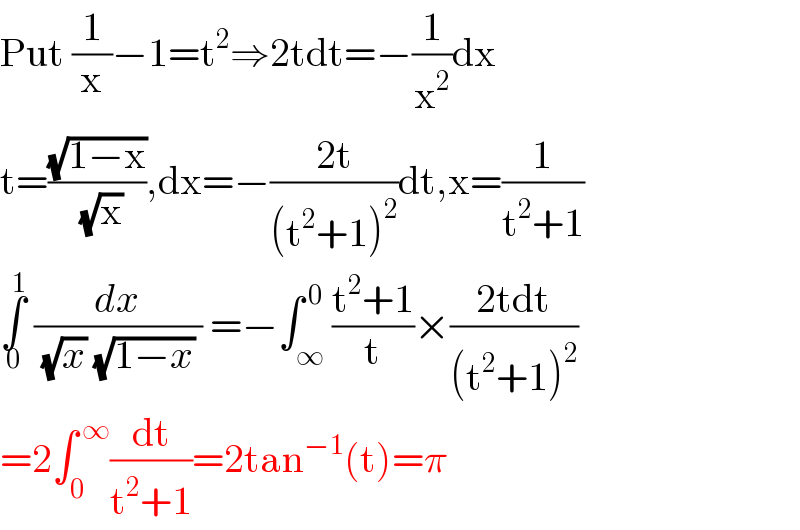
Commented by MJS_new last updated on 18/Oct/20
Commented by MJS_new last updated on 18/Oct/20
Answered by Bird last updated on 18/Oct/20
![I=∫_0 ^1 (dx/( (√x)(√(1−x)))) we do the chang. (√x)=t ⇒I =∫_0 ^1 ((2tdt)/(t(√(1−t^2 )))) =2∫_0 ^1 (dt/( (√(1−t^2 )))) =2[arcsint]_0 ^1 =2×(π/2)=π](Q118632.png)
Answered by mindispower last updated on 18/Oct/20
![=2∫_0 ^1 .(1/(2(√x))).(1/( (√(1−((√x))^2 ))))=2[arcsin((√x))]_0 ^1 =2.(π/2)=1π](Q118639.png)
