Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 118690 by Algoritm last updated on 19/Oct/20

Answered by benjo_mathlover last updated on 19/Oct/20

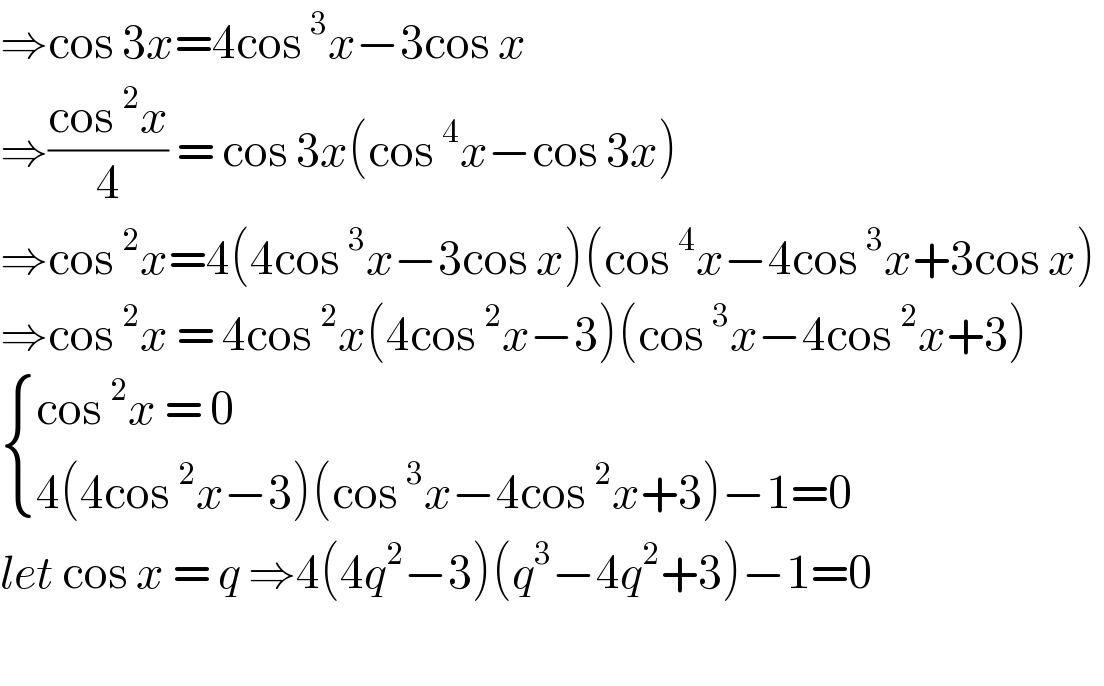
⇒cos 3x=4cos^3 x−3cos x ⇒((cos^2 x)/4) = cos 3x(cos^4 x−cos 3x) ⇒cos^2 x=4(4cos^3 x−3cos x)(cos^4 x−4cos^3 x+3cos x) ⇒cos^2 x = 4cos^2 x(4cos^2 x−3)(cos^3 x−4cos^2 x+3) { ((cos^2 x = 0)),((4(4cos^2 x−3)(cos^3 x−4cos^2 x+3)−1=0)) :} let cos x = q ⇒4(4q^2 −3)(q^3 −4q^2 +3)−1=0

$$\Rightarrow\mathrm{cos}\:\mathrm{3}{x}=\mathrm{4cos}\:^{\mathrm{3}} {x}−\mathrm{3cos}\:{x} \\ $$$$\Rightarrow\frac{\mathrm{cos}\:^{\mathrm{2}} {x}}{\mathrm{4}}\:=\:\mathrm{cos}\:\mathrm{3}{x}\left(\mathrm{cos}\:^{\mathrm{4}} {x}−\mathrm{cos}\:\mathrm{3}{x}\right) \\ $$$$\Rightarrow\mathrm{cos}\:^{\mathrm{2}} {x}=\mathrm{4}\left(\mathrm{4cos}\:^{\mathrm{3}} {x}−\mathrm{3cos}\:{x}\right)\left(\mathrm{cos}\:^{\mathrm{4}} {x}−\mathrm{4cos}\:^{\mathrm{3}} {x}+\mathrm{3cos}\:{x}\right)\: \\ $$$$\Rightarrow\mathrm{cos}\:^{\mathrm{2}} {x}\:=\:\mathrm{4cos}\:^{\mathrm{2}} {x}\left(\mathrm{4cos}\:^{\mathrm{2}} {x}−\mathrm{3}\right)\left(\mathrm{cos}\:^{\mathrm{3}} {x}−\mathrm{4cos}\:^{\mathrm{2}} {x}+\mathrm{3}\right) \\ $$$$\begin{cases}{\mathrm{cos}\:^{\mathrm{2}} {x}\:=\:\mathrm{0}}\\{\mathrm{4}\left(\mathrm{4cos}\:^{\mathrm{2}} {x}−\mathrm{3}\right)\left(\mathrm{cos}\:^{\mathrm{3}} {x}−\mathrm{4cos}\:^{\mathrm{2}} {x}+\mathrm{3}\right)−\mathrm{1}=\mathrm{0}}\end{cases} \\ $$$${let}\:\mathrm{cos}\:{x}\:=\:{q}\:\Rightarrow\mathrm{4}\left(\mathrm{4}{q}^{\mathrm{2}} −\mathrm{3}\right)\left({q}^{\mathrm{3}} −\mathrm{4}{q}^{\mathrm{2}} +\mathrm{3}\right)−\mathrm{1}=\mathrm{0} \\ $$$$ \\ $$

Answered by 1549442205PVT last updated on 19/Oct/20

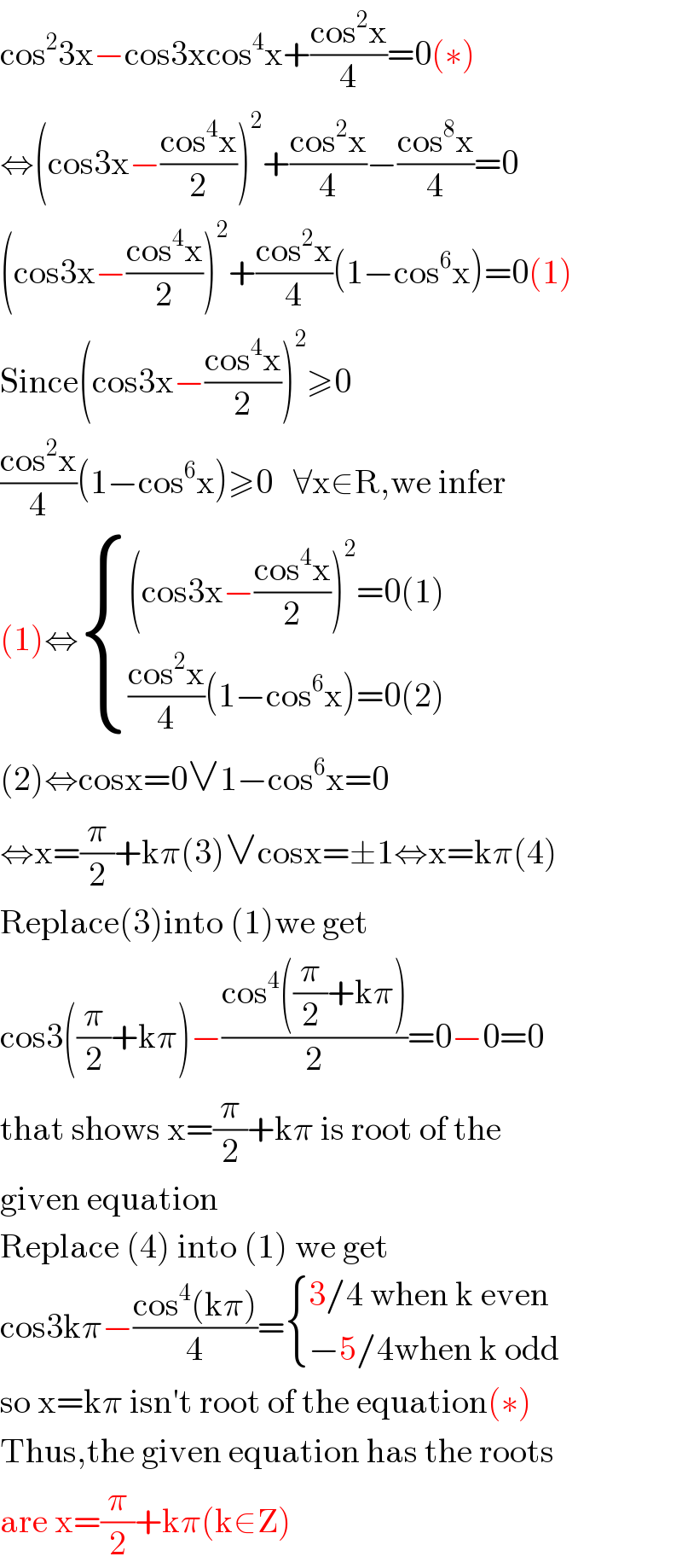
cos^2 3x−cos3xcos^4 x+((cos^2 x)/4)=0(∗) ⇔(cos3x−((cos^4 x)/2))^2 +((cos^2 x)/4)−((cos^8 x)/4)=0 (cos3x−((cos^4 x)/2))^2 +((cos^2 x)/4)(1−cos^6 x)=0(1) Since(cos3x−((cos^4 x)/2))^2 ≥0 ((cos^2 x)/4)(1−cos^6 x)≥0 ∀x∈R,we infer (1)⇔ { (((cos3x−((cos^4 x)/2))^2 =0(1))),((((cos^2 x)/4)(1−cos^6 x)=0(2))) :} (2)⇔cosx=0∨1−cos^6 x=0 ⇔x=(π/2)+kπ(3)∨cosx=±1⇔x=kπ(4) Replace(3)into (1)we get cos3((π/2)+kπ)−((cos^4 ((π/2)+kπ))/2)=0−0=0 that shows x=(π/2)+kπ is root of the given equation Replace (4) into (1) we get cos3kπ−((cos^4 (kπ))/4)= { ((3/4 when k even)),((−5/4when k odd)) :} so x=kπ isn′t root of the equation(∗) Thus,the given equation has the roots are x=(π/2)+kπ(k∈Z)

$$\mathrm{cos}^{\mathrm{2}} \mathrm{3x}−\mathrm{cos3xcos}^{\mathrm{4}} \mathrm{x}+\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{4}}=\mathrm{0}\left(\ast\right) \\ $$$$\Leftrightarrow\left(\mathrm{cos3x}−\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{4}}−\frac{\mathrm{cos}^{\mathrm{8}} \mathrm{x}}{\mathrm{4}}=\mathrm{0} \\ $$$$\left(\mathrm{cos3x}−\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{4}}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{6}} \mathrm{x}\right)=\mathrm{0}\left(\mathrm{1}\right) \\ $$$$\mathrm{Since}\left(\mathrm{cos3x}−\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} \geqslant\mathrm{0} \\ $$$$\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{4}}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{6}} \mathrm{x}\right)\geqslant\mathrm{0}\:\:\:\forall\mathrm{x}\in\mathrm{R},\mathrm{we}\:\mathrm{infer} \\ $$$$\left(\mathrm{1}\right)\Leftrightarrow\begin{cases}{\left(\mathrm{cos3x}−\frac{\mathrm{cos}^{\mathrm{4}} \mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} =\mathrm{0}\left(\mathrm{1}\right)}\\{\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}{\mathrm{4}}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{6}} \mathrm{x}\right)=\mathrm{0}\left(\mathrm{2}\right)}\end{cases} \\ $$$$\left(\mathrm{2}\right)\Leftrightarrow\mathrm{cosx}=\mathrm{0}\vee\mathrm{1}−\mathrm{cos}^{\mathrm{6}} \mathrm{x}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{x}=\frac{\pi}{\mathrm{2}}+\mathrm{k}\pi\left(\mathrm{3}\right)\vee\mathrm{cosx}=\pm\mathrm{1}\Leftrightarrow\mathrm{x}=\mathrm{k}\pi\left(\mathrm{4}\right) \\ $$$$\mathrm{Replace}\left(\mathrm{3}\right)\mathrm{into}\:\left(\mathrm{1}\right)\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{cos3}\left(\frac{\pi}{\mathrm{2}}+\mathrm{k}\pi\right)−\frac{\mathrm{cos}^{\mathrm{4}} \left(\frac{\pi}{\mathrm{2}}+\mathrm{k}\pi\right)}{\mathrm{2}}=\mathrm{0}−\mathrm{0}=\mathrm{0} \\ $$$$\mathrm{that}\:\mathrm{shows}\:\mathrm{x}=\frac{\pi}{\mathrm{2}}+\mathrm{k}\pi\:\mathrm{is}\:\mathrm{root}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{given}\:\mathrm{equation} \\ $$$$\mathrm{Replace}\:\left(\mathrm{4}\right)\:\mathrm{into}\:\left(\mathrm{1}\right)\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{cos3k}\pi−\frac{\mathrm{cos}^{\mathrm{4}} \left(\mathrm{k}\pi\right)}{\mathrm{4}}=\begin{cases}{\mathrm{3}/\mathrm{4}\:\mathrm{when}\:\mathrm{k}\:\mathrm{even}}\\{−\mathrm{5}/\mathrm{4when}\:\mathrm{k}\:\mathrm{odd}}\end{cases} \\ $$$$\mathrm{so}\:\mathrm{x}=\mathrm{k}\pi\:\mathrm{isn}'\mathrm{t}\:\mathrm{root}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\left(\ast\right) \\ $$$$\mathrm{Thus},\mathrm{the}\:\mathrm{given}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{the}\:\mathrm{roots} \\ $$$$\mathrm{are}\:\mathrm{x}=\frac{\pi}{\mathrm{2}}+\mathrm{k}\pi\left(\mathrm{k}\in\mathrm{Z}\right) \\ $$

Commented by bemath last updated on 19/Oct/20

it should be cos^2 3x−cos 3x cos^4 x+((cos^2 x)/4) = 0 sir

$${it}\:{should}\:{be}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{3}{x}−\mathrm{cos}\:\mathrm{3}{x}\:\mathrm{cos}\:^{\mathrm{4}} {x}+\frac{\mathrm{cos}\:^{\mathrm{2}} {x}}{\mathrm{4}}\:=\:\mathrm{0} \\ $$$${sir} \\ $$

Commented by 1549442205PVT last updated on 19/Oct/20

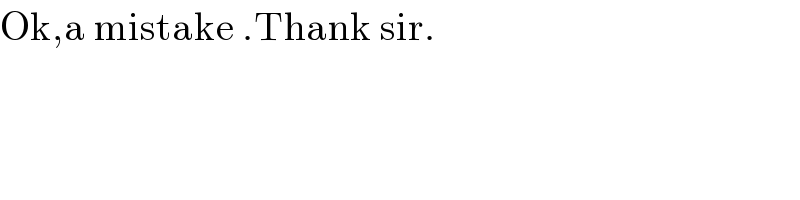
Ok,a mistake .Thank sir.

$$\mathrm{Ok},\mathrm{a}\:\mathrm{mistake}\:.\mathrm{Thank}\:\mathrm{sir}. \\ $$

Answered by MJS_new last updated on 19/Oct/20

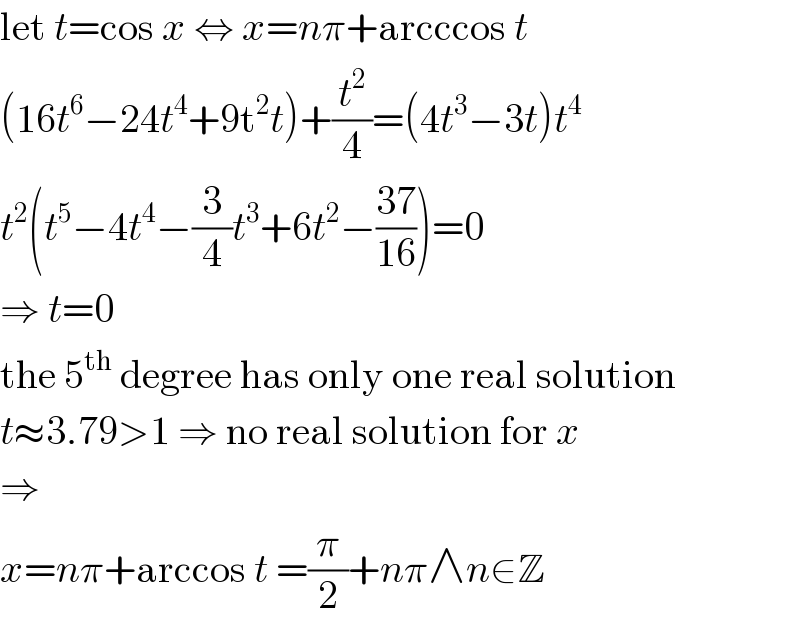
let t=cos x ⇔ x=nπ+arcccos t (16t^6 −24t^4 +9t^2 t)+(t^2 /4)=(4t^3 −3t)t^4 t^2 (t^5 −4t^4 −(3/4)t^3 +6t^2 −((37)/(16)))=0 ⇒ t=0 the 5^(th) degree has only one real solution t≈3.79>1 ⇒ no real solution for x ⇒ x=nπ+arccos t =(π/2)+nπ∧n∈Z

$$\mathrm{let}\:{t}=\mathrm{cos}\:{x}\:\Leftrightarrow\:{x}={n}\pi+\mathrm{arcccos}\:{t} \\ $$$$\left(\mathrm{16}{t}^{\mathrm{6}} −\mathrm{24}{t}^{\mathrm{4}} +\mathrm{9t}^{\mathrm{2}} {t}\right)+\frac{{t}^{\mathrm{2}} }{\mathrm{4}}=\left(\mathrm{4}{t}^{\mathrm{3}} −\mathrm{3}{t}\right){t}^{\mathrm{4}} \\ $$$${t}^{\mathrm{2}} \left({t}^{\mathrm{5}} −\mathrm{4}{t}^{\mathrm{4}} −\frac{\mathrm{3}}{\mathrm{4}}{t}^{\mathrm{3}} +\mathrm{6}{t}^{\mathrm{2}} −\frac{\mathrm{37}}{\mathrm{16}}\right)=\mathrm{0} \\ $$$$\Rightarrow\:{t}=\mathrm{0} \\ $$$$\mathrm{the}\:\mathrm{5}^{\mathrm{th}} \:\mathrm{degree}\:\mathrm{has}\:\mathrm{only}\:\mathrm{one}\:\mathrm{real}\:\mathrm{solution} \\ $$$${t}\approx\mathrm{3}.\mathrm{79}>\mathrm{1}\:\Rightarrow\:\mathrm{no}\:\mathrm{real}\:\mathrm{solution}\:\mathrm{for}\:{x} \\ $$$$\Rightarrow \\ $$$${x}={n}\pi+\mathrm{arccos}\:{t}\:=\frac{\pi}{\mathrm{2}}+{n}\pi\wedge{n}\in\mathbb{Z} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com