
Question and Answers Forum
Question Number 119335 by physicstutes last updated on 23/Oct/20

Answered by floor(10²Eta[1]) last updated on 23/Oct/20
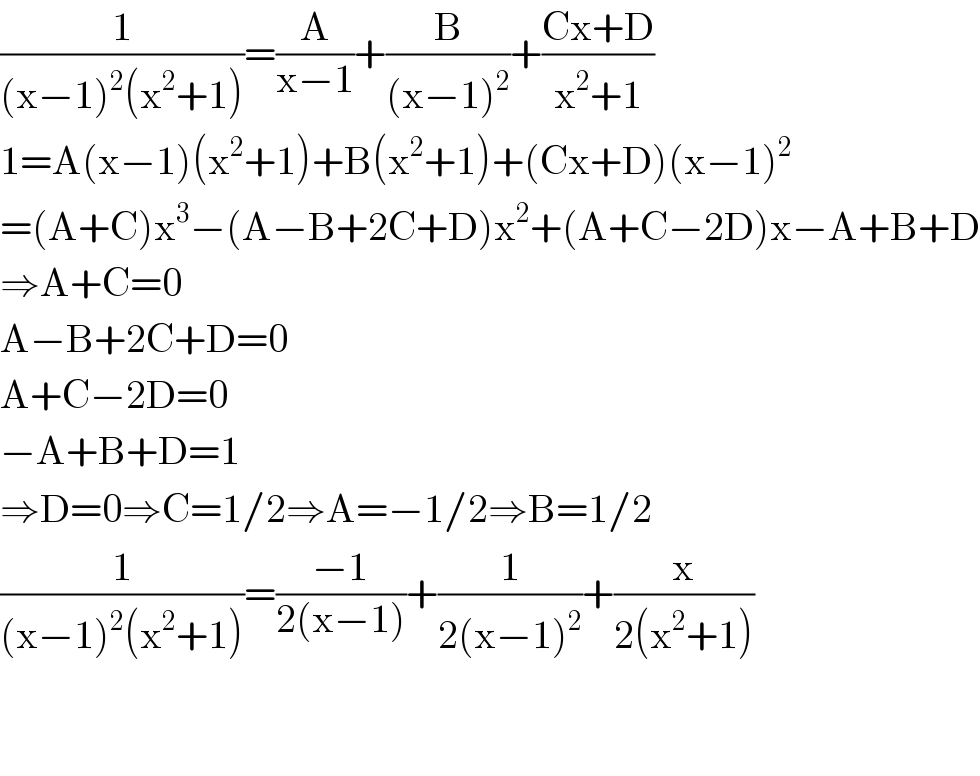
Answered by mathmax by abdo last updated on 24/Oct/20
![f(x)=(1/((x−1)^2 (x^2 +1))) ⇒f(x)=(a/(x−1))+(b/((x−1)^2 )) +((cx+d)/(x^2 +1)) b=(1/2) ,lim_(x→+∞) xf(x)=0 =a+c ⇒c=−a ⇒ f(x)=(a/(x−1)) +(1/(2(x−1)^2 )) +((−ax+d)/(x^2 +1)) f(0)=1 =−a+(1/2) +d ⇒−a+d=(1/2) ⇒a−d=−(1/2) f(2)=(1/5)=a +(1/2)+((−2a+d)/5) ⇒1=5a+(5/2)−2a+d ⇒ 3a+d=1−(5/2)=−(3/2) we have a=d−(1/2) ⇒3(d−(1/2))+d=−(3/2) ⇒4d=0 ⇒d=0 ⇒a=−(1/2) ⇒ f(x)=−(1/(2(x−1))) +(1/(2(x−1)^2 ))+(x/(2(x^2 +1))) so ∫_0 ^4 f(x)dx =−(1/2)∫_0 ^4 (dx/(x−1)) +(1/2)∫_0 ^4 (dx/((x−1)^2 )) +(1/4)∫_0 ^4 ((2x)/(x^2 +1))dx =−(1/2)[ln∣x−1∣]_0 ^4 −(1/2)[(1/(x−1))]_0 ^4 +(1/4)[ln(x^2 +1)]_0 ^4 =−(1/2)ln(3)−(1/2)((1/3)+1)+(1/4)ln(17) =−((ln3)/2)−(2/3) +((ln17)/4)](Q119423.png)
| ||
Question and Answers Forum | ||
Question Number 119335 by physicstutes last updated on 23/Oct/20 | ||
 | ||
Answered by floor(10²Eta[1]) last updated on 23/Oct/20 | ||
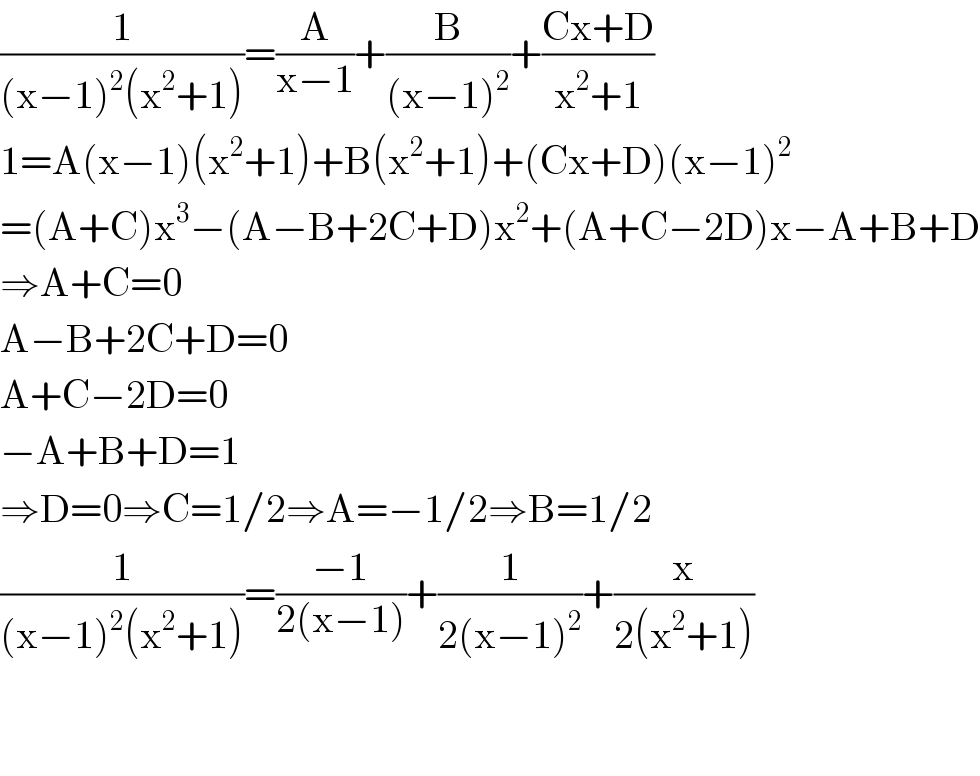 | ||
| ||
Answered by mathmax by abdo last updated on 24/Oct/20 | ||
![f(x)=(1/((x−1)^2 (x^2 +1))) ⇒f(x)=(a/(x−1))+(b/((x−1)^2 )) +((cx+d)/(x^2 +1)) b=(1/2) ,lim_(x→+∞) xf(x)=0 =a+c ⇒c=−a ⇒ f(x)=(a/(x−1)) +(1/(2(x−1)^2 )) +((−ax+d)/(x^2 +1)) f(0)=1 =−a+(1/2) +d ⇒−a+d=(1/2) ⇒a−d=−(1/2) f(2)=(1/5)=a +(1/2)+((−2a+d)/5) ⇒1=5a+(5/2)−2a+d ⇒ 3a+d=1−(5/2)=−(3/2) we have a=d−(1/2) ⇒3(d−(1/2))+d=−(3/2) ⇒4d=0 ⇒d=0 ⇒a=−(1/2) ⇒ f(x)=−(1/(2(x−1))) +(1/(2(x−1)^2 ))+(x/(2(x^2 +1))) so ∫_0 ^4 f(x)dx =−(1/2)∫_0 ^4 (dx/(x−1)) +(1/2)∫_0 ^4 (dx/((x−1)^2 )) +(1/4)∫_0 ^4 ((2x)/(x^2 +1))dx =−(1/2)[ln∣x−1∣]_0 ^4 −(1/2)[(1/(x−1))]_0 ^4 +(1/4)[ln(x^2 +1)]_0 ^4 =−(1/2)ln(3)−(1/2)((1/3)+1)+(1/4)ln(17) =−((ln3)/2)−(2/3) +((ln17)/4)](Q119423.png) | ||
| ||