Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 119580 by mathocean1 last updated on 25/Oct/20

Given k ∈ N. 1) justify these relations: 3^(2k) +1≡2[8] and 3^(2k+1) +1≡4[8]. 2) Given (E): 2^n −3^m =1. n and m are unknowed. • Show that if m is even , (E) does not have solution. ■ Deduct from the first question 1) that the couple (2;1) is the only solution of (E).

$$\mathrm{Given}\:\mathrm{k}\:\in\:\mathbb{N}. \\ $$$$\left.\mathrm{1}\right)\:\mathrm{justify}\:\mathrm{these}\:\mathrm{relations}: \\ $$$$\mathrm{3}^{\mathrm{2k}} +\mathrm{1}\equiv\mathrm{2}\left[\mathrm{8}\right]\:\mathrm{and}\:\mathrm{3}^{\mathrm{2k}+\mathrm{1}} +\mathrm{1}\equiv\mathrm{4}\left[\mathrm{8}\right]. \\ $$$$\left.\mathrm{2}\right)\:\mathrm{Given}\:\left(\mathrm{E}\right):\:\mathrm{2}^{\mathrm{n}} −\mathrm{3}^{\mathrm{m}} =\mathrm{1}.\:\mathrm{n}\:\mathrm{and}\:\mathrm{m}\:\mathrm{are}\:\mathrm{unknowed}. \\ $$$$\bullet\:\mathrm{Show}\:\mathrm{that}\:\mathrm{if}\:\mathrm{m}\:\mathrm{is}\:\mathrm{even}\:,\:\left(\mathrm{E}\right)\:\mathrm{does}\:\mathrm{not}\:\mathrm{have}\: \\ $$$$\mathrm{solution}. \\ $$$$\left.\blacksquare\:\mathrm{Deduct}\:\mathrm{from}\:\mathrm{the}\:\mathrm{first}\:\mathrm{question}\:\mathrm{1}\right)\:\mathrm{that}\:\mathrm{the} \\ $$$$\mathrm{couple}\:\left(\mathrm{2};\mathrm{1}\right)\:\mathrm{is}\:\mathrm{the}\:\mathrm{only}\:\mathrm{solution}\:\mathrm{of}\:\left(\mathrm{E}\right). \\ $$

Answered by mindispower last updated on 25/Oct/20

3^(2k+1) +1≡4[8] ?

$$\mathrm{3}^{\mathrm{2}{k}+\mathrm{1}} +\mathrm{1}\equiv\mathrm{4}\left[\mathrm{8}\right]\:? \\ $$

Commented by mathocean1 last updated on 25/Oct/20

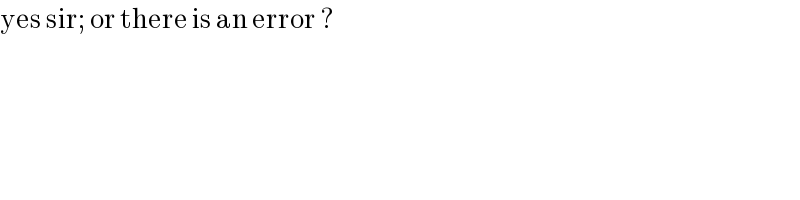
yes sir; or there is an error ?

$$\mathrm{yes}\:\mathrm{sir};\:\mathrm{or}\:\mathrm{there}\:\mathrm{is}\:\mathrm{an}\:\mathrm{error}\:? \\ $$

Answered by mindispower last updated on 25/Oct/20

3^(2k) +1=9^k +1≡1^k +1=2[8] 3^(2k+1) +1=9^k .3+1≡3.(1)^k +1≡4]8] (E)⇔2^n −(3^m +1)=0 m=2k ⇒2^n =(1+3^(2k) )≡2[8] withe 1⇒n=1 but n=1 (E)⇒2−9^k =1⇒k=0 (E) has solution (n,m)=(1,0) m=2k+1 ⇒2^n =(1+3^(2k+1) )≡4(8)...withe Quation 1 ⇒n=2, 4−3.9^k =1⇒k=0 m=2.0+1=1,n=2 solution are(n,m)∈{(1,0);(2,1)}

$$\mathrm{3}^{\mathrm{2}{k}} +\mathrm{1}=\mathrm{9}^{{k}} +\mathrm{1}\equiv\mathrm{1}^{{k}} +\mathrm{1}=\mathrm{2}\left[\mathrm{8}\right] \\ $$$$\left.\mathrm{3}^{\mathrm{2}{k}+\mathrm{1}} \left.+\mathrm{1}=\mathrm{9}^{{k}} .\mathrm{3}+\mathrm{1}\equiv\mathrm{3}.\left(\mathrm{1}\right)^{{k}} +\mathrm{1}\equiv\mathrm{4}\right]\mathrm{8}\right] \\ $$$$\left({E}\right)\Leftrightarrow\mathrm{2}^{{n}} −\left(\mathrm{3}^{{m}} +\mathrm{1}\right)=\mathrm{0} \\ $$$${m}=\mathrm{2}{k} \\ $$$$\Rightarrow\mathrm{2}^{{n}} =\left(\mathrm{1}+\mathrm{3}^{\mathrm{2}{k}} \right)\equiv\mathrm{2}\left[\mathrm{8}\right] \\ $$$${withe}\:\mathrm{1}\Rightarrow{n}=\mathrm{1} \\ $$$${but}\:{n}=\mathrm{1}\:\left({E}\right)\Rightarrow\mathrm{2}−\mathrm{9}^{{k}} =\mathrm{1}\Rightarrow{k}=\mathrm{0} \\ $$$$\left({E}\right)\:{has}\:{solution}\:\left({n},{m}\right)=\left(\mathrm{1},\mathrm{0}\right) \\ $$$${m}=\mathrm{2}{k}+\mathrm{1} \\ $$$$\Rightarrow\mathrm{2}^{{n}} =\left(\mathrm{1}+\mathrm{3}^{\mathrm{2}{k}+\mathrm{1}} \right)\equiv\mathrm{4}\left(\mathrm{8}\right)...{withe}\:{Quation}\:\mathrm{1} \\ $$$$\Rightarrow{n}=\mathrm{2}, \\ $$$$\mathrm{4}−\mathrm{3}.\mathrm{9}^{{k}} =\mathrm{1}\Rightarrow{k}=\mathrm{0} \\ $$$${m}=\mathrm{2}.\mathrm{0}+\mathrm{1}=\mathrm{1},{n}=\mathrm{2} \\ $$$${solution}\:{are}\left({n},{m}\right)\in\left\{\left(\mathrm{1},\mathrm{0}\right);\left(\mathrm{2},\mathrm{1}\right)\right\} \\ $$$$ \\ $$

Answered by 1549442205PVT last updated on 25/Oct/20

1a−We have 3^(2k) +1=9^k +1=(8+1)^k +1 =Σ_(m=0) ^(k) C_k ^m 8^(k−m) =(8^k +k.8^(k−1) +((k(k−))/2)8^(k−2) +...+8+1)+1≡2(mod8)(q.e.d) b−By above proof we have 3^(2k) +1=8q+2 ⇒3^(2k+1) +1=3.(3^(2k) +1)−2=3.(8q+2) −2=3.8q+4≡4(mod8)( q.e.d) 2)a−Consider the equation 2^n −3^m =1 If m is even then 2^n =3^(2k) +1(∗) (by above proof ).For n=0,1,2 we see the equation (∗) has no roots For n≥3 L.H.S (∗)is divisible by 8 while R.H.S isn′t divisible 8 since 3^(2k) +1≡2(mod8)(by above proof). Hence this case the equation has no roots (q.e.d) b−We see that (by directly checking) the (n,m)=(2,1) satisfy the equation 2^n −3^m =1(1).We prove that is unique solution of given equation.Indeed, When m is even the equation has no roots (above proof).We now consider the case m is odd.⇒m=2k+1.Then (1)⇔2^n =3^(2k+1) +1(∗∗) For n=0,1 It is clear that the equation (∗∗)has no roots For n≥2 L.H.S(∗∗)is divisible by 4 while R.H.S (∗∗)isn′t divisible (by above proof 1b).Hence this case the given has no roots which means the (n,m)=(2,1) is unique solution of the given equation (q.e.d)

$$\mathrm{1a}−\mathrm{We}\:\mathrm{have}\:\mathrm{3}^{\mathrm{2k}} +\mathrm{1}=\mathrm{9}^{\mathrm{k}} +\mathrm{1}=\left(\mathrm{8}+\mathrm{1}\right)^{\mathrm{k}} +\mathrm{1} \\ $$$$=\underset{\mathrm{m}=\mathrm{0}} {\overset{\mathrm{k}} {\Sigma}}\mathrm{C}_{\mathrm{k}} ^{\mathrm{m}} \mathrm{8}^{\mathrm{k}−\mathrm{m}} =\left(\mathrm{8}^{\mathrm{k}} +\mathrm{k}.\mathrm{8}^{\mathrm{k}−\mathrm{1}} +\frac{\mathrm{k}\left(\mathrm{k}−\right)}{\mathrm{2}}\mathrm{8}^{\mathrm{k}−\mathrm{2}} \right. \\ $$$$\left.+...+\mathrm{8}+\mathrm{1}\right)+\mathrm{1}\equiv\mathrm{2}\left(\mathrm{mod8}\right)\left(\boldsymbol{\mathrm{q}}.\boldsymbol{\mathrm{e}}.\boldsymbol{\mathrm{d}}\right) \\ $$$$\mathrm{b}−\mathrm{By}\:\mathrm{above}\:\mathrm{proof}\:\mathrm{we}\:\mathrm{have}\:\mathrm{3}^{\mathrm{2k}} +\mathrm{1}=\mathrm{8q}+\mathrm{2} \\ $$$$\Rightarrow\mathrm{3}^{\mathrm{2k}+\mathrm{1}} +\mathrm{1}=\mathrm{3}.\left(\mathrm{3}^{\mathrm{2k}} +\mathrm{1}\right)−\mathrm{2}=\mathrm{3}.\left(\mathrm{8q}+\mathrm{2}\right) \\ $$$$−\mathrm{2}=\mathrm{3}.\mathrm{8q}+\mathrm{4}\equiv\mathrm{4}\left(\mathrm{mod8}\right)\left(\:\boldsymbol{\mathrm{q}}.\boldsymbol{\mathrm{e}}.\boldsymbol{\mathrm{d}}\right) \\ $$$$\left.\mathrm{2}\right)\mathrm{a}−\mathrm{Consider}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{2}^{\mathrm{n}} −\mathrm{3}^{\mathrm{m}} =\mathrm{1} \\ $$$$\mathrm{If}\:\mathrm{m}\:\mathrm{is}\:\mathrm{even}\:\mathrm{then}\:\mathrm{2}^{\mathrm{n}} =\mathrm{3}^{\mathrm{2k}} +\mathrm{1}\left(\ast\right) \\ $$$$\left(\mathrm{by}\:\mathrm{above}\:\mathrm{proof}\:\right).\mathrm{For}\:\mathrm{n}=\mathrm{0},\mathrm{1},\mathrm{2}\:\mathrm{we}\:\mathrm{see} \\ $$$$\mathrm{the}\:\mathrm{equation}\:\left(\ast\right)\:\mathrm{has}\:\mathrm{no}\:\mathrm{roots} \\ $$$$\mathrm{For}\:\mathrm{n}\geqslant\mathrm{3}\:\mathrm{L}.\mathrm{H}.\mathrm{S}\:\left(\ast\right)\mathrm{is}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{8} \\ $$$$\mathrm{while}\:\mathrm{R}.\mathrm{H}.\mathrm{S}\:\mathrm{isn}'\mathrm{t}\:\mathrm{divisible}\:\mathrm{8}\:\mathrm{since} \\ $$$$\mathrm{3}^{\mathrm{2k}} +\mathrm{1}\equiv\mathrm{2}\left(\mathrm{mod8}\right)\left(\mathrm{by}\:\mathrm{above}\:\mathrm{proof}\right). \\ $$$$\mathrm{Hence}\:\mathrm{this}\:\mathrm{case}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{no}\:\mathrm{roots}\: \\ $$$$\left(\boldsymbol{\mathrm{q}}.\boldsymbol{\mathrm{e}}.\boldsymbol{\mathrm{d}}\right) \\ $$$$\mathrm{b}−\mathrm{We}\:\mathrm{see}\:\mathrm{that}\:\left(\mathrm{by}\:\mathrm{directly}\:\mathrm{checking}\right) \\ $$$$\mathrm{the}\:\left(\mathrm{n},\mathrm{m}\right)=\left(\mathrm{2},\mathrm{1}\right)\:\mathrm{satisfy}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{2}^{\mathrm{n}} −\mathrm{3}^{\mathrm{m}} =\mathrm{1}\left(\mathrm{1}\right).\mathrm{We}\:\mathrm{prove}\:\mathrm{that}\:\mathrm{is}\:\mathrm{unique} \\ $$$$\mathrm{solution}\:\mathrm{of}\:\mathrm{given}\:\mathrm{equation}.\mathrm{Indeed}, \\ $$$$\mathrm{When}\:\mathrm{m}\:\mathrm{is}\:\mathrm{even}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{no} \\ $$$$\mathrm{roots}\:\left(\mathrm{above}\:\mathrm{proof}\right).\mathrm{We}\:\mathrm{now}\:\mathrm{consider} \\ $$$$\mathrm{the}\:\mathrm{case}\:\mathrm{m}\:\mathrm{is}\:\mathrm{odd}.\Rightarrow\mathrm{m}=\mathrm{2k}+\mathrm{1}.\mathrm{Then} \\ $$$$\left(\mathrm{1}\right)\Leftrightarrow\mathrm{2}^{\mathrm{n}} =\mathrm{3}^{\mathrm{2k}+\mathrm{1}} +\mathrm{1}\left(\ast\ast\right) \\ $$$$\mathrm{For}\:\mathrm{n}=\mathrm{0},\mathrm{1}\:\mathrm{It}\:\mathrm{is}\:\mathrm{clear}\:\mathrm{that}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\left(\ast\ast\right)\mathrm{has}\:\mathrm{no}\:\mathrm{roots} \\ $$$$\mathrm{For}\:\mathrm{n}\geqslant\mathrm{2}\:\mathrm{L}.\mathrm{H}.\mathrm{S}\left(\ast\ast\right)\mathrm{is}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{4} \\ $$$$\mathrm{while}\:\mathrm{R}.\mathrm{H}.\mathrm{S}\:\left(\ast\ast\right)\mathrm{isn}'\mathrm{t}\:\mathrm{divisible}\:\left(\mathrm{by}\right. \\ $$$$\left.\mathrm{above}\:\mathrm{proof}\:\mathrm{1b}\right).\mathrm{Hence}\:\mathrm{this}\:\mathrm{case}\:\mathrm{the} \\ $$$$\mathrm{given}\:\mathrm{has}\:\mathrm{no}\:\mathrm{roots}\:\mathrm{which}\:\mathrm{means}\:\mathrm{the} \\ $$$$\left(\mathrm{n},\mathrm{m}\right)=\left(\mathrm{2},\mathrm{1}\right)\:\mathrm{is}\:\mathrm{unique}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{given}\:\mathrm{equation}\:\left(\boldsymbol{\mathrm{q}}.\boldsymbol{\mathrm{e}}.\boldsymbol{\mathrm{d}}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com