Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 119644 by bemath last updated on 25/Oct/20

(1) ∫ x^5 (√(x^3 +1)) dx (2) (dy/dx) = (3x−2y+1)^2

$$\:\left(\mathrm{1}\right)\:\int\:{x}^{\mathrm{5}} \:\sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:{dx}\: \\ $$$$\left(\mathrm{2}\right)\:\frac{{dy}}{{dx}}\:=\:\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)^{\mathrm{2}} \\ $$

Answered by benjo_mathlover last updated on 26/Oct/20

(1)∫ x^3 (x^2 (√(x^3 +1)) )dx D.I method ( by part ) { ((u=x^3 → du=3x^2 )),((v=(1/3)∫3x^2 (√(x^3 +1)) dx = (2/9)(x^3 +1)^(3/2) )) :} = (2/9)x^3 (x^3 +1)^(3/2) −(2/9)∫ (3x^2 )(x^3 +1)^(3/2) dx = (2/9)x^3 (x^3 +1)^(3/2) −(4/(45))(x^3 +1)^(5/2) + C = (2/9)(x^3 +1)^(3/2) (x^3 −(2/5)(x^3 +1))+ C = (2/(45))(x^3 +1)^(3/2) (3x^3 −2) + C

$$\left(\mathrm{1}\right)\int\:{x}^{\mathrm{3}} \:\left({x}^{\mathrm{2}} \:\sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:\right){dx}\: \\ $$$$\:\:\:\:\:\:{D}.{I}\:{method}\:\left(\:{by}\:{part}\:\right)\:\begin{cases}{{u}={x}^{\mathrm{3}} \rightarrow\:{du}=\mathrm{3}{x}^{\mathrm{2}} }\\{{v}=\frac{\mathrm{1}}{\mathrm{3}}\int\mathrm{3}{x}^{\mathrm{2}} \sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:{dx}\:=\:\frac{\mathrm{2}}{\mathrm{9}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\end{cases} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\mathrm{9}}{x}^{\mathrm{3}} \left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} −\frac{\mathrm{2}}{\mathrm{9}}\int\:\left(\mathrm{3}{x}^{\mathrm{2}} \right)\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} {dx} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\mathrm{9}}{x}^{\mathrm{3}} \left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} −\frac{\mathrm{4}}{\mathrm{45}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{5}}{\mathrm{2}}} +\:{C} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\mathrm{9}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \left({x}^{\mathrm{3}} −\frac{\mathrm{2}}{\mathrm{5}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)\right)+\:{C} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\mathrm{45}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \left(\mathrm{3}{x}^{\mathrm{3}} −\mathrm{2}\right)\:+\:{C}\: \\ $$

Answered by 1549442205PVT last updated on 26/Oct/20

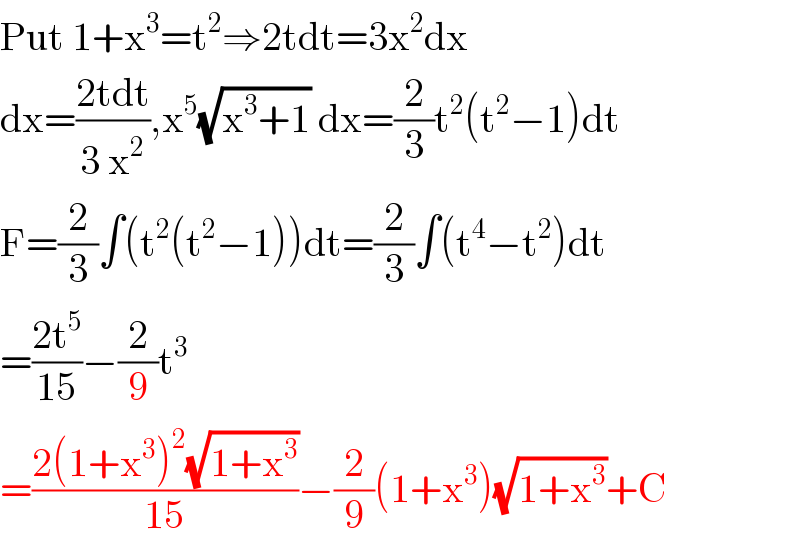
Put 1+x^3 =t^2 ⇒2tdt=3x^2 dx dx=((2tdt)/(3 x^2 )),x^5 (√(x^3 +1)) dx=(2/3)t^2 (t^2 −1)dt F=(2/3)∫(t^2 (t^2 −1))dt=(2/3)∫(t^4 −t^2 )dt =((2t^5 )/(15))−(2/9)t^3 =((2(1+x^3 )^2 (√(1+x^3 )))/(15))−(2/9)(1+x^3 )(√(1+x^3 ))+C

$$\mathrm{Put}\:\mathrm{1}+\mathrm{x}^{\mathrm{3}} =\mathrm{t}^{\mathrm{2}} \Rightarrow\mathrm{2tdt}=\mathrm{3x}^{\mathrm{2}} \mathrm{dx} \\ $$$$\mathrm{dx}=\frac{\mathrm{2tdt}}{\mathrm{3}\:\mathrm{x}^{\mathrm{2}} },\mathrm{x}^{\mathrm{5}} \sqrt{\mathrm{x}^{\mathrm{3}} +\mathrm{1}}\:\mathrm{dx}=\frac{\mathrm{2}}{\mathrm{3}}\mathrm{t}^{\mathrm{2}} \left(\mathrm{t}^{\mathrm{2}} −\mathrm{1}\right)\mathrm{dt} \\ $$$$\mathrm{F}=\frac{\mathrm{2}}{\mathrm{3}}\int\left(\mathrm{t}^{\mathrm{2}} \left(\mathrm{t}^{\mathrm{2}} −\mathrm{1}\right)\right)\mathrm{dt}=\frac{\mathrm{2}}{\mathrm{3}}\int\left(\mathrm{t}^{\mathrm{4}} −\mathrm{t}^{\mathrm{2}} \right)\mathrm{dt} \\ $$$$=\frac{\mathrm{2t}^{\mathrm{5}} }{\mathrm{15}}−\frac{\mathrm{2}}{\mathrm{9}}\mathrm{t}^{\mathrm{3}} \\ $$$$=\frac{\mathrm{2}\left(\mathrm{1}+\mathrm{x}^{\mathrm{3}} \right)^{\mathrm{2}} \sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{3}} }}{\mathrm{15}}−\frac{\mathrm{2}}{\mathrm{9}}\left(\mathrm{1}+\mathrm{x}^{\mathrm{3}} \right)\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{3}} }+\mathrm{C} \\ $$

Commented by benjo_mathlover last updated on 26/Oct/20

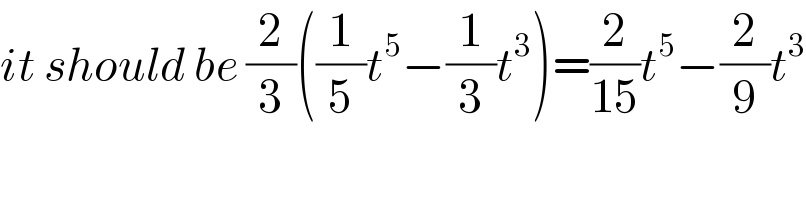
it should be (2/3)((1/5)t^5 −(1/3)t^3 )=(2/(15))t^5 −(2/9)t^3

$${it}\:{should}\:{be}\:\frac{\mathrm{2}}{\mathrm{3}}\left(\frac{\mathrm{1}}{\mathrm{5}}{t}^{\mathrm{5}} −\frac{\mathrm{1}}{\mathrm{3}}{t}^{\mathrm{3}} \right)=\frac{\mathrm{2}}{\mathrm{15}}{t}^{\mathrm{5}} −\frac{\mathrm{2}}{\mathrm{9}}{t}^{\mathrm{3}} \\ $$

Commented by 1549442205PVT last updated on 26/Oct/20

Ok,a mistake .I corrected.Thank sir

$$\mathrm{Ok},\mathrm{a}\:\mathrm{mistake}\:.\mathrm{I}\:\mathrm{corrected}.\mathrm{Thank}\:\mathrm{sir} \\ $$

Answered by benjo_mathlover last updated on 26/Oct/20

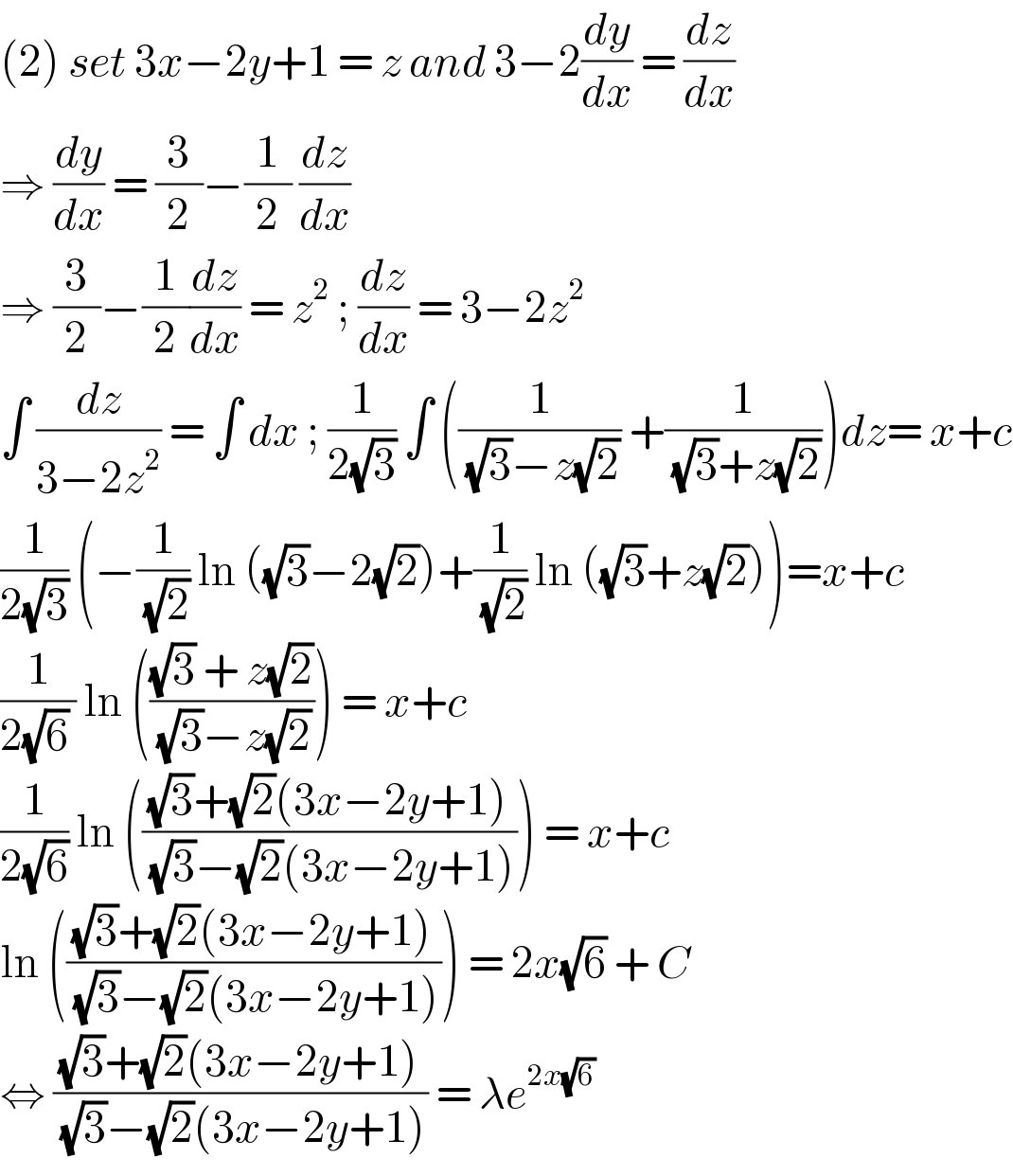
(2) set 3x−2y+1 = z and 3−2(dy/dx) = (dz/dx) ⇒ (dy/dx) = (3/2)−(1/2) (dz/dx) ⇒ (3/2)−(1/2)(dz/dx) = z^2 ; (dz/dx) = 3−2z^2 ∫ (dz/(3−2z^2 )) = ∫ dx ; (1/(2(√3))) ∫ ((1/( (√3)−z(√2))) +(1/( (√3)+z(√2))))dz= x+c (1/(2(√3))) (−(1/( (√2))) ln ((√3)−2(√2))+(1/( (√2))) ln ((√3)+z(√2)))=x+c (1/(2(√6) )) ln ((((√3) + z(√2))/( (√3)−z(√2)))) = x+c (1/(2(√6))) ln ((((√3)+(√2)(3x−2y+1))/( (√3)−(√2)(3x−2y+1)))) = x+c ln ((((√3)+(√2)(3x−2y+1))/( (√3)−(√2)(3x−2y+1)))) = 2x(√6) + C ⇔ (((√3)+(√2)(3x−2y+1))/( (√3)−(√2)(3x−2y+1))) = λe^(2x(√6))

$$\left(\mathrm{2}\right)\:{set}\:\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\:=\:{z}\:{and}\:\mathrm{3}−\mathrm{2}\frac{{dy}}{{dx}}\:=\:\frac{{dz}}{{dx}} \\ $$$$\Rightarrow\:\frac{{dy}}{{dx}}\:=\:\frac{\mathrm{3}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\:\frac{{dz}}{{dx}} \\ $$$$\Rightarrow\:\frac{\mathrm{3}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\frac{{dz}}{{dx}}\:=\:{z}^{\mathrm{2}} \:;\:\frac{{dz}}{{dx}}\:=\:\mathrm{3}−\mathrm{2}{z}^{\mathrm{2}} \\ $$$$\int\:\frac{{dz}}{\mathrm{3}−\mathrm{2}{z}^{\mathrm{2}} }\:=\:\int\:{dx}\:;\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\int\:\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}−{z}\sqrt{\mathrm{2}}}\:+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}+{z}\sqrt{\mathrm{2}}}\right){dz}=\:{x}+{c} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\left(−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{ln}\:\left(\sqrt{\mathrm{3}}−\mathrm{2}\sqrt{\mathrm{2}}\right)+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{ln}\:\left(\sqrt{\mathrm{3}}+{z}\sqrt{\mathrm{2}}\right)\right)={x}+{c} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{6}}\:}\:\mathrm{ln}\:\left(\frac{\sqrt{\mathrm{3}}\:+\:{z}\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{3}}−{z}\sqrt{\mathrm{2}}}\right)\:=\:{x}+{c}\: \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{6}}}\:\mathrm{ln}\:\left(\frac{\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)}\right)\:=\:{x}+{c} \\ $$$$\mathrm{ln}\:\left(\frac{\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)}\right)\:=\:\mathrm{2}{x}\sqrt{\mathrm{6}}\:+\:{C} \\ $$$$\Leftrightarrow\:\frac{\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\left(\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1}\right)}\:=\:\lambda{e}^{\mathrm{2}{x}\sqrt{\mathrm{6}}} \: \\ $$

Answered by TANMAY PANACEA last updated on 26/Oct/20

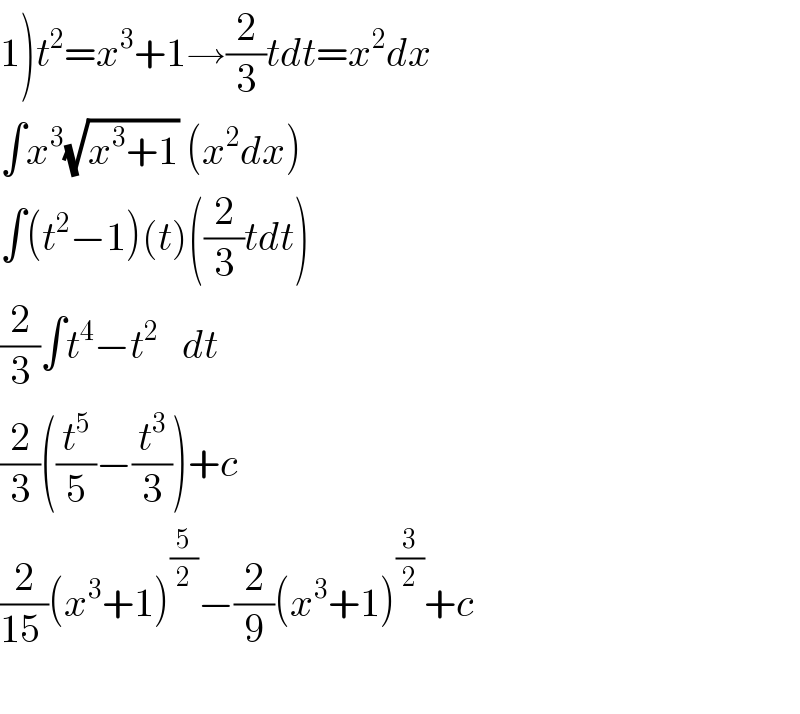
1)t^2 =x^3 +1→(2/3)tdt=x^2 dx ∫x^3 (√(x^3 +1)) (x^2 dx) ∫(t^2 −1)(t)((2/3)tdt) (2/3)∫t^4 −t^2 dt (2/3)((t^5 /5)−(t^3 /3))+c (2/(15 ))(x^3 +1)^(5/2) −(2/9)(x^3 +1)^(3/2) +c

$$\left.\mathrm{1}\right){t}^{\mathrm{2}} ={x}^{\mathrm{3}} +\mathrm{1}\rightarrow\frac{\mathrm{2}}{\mathrm{3}}{tdt}={x}^{\mathrm{2}} {dx} \\ $$$$\int{x}^{\mathrm{3}} \sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:\left({x}^{\mathrm{2}} {dx}\right) \\ $$$$\int\left({t}^{\mathrm{2}} −\mathrm{1}\right)\left({t}\right)\left(\frac{\mathrm{2}}{\mathrm{3}}{tdt}\right) \\ $$$$\frac{\mathrm{2}}{\mathrm{3}}\int{t}^{\mathrm{4}} −{t}^{\mathrm{2}} \:\:\:{dt} \\ $$$$\frac{\mathrm{2}}{\mathrm{3}}\left(\frac{{t}^{\mathrm{5}} }{\mathrm{5}}−\frac{{t}^{\mathrm{3}} }{\mathrm{3}}\right)+{c} \\ $$$$\frac{\mathrm{2}}{\mathrm{15}\:}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{5}}{\mathrm{2}}} −\frac{\mathrm{2}}{\mathrm{9}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} +{c} \\ $$$$ \\ $$

Answered by Olaf last updated on 26/Oct/20

(2) u = 3x−2y+1 (du/dx) = 3−2(dy/dx) (dy/dx) = −(1/2).(du/dx)+(3/2) −(1/2).(du/dx)+(3/2) = u^2 (du/dx) = 3−2u^2 (du/(3−2u^2 )) = dx (du/(((√3)−(√2)u)((√3)+(√2)u))) = dx (1/(2(√3)))[(1/( (√3)−(√2)u))+(1/( (√(3+))(√2)u))]du = dx (1/(2(√3)))[−(1/( (√2)))ln∣(√3)−(√2)u∣+(1/( (√2)))ln∣(√3)+(√2)u∣] = x+C_1 ln∣(((√3)+(√2)u)/( (√3)−(√2)u))∣ = 2(√6)x+C_2 ∣(((√3)+(√2)u)/( (√3)−(√2)u))∣ = C_3 e^(2(√6)x) (((√3)+(√2)u)/( (√3)−(√2)u)) = C_4 e^(2(√6)x) (√3)+(√2)u = ((√3)−(√2)u)C_4 e^(2(√6)x) (√2)u(1+C_4 e^(2(√6)x) ) = (√3)(−1+C_4 e^(2(√6)x) ) u = (√(3/2))(((−1+C_4 e^(2(√6)x) )/(1+C_4 e^(2(√6)x) ))) = 3x−2y+1 y = − (1/2)(√(3/2))(((−1+C_4 e^(2(√6)x) )/(1+C_4 e^(2(√6)x) )))+(3/2)x+(1/2)

$$\left(\mathrm{2}\right) \\ $$$${u}\:=\:\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1} \\ $$$$\frac{{du}}{{dx}}\:=\:\mathrm{3}−\mathrm{2}\frac{{dy}}{{dx}} \\ $$$$\frac{{dy}}{{dx}}\:=\:−\frac{\mathrm{1}}{\mathrm{2}}.\frac{{du}}{{dx}}+\frac{\mathrm{3}}{\mathrm{2}} \\ $$$$ \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}}.\frac{{du}}{{dx}}+\frac{\mathrm{3}}{\mathrm{2}}\:=\:{u}^{\mathrm{2}} \\ $$$$\frac{{du}}{{dx}}\:=\:\mathrm{3}−\mathrm{2}{u}^{\mathrm{2}} \\ $$$$\frac{{du}}{\mathrm{3}−\mathrm{2}{u}^{\mathrm{2}} }\:=\:{dx} \\ $$$$\frac{{du}}{\left(\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}\right)\left(\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}{u}\right)}\:=\:{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\left[\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}+}\sqrt{\mathrm{2}}{u}}\right]{du}\:=\:{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\left[−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{ln}\mid\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}\mid+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{ln}\mid\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}{u}\mid\right]\:=\:{x}+\mathrm{C}_{\mathrm{1}} \\ $$$$\mathrm{ln}\mid\frac{\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}{u}}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}}\mid\:=\:\mathrm{2}\sqrt{\mathrm{6}}{x}+\mathrm{C}_{\mathrm{2}} \\ $$$$\mid\frac{\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}{u}}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}}\mid\:=\:\mathrm{C}_{\mathrm{3}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} \\ $$$$\frac{\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}{u}}{\:\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}}\:=\:\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} \\ $$$$\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}}{u}\:=\:\left(\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}{u}\right)\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} \\ $$$$\sqrt{\mathrm{2}}{u}\left(\mathrm{1}+\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} \right)\:=\:\sqrt{\mathrm{3}}\left(−\mathrm{1}+\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} \right) \\ $$$${u}\:=\:\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\left(\frac{−\mathrm{1}+\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} }{\mathrm{1}+\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} }\right)\:=\:\mathrm{3}{x}−\mathrm{2}{y}+\mathrm{1} \\ $$$${y}\:=\:−\:\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\left(\frac{−\mathrm{1}+\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} }{\mathrm{1}+\mathrm{C}_{\mathrm{4}} {e}^{\mathrm{2}\sqrt{\mathrm{6}}{x}} }\right)+\frac{\mathrm{3}}{\mathrm{2}}{x}+\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com