Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 120195 by SOMEDAVONG last updated on 30/Oct/20

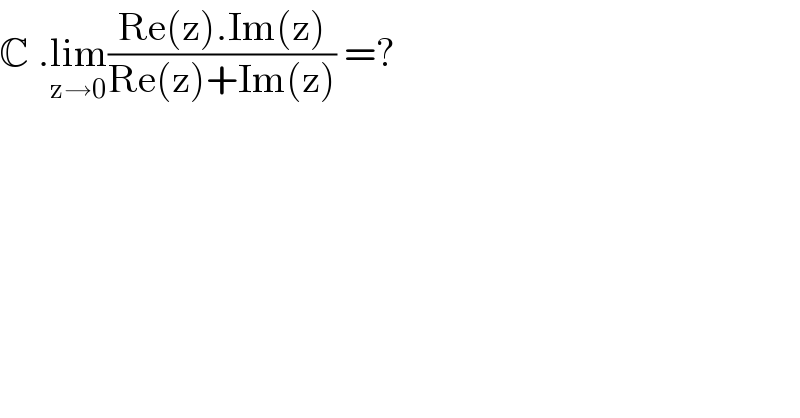
C .lim_(z→0) ((Re(z).Im(z))/(Re(z)+Im(z))) =?

$$\mathbb{C}\:.\underset{\mathrm{z}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{Re}\left(\mathrm{z}\right).\mathrm{Im}\left(\mathrm{z}\right)}{\mathrm{Re}\left(\mathrm{z}\right)+\mathrm{Im}\left(\mathrm{z}\right)}\:=? \\ $$

Answered by snipers237 last updated on 30/Oct/20

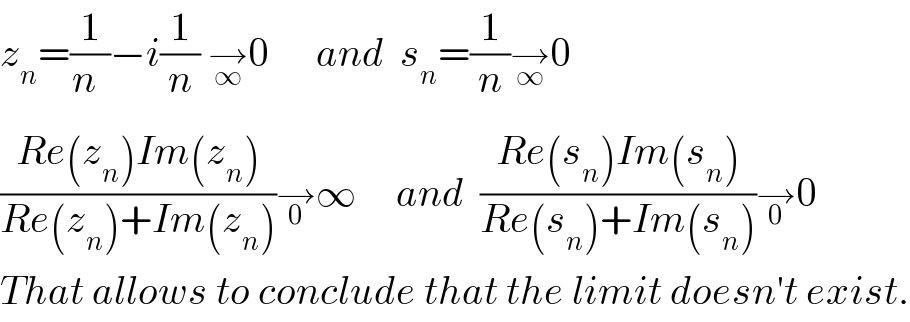
z_n =(1/n_ )−i(1/n) →_∞ 0 and s_n =(1/n)→_∞ 0 ((Re(z_n )Im(z_n ))/(Re(z_n )+Im(z_n )))→_0 ∞ and ((Re(s_n )Im(s_n ))/(Re(s_n )+Im(s_n )))→_0 0 That allows to conclude that the limit doesn′t exist.

$${z}_{{n}} =\frac{\mathrm{1}}{{n}_{} }−{i}\frac{\mathrm{1}}{{n}}\:\underset{\infty} {\rightarrow}\mathrm{0}\:\:\:\:\:\:{and}\:\:{s}_{{n}} =\frac{\mathrm{1}}{{n}}\underset{\infty} {\rightarrow}\mathrm{0} \\ $$$$\frac{{Re}\left({z}_{{n}} \right){Im}\left({z}_{{n}} \right)}{{Re}\left({z}_{{n}} \right)+{Im}\left({z}_{{n}} \right)}\underset{\mathrm{0}} {\rightarrow}\infty\:\:\:\:\:{and}\:\:\frac{{Re}\left({s}_{{n}} \right){Im}\left({s}_{{n}} \right)}{{Re}\left({s}_{{n}} \right)+{Im}\left({s}_{{n}} \right)}\underset{\mathrm{0}} {\rightarrow}\mathrm{0} \\ $$$${That}\:{allows}\:{to}\:{conclude}\:{that}\:{the}\:{limit}\:{doesn}'{t}\:{exist}. \\ $$

Commented by SOMEDAVONG last updated on 30/Oct/20

Thanks sir

$$\mathrm{Thanks}\:\mathrm{sir}\: \\ $$

Answered by MJS_new last updated on 30/Oct/20

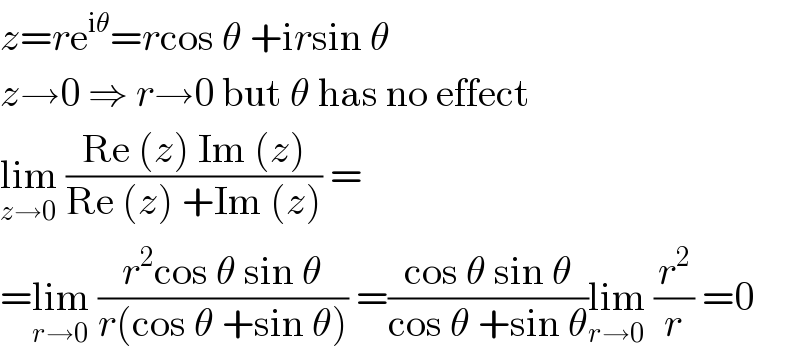
z=re^(iθ) =rcos θ +irsin θ z→0 ⇒ r→0 but θ has no effect lim_(z→0) ((Re (z) Im (z))/(Re (z) +Im (z))) = =lim_(r→0) ((r^2 cos θ sin θ)/(r(cos θ +sin θ))) =((cos θ sin θ)/(cos θ +sin θ))lim_(r→0) (r^2 /r) =0

$${z}={r}\mathrm{e}^{\mathrm{i}\theta} ={r}\mathrm{cos}\:\theta\:+\mathrm{i}{r}\mathrm{sin}\:\theta \\ $$$${z}\rightarrow\mathrm{0}\:\Rightarrow\:{r}\rightarrow\mathrm{0}\:\mathrm{but}\:\theta\:\mathrm{has}\:\mathrm{no}\:\mathrm{effect} \\ $$$$\underset{{z}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{Re}\:\left({z}\right)\:\mathrm{Im}\:\left({z}\right)}{\mathrm{Re}\:\left({z}\right)\:+\mathrm{Im}\:\left({z}\right)}\:= \\ $$$$=\underset{{r}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{r}^{\mathrm{2}} \mathrm{cos}\:\theta\:\mathrm{sin}\:\theta}{{r}\left(\mathrm{cos}\:\theta\:+\mathrm{sin}\:\theta\right)}\:=\frac{\mathrm{cos}\:\theta\:\mathrm{sin}\:\theta}{\mathrm{cos}\:\theta\:+\mathrm{sin}\:\theta}\underset{{r}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{r}^{\mathrm{2}} }{{r}}\:=\mathrm{0}\: \\ $$

Answered by MJS_new last updated on 30/Oct/20

let z=(1/a)+(1/b)i z→0 means a→∞∧b→∞ lim_( { ((a→∞)),((b→∞)) :}) (((1/a)×(1/b))/((1/a)+(1/b))) =lim_( { ((a→∞)),((b→∞)) :}) (1/(a+b)) =0

$$\mathrm{let}\:{z}=\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}\mathrm{i} \\ $$$${z}\rightarrow\mathrm{0}\:\mathrm{means}\:{a}\rightarrow\infty\wedge{b}\rightarrow\infty \\ $$$$\underset{\begin{cases}{{a}\rightarrow\infty}\\{{b}\rightarrow\infty}\end{cases}} {\mathrm{lim}}\:\frac{\frac{\mathrm{1}}{{a}}×\frac{\mathrm{1}}{{b}}}{\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}}\:=\underset{\begin{cases}{{a}\rightarrow\infty}\\{{b}\rightarrow\infty}\end{cases}} {\mathrm{lim}}\:\frac{\mathrm{1}}{{a}+{b}}\:=\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com