
Question Number 120297 by bramlexs22 last updated on 30/Oct/20
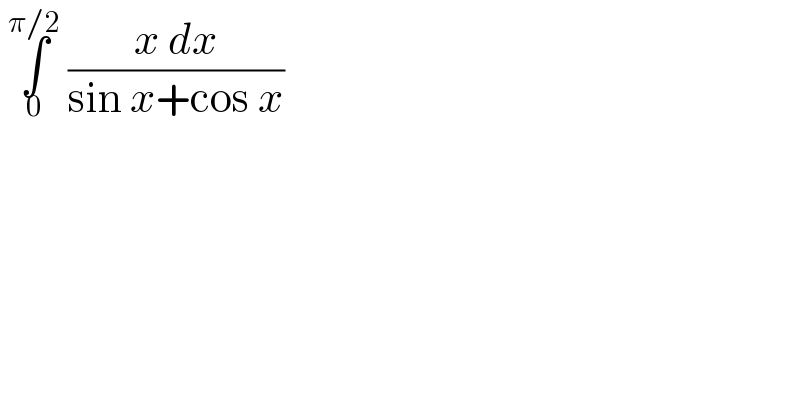
$$\:\underset{\mathrm{0}} {\overset{\pi/\mathrm{2}} {\int}}\:\frac{{x}\:{dx}}{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}} \\ $$
Answered by Dwaipayan Shikari last updated on 30/Oct/20
![∫_0 ^(π/2) (x/(sinx+cosx))dx=∫_0 ^(π/2) (((π/2)−x)/(sinx+cosx))dx=I 2I=∫_0 ^(π/2) ((π/2)/(sinx+cosx))dx (4/π)I=2∫_0 ^1 (1/(2t+1−t^2 ))dt tan(x/2)=t (2/π)I=∫_0 ^1 (1/(2−(t−1)^2 )) (2/π)I=−(1/(2(√2)))[(log(((t−1−(√2))/(t−1+(√2)))))]_0 ^1 (2/π)I=−(1/(2(√2)))(0−log((((√2)+1)/( (√2)−1))) I=(π/(4(√2)))log((((√2)+1)/( (√2)−1)))=(π/( 2(√2)))log((√2)+1)](Q120305.png)
$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{x}}{{sinx}+{cosx}}{dx}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\frac{\pi}{\mathrm{2}}−{x}}{{sinx}+{cosx}}{dx}={I} \\ $$$$\mathrm{2}{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\frac{\pi}{\mathrm{2}}}{{sinx}+{cosx}}{dx} \\ $$$$\frac{\mathrm{4}}{\pi}{I}=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}{t}+\mathrm{1}−{t}^{\mathrm{2}} }{dt}\:\:\:\:\:\:\:\:\:\:{tan}\frac{{x}}{\mathrm{2}}={t} \\ $$$$\frac{\mathrm{2}}{\pi}{I}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}−\left({t}−\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\frac{\mathrm{2}}{\pi}{I}=−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left[\left({log}\left(\frac{{t}−\mathrm{1}−\sqrt{\mathrm{2}}}{{t}−\mathrm{1}+\sqrt{\mathrm{2}}}\right)\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$\frac{\mathrm{2}}{\pi}{I}=−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left(\mathrm{0}−{log}\left(\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}\right)\right. \\ $$$${I}=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{2}}}{log}\left(\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}\right)=\frac{\pi}{\:\mathrm{2}\sqrt{\mathrm{2}}}{log}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$
Commented by peter frank last updated on 30/Oct/20
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{both} \\ $$
Answered by Bird last updated on 30/Oct/20
![A=∫_0 ^(π/2) ((xdx)/(sinx +cosx))dx ⇒A=_(x=(π/2)−t) ∫_(π/2) ^0 ((((π/2)−t))/(cost+sint))(−dt) =∫_0 ^(π/2) (((π/2)−t)/(cost +sint))dt =(π/2)∫_0 ^(π/2) (dx/(cosx +sinx))−A ⇒ A=(π/4)∫_0 ^(π/2) (dx/(cosx +sinx)) we have ∫_0 ^(π/2) (dx/(cosx +sinx)) =_(tsn((x/2))=u) ∫_0 ^1 ((2du)/((1+u^2 )(((1−u^2 )/(1+u^2 ))+((2u)/(1+u^2 ))))) =∫_0 ^1 ((2du)/(1−u^2 +2u)) =−2 ∫_0 ^1 (du/(u^2 −2u−1)) =−2∫_0 ^1 (du/((u−1)^2 −2)) =−2∫_0 ^1 (du/((u−1−(√2))(u−1+(√2)))) =−(2/(2(√2)))∫_0 ^1 ((1/(u−1−(√2)))−(1/(u−1+(√2))))du =(1/( (√2)))[ln∣((u−1+(√2))/(u−1−(√2)))∣]_0 ^1 =(1/( (√2))){−ln∣((−1+(√2))/(−1−(√2)))∣} =(1/( (√2)))ln(((1+(√2))/( (√2)−1))) ⇒ A =(π/(4(√2)))ln((((√2)+1)/( (√2)−1)))](Q120315.png)
$${A}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{xdx}}{{sinx}\:+{cosx}}{dx} \\ $$$$\Rightarrow{A}=_{{x}=\frac{\pi}{\mathrm{2}}−{t}} \:\:\:\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{0}} \frac{\left(\frac{\pi}{\mathrm{2}}−{t}\right)}{{cost}+{sint}}\left(−{dt}\right) \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\frac{\pi}{\mathrm{2}}−{t}}{{cost}\:+{sint}}{dt} \\ $$$$=\frac{\pi}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{dx}}{{cosx}\:+{sinx}}−{A}\:\Rightarrow \\ $$$${A}=\frac{\pi}{\mathrm{4}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{dx}}{{cosx}\:+{sinx}} \\ $$$${we}\:{have}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{dx}}{{cosx}\:+{sinx}} \\ $$$$=_{{tsn}\left(\frac{{x}}{\mathrm{2}}\right)={u}} \:\:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{2}{du}}{\left(\mathrm{1}+{u}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }+\frac{\mathrm{2}{u}}{\mathrm{1}+{u}^{\mathrm{2}} }\right)} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}{du}}{\mathrm{1}−{u}^{\mathrm{2}} +\mathrm{2}{u}}\:=−\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{du}}{{u}^{\mathrm{2}} −\mathrm{2}{u}−\mathrm{1}} \\ $$$$=−\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{du}}{\left({u}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}} \\ $$$$=−\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{du}}{\left({u}−\mathrm{1}−\sqrt{\mathrm{2}}\right)\left({u}−\mathrm{1}+\sqrt{\mathrm{2}}\right)} \\ $$$$=−\frac{\mathrm{2}}{\mathrm{2}\sqrt{\mathrm{2}}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\frac{\mathrm{1}}{{u}−\mathrm{1}−\sqrt{\mathrm{2}}}−\frac{\mathrm{1}}{{u}−\mathrm{1}+\sqrt{\mathrm{2}}}\right){du} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\left[{ln}\mid\frac{{u}−\mathrm{1}+\sqrt{\mathrm{2}}}{{u}−\mathrm{1}−\sqrt{\mathrm{2}}}\mid\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\left\{−{ln}\mid\frac{−\mathrm{1}+\sqrt{\mathrm{2}}}{−\mathrm{1}−\sqrt{\mathrm{2}}}\mid\right\} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{ln}\left(\frac{\mathrm{1}+\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}\right)\:\Rightarrow \\ $$$${A}\:=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{2}}}{ln}\left(\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}\right) \\ $$
Answered by TANMAY PANACEA last updated on 30/Oct/20
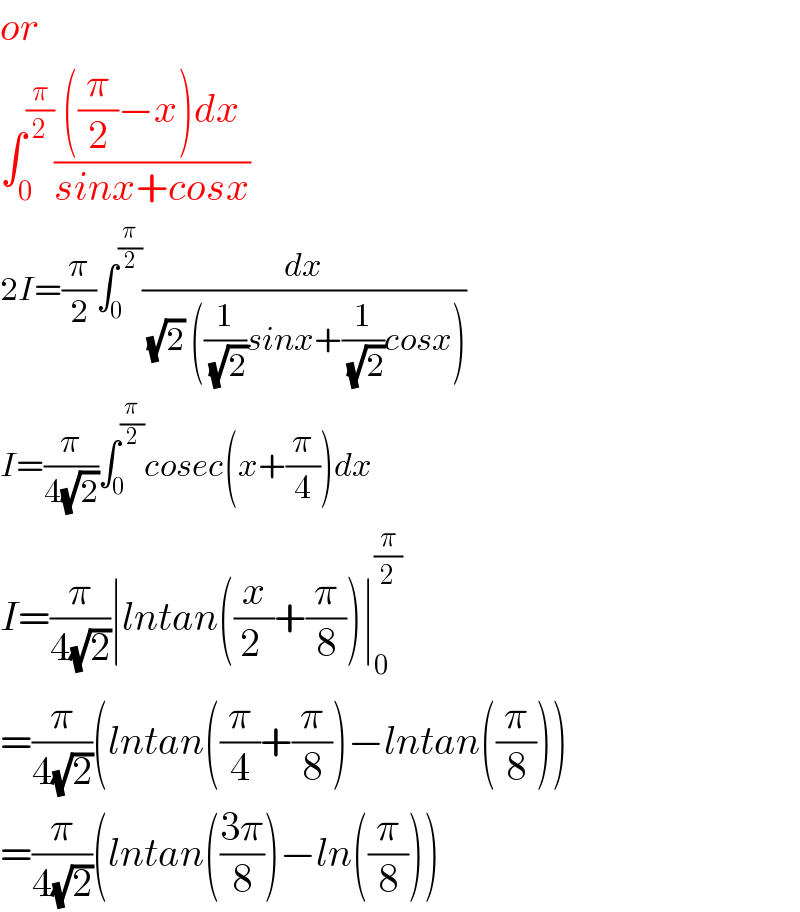
$${or} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\left(\frac{\pi}{\mathrm{2}}−{x}\right){dx}}{{sinx}+{cosx}} \\ $$$$\mathrm{2}{I}=\frac{\pi}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{dx}}{\:\sqrt{\mathrm{2}}\:\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{sinx}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{cosx}\right)} \\ $$$${I}=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{2}}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cosec}\left({x}+\frac{\pi}{\mathrm{4}}\right){dx} \\ $$$${I}=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{2}}}\mid{lntan}\left(\frac{{x}}{\mathrm{2}\:}+\frac{\pi}{\mathrm{8}}\right)\mid_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \\ $$$$=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{2}}}\left({lntan}\left(\frac{\pi}{\mathrm{4}}+\frac{\pi}{\mathrm{8}}\right)−{lntan}\left(\frac{\pi}{\mathrm{8}}\right)\right) \\ $$$$=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{2}}}\left({lntan}\left(\frac{\mathrm{3}\pi}{\mathrm{8}}\right)−{ln}\left(\frac{\pi}{\mathrm{8}}\right)\right) \\ $$
