Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 120325 by Ar Brandon last updated on 30/Oct/20

Let f:R→R be a function satisfying the functional relation (f(x))^y +(f(y))^x =2f(xy) for all x, y ∈R and it is given that f(1)=1/2. Answer the following questions. (i) f(x+y)= (A) f(x)+f(y) (B) f(x)f(y) (C) f(x^y y^x ) (D) ((f(x))/(f(y))) (ii) f(xy)= (A) f(x)f(y) (B) f(x)+f(y) (C) (f(x))^y (D) (f(xy))^(xy) (iii) Σ_(k=0) ^∞ f(k)= (A) 5/2 (B) 3/2 (C) 3 (D) 2

$$\mathrm{Let}\:{f}:\mathbb{R}\rightarrow\mathbb{R}\:\mathrm{be}\:\mathrm{a}\:\mathrm{function}\:\mathrm{satisfying}\:\mathrm{the} \\ $$$$\mathrm{functional}\:\mathrm{relation} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({f}\left(\mathrm{x}\right)\right)^{\mathrm{y}} +\left({f}\left(\mathrm{y}\right)\right)^{\mathrm{x}} =\mathrm{2}{f}\left(\mathrm{xy}\right) \\ $$$$\mathrm{for}\:\mathrm{all}\:\mathrm{x},\:\mathrm{y}\:\in\mathbb{R}\:\mathrm{and}\:\mathrm{it}\:\mathrm{is}\:\mathrm{given}\:\mathrm{that}\:{f}\left(\mathrm{1}\right)=\mathrm{1}/\mathrm{2}.\:\mathrm{Answer} \\ $$$$\mathrm{the}\:\mathrm{following}\:\mathrm{questions}. \\ $$$$\left(\boldsymbol{\mathrm{i}}\right)\:\:\:\:{f}\left(\mathrm{x}+\mathrm{y}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{A}\right)\:{f}\left(\mathrm{x}\right)+{f}\left(\mathrm{y}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\:{f}\left(\mathrm{x}\right){f}\left(\mathrm{y}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:{f}\left(\mathrm{x}^{\mathrm{y}} \mathrm{y}^{\mathrm{x}} \right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\:\:\frac{{f}\left(\mathrm{x}\right)}{{f}\left(\mathrm{y}\right)} \\ $$$$\left(\boldsymbol{\mathrm{ii}}\right)\:\:\:\:{f}\left(\mathrm{xy}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\:\left(\mathrm{A}\right)\:{f}\left(\mathrm{x}\right){f}\left(\mathrm{y}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:{f}\left(\mathrm{x}\right)+{f}\left(\mathrm{y}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\left({f}\left(\mathrm{x}\right)\right)^{\mathrm{y}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\:\left({f}\left(\mathrm{xy}\right)\right)^{\mathrm{xy}} \\ $$$$\left(\boldsymbol{\mathrm{iii}}\right)\:\:\underset{\mathrm{k}=\mathrm{0}} {\overset{\infty} {\sum}}{f}\left({k}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{A}\right)\:\mathrm{5}/\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{3}/\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\mathrm{3}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{2} \\ $$

Commented by Dwaipayan Shikari last updated on 30/Oct/20

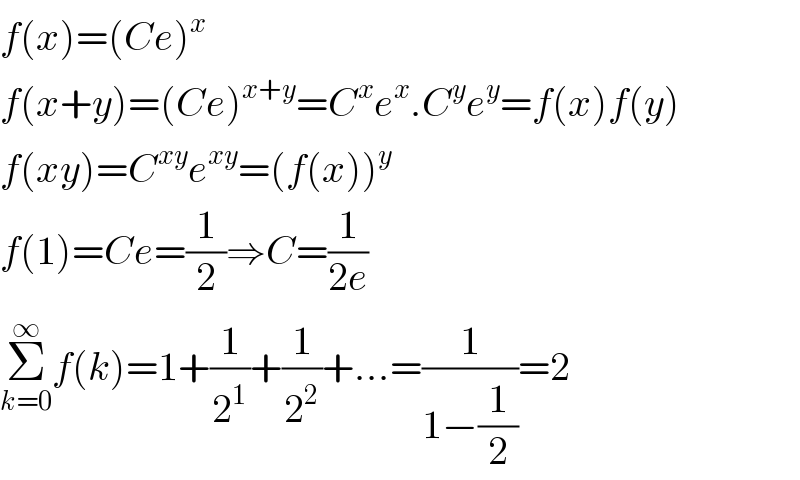
f(x)=(Ce)^x f(x+y)=(Ce)^(x+y) =C^x e^x .C^y e^y =f(x)f(y) f(xy)=C^(xy) e^(xy) =(f(x))^y f(1)=Ce=(1/2)⇒C=(1/(2e)) Σ_(k=0) ^∞ f(k)=1+(1/2^1 )+(1/2^2 )+...=(1/(1−(1/2)))=2

$${f}\left({x}\right)=\left({Ce}\right)^{{x}} \\ $$$${f}\left({x}+{y}\right)=\left({Ce}\right)^{{x}+{y}} ={C}^{{x}} {e}^{{x}} .{C}^{{y}} {e}^{{y}} ={f}\left({x}\right){f}\left({y}\right) \\ $$$${f}\left({xy}\right)={C}^{{xy}} {e}^{{xy}} =\left({f}\left({x}\right)\right)^{{y}} \\ $$$${f}\left(\mathrm{1}\right)={Ce}=\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow{C}=\frac{\mathrm{1}}{\mathrm{2}{e}} \\ $$$$\underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{f}\left({k}\right)=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{1}} }+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+...=\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}}=\mathrm{2} \\ $$

Commented by Ar Brandon last updated on 30/Oct/20

Wow ! Thanks bro. Are there any calculations to get f(x)=(Ce)^x ?

$$\mathrm{Wow}\:!\:\mathrm{Thanks}\:\mathrm{bro}.\:\mathrm{Are}\:\mathrm{there}\:\mathrm{any}\: \\ $$$$\:\mathrm{calculations}\:\mathrm{to}\:\mathrm{get}\:{f}\left(\mathrm{x}\right)=\left(\mathrm{Ce}\right)^{\mathrm{x}} \:? \\ $$

Commented by Dwaipayan Shikari last updated on 30/Oct/20

I just assumed it

$${I}\:{just}\:{assumed}\:{it} \\ $$

Commented by mindispower last updated on 30/Oct/20

f(x)^y +f(y)^x =2f(xy),x=1 ⇒f(1)^y +f(y)=2f(y) f(y)=f(1)^y =(1/2^y )

$${f}\left({x}\right)^{{y}} +{f}\left({y}\right)^{{x}} =\mathrm{2}{f}\left({xy}\right),{x}=\mathrm{1} \\ $$$$\Rightarrow{f}\left(\mathrm{1}\right)^{{y}} +{f}\left({y}\right)=\mathrm{2}{f}\left({y}\right) \\ $$$${f}\left({y}\right)={f}\left(\mathrm{1}\right)^{{y}} =\frac{\mathrm{1}}{\mathrm{2}^{{y}} } \\ $$

Commented by Dwaipayan Shikari last updated on 30/Oct/20

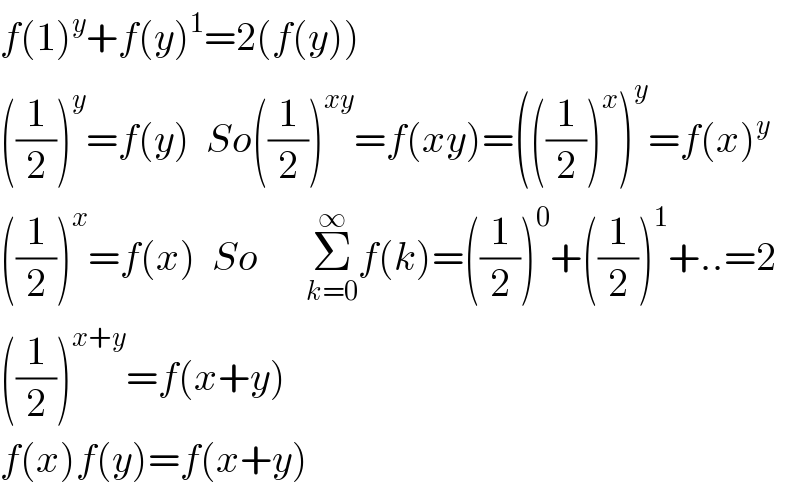
f(1)^y +f(y)^1 =2(f(y)) ((1/2))^y =f(y) So((1/2))^(xy) =f(xy)=(((1/2))^x )^y =f(x)^y ((1/2))^x =f(x) So Σ_(k=0) ^∞ f(k)=((1/2))^0 +((1/2))^1 +..=2 ((1/2))^(x+y) =f(x+y) f(x)f(y)=f(x+y)

$${f}\left(\mathrm{1}\right)^{{y}} +{f}\left({y}\right)^{\mathrm{1}} =\mathrm{2}\left({f}\left({y}\right)\right) \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{y}} ={f}\left({y}\right)\:\:{So}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{xy}} ={f}\left({xy}\right)=\left(\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{x}} \right)^{{y}} ={f}\left({x}\right)^{{y}} \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{x}} ={f}\left({x}\right)\:\:{So}\:\:\:\:\:\:\underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{f}\left({k}\right)=\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{0}} +\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{1}} +..=\mathrm{2} \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{x}+{y}} ={f}\left({x}+{y}\right) \\ $$$${f}\left({x}\right){f}\left({y}\right)={f}\left({x}+{y}\right) \\ $$

Commented by Ar Brandon last updated on 30/Oct/20

Thanks��

Terms of Service

Privacy Policy

Contact: info@tinkutara.com