
Question and Answers Forum
Previous in Differential Equation Next in Differential Equation
Question Number 121633 by liberty last updated on 10/Nov/20
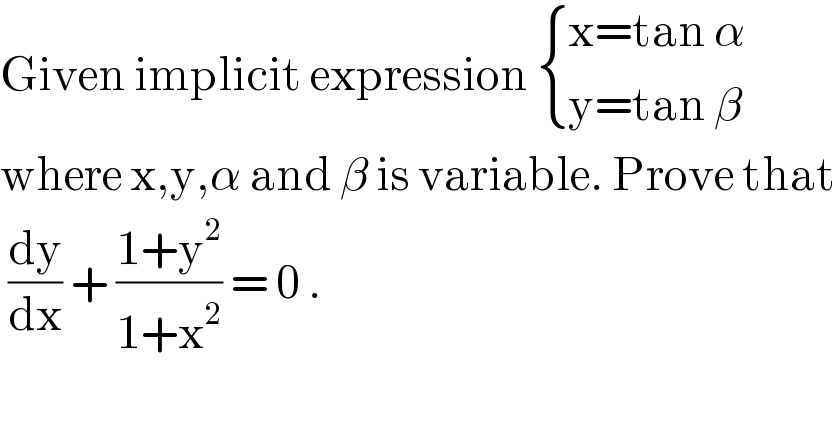
Commented by Dwaipayan Shikari last updated on 10/Nov/20
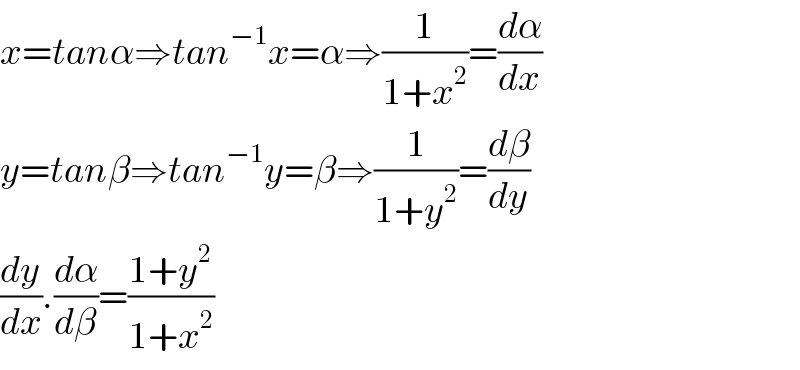
Commented by liberty last updated on 10/Nov/20

Commented by Dwaipayan Shikari last updated on 10/Nov/20
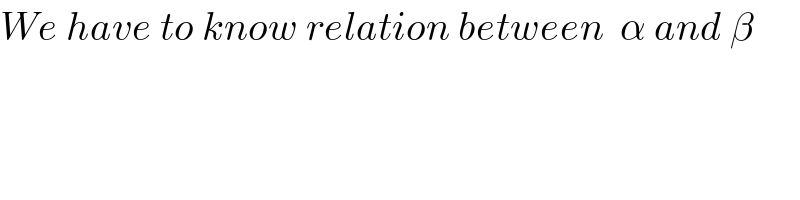
Commented by Dwaipayan Shikari last updated on 10/Nov/20

