Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 123037 by benjo_mathlover last updated on 22/Nov/20

$$\int \frac{\sqrt{1-x}}{1-\sqrt{x}}dx$$$$$$

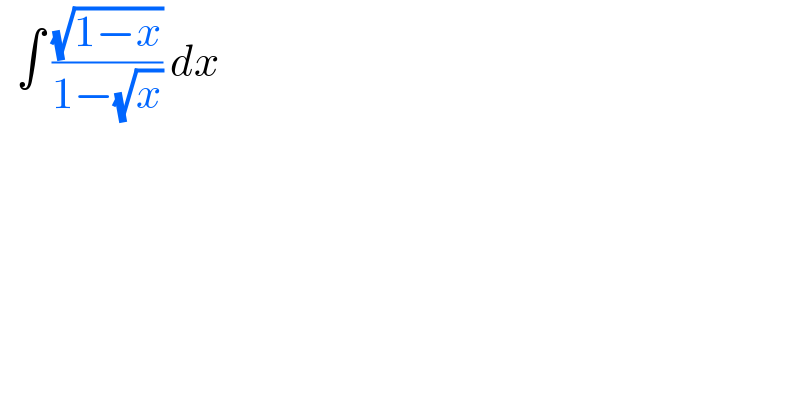
Answered by MJS_new last updated on 22/Nov/20

$$\int \frac{\sqrt{1-x}}{1-\sqrt{x}}dx=$$$$[t=\frac{\sqrt{1-x}}{1-\sqrt{x}}\to dx=2\sqrt{x}(1-\sqrt{x})\sqrt{1-x}]$$$$=8\int \frac{t^2(t^2-1)}{{(t^2+1)}^3}dt=$$$$\left[{\mathrm{Ostrogradski}}^{\prime }\mathrm{s}\mathrm{Method}\right]$$$$=-\frac{2t(3t^2+1)}{{(t^2+1)}^2}+2\int \frac{dt}{t^2+1}=$$$$=-(2+\sqrt{x})\sqrt{1-x}+2\arctan \frac{\sqrt{1-x}}{1-\sqrt{x}}+C$$$$\mathrm{btw}2\arctan \frac{\sqrt{1-x}}{1-\sqrt{x}}=-\arcsin \sqrt{1-x}=-\arccos \sqrt{x}$$

Answered by ajfour last updated on 22/Nov/20

$$letx=\cos {}^{2}2\theta$$$$\Rightarrow dx=-4\sin 2\theta \cos 2\theta d\theta$$$$I=\int \frac{\sin 2\theta }{2\sin {}^2\theta }(-4\sin 2\theta \cos 2\theta )d\theta$$$$=-8\int \cos {}^2\theta (2\cos {}^2\theta -1)d\theta$$$$=-4\int (1+\cos 2\theta )\cos 2\theta d\theta$$$$=-2\sin 2\theta -2\int (1+\cos 4\theta )d\theta$$$$=-2\sin 2\theta -2\theta +\frac{\sin 4\theta }{2}+c$$$$I=-2\sqrt{1-x}-{\cos }^{-1}\sqrt{x}+\sqrt{x-x^2}+c$$

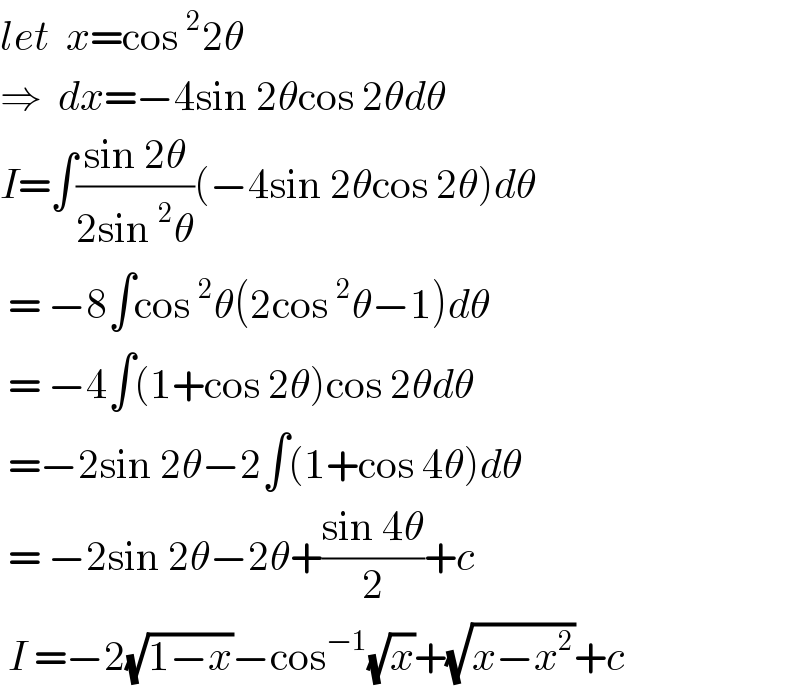
Answered by Dwaipayan Shikari last updated on 22/Nov/20

$$\int \frac{\sqrt{1-x}}{1-\sqrt{x}}dx1-x=t^2\Rightarrow -1=2t\frac{dt}{dx}(1+\sqrt{x})(1-\sqrt{x})=t^2$$$$-\int \frac{2t^{2}dt}{1-\sqrt{1-t^2}}x=1-t^2$$$$=-\int \frac{2t^2(1+\sqrt{1-t^2})}{1-1+t^2}dt$$$$=-2\int (1+\sqrt{1-t^2})dt$$$$=-2t-2\int \sqrt{1-t^2}dt$$$$=-2\sqrt{1-x}-2\int cos\theta \sqrt{1-{sin}^2\theta }d\theta t=sin\theta$$$$=-2\sqrt{1-x}-\theta -\frac{1}{2}sin2\theta$$$$=-2\sqrt{1-x}-{sin}^{-1}\left(\sqrt{1-x}\right)-\sqrt{x-x^2}$$

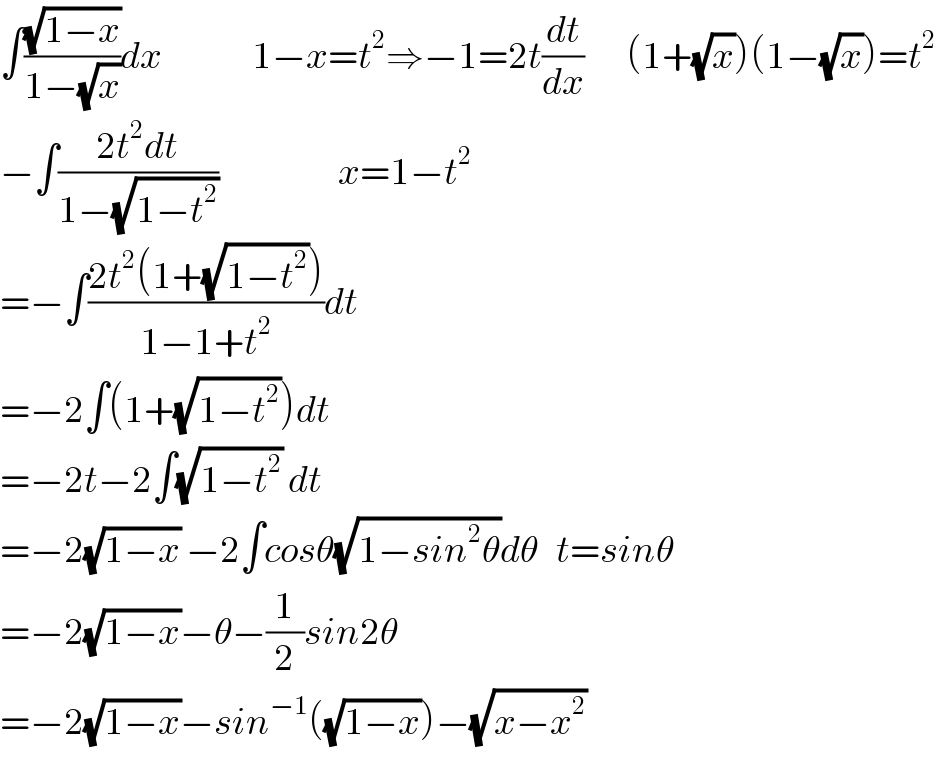
Answered by liberty last updated on 23/Nov/20

$$\lambda \left(x\right)=\int \frac{\sqrt{(1+\sqrt{x})(1-\sqrt{x})}}{1-\sqrt{x}}dx$$$$\lambda \left(x\right)=\int \frac{\sqrt{1+\sqrt{x}}}{\sqrt{1-\sqrt{x}}}dx$$$$let\sqrt{x}=\cos \mathrm{\varnothing },x=\cos {}^2\mathrm{\varnothing },dx=-2\sin \mathrm{\varnothing }\cos \mathrm{\varnothing }d\mathrm{\varnothing }$$$$\lambda \left(x\right)=\int \frac{\sqrt{1+\cos {}^2\mathrm{\varnothing }}(-2\sin \mathrm{\varnothing }\cos \mathrm{\varnothing })d\mathrm{\varnothing }}{\sin \mathrm{\varnothing }}$$$$\lambda \left(x\right)=-2\int \sqrt{2-\sin {}^2\mathrm{\varnothing }}d\left(\sin \mathrm{\varnothing }\right)$$$$let\sin \mathrm{\varnothing }=\sqrt{2}\cos \gamma$$$$\lambda \left(x\right)=-2\int \sqrt{2(1-\cos {}^2\gamma )}d\left(\sqrt{2}\cos \gamma \right)$$$$\lambda \left(x\right)=4\int {\sin }^2\gamma d\gamma =2\int (1-\cos 2\gamma )d\gamma$$$$\lambda \left(x\right)=2(\gamma -\sin \gamma \cos \gamma )+c$$$$\lambda \left(x\right)={2\cos }^{-1}\left(\frac{\sin \mathrm{\varnothing }}{\sqrt{2}}\right)-2\left(\frac{\sin \mathrm{\varnothing }}{\sqrt{2}}\right)\left(\sqrt{1-\left(\frac{\sin {}^2\mathrm{\varnothing }}{2}\right)}\right)+c$$$$\lambda \left(x\right)={2\cos }^{-1}\left(\sqrt{\frac{1-x}{2}}\right)-2\left(\sqrt{\frac{1-x}{2}}\right)\left(\sqrt{\frac{1+x}{2}}\right)+c$$$$\lambda \left(x\right)={2\cos }^{-1}\left(\sqrt{\frac{1-x}{2}}\right)-\sqrt{1-x^2}+c$$

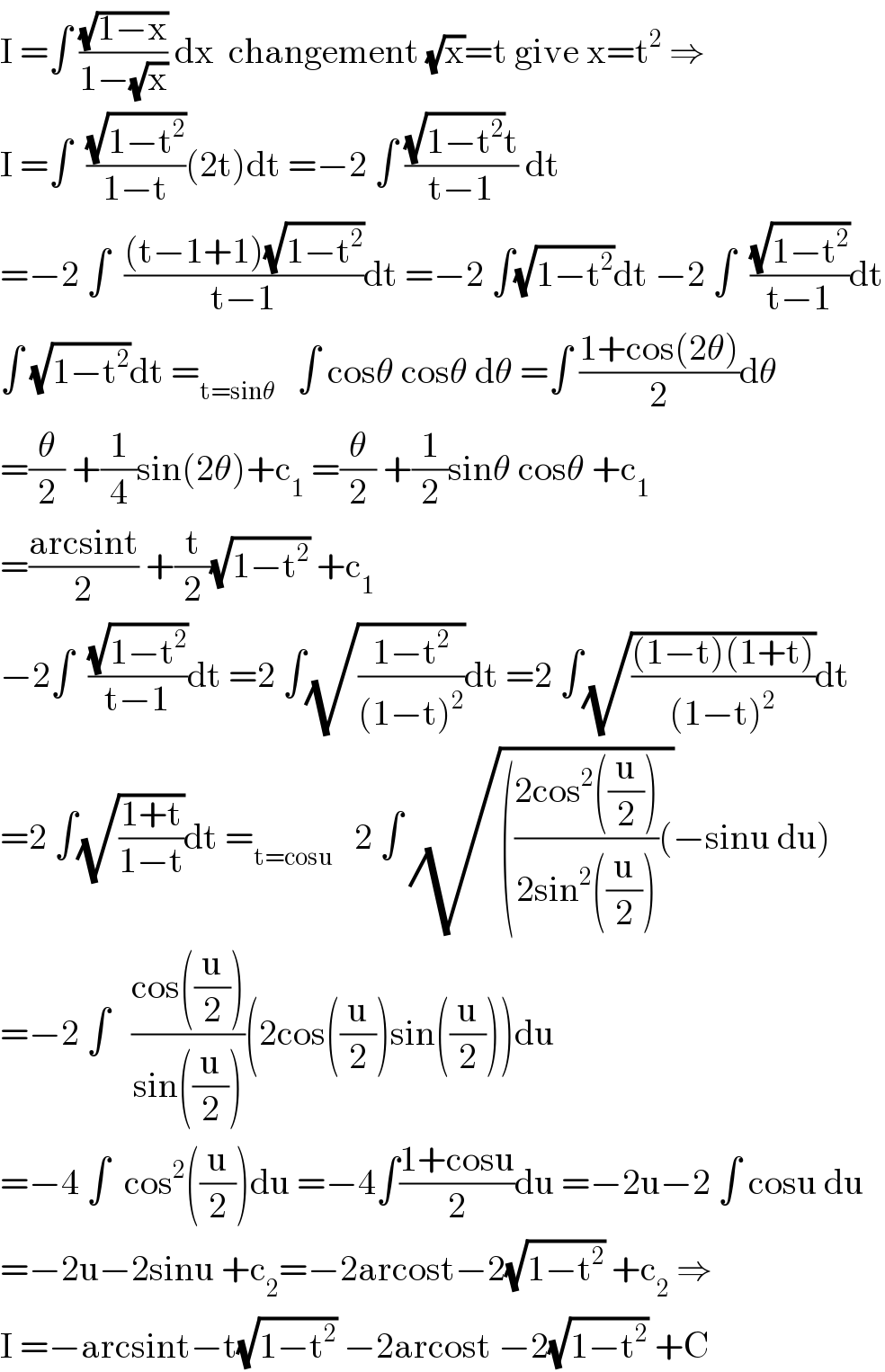
Answered by mathmax by abdo last updated on 22/Nov/20

$$\mathrm{I}=\int \frac{\sqrt{1-\mathrm{x}}}{1-\sqrt{\mathrm{x}}}\mathrm{dx}\mathrm{changement}\sqrt{\mathrm{x}}=\mathrm{t}\mathrm{give}\mathrm{x}={\mathrm{t}}^2\Rightarrow$$$$\mathrm{I}=\int \frac{\sqrt{1-{\mathrm{t}}^2}}{1-\mathrm{t}}\left(2\mathrm{t}\right)\mathrm{dt}=-2\int \frac{\sqrt{1-{\mathrm{t}}^2}\mathrm{t}}{\mathrm{t}-1}\mathrm{dt}$$$$=-2\int \frac{(\mathrm{t}-1+1)\sqrt{1-{\mathrm{t}}^2}}{\mathrm{t}-1}\mathrm{dt}=-2\int \sqrt{1-{\mathrm{t}}^2}\mathrm{dt}-2\int \frac{\sqrt{1-{\mathrm{t}}^2}}{\mathrm{t}-1}\mathrm{dt}$$$$\int \sqrt{1-{\mathrm{t}}^2}\mathrm{dt}{=}_{\mathrm{t}=\sin \theta }\int \cos \theta \cos \theta \mathrm{d}\theta =\int \frac{1+\cos \left(2\theta \right)}{2}\mathrm{d}\theta$$$$=\frac{\theta }{2}+\frac{1}{4}\sin \left(2\theta \right)+{\mathrm{c}}_1=\frac{\theta }{2}+\frac{1}{2}\sin \theta \cos \theta +{\mathrm{c}}_1$$$$=\frac{\mathrm{arcsint}}{2}+\frac{\mathrm{t}}{2}\sqrt{1-{\mathrm{t}}^2}+{\mathrm{c}}_1$$$$-2\int \frac{\sqrt{1-{\mathrm{t}}^2}}{\mathrm{t}-1}\mathrm{dt}=2\int \sqrt{\frac{1-{\mathrm{t}}^2}{{(1-\mathrm{t})}^2}}\mathrm{dt}=2\int \sqrt{\frac{(1-\mathrm{t})(1+\mathrm{t})}{{(1-\mathrm{t})}^2}}\mathrm{dt}$$$$=2\int \sqrt{\frac{1+\mathrm{t}}{1-\mathrm{t}}}\mathrm{dt}{=}_{\mathrm{t}=\mathrm{cosu}}2\int \sqrt{(\frac{{2\cos }^2\left(\frac{\mathrm{u}}{2}\right)}{{2\sin }^2\left(\frac{\mathrm{u}}{2}\right)}(}-\mathrm{sinu}\mathrm{du})$$$$=-2\int \frac{\cos \left(\frac{\mathrm{u}}{2}\right)}{\sin \left(\frac{\mathrm{u}}{2}\right)}\left(2\cos \left(\frac{\mathrm{u}}{2}\right)\sin \left(\frac{\mathrm{u}}{2}\right)\right)\mathrm{du}$$$$=-4\int {\cos }^2\left(\frac{\mathrm{u}}{2}\right)\mathrm{du}=-4\int \frac{1+\mathrm{cosu}}{2}\mathrm{du}=-2\mathrm{u}-2\int \mathrm{cosu}\mathrm{du}$$$$=-2\mathrm{u}-2\mathrm{sinu}+{\mathrm{c}}_2=-2\mathrm{arcost}-2\sqrt{1-{\mathrm{t}}^2}+{\mathrm{c}}_2\Rightarrow$$$$\mathrm{I}=-\mathrm{arcsint}-\mathrm{t}\sqrt{1-{\mathrm{t}}^2}-2\mathrm{arcost}-2\sqrt{1-{\mathrm{t}}^2}+\mathrm{C}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com