Question and Answers Forum
Question Number 122506 by mathace last updated on 17/Nov/20

Answered by TANMAY PANACEA last updated on 17/Nov/20

Commented by mathace last updated on 17/Nov/20
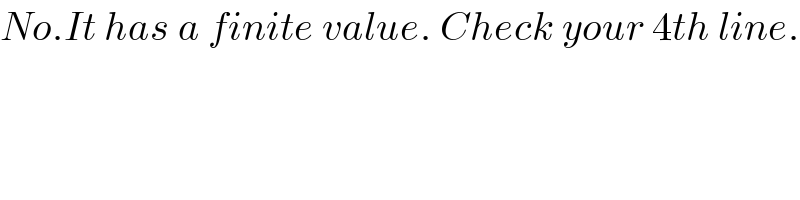
Commented by TANMAY PANACEA last updated on 17/Nov/20

Commented by mindispower last updated on 17/Nov/20

Commented by Dwaipayan Shikari last updated on 18/Nov/20

Commented by mathace last updated on 18/Nov/20

Commented by Dwaipayan Shikari last updated on 18/Nov/20
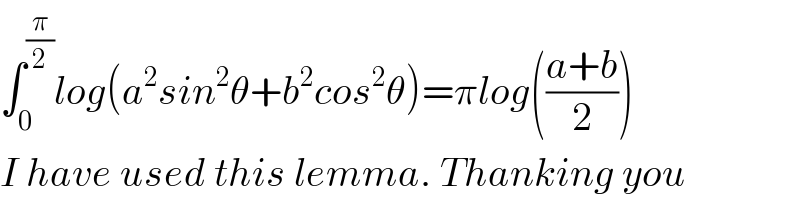
Commented by mnjuly1970 last updated on 18/Nov/20