(d^2 y/dx^2 ) − 4(dy/dx) +11y = e^(−2x) .sin 4x


Question and Answers Forum

All Questions Topic List
Differential Equation Questions
Previous in All Question Next in All Question
Previous in Differential Equation Next in Differential Equation
Question Number 123107 by benjo_mathlover last updated on 23/Nov/20

$\frac{d^{2} y}{{d x}^{2}} - 4 \frac{d y}{d x} + 11 y = e^{- 2 x} . \sin 4 x$
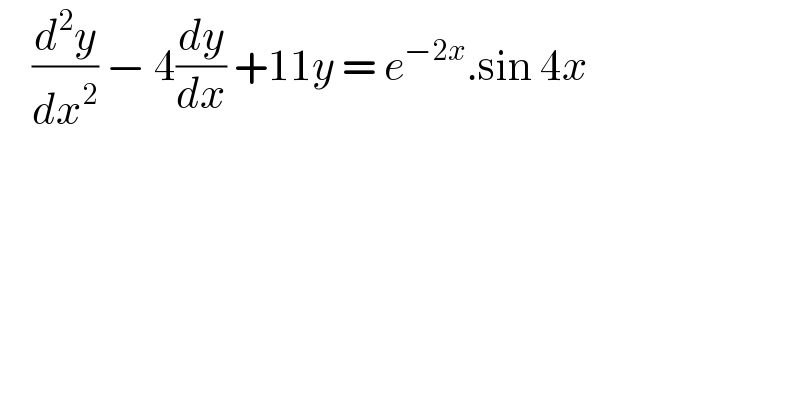
	Answered by mathmax by abdo last updated on 23/Nov/20
	$h→y^(′′) −4y^′ +11y =0→r^2 −4r+11=0 Δ^′ =4−11=−7 ⇒r_1 =2+i(√7) and r_2 =2−i(√7) y_h =ae^((2+i(√7))x) +b e^((2−i(√7))x) =e^(2x) (αcos((√7)x)+β sin((√7)x)) =αu_1 +βu_2 W(u_1 ,u_2 ) = determinant (((e^(2x) cos((√7)x) e^(2x) sin((√7)x))),((e^(2x) (2cos((√7)x)−(√7)sin((√7))x e^(2x) (2sin((√7)x)+(√7)cos((√7)x)))) =e^(4x) {2cos((√7)x)sin((√7)x)+(√7)cos^2 ((√7)x)} −e^(4x) {2sin((√7)x)cos((√7)x)−(√7)sin^2 ((√7)x)} =(√7)e^(4x) ≠0 W_1 = determinant (((0 e^(2x) sin((√7)x))),((e^(−2x) sin(4x) e^(2x) (2sin((√7)x)+(√7)cos((√7)x)))) =−sin(4x)sin((√7)x) W_2 = determinant (((e^(2x) cos((√7)x) 0)),((e^(2x) (2cos((√7)x)−(√7)sin((√7)x) e^(−2x) sin(4x)))) =cos((√7)x)sin(4x) v_1 =∫ (w_1 /w)dx =−∫ ((sin(4x)sin((√7)x))/( (√7)e^(4x) ))dx =(1/( (√7)))∫ e^(−4x) sin(4x)sin((√7)x)dx (eazy to find by use sina.sinb=....) v_2 =∫ (w_2 /w)dx =∫ ((cos((√7)x)sin(4x))/( (√7)e^(4x) ))dx =(1/( (√7)))∫ e^(−4x) cos((√7)x)sin(4x)dx =.....(use trigo.formulae) ⇒y_p =u_1 v_1 +u_2 v_2 the general solution is y =y_h +y_p$
	$h \to y^{^{″}} - {4 y}^{^{'}} + 11 y = 0 \to r^{2} - 4 r + 11 = 0$ $Δ^{^{'}} = 4 - 11 = - 7 \Rightarrow r_{1} = 2 + i \sqrt{7} and r_{2} = 2 - i \sqrt{7}$ $y_{h} = {ae}^{(2 + i \sqrt{7}) x} + b e^{(2 - i \sqrt{7}) x} = e^{2 x} (α \cos (\sqrt{7} x) + β \sin (\sqrt{7} x)) = α u_{1} + β u_{2}$ $W (u_{1}, u_{2}) = \| \begin{matrix} e^{2 x} \cos (\sqrt{7} x) e^{2 x} \sin (\sqrt{7} x) \\ e^{2 x} (2 \cos (\sqrt{7} x) - \sqrt{7} \sin (\sqrt{7}) x e^{2 x} (2 \sin (\sqrt{7} x) + \sqrt{7} \cos (\sqrt{7} x) \end{matrix} \|$ $= e^{4 x} {2 \cos (\sqrt{7} x) \sin (\sqrt{7} x) + \sqrt{7} \cos^{2} (\sqrt{7} x)}$ $- e^{4 x} {2 \sin (\sqrt{7} x) \cos (\sqrt{7} x) - \sqrt{7} \sin^{2} (\sqrt{7} x)}$ $= \sqrt{7} e^{4 x} \neq 0$ $W_{1} = \| \begin{matrix} 0 e^{2 x} \sin (\sqrt{7} x) \\ e^{- 2 x} \sin (4 x) e^{2 x} (2 \sin (\sqrt{7} x) + \sqrt{7} \cos (\sqrt{7} x) \end{matrix} \|$ $= - \sin (4 x) \sin (\sqrt{7} x)$ $W_{2} = \| \begin{matrix} e^{2 x} \cos (\sqrt{7} x) 0 \\ e^{2 x} (2 \cos (\sqrt{7} x) - \sqrt{7} \sin (\sqrt{7} x) e^{- 2 x} \sin (4 x) \end{matrix} \|$ $= \cos (\sqrt{7} x) \sin (4 x)$ $v_{1} = \int \frac{w_{1}}{w} dx = - \int \frac{\sin (4 x) \sin (\sqrt{7} x)}{\sqrt{7} e^{4 x}} dx = \frac{1}{\sqrt{7}} \int e^{- 4 x} \sin (4 x) \sin (\sqrt{7} x) dx$ $(eazy to find by use sina . sinb = . . . .)$ $v_{2} = \int \frac{w_{2}}{w} dx = \int \frac{\cos (\sqrt{7} x) \sin (4 x)}{\sqrt{7} e^{4 x}} dx = \frac{1}{\sqrt{7}} \int e^{- 4 x} \cos (\sqrt{7} x) \sin (4 x) dx$ $= . . . . . (use trigo . formulae) \Rightarrow y_{p} = u_{1} v_{1} + u_{2} v_{2}$ $the general solution is y = y_{h} + y_{p}$
	Commented by peter frank last updated on 24/Nov/20

	$thank you$
	Commented by talminator2856791 last updated on 08/Dec/20

	$how do you make big line like that$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum