Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 123232 by mohammad17 last updated on 24/Nov/20

$$provethatcot5x=\frac{1-10{tan}^{2}x+5{tan}^{4}x}{{tan}^{5}x-10{tan}^{3}x+5tanx}?$$

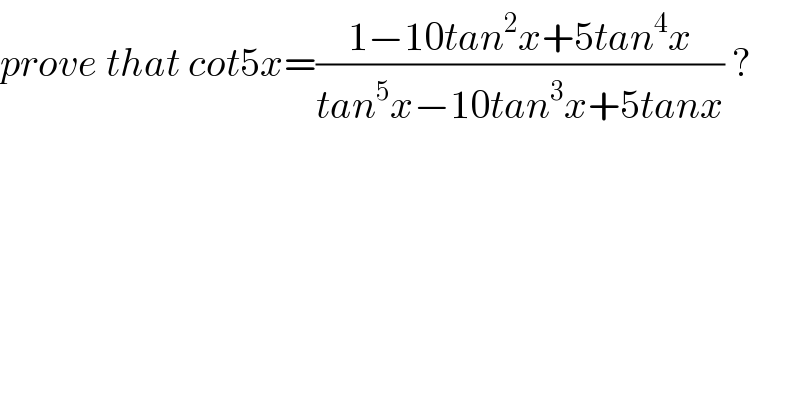
Answered by $@y@m last updated on 24/Nov/20

$$\tan (2x+3x)=\frac{\tan 2x+\tan 3x}{1-\tan 2x.\tan 3x}$$$$\Rightarrow \tan 5x=\frac{\frac{2\tan x}{1-\tan {}^{2}x}+\frac{3\tan x-\tan {}^{3}x}{1-3\tan {}^{2}x}}{1-\left(\frac{2\tan x}{1-\tan {}^{2}x}\right)\left(\frac{3\tan x-\tan {}^{3}x}{1-3\tan {}^{2}x}\right)}$$$$=\frac{2\tan x(1-3\tan {}^{2}x)+(1-\tan {}^{2}x)(3\tan x-\tan {}^{3}x)}{(1-\tan {}^{2}x)(1-3\tan {}^{2}x)-2\tan x(3\tan x-\tan {}^{3}x)}$$$$=\frac{2\tan x-{6\tan }^{3}x+3\tan x-{3\tan }^{3}x-{\tan }^{3}x+{\tan }^{5}x}{1-\tan {}^{2}x-3\tan {}^{2}x+3\tan {}^{4}x-{6\tan }^{2}x+{2\tan }^{4}x}$$$$=\frac{\tan {}^{5}x-10\tan {}^{3}x+5\tan x}{1-10\tan {}^{2}x+5\tan {}^{4}x}$$

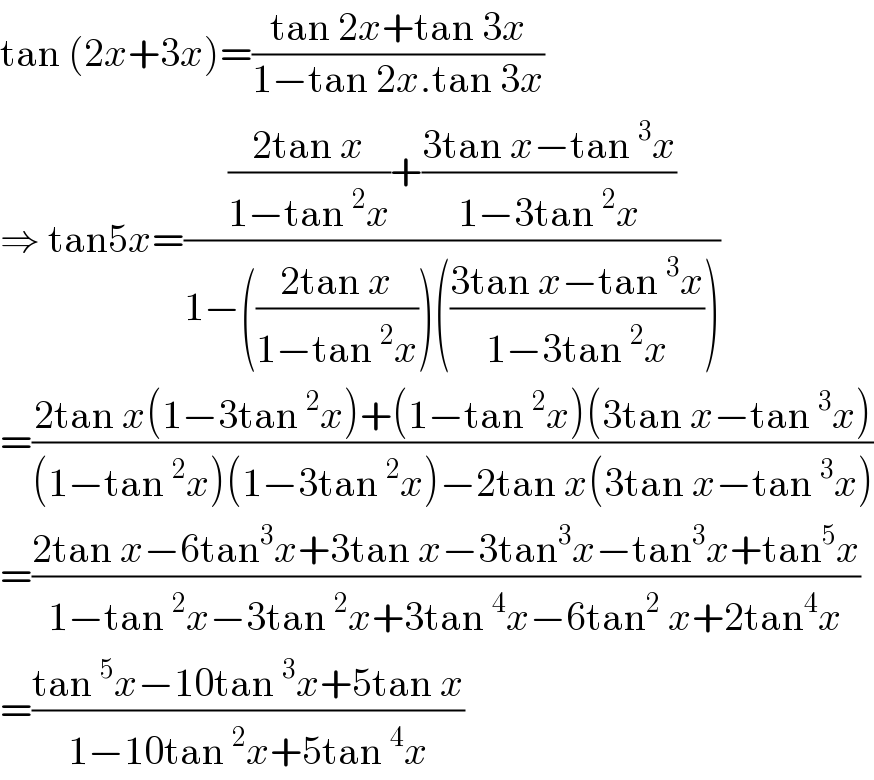
Commented by mohammad17 last updated on 24/Nov/20

$$thankyousir$$

Answered by som(math1967) last updated on 24/Nov/20

$$\cot 5\mathrm{x}$$$$=\frac{1}{\tan (3\mathrm{x}+2\mathrm{x})}=\frac{1-\tan 3\mathrm{xtan}2\mathrm{x}}{\tan 3\mathrm{x}+\tan 2\mathrm{x}}$$$$=\frac{1-\frac{(3\mathrm{tanx}-{\tan }^3\mathrm{x})\left(2\mathrm{tanx}\right)}{(1-{3\tan }^2\mathrm{x})(1-{\tan }^2\mathrm{x})}}{\frac{3\mathrm{tanx}-{\tan }^3\mathrm{x}}{1-{3\tan }^2\mathrm{x}}+\frac{2\mathrm{tanx}}{1-{\tan }^2\mathrm{x}}}$$$$\mathrm{let}\mathrm{tanx}=\mathrm{a}$$$$\frac{(1-{3\mathrm{a}}^2)(1-{\mathrm{a}}^2)-{6\mathrm{a}}^2+{2\mathrm{a}}^4}{(1-{\mathrm{a}}^2)(3\mathrm{a}-{\mathrm{a}}^3)+2\mathrm{a}-{6\mathrm{a}}^3}$$$$=\frac{1-{\mathrm{a}}^2-{3\mathrm{a}}^2+{3\mathrm{a}}^4-{6\mathrm{a}}^2+{2\mathrm{a}}^4}{3\mathrm{a}-{\mathrm{a}}^3-{3\mathrm{a}}^3+{\mathrm{a}}^5+2\mathrm{a}-{6\mathrm{a}}^3}$$$$=\frac{1-{10\mathrm{a}}^2+{5\mathrm{a}}^4}{5\mathrm{a}-{10\mathrm{a}}^3+{\mathrm{a}}^5}$$$$\mathrm{now}\mathrm{put}\mathrm{a}=\mathrm{tanx}$$

Commented by mohammad17 last updated on 24/Nov/20

$$thankyousir$$

Answered by TANMAY PANACEA last updated on 24/Nov/20

$$tan\left(nx\right)=\frac{s_1-s_3+s_5-s_7+...}{1-s_2+s_4-s_6+..}$$$$tan5x=\frac{s_1-s_3+s_5}{1-s_2+s_4}$$$$s_1=5C_{1}tanx=5tanx$$$$s_3=5C_3{tan}^{3}x=\frac{5\times 4}{2}{tan}^{3}x=10{tan}^{3}x$$$$s_5=5C_5{tan}^{5}x={tan}^{5}x$$$$s_2=5c_2{tan}^{2}x=10{tan}^{2}x$$$$s_4=5c_4{tan}^{4}x=5{tan}^{4}x$$$$cot5x=\frac{1}{tan5x}=\frac{1-10{tan}^{2}x+5{tan}^{4}x}{5tanx-10{tan}^{3}x+{tan}^{5}x}$$

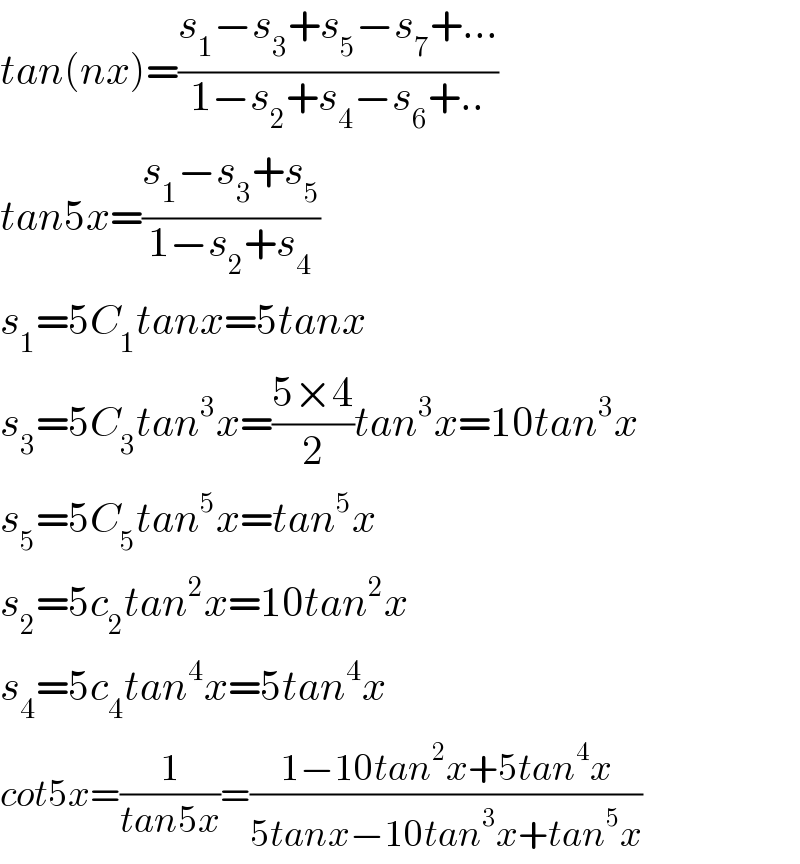
Commented by som(math1967) last updated on 25/Nov/20

$$\mathrm{Nice}\mathrm{sir}$$

Commented by som(math1967) last updated on 25/Nov/20

খুব ভাল

Commented by TANMAY PANACEA last updated on 25/Nov/20

$$thankyou...$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com