What is the shortest distance between two parabolas y^2 = x−2 and x^2 = y−2 .


Question and Answers Forum

All Questions Topic List
Differentiation Questions
Previous in All Question Next in All Question
Previous in Differentiation Next in Differentiation
Question Number 123243 by benjo_mathlover last updated on 24/Nov/20

$W h a t i s t h e s h o r t e s t d i s t a n c e$ $b e t w e e n t w o p a r a b o l a s$ $y^{2} = x - 2 a n d x^{2} = y - 2 .$
Commented by liberty last updated on 24/Nov/20
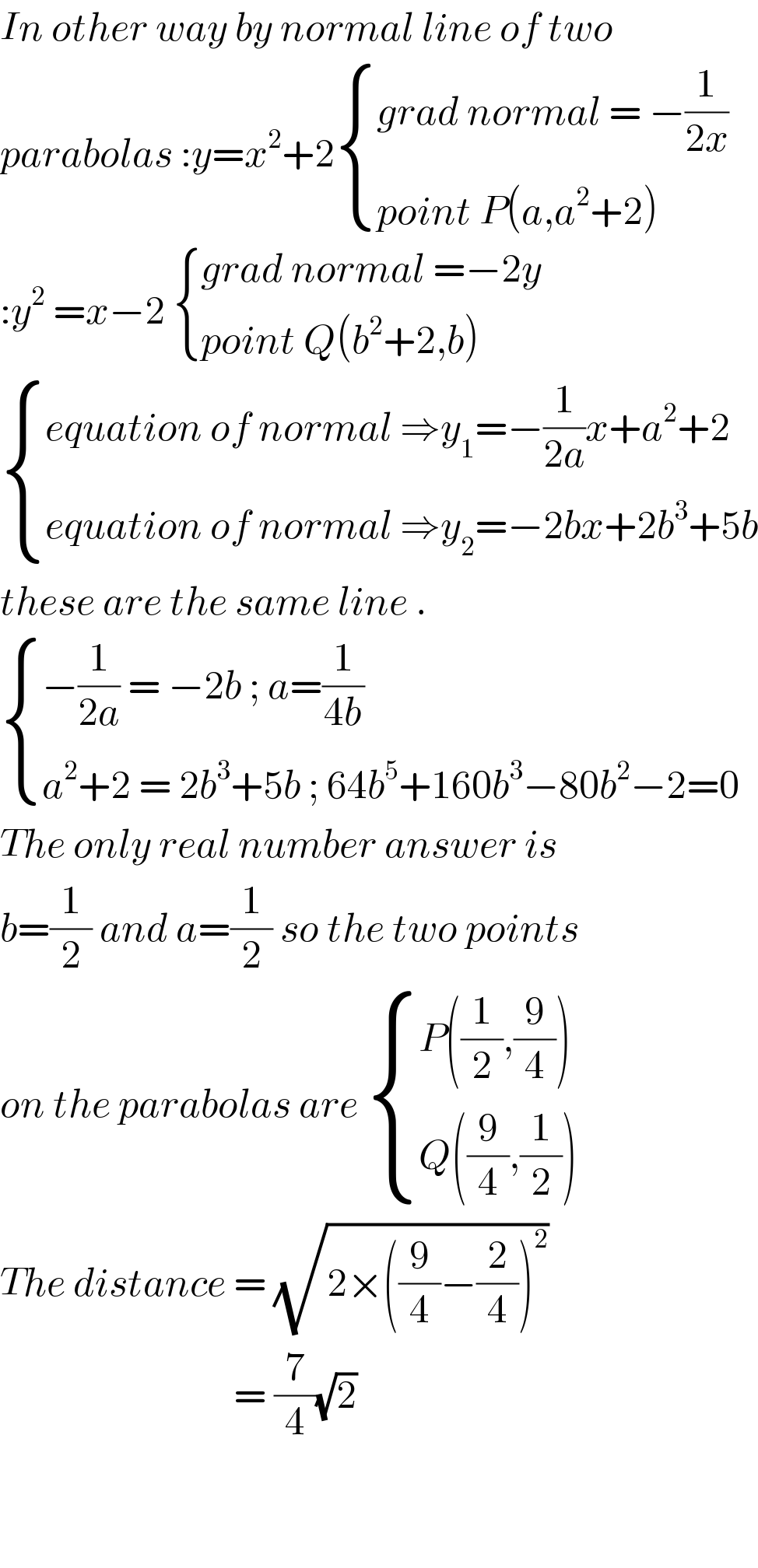
$In other way by normal line of two parabolas :y=x^2 +2 { ((grad normal = −(1/(2x)))),((point P(a,a^2 +2))) :} :y^2 =x−2 { ((grad normal =−2y)),((point Q(b^2 +2,b) )) :} { ((equation of normal ⇒y_1 =−(1/(2a))x+a^2 +2)),((equation of normal ⇒y_2 =−2bx+2b^3 +5b)) :} these are the same line . { ((−(1/(2a)) = −2b ; a=(1/(4b)))),((a^2 +2 = 2b^3 +5b ; 64b^5 +160b^3 −80b^2 −2=0)) :} The only real number answer is b=(1/2) and a=(1/2) so the two points on the parabolas are { ((P((1/2),(9/4)))),((Q((9/4),(1/2)))) :} The distance = (√(2×((9/4)−(2/4))^2 )) = (7/4)(√2)$
$I n o t h e r w a y b y n o r m a l l i n e o f t w o$ $p a r a b o l a s : y = x^{2} + 2 {\begin{cases} g r a d n o r m a l = - \frac{1}{2 x} \\ p o i n t P (a, a^{2} + 2) \end{cases}$ $: y^{2} = x - 2 {\begin{cases} g r a d n o r m a l = - 2 y \\ p o i n t Q (b^{2} + 2, b) \end{cases}$ ${\begin{cases} e q u a t i o n o f n o r m a l \Rightarrow y_{1} = - \frac{1}{2 a} x + a^{2} + 2 \\ e q u a t i o n o f n o r m a l \Rightarrow y_{2} = - 2 b x + 2 b^{3} + 5 b \end{cases}$ $t h e s e a r e t h e s a m e l i n e .$ ${\begin{cases} - \frac{1}{2 a} = - 2 b; a = \frac{1}{4 b} \\ a^{2} + 2 = 2 b^{3} + 5 b; 64 b^{5} + 160 b^{3} - 80 b^{2} - 2 = 0 \end{cases}$ $T h e o n l y r e a l n u m b e r a n s w e r i s$ $b = \frac{1}{2} a n d a = \frac{1}{2} s o t h e t w o p o i n t s$ $o n t h e p a r a b o l a s a r e {\begin{cases} P (\frac{1}{2}, \frac{9}{4}) \\ Q (\frac{9}{4}, \frac{1}{2}) \end{cases}$ $T h e d i s t a n c e = \sqrt{2 \times {(\frac{9}{4} - \frac{2}{4})}^{2}}$ $= \frac{7}{4} \sqrt{2}$
Commented by benjo_mathlover last updated on 24/Nov/20

Commented by benjo_mathlover last updated on 24/Nov/20

$c o r r e c t . t h a n k y o u$
	Answered by bobhans last updated on 24/Nov/20

	$(∙) y^{2} = x - 2 (∙) x^{2} = y - 2$ $2 y . y^{'} = 1 2 x = y^{'}$ $g r a d y^{'} = \frac{1}{2 y} = \frac{1}{2 a} g r a d y^{'} = 2 x = 2 b$ $P o i n t A (a^{2} + 2, a) P o i n t B (b, b^{2} + 2)$ $t a n g e n t l i n e t a n g e n t l i n e$ $y = \frac{1}{2 a} (x - (a^{2} + 2)) + a y = 2 b (x - b) + b^{2} + 2$ $t w o t a n g e n t l i n e a r e p a r a l l e l$ $\Rightarrow \frac{1}{2 a} = 2 b \Leftrightarrow b = \frac{1}{4 a}$ $s o t h e s h o r t e s t d i s t a n c e i s$ $∣ A B ∣ = \sqrt{{(a^{2} + 2 - b)}^{2} + {(a - (b^{2} + 2))}^{2}}$ $= \sqrt{{(a^{2} + 2 - \frac{1}{4 a})}^{2} + {(a - \frac{1}{16 a^{2}} - 2)}^{2}}$ $= \sqrt{{(\frac{4 a^{3} + 8 a - 1}{4 a})}^{2} + {(\frac{16 a^{3} - 32 a^{2} - 1}{16 a^{2}})}^{2}}$ $w e c o m p u t e \frac{d ∣ A B ∣}{d a} = 0$ $w e g e t a = \frac{1}{2} \land b = \frac{1}{2}$ $∣ A B ∣_{m i n} = \sqrt{{(\frac{1}{4} + 2 - \frac{1}{2})}^{2} + {(\frac{1}{2} - \frac{1}{4} - 2)}^{2}}$ $= \sqrt{2 {(\frac{9}{4} - \frac{2}{4})}^{2}} = \frac{7 \sqrt{2}}{4} .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum