Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 1239 by Rasheed Ahmad last updated on 17/Jul/15

Rasheed Ahmad (Rasheed Soomro) •For f(x) where x and f(x) both are real the (x,f(x)) can be plotted as a point easily. •Now consider F(X) where X and F(X) are complex numbers. How can (X,F(X)) be plotted? For a particular example: (3+2i,4−5i) how can be plotted?

$${Rasheed}\:{Ahmad}\:\left({Rasheed}\:{Soomro}\right) \\ $$$$\bullet\mathrm{For}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{where}\:\mathrm{x}\:\mathrm{and}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{both}\:{are} \\ $$$$\mathrm{real}\:\mathrm{the}\:\left(\mathrm{x},{f}\left({x}\right)\right)\:{can}\:{be}\:{plotted}\:{as} \\ $$$${a}\:{point}\:{easily}.\:\bullet{Now}\:{consider}\:{F}\left({X}\right) \\ $$$${where}\:{X}\:{and}\:{F}\left({X}\right)\:{are}\:{complex}\: \\ $$$${numbers}.\:{How}\:{can}\:\left({X},{F}\left({X}\right)\right)\:{be} \\ $$$${plotted}?\:{For}\:{a}\:{particular}\:{example}:\:\left(\mathrm{3}+\mathrm{2}{i},\mathrm{4}−\mathrm{5}{i}\right) \\ $$$${how}\:{can}\:{be}\:{plotted}? \\ $$$$ \\ $$$$ \\ $$

Commented by 123456 last updated on 18/Jul/15

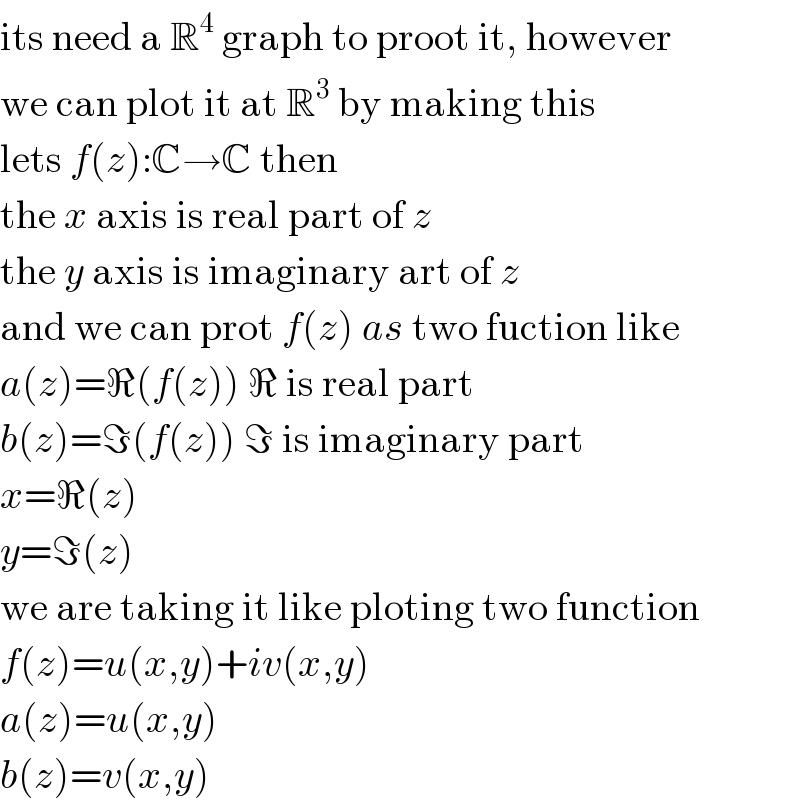
its need a R^4 graph to proot it, however we can plot it at R^3 by making this lets f(z):C→C then the x axis is real part of z the y axis is imaginary art of z and we can prot f(z) as two fuction like a(z)=ℜ(f(z)) ℜ is real part b(z)=ℑ(f(z)) ℑ is imaginary part x=ℜ(z) y=ℑ(z) we are taking it like ploting two function f(z)=u(x,y)+iv(x,y) a(z)=u(x,y) b(z)=v(x,y)

$$\mathrm{its}\:\mathrm{need}\:\mathrm{a}\:\mathbb{R}^{\mathrm{4}} \:\mathrm{graph}\:\mathrm{to}\:\mathrm{proot}\:\mathrm{it},\:\mathrm{however} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{plot}\:\mathrm{it}\:\mathrm{at}\:\mathbb{R}^{\mathrm{3}} \:\mathrm{by}\:\mathrm{making}\:\mathrm{this} \\ $$$$\mathrm{lets}\:{f}\left({z}\right):\mathbb{C}\rightarrow\mathbb{C}\:\mathrm{then} \\ $$$$\mathrm{the}\:{x}\:\mathrm{axis}\:\mathrm{is}\:\mathrm{real}\:\mathrm{part}\:\mathrm{of}\:{z} \\ $$$$\mathrm{the}\:{y}\:\mathrm{axis}\:\mathrm{is}\:\mathrm{imaginary}\:\mathrm{art}\:\mathrm{of}\:{z} \\ $$$$\mathrm{and}\:\mathrm{we}\:\mathrm{can}\:\mathrm{prot}\:{f}\left({z}\right)\:{as}\:\mathrm{two}\:\mathrm{fuction}\:\mathrm{like} \\ $$$${a}\left({z}\right)=\Re\left({f}\left({z}\right)\right)\:\Re\:\mathrm{is}\:\mathrm{real}\:\mathrm{part} \\ $$$${b}\left({z}\right)=\Im\left({f}\left({z}\right)\right)\:\Im\:\mathrm{is}\:\mathrm{imaginary}\:\mathrm{part} \\ $$$${x}=\Re\left({z}\right) \\ $$$${y}=\Im\left({z}\right) \\ $$$$\mathrm{we}\:\mathrm{are}\:\mathrm{taking}\:\mathrm{it}\:\mathrm{like}\:\mathrm{ploting}\:\mathrm{two}\:\mathrm{function} \\ $$$${f}\left({z}\right)={u}\left({x},{y}\right)+{iv}\left({x},{y}\right) \\ $$$${a}\left({z}\right)={u}\left({x},{y}\right) \\ $$$${b}\left({z}\right)={v}\left({x},{y}\right) \\ $$

Commented by Rasheed Soomro last updated on 18/Jul/15

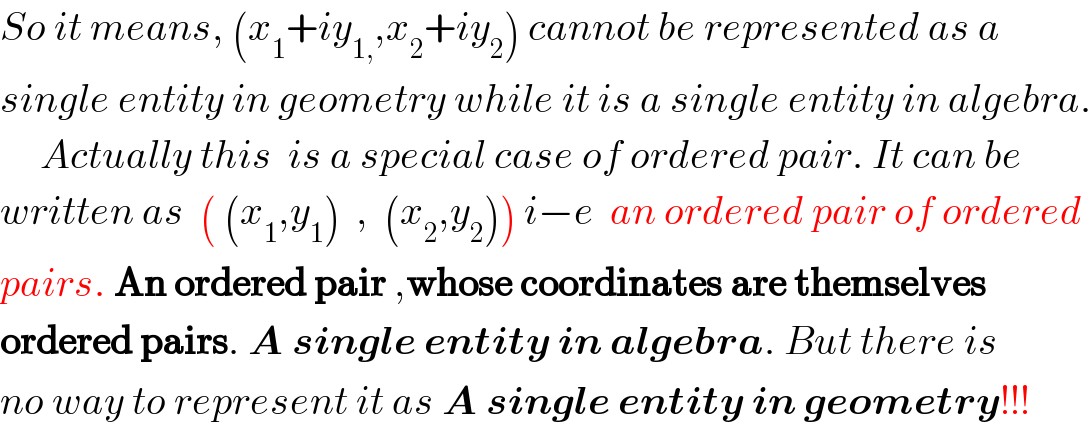
So it means, (x_1 +iy_(1,) ,x_2 +iy_2 ) cannot be represented as a single entity in geometry while it is a single entity in algebra. Actually this is a special case of ordered pair. It can be written as ( (x_1 ,y_1 ) , (x_2 ,y_2 )) i−e an ordered pair of ordered pairs. An ordered pair ,whose coordinates are themselves ordered pairs. A single entity in algebra. But there is no way to represent it as A single entity in geometry!!!

$${So}\:{it}\:{means},\:\left({x}_{\mathrm{1}} +{iy}_{\mathrm{1},} ,{x}_{\mathrm{2}} +{iy}_{\mathrm{2}} \right)\:{cannot}\:{be}\:{represented}\:{as}\:{a} \\ $$$${single}\:{entity}\:{in}\:{geometry}\:{while}\:{it}\:{is}\:{a}\:{single}\:{entity}\:{in}\:{algebra}. \\ $$$$\:\:\:\:\:{Actually}\:{this}\:\:{is}\:{a}\:{special}\:{case}\:{of}\:{ordered}\:{pair}.\:{It}\:{can}\:{be}\: \\ $$$${written}\:{as}\:\:\left(\:\left({x}_{\mathrm{1}} ,{y}_{\mathrm{1}} \right)\:\:,\:\:\left({x}_{\mathrm{2}} ,{y}_{\mathrm{2}} \right)\right)\:{i}−{e}\:\:{an}\:{ordered}\:{pair}\:{of}\:{ordered} \\ $$$${pairs}.\:\boldsymbol{\mathrm{An}}\:\boldsymbol{\mathrm{ordered}}\:\boldsymbol{\mathrm{pair}}\:,\boldsymbol{\mathrm{whose}}\:\boldsymbol{\mathrm{coordinates}}\:\boldsymbol{\mathrm{are}}\:\boldsymbol{\mathrm{themselves}}\: \\ $$$$\boldsymbol{\mathrm{ordered}}\:\boldsymbol{\mathrm{pairs}}.\:\boldsymbol{{A}}\:\boldsymbol{{single}}\:\boldsymbol{{entity}}\:\boldsymbol{{in}}\:\boldsymbol{{algebra}}.\:{But}\:{there}\:{is} \\ $$$${no}\:{way}\:{to}\:{represent}\:{it}\:{as}\:\boldsymbol{{A}}\:\boldsymbol{{single}}\:\boldsymbol{{entity}}\:\boldsymbol{{in}}\:\boldsymbol{{geometry}}!!! \\ $$

Commented by 123456 last updated on 18/Jul/15

you can use the idea of vector fields since complex numbers are similiar to it then you can plot it in R^2 x=ℜ(z) real part y=ℑ(z) im part then every point of it has a vector f(z)=u(z)+iv(z) u(x,y)+iv(x,y) u^→ (z)=u(z)e_x ^→ +v(z)e_y ^→ u^→ (x,y) u(x,y)e_x ^→ +v(x,y)e_y ^→

$$\mathrm{you}\:\mathrm{can}\:\mathrm{use}\:\mathrm{the}\:\mathrm{idea}\:\mathrm{of}\:\mathrm{vector}\:\mathrm{fields} \\ $$$$\mathrm{since}\:\mathrm{complex}\:\mathrm{numbers}\:\mathrm{are}\:\mathrm{similiar} \\ $$$$\mathrm{to}\:\mathrm{it} \\ $$$$\mathrm{then}\:\mathrm{you}\:\mathrm{can}\:\mathrm{plot}\:\mathrm{it}\:\mathrm{in}\:\mathbb{R}^{\mathrm{2}} \\ $$$${x}=\Re\left({z}\right)\:\:\:\mathrm{real}\:\mathrm{part} \\ $$$${y}=\Im\left({z}\right)\:\:\:\:\mathrm{im}\:\mathrm{part} \\ $$$$\mathrm{then}\:\mathrm{every}\:\mathrm{point}\:\mathrm{of}\:\mathrm{it}\:\mathrm{has}\:\mathrm{a}\:\mathrm{vector} \\ $$$${f}\left({z}\right)={u}\left({z}\right)+{iv}\left({z}\right) \\ $$$${u}\left({x},{y}\right)+{iv}\left({x},{y}\right) \\ $$$$\overset{\rightarrow} {{u}}\left({z}\right)={u}\left({z}\right)\overset{\rightarrow} {{e}}_{{x}} +{v}\left({z}\right)\overset{\rightarrow} {{e}}_{{y}} \\ $$$$\overset{\rightarrow} {{u}}\left({x},{y}\right)\:\:\:\:\:\:{u}\left({x},{y}\right)\overset{\rightarrow} {{e}}_{{x}} +{v}\left({x},{y}\right)\overset{\rightarrow} {{e}}_{{y}} \\ $$

Commented by Rasheed Soomro last updated on 18/Jul/15

Thanks Sir (123456)

$$\:\:\:\:\:\:\:\:\:\:{Thanks}\:{Sir}\:\left(\mathrm{123456}\right)\:\:\:\:\: \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com