Question and Answers Forum

All Questions      Topic List

Differential Equation Questions

Previous in All Question      Next in All Question      

Previous in Differential Equation      Next in Differential Equation      

Question Number 123982 by Ar Brandon last updated on 29/Nov/20

Consider the D.E (E): x(x^2 +1)y′−2y=x^3 (x−1)^2 e^(−x) a\ Resolve the DE x(x^3 +1)y′−2y=0 b\We wish to find a function g(x) such that the function h(x) defined by h(x)=(x^3 /(x^2 +1))g(x) is a particular solution of the equation (E) i\ Show that for it to be as such, we need to have g′(x)=(x−1)^2 e^(−x) ii\ Determine the real numbers α, β, and γ such that the function x→αx^2 +βx+γ is a primitive in the interval ]0,+∞[ of the function x→(x−1)^2 e^(−x) iii\ Deduce a particular solution of the equation (E) then the general solution of the equation (E)

$$\mathrm{Consider}\:\mathrm{the}\:\mathrm{D}.\mathrm{E}\:\left(\mathrm{E}\right):\:\mathrm{x}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\mathrm{y}'−\mathrm{2y}=\mathrm{x}^{\mathrm{3}} \left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \\ $$$$\mathrm{a}\backslash\:\mathrm{Resolve}\:\mathrm{the}\:\mathrm{DE}\:\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)\mathrm{y}'−\mathrm{2y}=\mathrm{0} \\ $$$$\mathrm{b}\backslash\mathrm{We}\:\mathrm{wish}\:\mathrm{to}\:\mathrm{find}\:\mathrm{a}\:\mathrm{function}\:\mathrm{g}\left(\mathrm{x}\right)\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{function} \\ $$$$\mathrm{h}\left(\mathrm{x}\right)\:\mathrm{defined}\:\mathrm{by}\:\mathrm{h}\left(\mathrm{x}\right)=\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\mathrm{g}\left(\mathrm{x}\right) \\ $$$$\mathrm{is}\:\mathrm{a}\:\mathrm{particular}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:\left(\mathrm{E}\right) \\ $$$$\mathrm{i}\backslash\:\mathrm{Show}\:\mathrm{that}\:\mathrm{for}\:\mathrm{it}\:\mathrm{to}\:\mathrm{be}\:\mathrm{as}\:\mathrm{such},\:\mathrm{we}\:\mathrm{need}\:\mathrm{to}\:\mathrm{have} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{g}'\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \\ $$$$\mathrm{ii}\backslash\:\mathrm{Determine}\:\mathrm{the}\:\mathrm{real}\:\mathrm{numbers}\:\alpha,\:\beta,\:\mathrm{and}\:\gamma\:\mathrm{such}\:\mathrm{that} \\ $$$$\mathrm{the}\:\mathrm{function}\:\mathrm{x}\rightarrow\alpha\mathrm{x}^{\mathrm{2}} +\beta\mathrm{x}+\gamma\:\mathrm{is}\:\mathrm{a}\:\mathrm{primitive}\:\mathrm{in}\:\mathrm{the}\:\mathrm{interval} \\ $$$$\left.\right]\mathrm{0},+\infty\left[\:\mathrm{of}\:\mathrm{the}\:\mathrm{function}\:\mathrm{x}\rightarrow\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \right. \\ $$$$\mathrm{iii}\backslash\:\mathrm{Deduce}\:\mathrm{a}\:\mathrm{particular}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:\left(\mathrm{E}\right)\:\mathrm{then} \\ $$$$\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:\left(\mathrm{E}\right) \\ $$$$ \\ $$

Answered by mathmax by abdo last updated on 29/Nov/20

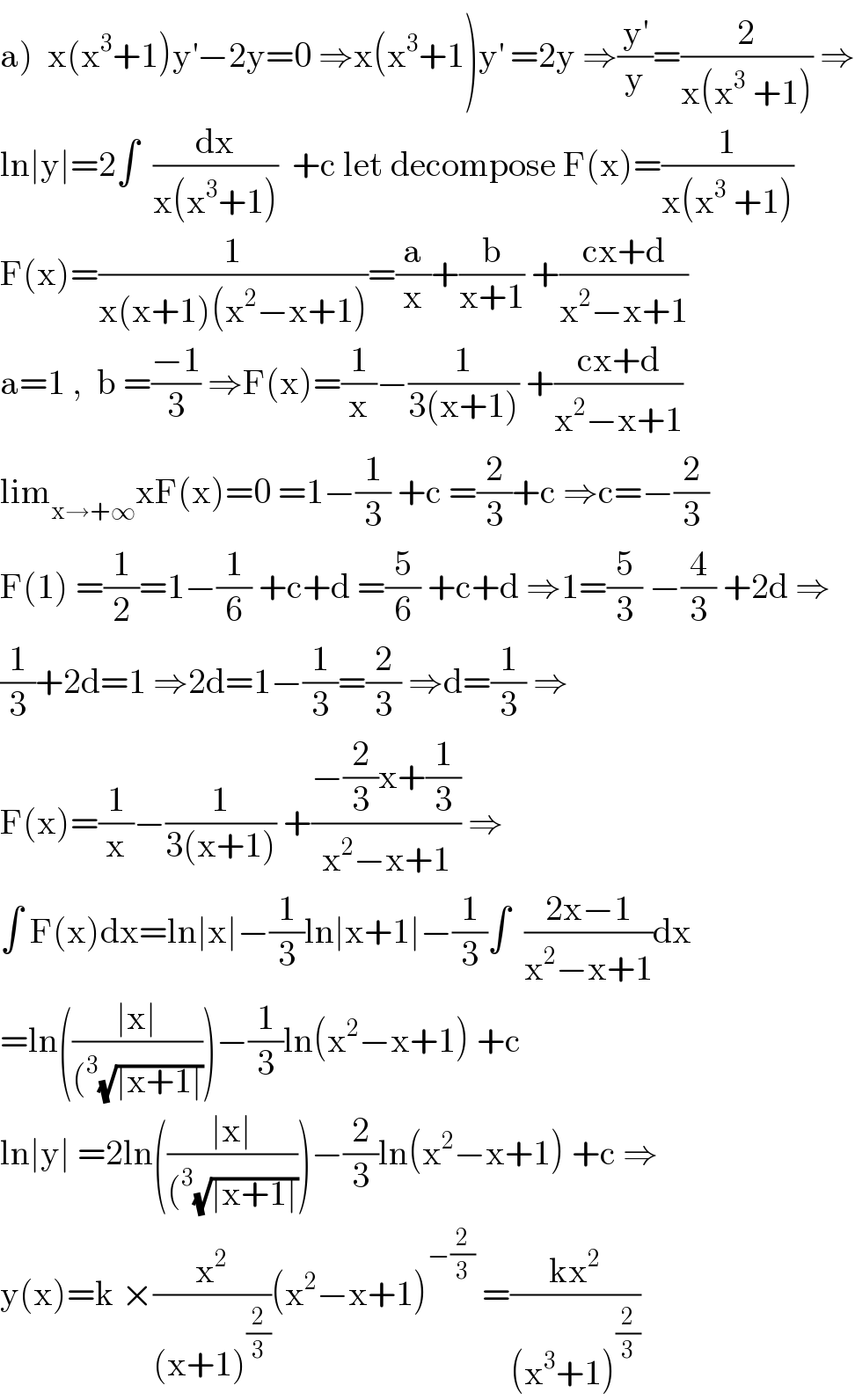
a) x(x^3 +1)y^′ −2y=0 ⇒x(x^3 +1)y^′ =2y ⇒(y^′ /y)=(2/(x(x^3 +1))) ⇒ ln∣y∣=2∫ (dx/(x(x^3 +1))) +c let decompose F(x)=(1/(x(x^3 +1))) F(x)=(1/(x(x+1)(x^2 −x+1)))=(a/x)+(b/(x+1)) +((cx+d)/(x^2 −x+1)) a=1 , b =((−1)/3) ⇒F(x)=(1/x)−(1/(3(x+1))) +((cx+d)/(x^2 −x+1)) lim_(x→+∞) xF(x)=0 =1−(1/3) +c =(2/3)+c ⇒c=−(2/3) F(1) =(1/2)=1−(1/6) +c+d =(5/6) +c+d ⇒1=(5/3) −(4/3) +2d ⇒ (1/3)+2d=1 ⇒2d=1−(1/3)=(2/3) ⇒d=(1/3) ⇒ F(x)=(1/x)−(1/(3(x+1))) +((−(2/3)x+(1/3))/(x^2 −x+1)) ⇒ ∫ F(x)dx=ln∣x∣−(1/3)ln∣x+1∣−(1/3)∫ ((2x−1)/(x^2 −x+1))dx =ln(((∣x∣)/((^3 (√(∣x+1∣)))))−(1/3)ln(x^2 −x+1) +c ln∣y∣ =2ln(((∣x∣)/((^3 (√(∣x+1∣)))))−(2/3)ln(x^2 −x+1) +c ⇒ y(x)=k ×(x^2 /((x+1)^(2/3) ))(x^2 −x+1)^(−(2/3)) =((kx^2 )/((x^3 +1)^(2/3) ))

$$\left.\mathrm{a}\right)\:\:\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)\mathrm{y}^{'} −\mathrm{2y}=\mathrm{0}\:\Rightarrow\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)\mathrm{y}^{'} \:=\mathrm{2y}\:\Rightarrow\frac{\mathrm{y}^{'} }{\mathrm{y}}=\frac{\mathrm{2}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} \:+\mathrm{1}\right)}\:\Rightarrow \\ $$$$\mathrm{ln}\mid\mathrm{y}\mid=\mathrm{2}\int\:\:\frac{\mathrm{dx}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)}\:\:+\mathrm{c}\:\mathrm{let}\:\mathrm{decompose}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} \:+\mathrm{1}\right)} \\ $$$$\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}\left(\mathrm{x}+\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)}=\frac{\mathrm{a}}{\mathrm{x}}+\frac{\mathrm{b}}{\mathrm{x}+\mathrm{1}}\:+\frac{\mathrm{cx}+\mathrm{d}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}} \\ $$$$\mathrm{a}=\mathrm{1}\:,\:\:\mathrm{b}\:=\frac{−\mathrm{1}}{\mathrm{3}}\:\Rightarrow\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{3}\left(\mathrm{x}+\mathrm{1}\right)}\:+\frac{\mathrm{cx}+\mathrm{d}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}} \\ $$$$\mathrm{lim}_{\mathrm{x}\rightarrow+\infty} \mathrm{xF}\left(\mathrm{x}\right)=\mathrm{0}\:=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}\:+\mathrm{c}\:=\frac{\mathrm{2}}{\mathrm{3}}+\mathrm{c}\:\Rightarrow\mathrm{c}=−\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\mathrm{F}\left(\mathrm{1}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{6}}\:+\mathrm{c}+\mathrm{d}\:=\frac{\mathrm{5}}{\mathrm{6}}\:+\mathrm{c}+\mathrm{d}\:\Rightarrow\mathrm{1}=\frac{\mathrm{5}}{\mathrm{3}}\:−\frac{\mathrm{4}}{\mathrm{3}}\:+\mathrm{2d}\:\Rightarrow \\ $$$$\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{2d}=\mathrm{1}\:\Rightarrow\mathrm{2d}=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{2}}{\mathrm{3}}\:\Rightarrow\mathrm{d}=\frac{\mathrm{1}}{\mathrm{3}}\:\Rightarrow \\ $$$$\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{3}\left(\mathrm{x}+\mathrm{1}\right)}\:+\frac{−\frac{\mathrm{2}}{\mathrm{3}}\mathrm{x}+\frac{\mathrm{1}}{\mathrm{3}}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\:\Rightarrow \\ $$$$\int\:\mathrm{F}\left(\mathrm{x}\right)\mathrm{dx}=\mathrm{ln}\mid\mathrm{x}\mid−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{ln}\mid\mathrm{x}+\mathrm{1}\mid−\frac{\mathrm{1}}{\mathrm{3}}\int\:\:\frac{\mathrm{2x}−\mathrm{1}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\mathrm{dx} \\ $$$$=\mathrm{ln}\left(\frac{\mid\mathrm{x}\mid}{\left(^{\mathrm{3}} \sqrt{\mid\mathrm{x}+\mathrm{1}\mid}\right.}\right)−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)\:+\mathrm{c} \\ $$$$\mathrm{ln}\mid\mathrm{y}\mid\:=\mathrm{2ln}\left(\frac{\mid\mathrm{x}\mid}{\left(^{\mathrm{3}} \sqrt{\mid\mathrm{x}+\mathrm{1}\mid}\right.}\right)−\frac{\mathrm{2}}{\mathrm{3}}\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)\:+\mathrm{c}\:\Rightarrow \\ $$$$\mathrm{y}\left(\mathrm{x}\right)=\mathrm{k}\:×\frac{\mathrm{x}^{\mathrm{2}} }{\left(\mathrm{x}+\mathrm{1}\right)^{\frac{\mathrm{2}}{\mathrm{3}}} }\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{−\frac{\mathrm{2}}{\mathrm{3}}} \:=\frac{\mathrm{kx}^{\mathrm{2}} }{\left(\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)^{\frac{\mathrm{2}}{\mathrm{3}}} } \\ $$

Answered by mathmax by abdo last updated on 29/Nov/20

b) h(x)=(x^3 /(x^(2 ) +1))g(x) ⇒h^(′ ) =((3x^2 (x^2 +1)−x^3 (2x))/((x^2 +1)^2 ))g +(x^3 /(x^2 +1))g^′ =((3x^4 +3x^2 −2x^4 )/((x^2 +1)^2 ))g +(x^3 /(x^2 +1))g^′ =((x^(4 ) +3x^2 )/((x^2 +1)^2 ))g +(x^3 /(x^2 +1))g^′ h solution of e ⇒ x(x^2 +1)(((x^4 +3x^2 )/((x^2 +1)^2 ))g +(x^3 /(x^2 +1))g^′ )−((2x^3 )/(x^2 +1))g =x^3 (x−1)e^(−x) ⇒ ((x(x^4 +3x^2 ))/(x^2 +1))g +x^4 g^′ −((2x^3 )/(x^2 +1))g =x^3 (x−1)e^(−x) ⇒ ((x^5 +3x^3 −2x^3 )/(x^2 +1))g +x^4 g^′ =x^3 (x−1)^2 e^(−x) ⇒ x^(3 ) g +x^4 g^′ =x^3 (x−1)^2 e^(−x) ⇒g+xg^′ =(x−1)^2 e^(−x) h→ xg^′ =−g ⇒(g^′ /g)=−(1/x) ⇒ln∣g∣=−ln∣x∣ +c ⇒g=(k/x) mvc method →g^′ =(k^′ /x)−(k/x^2 ) and e⇒k^′ −(k/x) +(k/x)=(x−1)^2 e^(−x) ⇒ ⇒k^′ =(x−1)^2 e^(−x) ⇒k =∫ (x−1)^2 e^(−x) dx =−(x−1)^2 e^(−x) +∫ 2(x−1)e^(−x ) dx =−(x−1)^2 e^(−x) +2{ −(x−1)e^(−x) +∫ e^(−x) dx} =−(x−1)^2 e^(−x) +2{−xe^(−x) } +c ={−(x−1)^2 −2x}e^(−x) +c ={ −(x^2 −2x+1)−2x}e^(−x) +c =−(x^2 +1)e^(−x) +c ⇒g(x)=((c−(x^2 +1)e^(−x) )/x)....

$$\left.\mathrm{b}\right)\:\:\mathrm{h}\left(\mathrm{x}\right)=\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}\:} +\mathrm{1}}\mathrm{g}\left(\mathrm{x}\right)\:\Rightarrow\mathrm{h}^{'\:} =\frac{\mathrm{3x}^{\mathrm{2}} \left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)−\mathrm{x}^{\mathrm{3}} \left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\mathrm{g}\: \\ $$$$+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}^{'} \:=\frac{\mathrm{3x}^{\mathrm{4}} \:+\mathrm{3x}^{\mathrm{2}} −\mathrm{2x}^{\mathrm{4}} }{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\mathrm{g}\:+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}^{'} \\ $$$$=\frac{\mathrm{x}^{\mathrm{4}\:} +\mathrm{3x}^{\mathrm{2}} }{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\mathrm{g}\:+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}^{'} \:\:\:\:\:\mathrm{h}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{e}\:\Rightarrow \\ $$$$\mathrm{x}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\left(\frac{\mathrm{x}^{\mathrm{4}} \:+\mathrm{3x}^{\mathrm{2}} }{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\mathrm{g}\:+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}^{'} \right)−\frac{\mathrm{2x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}\:=\mathrm{x}^{\mathrm{3}} \left(\mathrm{x}−\mathrm{1}\right)\mathrm{e}^{−\mathrm{x}} \:\Rightarrow \\ $$$$\frac{\mathrm{x}\left(\mathrm{x}^{\mathrm{4}} \:+\mathrm{3x}^{\mathrm{2}} \right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}\:+\mathrm{x}^{\mathrm{4}} \mathrm{g}^{'} −\frac{\mathrm{2x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}\:=\mathrm{x}^{\mathrm{3}} \left(\mathrm{x}−\mathrm{1}\right)\mathrm{e}^{−\mathrm{x}} \:\Rightarrow \\ $$$$\frac{\mathrm{x}^{\mathrm{5}} \:+\mathrm{3x}^{\mathrm{3}} −\mathrm{2x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{g}\:\:+\mathrm{x}^{\mathrm{4}} \:\mathrm{g}^{'} \:=\mathrm{x}^{\mathrm{3}} \left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \:\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{3}\:} \:\mathrm{g}\:+\mathrm{x}^{\mathrm{4}} \:\mathrm{g}^{'} \:=\mathrm{x}^{\mathrm{3}} \left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \:\Rightarrow\mathrm{g}+\mathrm{xg}^{'} \:=\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \\ $$$$\mathrm{h}\rightarrow\:\mathrm{xg}^{'} \:=−\mathrm{g}\:\Rightarrow\frac{\mathrm{g}^{'} }{\mathrm{g}}=−\frac{\mathrm{1}}{\mathrm{x}}\:\Rightarrow\mathrm{ln}\mid\mathrm{g}\mid=−\mathrm{ln}\mid\mathrm{x}\mid\:+\mathrm{c}\:\Rightarrow\mathrm{g}=\frac{\mathrm{k}}{\mathrm{x}} \\ $$$$\mathrm{mvc}\:\mathrm{method}\:\rightarrow\mathrm{g}^{'} \:=\frac{\mathrm{k}^{'} }{\mathrm{x}}−\frac{\mathrm{k}}{\mathrm{x}^{\mathrm{2}} }\:\:\mathrm{and}\:\mathrm{e}\Rightarrow\mathrm{k}^{'} −\frac{\mathrm{k}}{\mathrm{x}}\:+\frac{\mathrm{k}}{\mathrm{x}}=\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \:\Rightarrow \\ $$$$\Rightarrow\mathrm{k}^{'} \:=\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \:\Rightarrow\mathrm{k}\:=\int\:\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \:\mathrm{dx} \\ $$$$=−\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} +\int\:\mathrm{2}\left(\mathrm{x}−\mathrm{1}\right)\mathrm{e}^{−\mathrm{x}\:} \mathrm{dx} \\ $$$$=−\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \:\mathrm{e}^{−\mathrm{x}} \:+\mathrm{2}\left\{\:\:−\left(\mathrm{x}−\mathrm{1}\right)\mathrm{e}^{−\mathrm{x}} +\int\:\mathrm{e}^{−\mathrm{x}} \:\mathrm{dx}\right\} \\ $$$$=−\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{e}^{−\mathrm{x}} \:+\mathrm{2}\left\{−\mathrm{xe}^{−\mathrm{x}} \right\}\:+\mathrm{c} \\ $$$$=\left\{−\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2x}\right\}\mathrm{e}^{−\mathrm{x}} +\mathrm{c}\:=\left\{\:−\left(\mathrm{x}^{\mathrm{2}} −\mathrm{2x}+\mathrm{1}\right)−\mathrm{2x}\right\}\mathrm{e}^{−\mathrm{x}} \:+\mathrm{c}\: \\ $$$$=−\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)\mathrm{e}^{−\mathrm{x}} \:+\mathrm{c}\:\Rightarrow\mathrm{g}\left(\mathrm{x}\right)=\frac{\mathrm{c}−\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)\mathrm{e}^{−\mathrm{x}} }{\mathrm{x}}.... \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com