Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 124076 by TANMAY PANACEA last updated on 30/Nov/20

intregation...collected problem

$${intregation}...{collected}\:{problem} \\ $$

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

calculus collected roblem

$$\boldsymbol{{calculus}}\:\boldsymbol{{collected}}\:\boldsymbol{{roblem}} \\ $$

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by TANMAY PANACEA last updated on 30/Nov/20

Commented by Dwaipayan Shikari last updated on 30/Nov/20

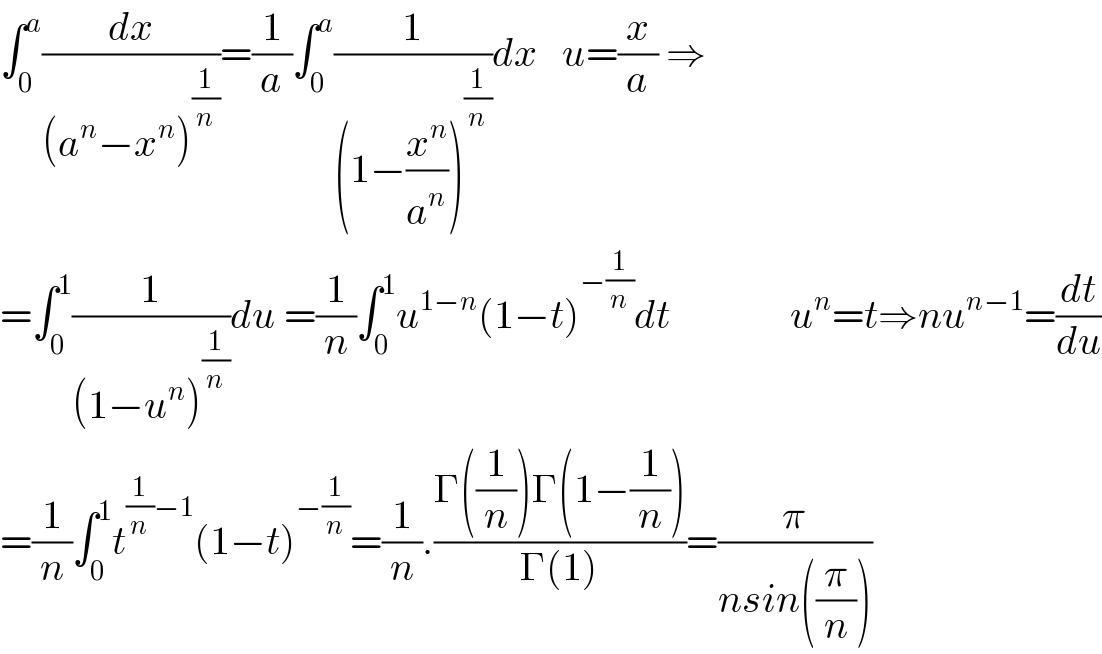
∫_0 ^a (dx/((a^n −x^n )^(1/n) ))=(1/a)∫_0 ^a (1/((1−(x^n /a^n ))^(1/n) ))dx u=(x/a) ⇒ =∫_0 ^1 (1/((1−u^n )^(1/n) ))du =(1/n)∫_0 ^1 u^(1−n) (1−t)^(−(1/n)) dt u^n =t⇒nu^(n−1) =(dt/du) =(1/n)∫_0 ^1 t^((1/n)−1) (1−t)^(−(1/n)) =(1/n).((Γ((1/n))Γ(1−(1/n)))/(Γ(1)))=(π/(nsin((π/n))))

$$\int_{\mathrm{0}} ^{{a}} \frac{{dx}}{\left({a}^{{n}} −{x}^{{n}} \right)^{\frac{\mathrm{1}}{{n}}} }=\frac{\mathrm{1}}{{a}}\int_{\mathrm{0}} ^{{a}} \frac{\mathrm{1}}{\left(\mathrm{1}−\frac{{x}^{{n}} }{{a}^{{n}} }\right)^{\frac{\mathrm{1}}{{n}}} }{dx}\:\:\:{u}=\frac{{x}}{{a}}\:\Rightarrow \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\left(\mathrm{1}−{u}^{{n}} \right)^{\frac{\mathrm{1}}{{n}}} }{du}\:=\frac{\mathrm{1}}{{n}}\int_{\mathrm{0}} ^{\mathrm{1}} {u}^{\mathrm{1}−{n}} \left(\mathrm{1}−{t}\right)^{−\frac{\mathrm{1}}{{n}}} {dt}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{u}^{{n}} ={t}\Rightarrow{nu}^{{n}−\mathrm{1}} =\frac{{dt}}{{du}} \\ $$$$=\frac{\mathrm{1}}{{n}}\int_{\mathrm{0}} ^{\mathrm{1}} {t}^{\frac{\mathrm{1}}{{n}}−\mathrm{1}} \left(\mathrm{1}−{t}\right)^{−\frac{\mathrm{1}}{{n}}} =\frac{\mathrm{1}}{{n}}.\frac{\Gamma\left(\frac{\mathrm{1}}{{n}}\right)\Gamma\left(\mathrm{1}−\frac{\mathrm{1}}{{n}}\right)}{\Gamma\left(\mathrm{1}\right)}=\frac{\pi}{{nsin}\left(\frac{\pi}{{n}}\right)}\: \\ $$

Commented by TANMAY PANACEA last updated on 30/Nov/20

thank you but question no 22 pls

$${thank}\:{you}\:{but}\:{question}\:{no}\:\mathrm{22}\:{pls} \\ $$

Commented by mnjuly1970 last updated on 30/Nov/20

note:: Γ(x)Γ(1−x)=(π/(sin(πx))) note:: ∫_0 ^( π) ln(sin(x))dx=−πln(2) Ω=∫_0 ^( 1) ln(Γ(x))dx Ω=∫_0 ^( 1) ln(Γ(1−x))dx 2Ω=∫_0 ^( 1) ln[(Γ(x)Γ(1−x))]dx 2Ω =^(euler reflection formula) ∫_0 ^( 1) ln((π/(sin(πx))))dx =ln(π)−∫_0 ^( 1) ln(sin(πx))dx =^(πx=t) ln(π)−(1/π)∫_0 ^( π) ln(sin(x))dx =ln(π)−(1/π)(−πln(2)) =ln(π)+ln(2)=ln(2π) ∴ Ω=(1/2)ln(2π)=ln((√(2π)) )✓

$$\:\:\:\:{note}::\:\Gamma\left({x}\right)\Gamma\left(\mathrm{1}−{x}\right)=\frac{\pi}{{sin}\left(\pi{x}\right)} \\ $$$$\:{note}::\:\:\int_{\mathrm{0}} ^{\:\pi} {ln}\left({sin}\left({x}\right)\right){dx}=−\pi{ln}\left(\mathrm{2}\right) \\ $$$$\Omega=\int_{\mathrm{0}} ^{\:\mathrm{1}} {ln}\left(\Gamma\left({x}\right)\right){dx} \\ $$$$\Omega=\int_{\mathrm{0}} ^{\:\mathrm{1}} {ln}\left(\Gamma\left(\mathrm{1}−{x}\right)\right){dx} \\ $$$$\:\mathrm{2}\Omega=\int_{\mathrm{0}} ^{\:\mathrm{1}} {ln}\left[\left(\Gamma\left({x}\right)\Gamma\left(\mathrm{1}−{x}\right)\right)\right]{dx} \\ $$$$\:\:\mathrm{2}\Omega\:\overset{{euler}\:{reflection}\:{formula}} {=}\int_{\mathrm{0}} ^{\:\mathrm{1}} {ln}\left(\frac{\pi}{{sin}\left(\pi{x}\right)}\right){dx} \\ $$$$={ln}\left(\pi\right)−\int_{\mathrm{0}} ^{\:\mathrm{1}} {ln}\left({sin}\left(\pi{x}\right)\right){dx} \\ $$$$\overset{\pi{x}={t}} {=}{ln}\left(\pi\right)−\frac{\mathrm{1}}{\pi}\int_{\mathrm{0}} ^{\:\pi} {ln}\left({sin}\left({x}\right)\right){dx} \\ $$$$={ln}\left(\pi\right)−\frac{\mathrm{1}}{\pi}\left(−\pi{ln}\left(\mathrm{2}\right)\right) \\ $$$$={ln}\left(\pi\right)+{ln}\left(\mathrm{2}\right)={ln}\left(\mathrm{2}\pi\right) \\ $$$$\therefore\:\:\Omega=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{2}\pi\right)={ln}\left(\sqrt{\mathrm{2}\pi}\:\right)\checkmark \\ $$

Commented by TANMAY PANACEA last updated on 30/Nov/20

excellent

$${excellent} \\ $$

Commented by mnjuly1970 last updated on 30/Nov/20

you are welcom sir tanmay..

$${you}\:{are}\:{welcom}\:{sir}\:{tanmay}.. \\ $$

Commented by Dwaipayan Shikari last updated on 30/Nov/20

Γ(s)Γ(1−s)=(π/(sinπs)) log(Γ(s)+log(Γ(1−s))=log(π)−log(sinπs) ∫_0 ^1 log(Γ(s))=∫_0 ^1 log(Γ(1−s))ds ∫_0 ^1 2log(Γ(s))=(1/2)∫_0 ^1 log(π)ds−(1/2)∫_0 ^1 log(sinπs)ds ∫_0 ^1 log(Γ(s))=(1/2)log(π)−(1/(2π))∫_0 ^π log(sinu)du u=2t⇒1=2(dt/du) =(1/2)log(π)−(2/(2π))∫_0 ^(π/2) log(2)+log(sint)+log(cost) =(1/2)log(π)−(1/2)log(2)+(1/2)log(4) =(1/2)log(2π)

$$\Gamma\left({s}\right)\Gamma\left(\mathrm{1}−{s}\right)=\frac{\pi}{{sin}\pi{s}} \\ $$$${log}\left(\Gamma\left({s}\right)+{log}\left(\Gamma\left(\mathrm{1}−{s}\right)\right)={log}\left(\pi\right)−{log}\left({sin}\pi{s}\right)\right. \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {log}\left(\Gamma\left({s}\right)\right)=\int_{\mathrm{0}} ^{\mathrm{1}} {log}\left(\Gamma\left(\mathrm{1}−{s}\right)\right){ds} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{2}{log}\left(\Gamma\left({s}\right)\right)=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} {log}\left(\pi\right){ds}−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} {log}\left({sin}\pi{s}\right){ds} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {log}\left(\Gamma\left({s}\right)\right)=\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\pi\right)−\frac{\mathrm{1}}{\mathrm{2}\pi}\int_{\mathrm{0}} ^{\pi} {log}\left({sinu}\right){du}\:\:\:\:\:{u}=\mathrm{2}{t}\Rightarrow\mathrm{1}=\mathrm{2}\frac{{dt}}{{du}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\pi\right)−\frac{\mathrm{2}}{\mathrm{2}\pi}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {log}\left(\mathrm{2}\right)+{log}\left({sint}\right)+{log}\left({cost}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\pi\right)−\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\mathrm{2}\right)+\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\mathrm{4}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\mathrm{2}\pi\right) \\ $$

Commented by Dwaipayan Shikari last updated on 30/Nov/20

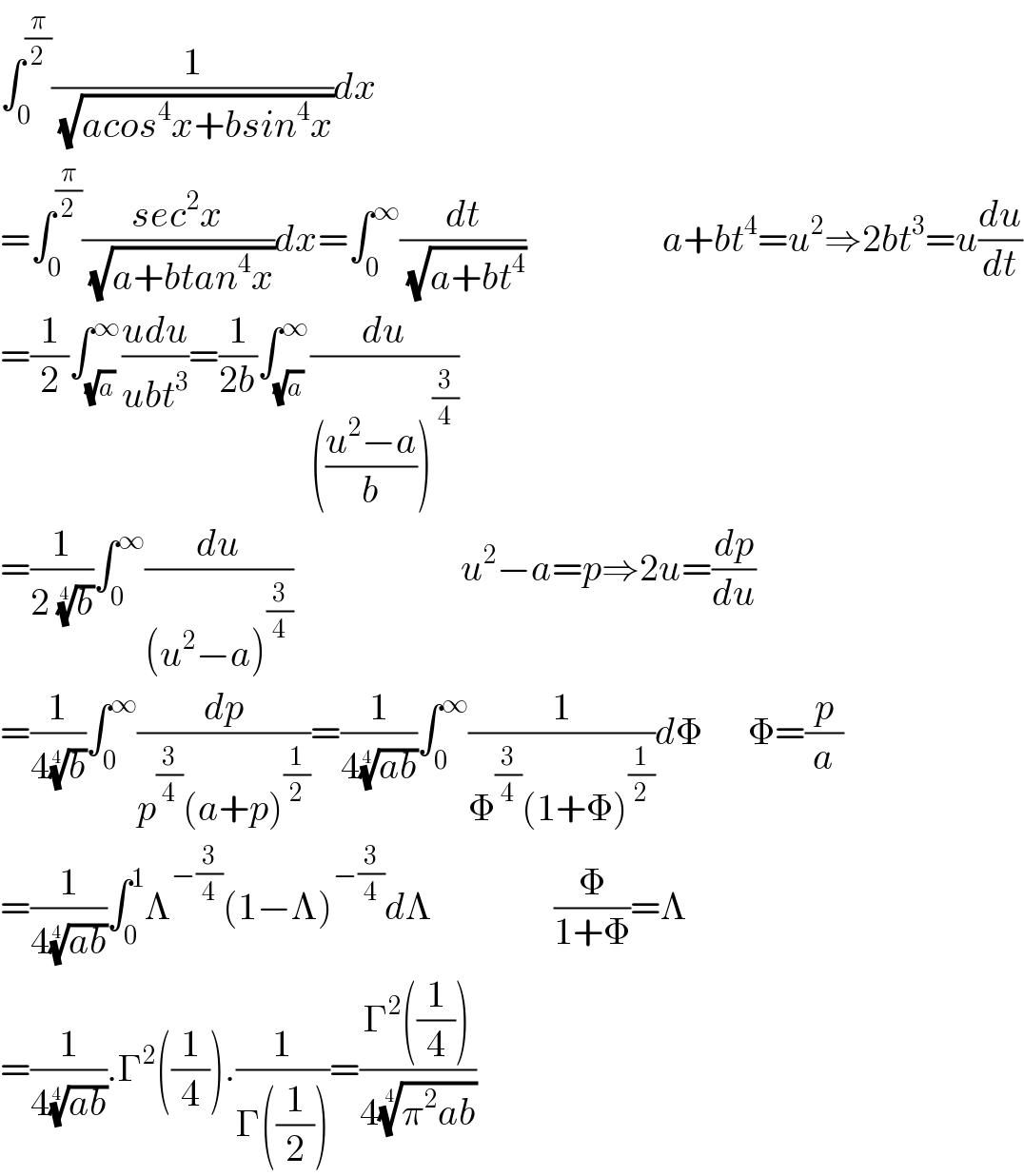
∫_0 ^(π/2) (1/( (√(acos^4 x+bsin^4 x))))dx =∫_0 ^(π/2) ((sec^2 x)/( (√(a+btan^4 x))))dx=∫_0 ^∞ (dt/( (√(a+bt^4 )))) a+bt^4 =u^2 ⇒2bt^3 =u(du/dt) =(1/2)∫_(√a) ^∞ ((udu)/(ubt^3 ))=(1/(2b))∫_(√a) ^∞ (du/((((u^2 −a)/b))^(3/4) )) =(1/(2 (b)^(1/4) ))∫_0 ^∞ (du/((u^2 −a)^(3/4) )) u^2 −a=p⇒2u=(dp/du) =(1/(4(b)^(1/4) ))∫_0 ^∞ (dp/(p^(3/4) (a+p)^(1/2) ))=(1/(4((ab))^(1/4) ))∫_0 ^∞ (1/(Φ^(3/4) (1+Φ)^(1/2) ))dΦ Φ=(p/a) =(1/(4((ab))^(1/4) ))∫_0 ^1 Λ^(−(3/4)) (1−Λ)^(−(3/4)) dΛ (Φ/(1+Φ))=Λ =(1/(4((ab))^(1/4) )).Γ^2 ((1/4)).(1/(Γ((1/2))))=((Γ^2 ((1/4)))/(4((π^2 ab))^(1/4) ))

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{1}}{\:\sqrt{{acos}^{\mathrm{4}} {x}+{bsin}^{\mathrm{4}} {x}}}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{sec}^{\mathrm{2}} {x}}{\:\sqrt{{a}+{btan}^{\mathrm{4}} {x}}}{dx}=\int_{\mathrm{0}} ^{\infty} \frac{{dt}}{\:\sqrt{{a}+{bt}^{\mathrm{4}} }}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{a}+{bt}^{\mathrm{4}} ={u}^{\mathrm{2}} \Rightarrow\mathrm{2}{bt}^{\mathrm{3}} ={u}\frac{{du}}{{dt}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\sqrt{{a}}} ^{\infty} \frac{{udu}}{{ubt}^{\mathrm{3}} }=\frac{\mathrm{1}}{\mathrm{2}{b}}\int_{\sqrt{{a}}} ^{\infty} \frac{{du}}{\left(\frac{{u}^{\mathrm{2}} −{a}}{{b}}\right)^{\frac{\mathrm{3}}{\mathrm{4}}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\:\sqrt[{\mathrm{4}}]{{b}}}\int_{\mathrm{0}} ^{\infty} \frac{{du}}{\left({u}^{\mathrm{2}} −{a}\right)^{\frac{\mathrm{3}}{\mathrm{4}}} }\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{u}^{\mathrm{2}} −{a}={p}\Rightarrow\mathrm{2}{u}=\frac{{dp}}{{du}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt[{\mathrm{4}}]{{b}}}\int_{\mathrm{0}} ^{\infty} \frac{{dp}}{{p}^{\frac{\mathrm{3}}{\mathrm{4}}} \left({a}+{p}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} }=\frac{\mathrm{1}}{\mathrm{4}\sqrt[{\mathrm{4}}]{{ab}}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{1}}{\Phi^{\frac{\mathrm{3}}{\mathrm{4}}} \left(\mathrm{1}+\Phi\right)^{\frac{\mathrm{1}}{\mathrm{2}}} }{d}\Phi\:\:\:\:\:\:\Phi=\frac{{p}}{{a}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt[{\mathrm{4}}]{{ab}}}\int_{\mathrm{0}} ^{\mathrm{1}} \Lambda^{−\frac{\mathrm{3}}{\mathrm{4}}} \left(\mathrm{1}−\Lambda\right)^{−\frac{\mathrm{3}}{\mathrm{4}}} {d}\Lambda\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\Phi}{\mathrm{1}+\Phi}=\Lambda \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt[{\mathrm{4}}]{{ab}}}.\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right).\frac{\mathrm{1}}{\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}=\frac{\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)}{\mathrm{4}\sqrt[{\mathrm{4}}]{\pi^{\mathrm{2}} {ab}}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com