∫_0 ^( (π/4)) ((sin(ς)dς)/(cos(ς)+sin(ς)))dς where ς : zeta


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 124963 by 676597498 last updated on 07/Dec/20

$\int_{0}^{\frac{π}{4}} \frac{\sin (ς) d ς}{\cos (ς) + \sin (ς)} d ς$ $where ς : zeta$
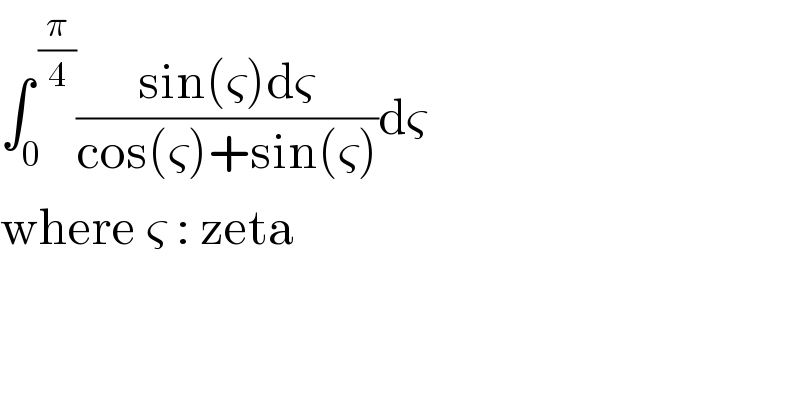
Commented by MJS_new last updated on 07/Dec/20

$syntax error .$
	Answered by Dwaipayan Shikari last updated on 07/Dec/20

	$\int_{0}^{\frac{π}{4}} \frac{1}{t a n (ζ) + 1} d ζ = \int_{0}^{1} \frac{1}{t + 1} . \frac{1}{t^{2} + 1} d t t a n ζ = t \Rightarrow {s e c}^{2} ζ = \frac{d t}{d ζ}$ $= \frac{1}{2} \int_{0}^{1} \frac{1}{t + 1} - \frac{t - 1}{t^{2} + 1} d t$ $= \frac{1}{2} l o g (2) - \frac{1}{4} \int_{0}^{1} \frac{2 t}{t^{2} + 1} + \frac{1}{2} \int_{0}^{1} \frac{1}{1 + t^{2}} d t$ $= - \frac{1}{4} l o g (2) + \frac{π}{8}$
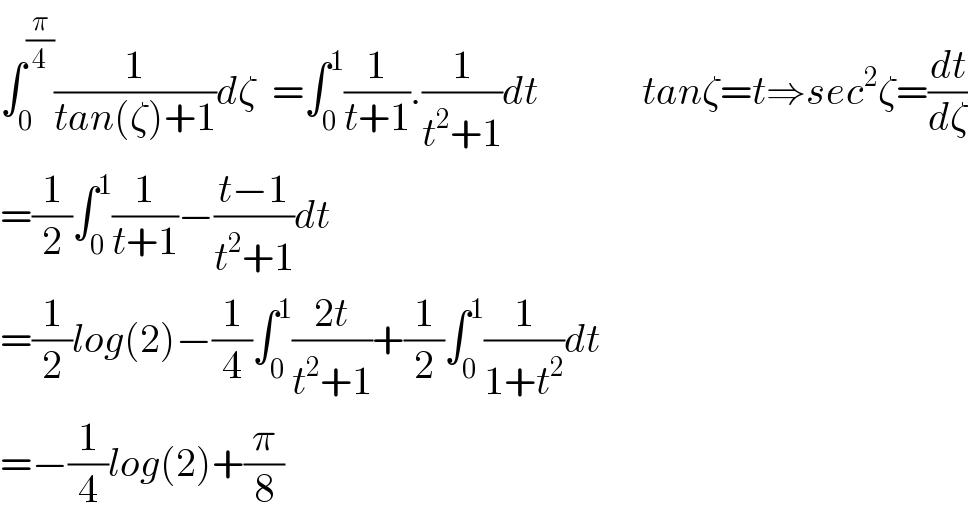
	Commented by 676597498 last updated on 07/Dec/20

	$thanks$ $but there are 2 (d ς)$ $look again$ $it is \int \frac{\sin (ς) d ς}{\cos (ς) + \sin (ς)} d ς$
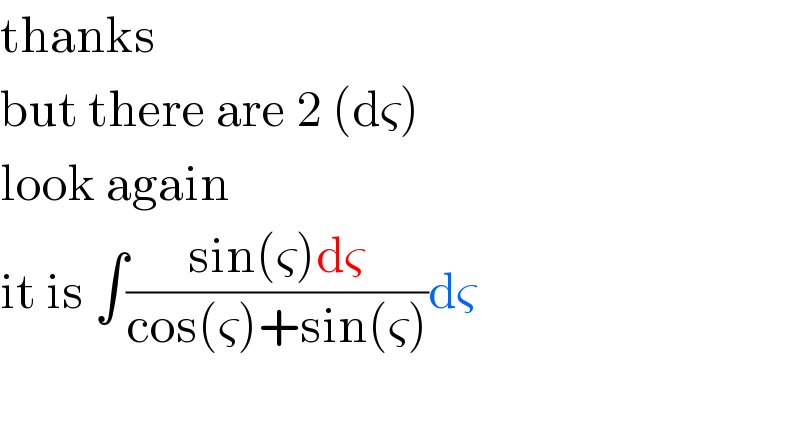
	Commented by Dwaipayan Shikari last updated on 07/Dec/20

	$S o r r y b u t i t {d o e s n}^{'} t m a k e a n y s e n s e t o m e$
	Commented by 676597498 last updated on 07/Dec/20

	$it makes sense$
	Commented by mr W last updated on 07/Dec/20

	$it makes N o sense!$
	Commented by MJS_new last updated on 07/Dec/20

	$if it makes sense, what sense does it make ?$
	Answered by mathmax by abdo last updated on 07/Dec/20
	$A =∫_0 ^(π/4) ((sinx)/(cosx +sinx))dx we do the changement tan((x/2))=t ⇒ A =∫_0 ^((√2)−1) (((2t)/(1+t^2 ))/(((1−t^2 )/(1+t^2 ))+((2t)/(1+t^2 ))))((2dt)/(1+t^2 )) =∫_0 ^((√2)−1) ((4t)/((t^2 +1)(−t^2 +2t+1)))dt =−4 ∫_0 ^((√2)−1) ((tdt)/((t^2 +1)(t^2 −2t−1))) let decompose F(t)=(t/((t^2 +1)(t^2 −2t−1))) t^2 −2t−1→Δ^′ =1+1=2 ⇒t_1 =1+(√2) and t_2 =1−(√2) ⇒ F(t)=(t/((t−t_1 )(t−t_2 )(t^2 +1))) =(a/(t−t_1 )) +(b/(t−t_2 )) +((mt +n)/(t^2 +1)) a=(t_1 /(2(√2)(t_1 ^2 +1))) =((1+(√2))/(2(√2)(3+2(√2)+1)))=((1+(√2))/(2(√2)(4+2(√2))))=((1+(√2))/(8(√2)+8))=(1/8) b =(t_2 /(−2(√2)(t_2 ^2 +1))) =((1−(√2))/(−2(√2)(3−2(√2)+1)))=((1−(√2))/(−2(√2)(4−2(√2)))) =((1−(√2))/(−8(√2)+8)) =(1/8) lim_(t→+∞) tF(t)=0=a+b+m ⇒m=−(1/4) F(o)=0 =−(a/t_1 )−(b/t_2 ) +n ⇒n=(a/t_1 )+(b/t_2 ) =(1/(8(1+(√2))))+(1/(8(1−(√2)))) =(1/8)((2/((−1))))=−(1/4) ⇒ F(t)=(1/(8(t−(1+(√2))))+(1/(8(t−(1−(√2)))))−(1/4)×((t+1)/(t^2 +1)) ⇒ ∫_0 ^((√2)−1) F(t)dt =(1/8)∫_0 ^((√2)−1) (dt/(t−1−(√2))) +(1/8)∫_0 ^((√2)−1) (dt/(t−1+(√2))) −(1/8)∫_0 ^((√2)−1) ((2t)/(t^2 +1))−(1/4)∫_0 ^((√2)−1) (dt/(t^2 +1)) =(1/8)[ln∣(t−1)^2 −2∣]_0 ^((√2)−1) −(1/8)[ln(t^2 +1)]_0 ^((√2)−1) −(1/4)[arctant]_0 ^((√2)−1) =(1/8){ln∣((√2)−2)^2 −2∣−(1/8)ln(((√2)−1)^2 +1)−(1/4)×(π/8) =(1/8)ln∣2−4(√2)+4−2∣−(1/8)ln(3−2(√2)+1)−(π/(32)) =(1/8)ln(4(√2)−4)−(1/8)ln(4−2(√2))−(π/(32)) ⇒ I=−(1/2)ln(4(√2)−4)+(1/2)ln(4−2(√2))+(π/8)$
	$A = \int_{0}^{\frac{π}{4}} \frac{sinx}{cosx + sinx} dx we do the changement \tan (\frac{x}{2}) = t \Rightarrow$ $A = \int_{0}^{\sqrt{2} - 1} \frac{\frac{2 t}{1 + t^{2}}}{\frac{1 - t^{2}}{1 + t^{2}} + \frac{2 t}{1 + t^{2}}} \frac{2 dt}{1 + t^{2}} = \int_{0}^{\sqrt{2} - 1} \frac{4 t}{(t^{2} + 1) (- t^{2} + 2 t + 1)} dt$ $= - 4 \int_{0}^{\sqrt{2} - 1} \frac{tdt}{(t^{2} + 1) (t^{2} - 2 t - 1)} let decompose$ $F (t) = \frac{t}{(t^{2} + 1) (t^{2} - 2 t - 1)}$ $t^{2} - 2 t - 1 \to Δ^{^{'}} = 1 + 1 = 2 \Rightarrow t_{1} = 1 + \sqrt{2} and t_{2} = 1 - \sqrt{2} \Rightarrow$ $F (t) = \frac{t}{(t - t_{1}) (t - t_{2}) (t^{2} + 1)} = \frac{a}{t - t_{1}} + \frac{b}{t - t_{2}} + \frac{mt + n}{t^{2} + 1}$ $a = \frac{t_{1}}{2 \sqrt{2} (t_{1}^{2} + 1)} = \frac{1 + \sqrt{2}}{2 \sqrt{2} (3 + 2 \sqrt{2} + 1)} = \frac{1 + \sqrt{2}}{2 \sqrt{2} (4 + 2 \sqrt{2})} = \frac{1 + \sqrt{2}}{8 \sqrt{2} + 8} = \frac{1}{8}$ $b = \frac{t_{2}}{- 2 \sqrt{2} (t_{2}^{2} + 1)} = \frac{1 - \sqrt{2}}{- 2 \sqrt{2} (3 - 2 \sqrt{2} + 1)} = \frac{1 - \sqrt{2}}{- 2 \sqrt{2} (4 - 2 \sqrt{2})}$ $= \frac{1 - \sqrt{2}}{- 8 \sqrt{2} + 8} = \frac{1}{8}$ $\lim_{t \to + \infty} tF (t) = 0 = a + b + m \Rightarrow m = - \frac{1}{4}$ $F (o) = 0 = - \frac{a}{t_{1}} - \frac{b}{t_{2}} + n \Rightarrow n = \frac{a}{t_{1}} + \frac{b}{t_{2}} = \frac{1}{8 (1 + \sqrt{2})} + \frac{1}{8 (1 - \sqrt{2})}$ $= \frac{1}{8} (\frac{2}{(- 1)}) = - \frac{1}{4} \Rightarrow$ $F (t) = \frac{1}{8 (t - (1 + \sqrt{2})} + \frac{1}{8 (t - (1 - \sqrt{2}))} - \frac{1}{4} \times \frac{t + 1}{t^{2} + 1} \Rightarrow$ $\int_{0}^{\sqrt{2} - 1} F (t) dt = \frac{1}{8} \int_{0}^{\sqrt{2} - 1} \frac{dt}{t - 1 - \sqrt{2}} + \frac{1}{8} \int_{0}^{\sqrt{2} - 1} \frac{dt}{t - 1 + \sqrt{2}}$ $- \frac{1}{8} \int_{0}^{\sqrt{2} - 1} \frac{2 t}{t^{2} + 1} - \frac{1}{4} \int_{0}^{\sqrt{2} - 1} \frac{dt}{t^{2} + 1}$ $= \frac{1}{8} {[\ln ∣ {(t - 1)}^{2} - 2 ∣]}_{0}^{\sqrt{2} - 1} - \frac{1}{8} {[\ln (t^{2} + 1)]}_{0}^{\sqrt{2} - 1} - \frac{1}{4} {[arctant]}_{0}^{\sqrt{2} - 1}$ $= \frac{1}{8} {\ln ∣ {(\sqrt{2} - 2)}^{2} - 2 ∣ - \frac{1}{8} \ln ({(\sqrt{2} - 1)}^{2} + 1) - \frac{1}{4} \times \frac{π}{8}$ $= \frac{1}{8} \ln ∣ 2 - 4 \sqrt{2} + 4 - 2 ∣ - \frac{1}{8} \ln (3 - 2 \sqrt{2} + 1) - \frac{π}{32}$ $= \frac{1}{8} \ln (4 \sqrt{2} - 4) - \frac{1}{8} \ln (4 - 2 \sqrt{2}) - \frac{π}{32} \Rightarrow$ $I = - \frac{1}{2} \ln (4 \sqrt{2} - 4) + \frac{1}{2} \ln (4 - 2 \sqrt{2}) + \frac{π}{8}$
	Commented by talminator2856791 last updated on 07/Dec/20

	$abdo to the rescue!!!!!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum