
Question and Answers Forum
Question Number 125276 by Study last updated on 09/Dec/20
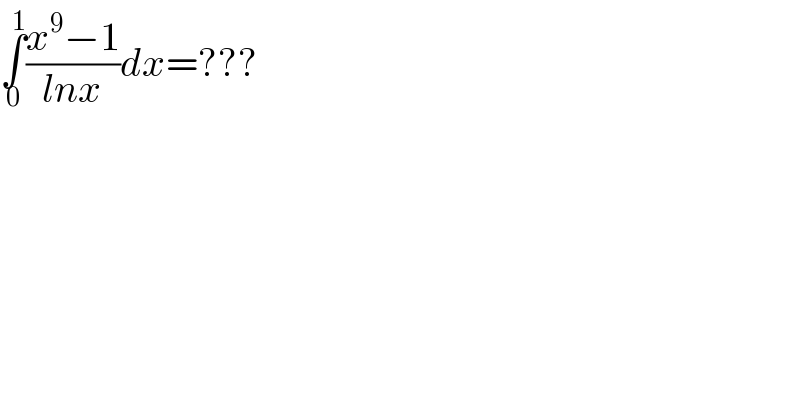
Answered by Dwaipayan Shikari last updated on 09/Dec/20
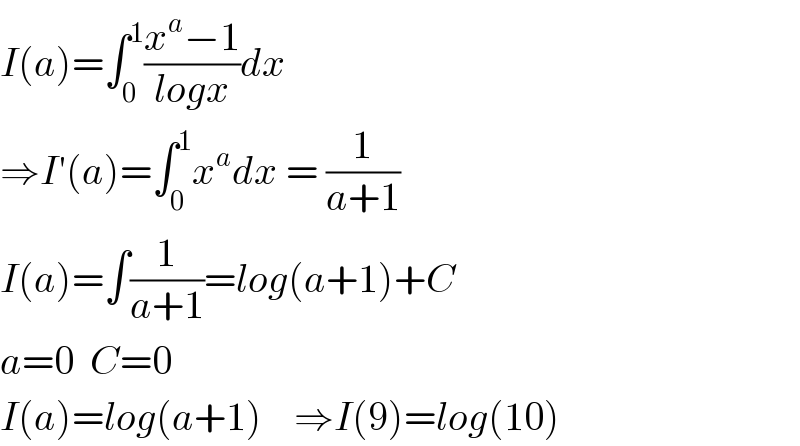
Answered by mathmax by abdo last updated on 09/Dec/20
![I=∫_0 ^1 ((x^9 −1)/(lnx))dx changement lnx=−t give x=e^(−t) I =−∫_0 ^∞ ((e^(−9t) −1)/(−t))(−e^(−t) )dt =−∫_0 ^∞ ((e^(−10t) −e^(−t) )/t)dt =∫_0 ^∞ ((e^(−t) −e^(−10t) )/t)dt let f(x) =∫_0 ^∞ ((e^(−t) −e^(−10t) )/t)e^(−xt) dt with[x>0 f^′ (x)=−∫_0 ^∞ (e^(−t) −e^(−10t) )e^(−xt) dt =∫_0 ^∞ (e^(−(x+10)t) −e^(−(x+1)t) )dt =[−(1/(x+10))e^(−(x+10)t) +(1/(x+1))e^(−(x+1)t) ]_0 ^∞ =(1/(x+10))−(1/(x+1)) ⇒f(x)=ln(((x+10)/(x+1)))+C due to continuity ∃m >0 /∣f(x)∣≤m∫_0 ^∞ e^(−xt) dt =(m/x)→0 (x→+∞) ⇒c=0 ⇒ f(x)=ln(((x+10)/(x+1))) and I =f(0) =ln(10)](Q125299.png)
| ||
Question and Answers Forum | ||
Question Number 125276 by Study last updated on 09/Dec/20 | ||
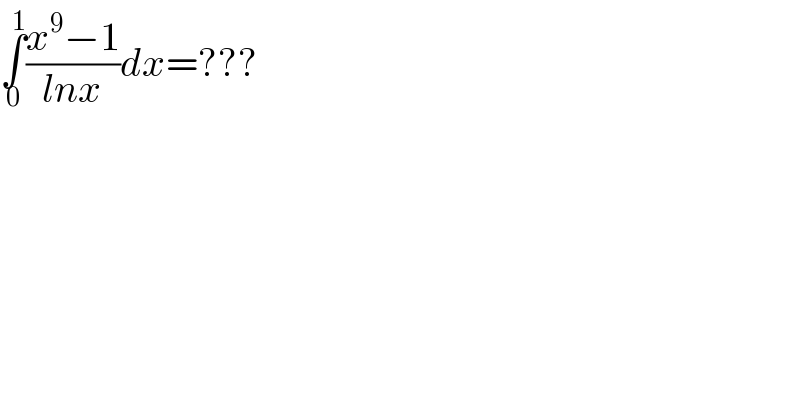 | ||
Answered by Dwaipayan Shikari last updated on 09/Dec/20 | ||
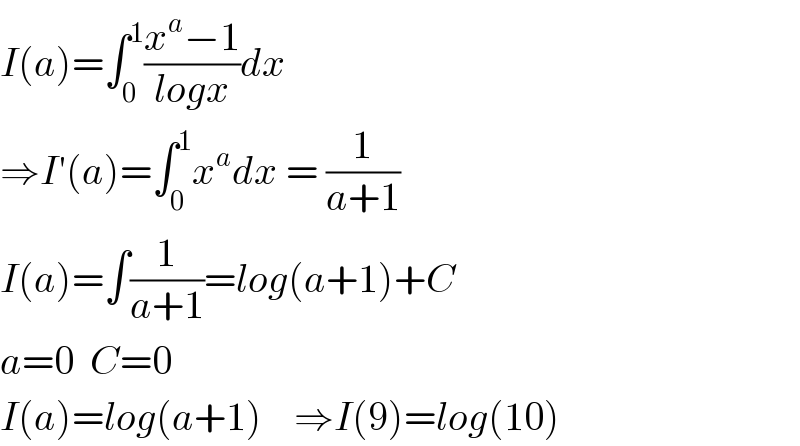 | ||
| ||
Answered by mathmax by abdo last updated on 09/Dec/20 | ||
![I=∫_0 ^1 ((x^9 −1)/(lnx))dx changement lnx=−t give x=e^(−t) I =−∫_0 ^∞ ((e^(−9t) −1)/(−t))(−e^(−t) )dt =−∫_0 ^∞ ((e^(−10t) −e^(−t) )/t)dt =∫_0 ^∞ ((e^(−t) −e^(−10t) )/t)dt let f(x) =∫_0 ^∞ ((e^(−t) −e^(−10t) )/t)e^(−xt) dt with[x>0 f^′ (x)=−∫_0 ^∞ (e^(−t) −e^(−10t) )e^(−xt) dt =∫_0 ^∞ (e^(−(x+10)t) −e^(−(x+1)t) )dt =[−(1/(x+10))e^(−(x+10)t) +(1/(x+1))e^(−(x+1)t) ]_0 ^∞ =(1/(x+10))−(1/(x+1)) ⇒f(x)=ln(((x+10)/(x+1)))+C due to continuity ∃m >0 /∣f(x)∣≤m∫_0 ^∞ e^(−xt) dt =(m/x)→0 (x→+∞) ⇒c=0 ⇒ f(x)=ln(((x+10)/(x+1))) and I =f(0) =ln(10)](Q125299.png) | ||
| ||