Question Number 125368 by bemath last updated on 10/Dec/20
$$\:{How}\:{to}\:{derive}\:{the}\:{formula}\: \\ $$$${for}\:{finding}\:{the}\:{volume}\:{of}\: \\ $$$${spherical}\:{curl}\:? \\ $$
Commented by Ar Brandon last updated on 10/Dec/20
https://www.therightgate.com/deriving-curl-in-cylindrical-and-spherical/
Commented by bemath last updated on 10/Dec/20
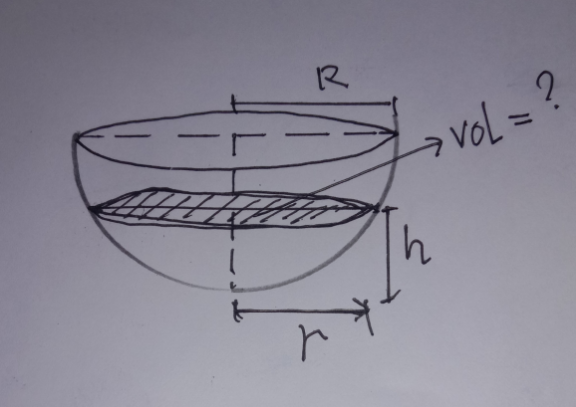
Commented by bemath last updated on 10/Dec/20
$${in}\:{qn}\:\mathrm{125322}\: \\ $$$${V}\:=\:\pi{h}^{\mathrm{2}} \left({R}−\frac{{h}}{\mathrm{3}}\right).\:{how}\:{got}\:{it}? \\ $$
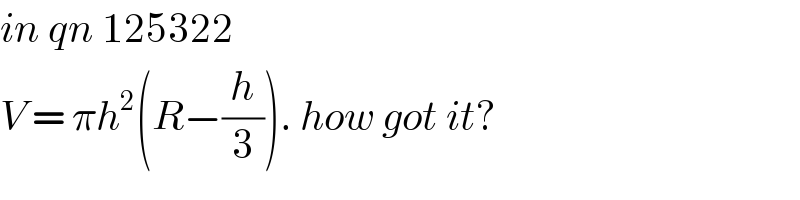
Commented by mr W last updated on 10/Dec/20
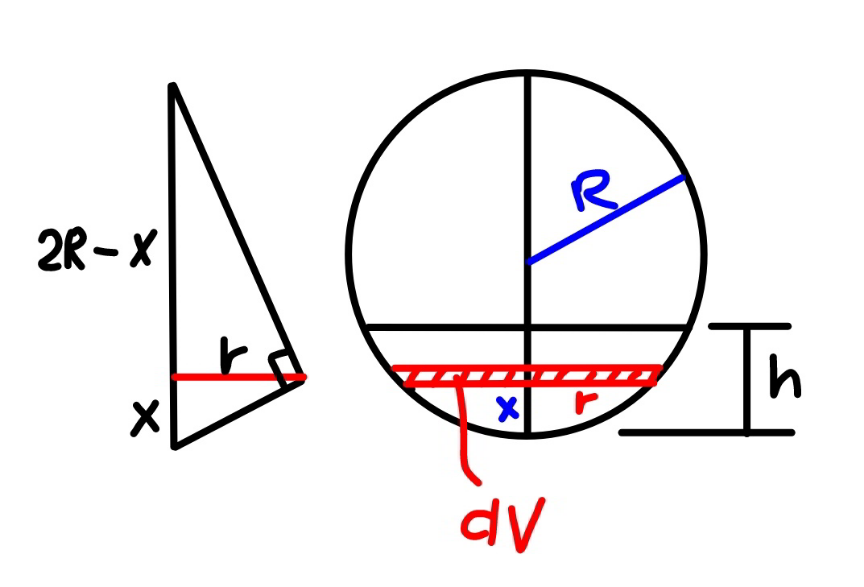
![(x/r)=(r/(2R−x)) ⇒r^2 =x(2R−x) dV=πr^2 dx V=π∫_0 ^h r^2 dx=π∫_0 ^h x(2R−x)dx =π[Rx^2 −(x^3 /3)]_0 ^h =π(Rh^2 −(h^3 /3)) =πh^2 (R−(h/3)) ⇒proved with h=R: V=πR^2 (R−(R/3))=((2πR^3 )/3)=hemisphere with h=2R: V=π4R^2 (R−((2R)/3))=((4πR^3 )/3)=sphere](Q125380.png)
$$\frac{{x}}{{r}}=\frac{{r}}{\mathrm{2}{R}−{x}} \\ $$$$\Rightarrow{r}^{\mathrm{2}} ={x}\left(\mathrm{2}{R}−{x}\right) \\ $$$${dV}=\pi{r}^{\mathrm{2}} {dx} \\ $$$${V}=\pi\int_{\mathrm{0}} ^{{h}} {r}^{\mathrm{2}} {dx}=\pi\int_{\mathrm{0}} ^{{h}} {x}\left(\mathrm{2}{R}−{x}\right){dx} \\ $$$$=\pi\left[{Rx}^{\mathrm{2}} −\frac{{x}^{\mathrm{3}} }{\mathrm{3}}\right]_{\mathrm{0}} ^{{h}} \\ $$$$=\pi\left({Rh}^{\mathrm{2}} −\frac{{h}^{\mathrm{3}} }{\mathrm{3}}\right) \\ $$$$=\pi{h}^{\mathrm{2}} \left({R}−\frac{{h}}{\mathrm{3}}\right)\:\Rightarrow{proved} \\ $$$$ \\ $$$${with}\:{h}={R}: \\ $$$${V}=\pi{R}^{\mathrm{2}} \left({R}−\frac{{R}}{\mathrm{3}}\right)=\frac{\mathrm{2}\pi{R}^{\mathrm{3}} }{\mathrm{3}}={hemisphere} \\ $$$${with}\:{h}=\mathrm{2}{R}: \\ $$$${V}=\pi\mathrm{4}{R}^{\mathrm{2}} \left({R}−\frac{\mathrm{2}{R}}{\mathrm{3}}\right)=\frac{\mathrm{4}\pi{R}^{\mathrm{3}} }{\mathrm{3}}={sphere} \\ $$
Commented by mr W last updated on 10/Dec/20
Commented by bemath last updated on 10/Dec/20
$${waw}....{thanks}\:{sir} \\ $$
Commented by bramlexs22 last updated on 11/Dec/20
![in other way V = π ∫_(R−h) ^R (R^2 −x^2 ) dx V = π (R^2 x−(x^3 /3))^R _(R−h) =π x(((3R^2 −x^2 )/3))_(R−h) ^R = π [((2R^3 )/3)−(R−h)(((3R^2 −R^2 +2Rh−h^2 )/3))] = ((2πR^3 )/3)−(1/3)π(R−h)(2R^2 +2Rh−h^2 ) = ((2πR^3 )/3)−(1/3)π(2R^3 −3Rh^2 +h^3 ) = πRh^2 −(1/3)πr^3 = πh^2 (R−(h/3)).](Q125432.png)
$${in}\:{other}\:{way}\: \\ $$$${V}\:=\:\pi\:\underset{{R}−{h}} {\overset{{R}} {\int}}\left({R}^{\mathrm{2}} −{x}^{\mathrm{2}} \right)\:{dx} \\ $$$${V}\:=\:\pi\:\left({R}^{\mathrm{2}} {x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}\underset{{R}−{h}} {\right)}^{{R}} \\ $$$$=\pi\:{x}\left(\frac{\mathrm{3}{R}^{\mathrm{2}} −{x}^{\mathrm{2}} }{\mathrm{3}}\right)_{{R}−{h}} ^{{R}} \\ $$$$=\:\pi\:\left[\frac{\mathrm{2}{R}^{\mathrm{3}} }{\mathrm{3}}−\left({R}−{h}\right)\left(\frac{\mathrm{3}{R}^{\mathrm{2}} −{R}^{\mathrm{2}} +\mathrm{2}{Rh}−{h}^{\mathrm{2}} }{\mathrm{3}}\right)\right] \\ $$$$=\:\frac{\mathrm{2}\pi{R}^{\mathrm{3}} }{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{3}}\pi\left({R}−{h}\right)\left(\mathrm{2}{R}^{\mathrm{2}} +\mathrm{2}{Rh}−{h}^{\mathrm{2}} \right) \\ $$$$=\:\frac{\mathrm{2}\pi{R}^{\mathrm{3}} }{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{3}}\pi\left(\mathrm{2}{R}^{\mathrm{3}} −\mathrm{3}{Rh}^{\mathrm{2}} +{h}^{\mathrm{3}} \right) \\ $$$$=\:\pi{Rh}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{3}}\pi{r}^{\mathrm{3}} \\ $$$$=\:\pi{h}^{\mathrm{2}} \left({R}−\frac{{h}}{\mathrm{3}}\right). \\ $$