Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 125639 by aurpeyz last updated on 12/Dec/20

Commented by ZiYangLee last updated on 13/Dec/20

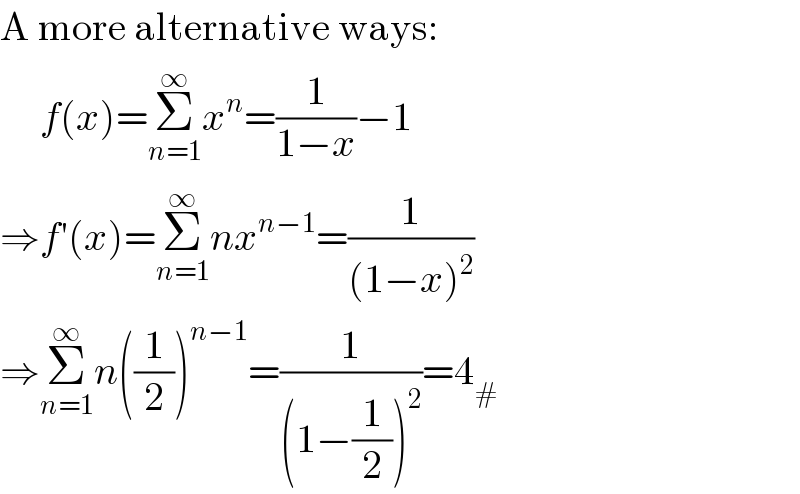
A more alternative ways: f(x)=Σ_(n=1) ^∞ x^n =(1/(1−x))−1 ⇒f′(x)=Σ_(n=1) ^∞ nx^(n−1) =(1/((1−x)^2 )) ⇒Σ_(n=1) ^∞ n((1/2))^(n−1) =(1/((1−(1/2))^2 ))=4_#

$$\mathrm{A}\:\mathrm{more}\:\mathrm{alternative}\:\mathrm{ways}: \\ $$$$\:\:\:\:\:{f}\left({x}\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{x}^{{n}} =\frac{\mathrm{1}}{\mathrm{1}−{x}}−\mathrm{1} \\ $$$$\Rightarrow{f}'\left({x}\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{nx}^{{n}−\mathrm{1}} =\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)^{\mathrm{2}} } \\ $$$$\Rightarrow\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{n}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}−\mathrm{1}} =\frac{\mathrm{1}}{\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} }=\mathrm{4}_{#} \\ $$

Commented by aurpeyz last updated on 14/Dec/20

i like this thanks

$${i}\:{like}\:{this}\:{thanks} \\ $$

Answered by Dwaipayan Shikari last updated on 12/Dec/20

S=1+(2/2^1 )+(3/2^2 )+(4/2^3 )+... (S/2)= (1/2)+(2/2^2 )+(3/2^3 )+... S−(S/2)=1+(1/2)+(1/2^2 )+...⇒(S/2)=(1/(1−(1/2)))⇒S=4

$${S}=\mathrm{1}+\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{1}} }+\frac{\mathrm{3}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{4}}{\mathrm{2}^{\mathrm{3}} }+... \\ $$$$\frac{{S}}{\mathrm{2}}=\:\:\:\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{3}}{\mathrm{2}^{\mathrm{3}} }+... \\ $$$${S}−\frac{{S}}{\mathrm{2}}=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+...\Rightarrow\frac{{S}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}}\Rightarrow{S}=\mathrm{4} \\ $$

Commented by aurpeyz last updated on 12/Dec/20

pls explain line 3

$${pls}\:{explain}\:{line}\:\mathrm{3} \\ $$

Commented by Dwaipayan Shikari last updated on 12/Dec/20

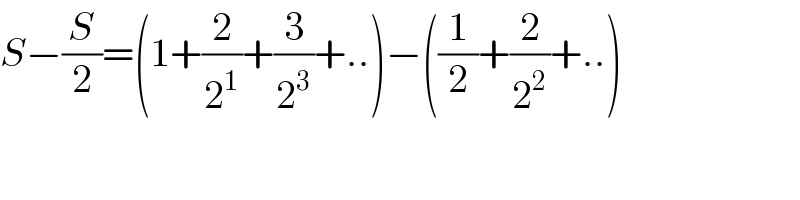
S−(S/2)=(1+(2/2^1 )+(3/2^3 )+..)−((1/2)+(2/2^2 )+..)

$${S}−\frac{{S}}{\mathrm{2}}=\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{1}} }+\frac{\mathrm{3}}{\mathrm{2}^{\mathrm{3}} }+..\right)−\left(\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{2}} }+..\right) \\ $$

Commented by aurpeyz last updated on 12/Dec/20

thanks

$${thanks} \\ $$

Answered by mathmax by abdo last updated on 12/Dec/20

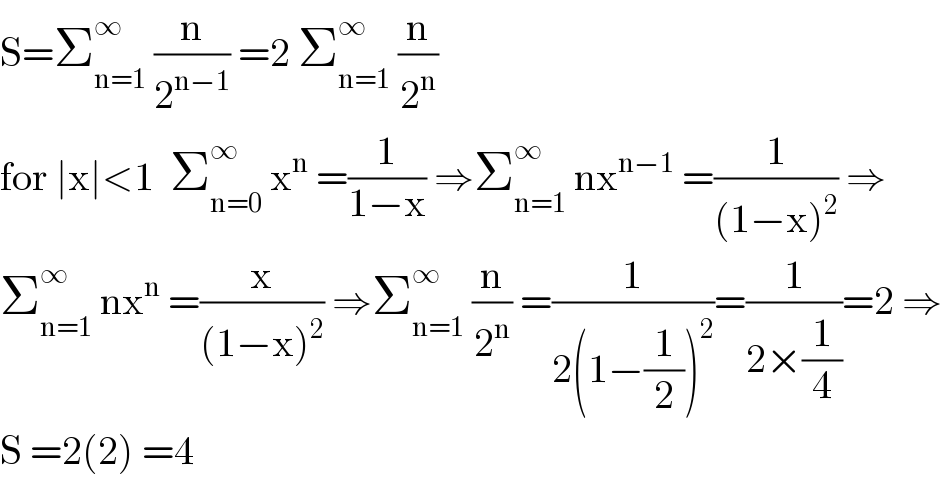
S=Σ_(n=1) ^∞ (n/2^(n−1) ) =2 Σ_(n=1) ^∞ (n/2^n ) for ∣x∣<1 Σ_(n=0) ^∞ x^n =(1/(1−x)) ⇒Σ_(n=1) ^∞ nx^(n−1) =(1/((1−x)^2 )) ⇒ Σ_(n=1) ^∞ nx^n =(x/((1−x)^2 )) ⇒Σ_(n=1) ^∞ (n/2^n ) =(1/(2(1−(1/2))^2 ))=(1/(2×(1/4)))=2 ⇒ S =2(2) =4

$$\mathrm{S}=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{n}}{\mathrm{2}^{\mathrm{n}−\mathrm{1}} }\:=\mathrm{2}\:\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{n}}{\mathrm{2}^{\mathrm{n}} } \\ $$$$\mathrm{for}\:\mid\mathrm{x}\mid<\mathrm{1}\:\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{x}^{\mathrm{n}} \:=\frac{\mathrm{1}}{\mathrm{1}−\mathrm{x}}\:\Rightarrow\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\mathrm{nx}^{\mathrm{n}−\mathrm{1}} \:=\frac{\mathrm{1}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{2}} }\:\Rightarrow \\ $$$$\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\mathrm{nx}^{\mathrm{n}} \:=\frac{\mathrm{x}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{2}} }\:\Rightarrow\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{n}}{\mathrm{2}^{\mathrm{n}} }\:=\frac{\mathrm{1}}{\mathrm{2}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}×\frac{\mathrm{1}}{\mathrm{4}}}=\mathrm{2}\:\Rightarrow \\ $$$$\mathrm{S}\:=\mathrm{2}\left(\mathrm{2}\right)\:=\mathrm{4} \\ $$

Commented by aurpeyz last updated on 13/Dec/20

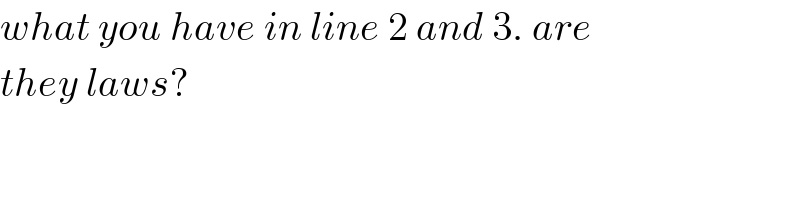
what you have in line 2 and 3. are they laws?

$${what}\:{you}\:{have}\:{in}\:{line}\:\mathrm{2}\:{and}\:\mathrm{3}.\:{are}\: \\ $$$${they}\:{laws}? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com