
Question and Answers Forum
Question Number 126383 by Lordose last updated on 20/Dec/20
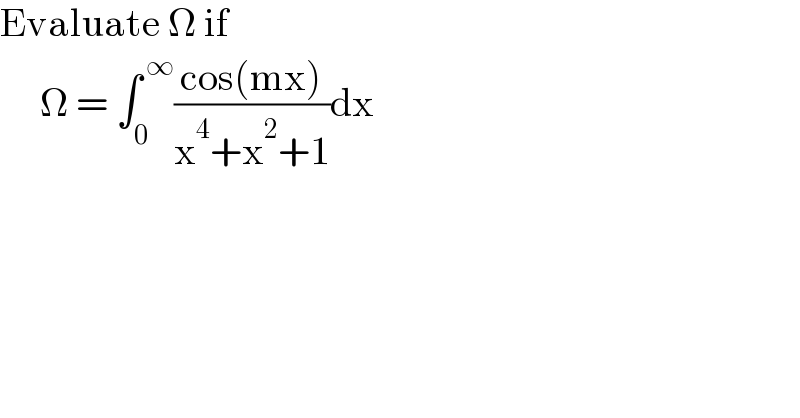
Answered by mathmax by abdo last updated on 20/Dec/20
![I =∫_0 ^∞ ((cos(mx))/(x^4 +x^2 +1))dx ⇒2I =∫_(−∞) ^(+∞) ((cos(mx))/(x^4 +x^2 +1))dx=Re(∫_(−∞) ^(+∞) (e^(imx) /(x^4 +x^2 +1))dx) let ϕ(z)=(e^(imz) /(z^4 +z^2 +1)) ]poles of ϕ? z^4 +z^2 +1=0 ⇒u^2 +u+1=0 (u=z^2 ) Δ=−3 ⇒u_1 =((−1+i(√3))/2)=e^((i2π)/3) and u_2 =((−1−i(√3))/2) =e^(−((i2π)/3)) ⇒ ϕ(z)=(e^(imz) /((z^2 −e^((i2π)/3) )(z^2 −e^(−((i2π)/3)) ))) =(e^(imz) /((z−e^((iπ)/3) )(z+e^((iπ)/3) )(z−e^(−((iπ)/3)) )(z+e^(−((iπ)/3)) ))) residus theorem give ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ{ Res(ϕ,e^((iπ)/3) )+Res(ϕ,−e^(−((iπ)/3)) )} Res(ϕ,e^((iπ)/3) ) =(e^(ime^((iπ)/3) ) /(2e^((iπ)/3) (2isin((π/3))))) =(1/(4i×((√3)/2))) e^(−((iπ)/3)) e^(im((1/2)+((i(√3))/2))) =(1/(2i(√3))) e^(i((m/2)−(π/3))) e^(−((√3)/2)m) Res(ϕ,−e^(−((iπ)/3)) ) =(e^(ime^(−((iπ)/3)) ) /(−2e^(−((iπ)/3)) (−2isin(((2π)/3))))) =(1/(2i(√3))) e^((iπ)/3) e^(im((1/2)−((i(√3))/2))) =(1/(2i(√3))) e^(i((m/2)+(π/3))) e^(((√3)/2)m) ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ×(1/(2i(√3))){ e^(−((√3)/2)m) e^(i((m/2)−(π/3))) +e^(((√3)/2)m) e^(i((m/2)+(π/3))) } =(π/( (√3))){e^(−((m(√3))/2)) (cos((m/2)−(π/3))+i sin((m/2)−(π/3))) +e^((m(√3))/2) ( cos((m/2)+(π/3))+isin((m/2)+(π/3)))} ⇒ I =(1/2)Re(∫...)=(π/( 2(√3))){ e^(−((m(√3))/2)) cos((m/2)−(π/3))+e^((m(√3))/(2 )) cos((m/2)+(π/3))}](Q126439.png)
| ||
Question and Answers Forum | ||
Question Number 126383 by Lordose last updated on 20/Dec/20 | ||
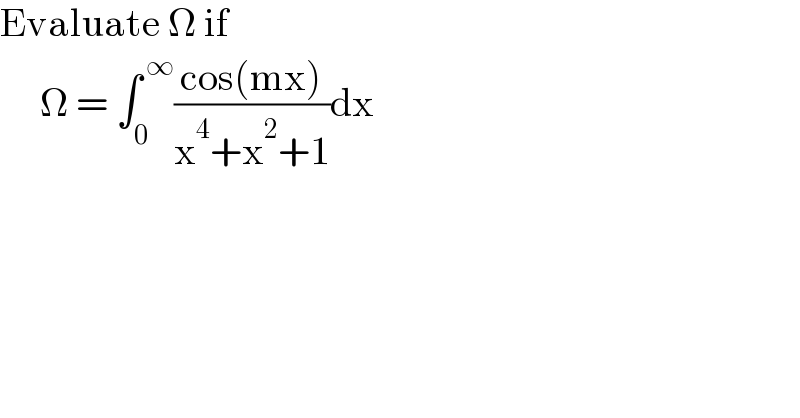 | ||
Answered by mathmax by abdo last updated on 20/Dec/20 | ||
![I =∫_0 ^∞ ((cos(mx))/(x^4 +x^2 +1))dx ⇒2I =∫_(−∞) ^(+∞) ((cos(mx))/(x^4 +x^2 +1))dx=Re(∫_(−∞) ^(+∞) (e^(imx) /(x^4 +x^2 +1))dx) let ϕ(z)=(e^(imz) /(z^4 +z^2 +1)) ]poles of ϕ? z^4 +z^2 +1=0 ⇒u^2 +u+1=0 (u=z^2 ) Δ=−3 ⇒u_1 =((−1+i(√3))/2)=e^((i2π)/3) and u_2 =((−1−i(√3))/2) =e^(−((i2π)/3)) ⇒ ϕ(z)=(e^(imz) /((z^2 −e^((i2π)/3) )(z^2 −e^(−((i2π)/3)) ))) =(e^(imz) /((z−e^((iπ)/3) )(z+e^((iπ)/3) )(z−e^(−((iπ)/3)) )(z+e^(−((iπ)/3)) ))) residus theorem give ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ{ Res(ϕ,e^((iπ)/3) )+Res(ϕ,−e^(−((iπ)/3)) )} Res(ϕ,e^((iπ)/3) ) =(e^(ime^((iπ)/3) ) /(2e^((iπ)/3) (2isin((π/3))))) =(1/(4i×((√3)/2))) e^(−((iπ)/3)) e^(im((1/2)+((i(√3))/2))) =(1/(2i(√3))) e^(i((m/2)−(π/3))) e^(−((√3)/2)m) Res(ϕ,−e^(−((iπ)/3)) ) =(e^(ime^(−((iπ)/3)) ) /(−2e^(−((iπ)/3)) (−2isin(((2π)/3))))) =(1/(2i(√3))) e^((iπ)/3) e^(im((1/2)−((i(√3))/2))) =(1/(2i(√3))) e^(i((m/2)+(π/3))) e^(((√3)/2)m) ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ×(1/(2i(√3))){ e^(−((√3)/2)m) e^(i((m/2)−(π/3))) +e^(((√3)/2)m) e^(i((m/2)+(π/3))) } =(π/( (√3))){e^(−((m(√3))/2)) (cos((m/2)−(π/3))+i sin((m/2)−(π/3))) +e^((m(√3))/2) ( cos((m/2)+(π/3))+isin((m/2)+(π/3)))} ⇒ I =(1/2)Re(∫...)=(π/( 2(√3))){ e^(−((m(√3))/2)) cos((m/2)−(π/3))+e^((m(√3))/(2 )) cos((m/2)+(π/3))}](Q126439.png) | ||
| ||