Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 127090 by physicstutes last updated on 26/Dec/20

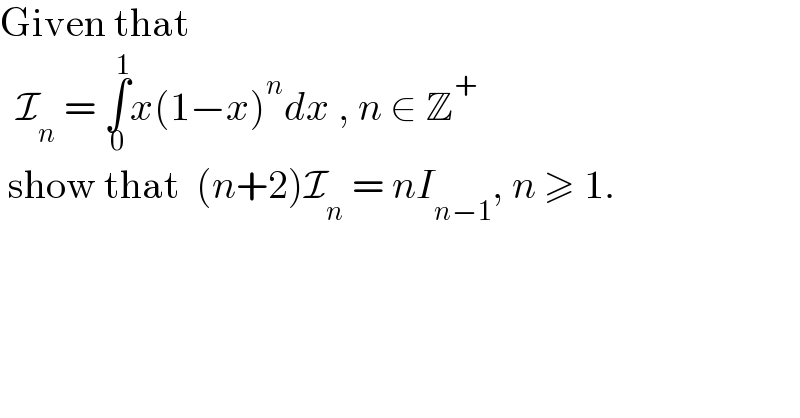
Given that I_n = ∫_0 ^1 x(1−x)^n dx , n ∈ Z^+ show that (n+2)I_n = nI_(n−1) , n ≥ 1.

$$\mathrm{Given}\:\mathrm{that}\: \\ $$$$\:\:\mathcal{I}_{{n}} \:=\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}{x}\left(\mathrm{1}−{x}\right)^{{n}} {dx}\:,\:{n}\:\in\:\mathbb{Z}^{+} \\ $$$$\:\mathrm{show}\:\mathrm{that}\:\:\left({n}+\mathrm{2}\right)\mathcal{I}_{{n}} \:=\:{nI}_{{n}−\mathrm{1}} ,\:{n}\:\geqslant\:\mathrm{1}. \\ $$

Answered by Dwaipayan Shikari last updated on 26/Dec/20

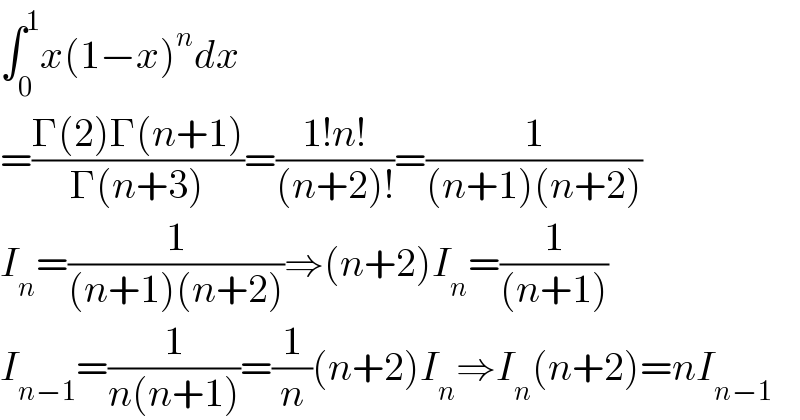
∫_0 ^1 x(1−x)^n dx =((Γ(2)Γ(n+1))/(Γ(n+3)))=((1!n!)/((n+2)!))=(1/((n+1)(n+2))) I_n =(1/((n+1)(n+2)))⇒(n+2)I_n =(1/((n+1))) I_(n−1) =(1/(n(n+1)))=(1/n)(n+2)I_n ⇒I_n (n+2)=nI_(n−1)

$$\int_{\mathrm{0}} ^{\mathrm{1}} {x}\left(\mathrm{1}−{x}\right)^{{n}} {dx} \\ $$$$=\frac{\Gamma\left(\mathrm{2}\right)\Gamma\left({n}+\mathrm{1}\right)}{\Gamma\left({n}+\mathrm{3}\right)}=\frac{\mathrm{1}!{n}!}{\left({n}+\mathrm{2}\right)!}=\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)} \\ $$$${I}_{{n}} =\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)}\Rightarrow\left({n}+\mathrm{2}\right){I}_{{n}} =\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)} \\ $$$${I}_{{n}−\mathrm{1}} =\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)}=\frac{\mathrm{1}}{{n}}\left({n}+\mathrm{2}\right){I}_{{n}} \Rightarrow{I}_{{n}} \left({n}+\mathrm{2}\right)={nI}_{{n}−\mathrm{1}} \\ $$

Answered by Olaf last updated on 26/Dec/20

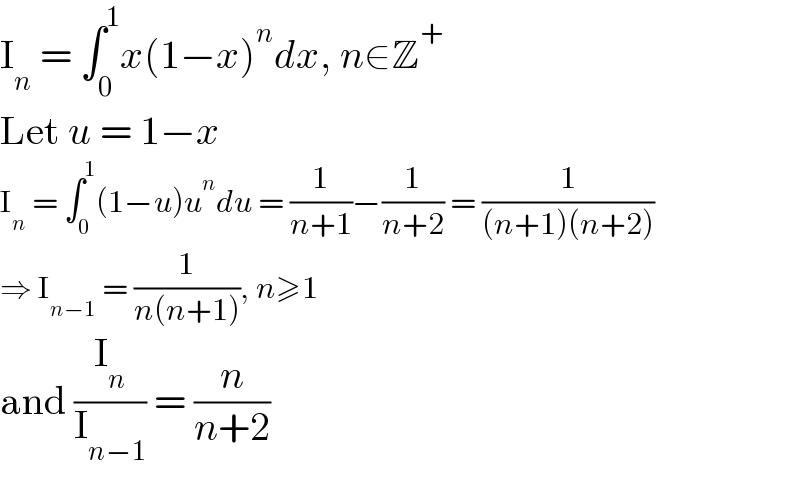
I_n = ∫_0 ^1 x(1−x)^n dx, n∈Z^+ Let u = 1−x I_n = ∫_0 ^1 (1−u)u^n du = (1/(n+1))−(1/(n+2)) = (1/((n+1)(n+2))) ⇒ I_(n−1) = (1/(n(n+1))), n≥1 and (I_n /I_(n−1) ) = (n/(n+2))

$$\mathrm{I}_{{n}} \:=\:\int_{\mathrm{0}} ^{\mathrm{1}} {x}\left(\mathrm{1}−{x}\right)^{{n}} {dx},\:{n}\in\mathbb{Z}^{+} \\ $$$$\mathrm{Let}\:{u}\:=\:\mathrm{1}−{x} \\ $$$$\mathrm{I}_{{n}} \:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}−{u}\right){u}^{{n}} {du}\:=\:\frac{\mathrm{1}}{{n}+\mathrm{1}}−\frac{\mathrm{1}}{{n}+\mathrm{2}}\:=\:\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)} \\ $$$$\Rightarrow\:\mathrm{I}_{{n}−\mathrm{1}} \:=\:\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)},\:{n}\geqslant\mathrm{1} \\ $$$$\mathrm{and}\:\frac{\mathrm{I}_{{n}} }{\mathrm{I}_{{n}−\mathrm{1}} }\:=\:\frac{{n}}{{n}+\mathrm{2}} \\ $$

Commented by physicstutes last updated on 26/Dec/20

thanks sirs, but how about a method using integration by parts by letting u = x and dv = (1−x)^n dx

$$\mathrm{thanks}\:\mathrm{sirs},\:\mathrm{but}\:\mathrm{how}\:\mathrm{about}\:\mathrm{a}\:\mathrm{method}\:\mathrm{using} \\ $$$$\mathrm{integration}\:\mathrm{by}\:\mathrm{parts} \\ $$$$\mathrm{by}\:\mathrm{letting}\:{u}\:=\:{x}\:\mathrm{and}\:{dv}\:=\:\left(\mathrm{1}−{x}\right)^{{n}} {dx} \\ $$

Answered by Ar Brandon last updated on 26/Dec/20

I_n =∫_0 ^1 x(1−x)^n dx, n∈Z^+ { ((u(x)=x)),((v′(x)=(1−x)^n )) :} ⇒ { ((u′(x)=1)),((v(x)=−(((1−x)^(n+1) )/(n+1)))) :} I_n =u(x)∙v(x)−∫v(x)∙u′(x)dx =[−((x(1−x)^(n+1) )/(n+1))]_0 ^1 +∫_0 ^1 (((1−x)^(n+1) )/(n+1))dx =−[(((1−x)^(n+2) )/((n+1)(n+2)))]_0 ^1 =(1/((n+1)(n+2))) I_(n−1) =(1/(n(n+1))) ⇒ (I_n /I_(n−1) )=(n/(n+2)) (n+2)I_n =nI_(n−1)

$$\mathcal{I}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}} \mathrm{dx},\:\mathrm{n}\in\mathbb{Z}^{+} \\ $$$$\begin{cases}{\mathrm{u}\left(\mathrm{x}\right)=\mathrm{x}}\\{\mathrm{v}'\left(\mathrm{x}\right)=\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}} }\end{cases}\:\Rightarrow\:\begin{cases}{\mathrm{u}'\left(\mathrm{x}\right)=\mathrm{1}}\\{\mathrm{v}\left(\mathrm{x}\right)=−\frac{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}+\mathrm{1}}}\end{cases} \\ $$$$\mathcal{I}_{\mathrm{n}} =\mathrm{u}\left(\mathrm{x}\right)\centerdot\mathrm{v}\left(\mathrm{x}\right)−\int\mathrm{v}\left(\mathrm{x}\right)\centerdot\mathrm{u}'\left(\mathrm{x}\right)\mathrm{dx} \\ $$$$\:\:\:\:\:=\left[−\frac{\mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}+\mathrm{1}}\right]_{\mathrm{0}} ^{\mathrm{1}} +\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}+\mathrm{1}}\mathrm{dx} \\ $$$$\:\:\:\:\:=−\left[\frac{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}+\mathrm{2}} }{\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{n}+\mathrm{2}\right)}\right]_{\mathrm{0}} ^{\mathrm{1}} =\frac{\mathrm{1}}{\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{n}+\mathrm{2}\right)} \\ $$$$\mathcal{I}_{\mathrm{n}−\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)}\:\Rightarrow\:\frac{\mathcal{I}_{\mathrm{n}} }{\mathcal{I}_{\mathrm{n}−\mathrm{1}} }=\frac{\mathrm{n}}{\mathrm{n}+\mathrm{2}} \\ $$$$\left(\mathrm{n}+\mathrm{2}\right)\mathcal{I}_{\mathrm{n}} =\mathrm{n}\mathcal{I}_{\mathrm{n}−\mathrm{1}} \\ $$

Commented by Ar Brandon last updated on 27/Dec/20

De nada !

$${De}\:{nada}\:! \\ $$

Commented by physicstutes last updated on 27/Dec/20

excellento! gracias

$$\mathrm{excellento}!\:\mathrm{gracias} \\ $$

Answered by Ar Brandon last updated on 26/Dec/20

I_n =∫_0 ^1 x(1−x)^n dx, n∈Z^+ { ((u(x)=(1−x)^n )),((v′(x)=x)) :} ⇒ { ((u′(x)=−n(1−x)^(n−1) )),((v(x)=(x^2 /2))) :} I_n =[(x^2 /2)∙(1−x)^n ]_0 ^1 +(n/2)∫_0 ^1 x^2 (1−x)^(n−1) dx =(n/2)∫_0 ^1 {−x(1−x−1)(1−x)^(n−1) dx =(n/2)∫_0 ^1 {−x(1−x)(1−x)^(n−1) +x(1−x)^(n−1) }dx =(n/2)∫_0 ^1 {−x(1−x)^n +x(1−x)^(n−1) }dx =(n/2){−I_n +I_(n−1) } ⇒ (1+(n/2))I_n =(n/2)I_(n−1) (n+2)I_n =nI_(n−1)

$$\mathcal{I}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}} \mathrm{dx},\:\mathrm{n}\in\mathbb{Z}^{+} \\ $$$$\begin{cases}{\mathrm{u}\left(\mathrm{x}\right)=\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}} }\\{\mathrm{v}'\left(\mathrm{x}\right)=\mathrm{x}}\end{cases}\:\Rightarrow\:\begin{cases}{\mathrm{u}'\left(\mathrm{x}\right)=−\mathrm{n}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}−\mathrm{1}} }\\{\mathrm{v}\left(\mathrm{x}\right)=\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}}\end{cases} \\ $$$$\mathcal{I}_{\mathrm{n}} =\left[\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\centerdot\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}} \right]_{\mathrm{0}} ^{\mathrm{1}} +\frac{\mathrm{n}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{2}} \left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}−\mathrm{1}} \mathrm{dx} \\ $$$$\:\:\:\:\:=\frac{\mathrm{n}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left\{−\mathrm{x}\left(\mathrm{1}−\mathrm{x}−\mathrm{1}\right)\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}−\mathrm{1}} \mathrm{dx}\right. \\ $$$$\:\:\:\:\:=\frac{\mathrm{n}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left\{−\mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}−\mathrm{1}} +\mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}−\mathrm{1}} \right\}\mathrm{dx} \\ $$$$\:\:\:\:\:=\frac{\mathrm{n}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left\{−\mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}} +\mathrm{x}\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{n}−\mathrm{1}} \right\}\mathrm{dx} \\ $$$$\:\:\:\:\:=\frac{\mathrm{n}}{\mathrm{2}}\left\{−\mathcal{I}_{\mathrm{n}} +\mathcal{I}_{\mathrm{n}−\mathrm{1}} \right\}\:\Rightarrow\:\left(\mathrm{1}+\frac{\mathrm{n}}{\mathrm{2}}\right)\mathcal{I}_{\mathrm{n}} =\frac{\mathrm{n}}{\mathrm{2}}\mathcal{I}_{\mathrm{n}−\mathrm{1}} \\ $$$$\left(\mathrm{n}+\mathrm{2}\right)\mathcal{I}_{\mathrm{n}} =\mathrm{n}\mathcal{I}_{\mathrm{n}−\mathrm{1}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com