prove the convergence of (α_n )_(n ) such that tan^(−1) ((α_n /n))−2α


Question and Answers Forum

All Questions Topic List
Limits Questions
Previous in All Question Next in All Question
Previous in Limits Next in Limits
Question Number 127501 by slahadjb last updated on 30/Dec/20

$p r o v e t h e c o n v e r g e n c e o f {(α_{n})}_{n} s u c h t h a t$ $\tan^{- 1} (\frac{α_{n}}{n}) - 2 α_{n} + 1 = 0$
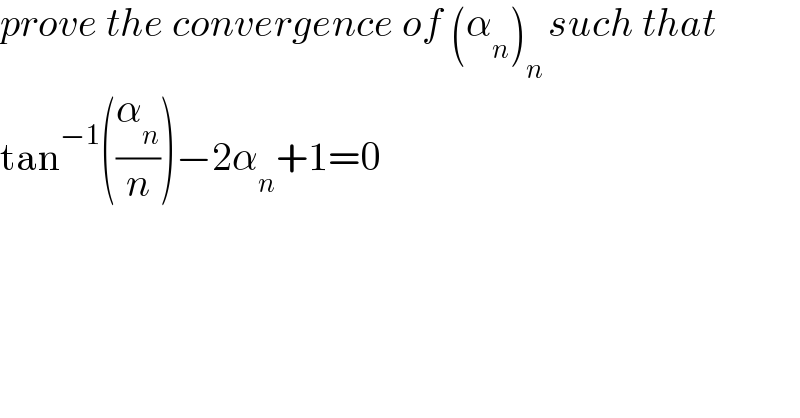
	Answered by mindispower last updated on 31/Dec/20

	$\Rightarrow 2 a_{n} = 1 + {t a n}^{-} (\frac{a_{n}}{n}) \Rightarrow a_{n} \in [- \frac{π}{4} + \frac{1}{2}, \frac{π}{4} + \frac{1}{2}]$ $b o u n d e d \Rightarrow \lim_{n \to \infty} \frac{a_{n}}{n} \to 0$ $\Rightarrow {t a n}^{-} (\frac{α_{n}}{n}) + 1 \Rightarrow 1 s i n c e g : x \to \tan^{- 1} (x) + 1 i s c o n t i n u s$ $2 a_{n} = g (\frac{a_{n}}{n}), 2 a_{n} \to g (0) = 1$ $a_{n} \to \frac{1}{2}$
	Commented by slahadjb last updated on 31/Dec/20

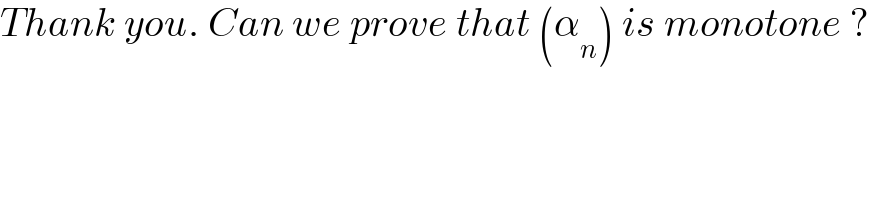
	$T h a n k y o u . C a n w e p r o v e t h a t (α_{n}) i s m o n o t o n e ?$
	Commented by mindispower last updated on 31/Dec/20

	$2 a_{n} = 1 + {t a n}^{-} (\frac{a_{n}}{n})$ $1 = 2 a_{n} - {t a n}^{-} (\frac{a n}{n})$ $f (x) = 2 x - {t a n}^{-} (\frac{x}{n}),$ $2 a_{n} - {t a n}^{-} (\frac{a_{n}}{n + 1}) = 1 + {t a n}^{-} (\frac{a_{n}}{n}) - {t a n}^{-} (\frac{a_{n}}{n + 1}) > 1$ $a_{n} > 0, \forall n ⩾ 4$ $⩾ 2 a_{n + 1} - {t a n}^{-} (\frac{a_{n + 1}}{n + 1}) = f (a_{n + 1})$ $f (a_{n}) ⩾ f (a_{n + 1}) \Rightarrow a_{n} > a_{n + 1} n > 4, c a u s e f i s i n c r e a s e$
	Commented by slahadjb last updated on 31/Dec/20

	$t h a n k y o u s o m u c h .$
	Commented by mindispower last updated on 31/Dec/20

	$p l e a s u r$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum