
Question and Answers Forum
Question Number 127982 by Dwaipayan Shikari last updated on 03/Jan/21

Commented by Dwaipayan Shikari last updated on 03/Jan/21
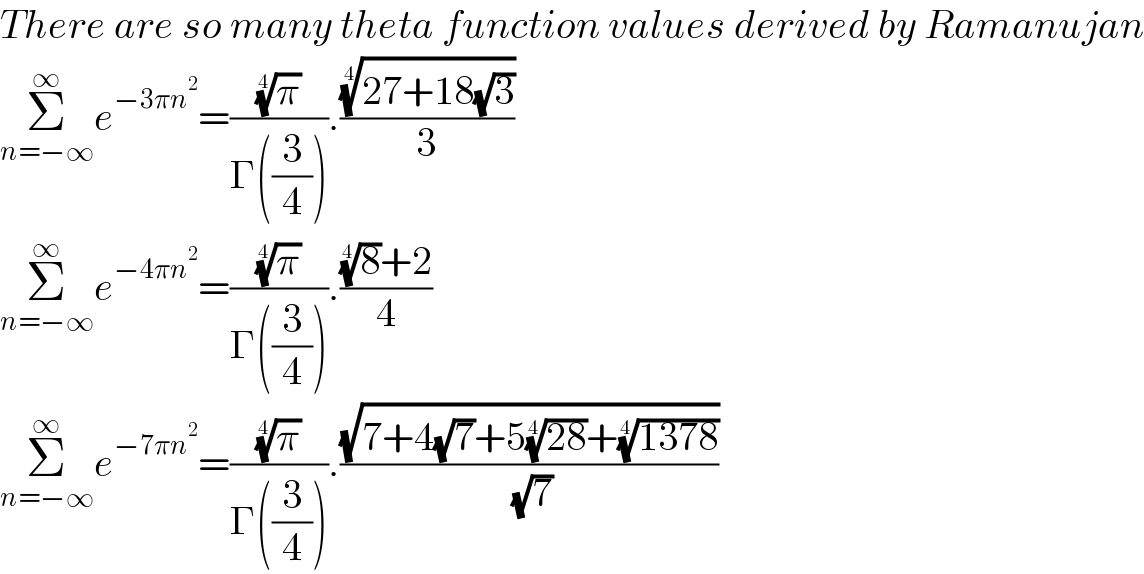
| ||
Question and Answers Forum | ||
Question Number 127982 by Dwaipayan Shikari last updated on 03/Jan/21 | ||
 | ||
Commented by Dwaipayan Shikari last updated on 03/Jan/21 | ||
 | ||